The Mathematics of demand functions

1. The Mathematics of Demand Functions

P
The Mathematics of Demand
Functions
D
Q
Lectures in Microeconomics-Charles W. Upton

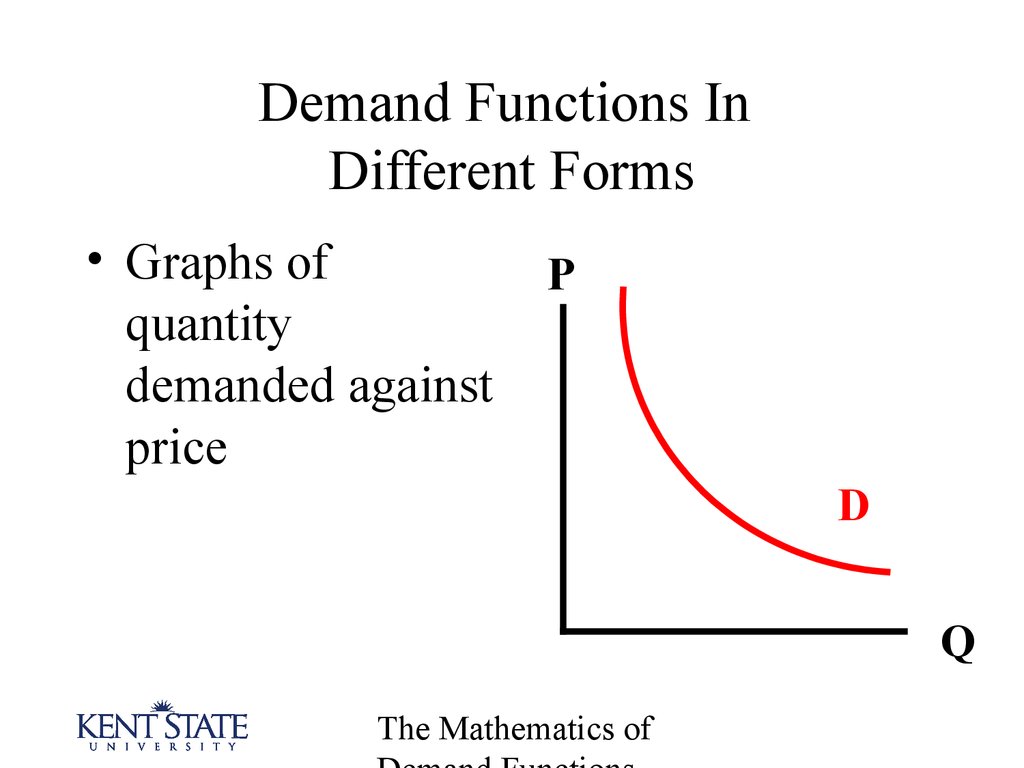
2. Demand Functions In Different Forms

• Graphs of
quantity
demanded against
price
P
D
Q
The Mathematics of

3. Demand Functions In Different Forms

• Graphs of
quantity
demanded against
price
P
– They need not be
straight lines
D
Q
The Mathematics of

4. Demand Functions In Different Forms

• Tables
Price
Quantity
$0.40
100
$0.50
90
$0.60
80
The Mathematics of

5. Demand Functions In Different Forms

• Mathematical
equations
Q=100-2P
The Mathematics of

6. Demand Functions In Different Forms

• Mathematical
equations
– Linear or Non
Linear
Q=100-2P
Q=10P-2
The Mathematics of

7. Indirect demand functions

• Gives price as a function of quantity, not the
other way around.
• We can always restate indirect demand
functions as direct demand functions and
vice versa.
The Mathematics of

8. A Graphical Interpretation

• Knowing price
we know quantity
demanded.
P
D
Q
The Mathematics of

9. A Graphical Interpretation

• It also works the
other way.
P
D
Q
The Mathematics of

10. Ditto with Tables

Price
Quantity
$0.40
100
$0.50
90
$0.60
80
The Mathematics of

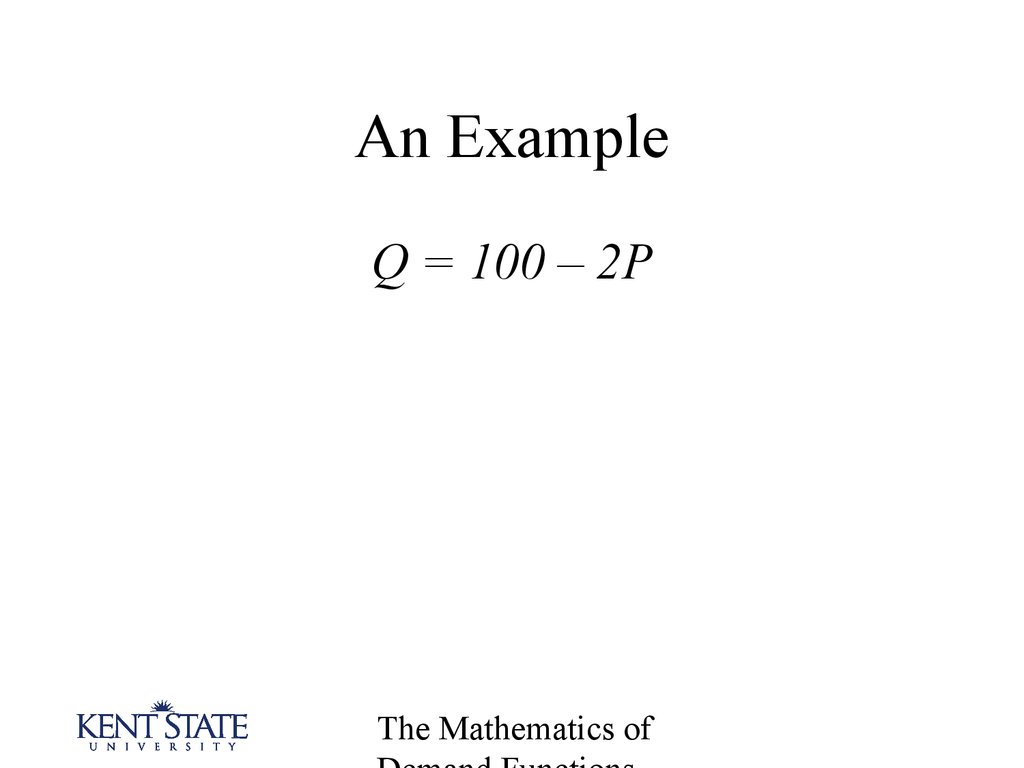
11. An Example

Q = 100 – 2P
The Mathematics of

12. An Example

Q = 100 – 2P
2P + Q = 100 – 2P +2P
The Mathematics of

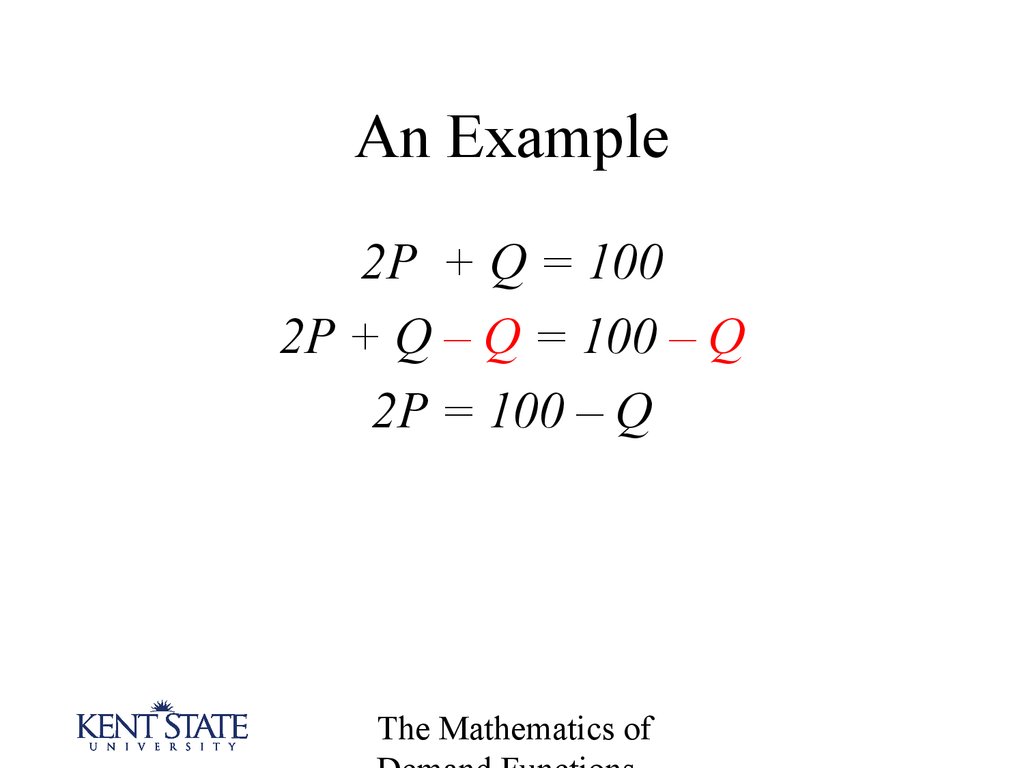
13. An Example

Q = 100 – 2P
2P + Q = 100 – 2P +2P
2P + Q = 100
The Mathematics of

14. An Example

2P + Q = 100
2P + Q – Q = 100 – Q
2P = 100 – Q
The Mathematics of

15. An Example

2P = 100 – Q
(1/2)[2P] = (1/2)[100-Q]
P = 50 – (1/2)Q
The Mathematics of

16. A Second Example

P = 50 – (1/2)Q
The Mathematics of

17. A Second Example

P = 50 – (1/2)Q
2P = 2[50-(1/2)Q]
2P = 100 – Q
The Mathematics of

18. A Second Example

2P = 100 – Q
Q + 2P = 100 – Q + Q
Q + 2P = 100
The Mathematics of

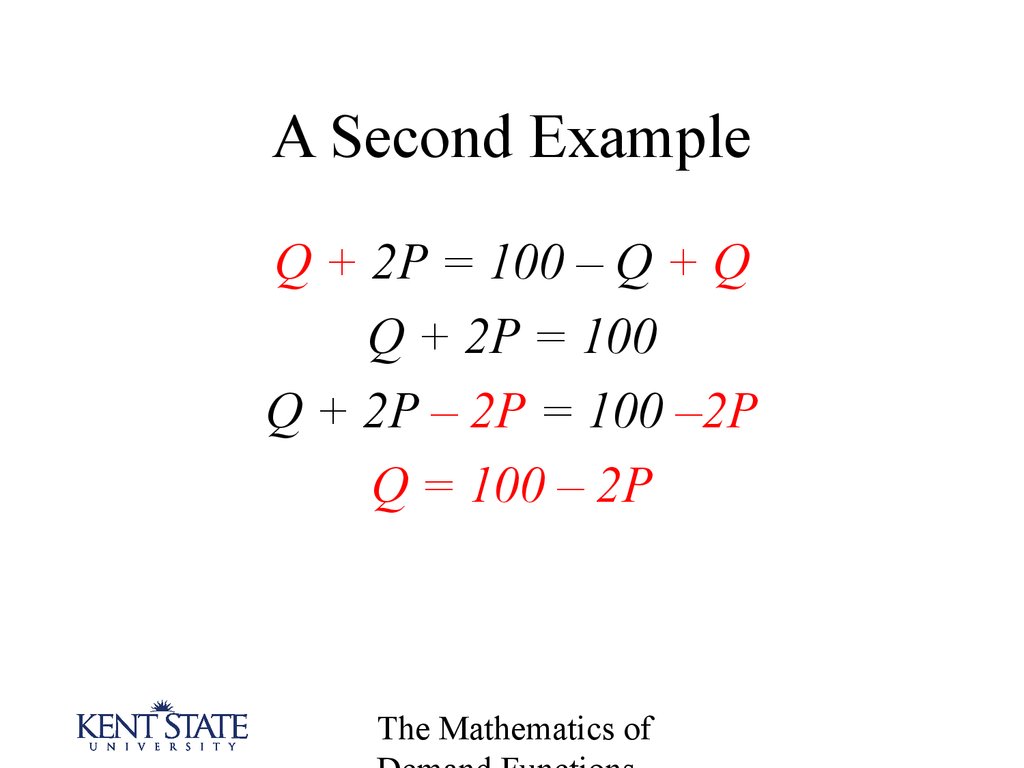
19. A Second Example

Q + 2P = 100 – Q + Q
Q + 2P = 100
Q + 2P – 2P = 100 –2P
Q = 100 – 2P
The Mathematics of

20. Some Assignments

P = 12 – 3Q
Q = 100 – 10P
The Mathematics of

21. Some Assignments

P = 12 – 3Q
Q = 4 – (1/3)P
Q = 100 – 10P
P = 10 – 0.1Q
The Mathematics of

22. End

©2004 Charles
W. Upton
The Mathematics of