Похожие презентации:
Recording Notice
1.
Recording NoticeThis lesson is
being recorded
2.
King’s Interhigh Logo3.
Learn 1: BuoyancyMaterials – Viscosity
4.
ObjectivesBe able to use the equation for density:
ρ=m/V
buoyancy = upthrust (weight of fluid displaced) - weight of
object.
5.
Page ReferenceUK pages 112-118
SLIDE:
5
International pages 68-70
6.
StarterIn what sense do objects ‘float’ in low
Earth orbit?
• There are similarities to floating in
water for certain objects.
• There is no apparent resultant force
in both cases but for different
reasons.
Space (apparent relative to craft)
0–0=0N
Earth 10 – 10 = 0 N
SLIDE:
6
7.
Buoyancy• On the next slide we will
see a list of the four
forces we know of.
• Some of the
consequences are listed.
• Upthrust is not a
fundamental force.
• It is the result of two
others working together –
gravity and electrostatic
SLIDE:
7
8.
Four ForcesGravitational (strong × 10-38)
• falling (weight)
• orbits
• planet formation
• star evolution
Electrostatic (strong × 10-2)
Contact:
friction
muscular
Non contact:
static
magnetism
Weak (strong × 10-13)
Nuclear decay
Strong
Atomic structure, fusion, fission
SLIDE:
8
Upthrust
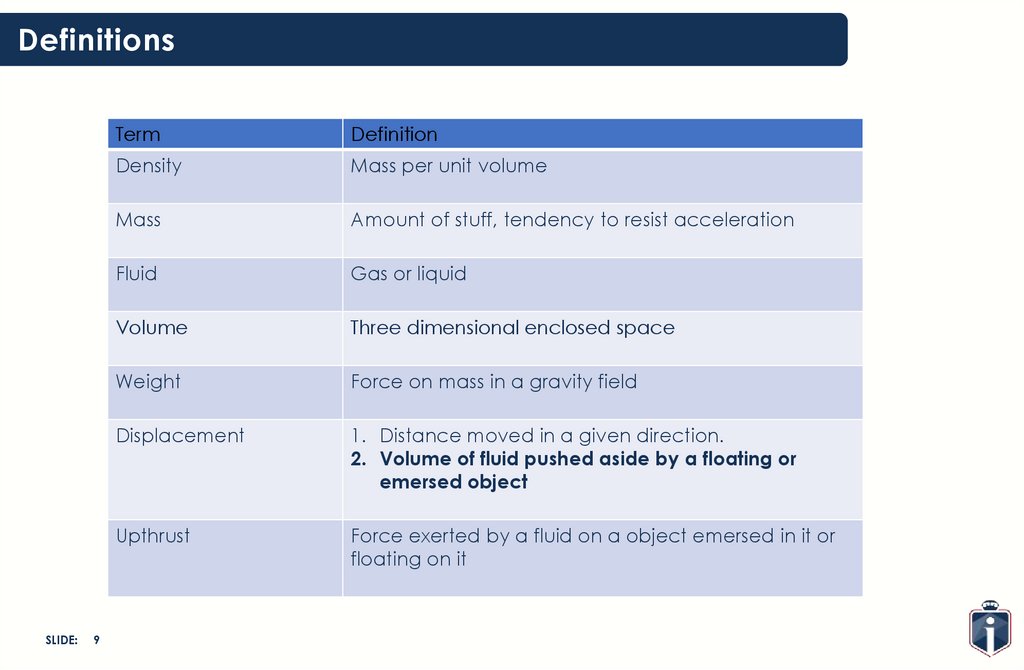
9.
DefinitionsSLIDE:
9
Term
Definition
Density
Mass per unit volume
Mass
Amount of stuff, tendency to resist acceleration
Fluid
Gas or liquid
Volume
Three dimensional enclosed space
Weight
Force on mass in a gravity field
Displacement
1. Distance moved in a given direction.
2. Volume of fluid pushed aside by a floating or
emersed object
Upthrust
Force exerted by a fluid on a object emersed in it or
floating on it
10.
Eureka• Archimedes principle:
The upward force on a
submerged or partially
submerged object equals the
weight of the fluid displaced.
• But why is this so?
SLIDE:
10
11.
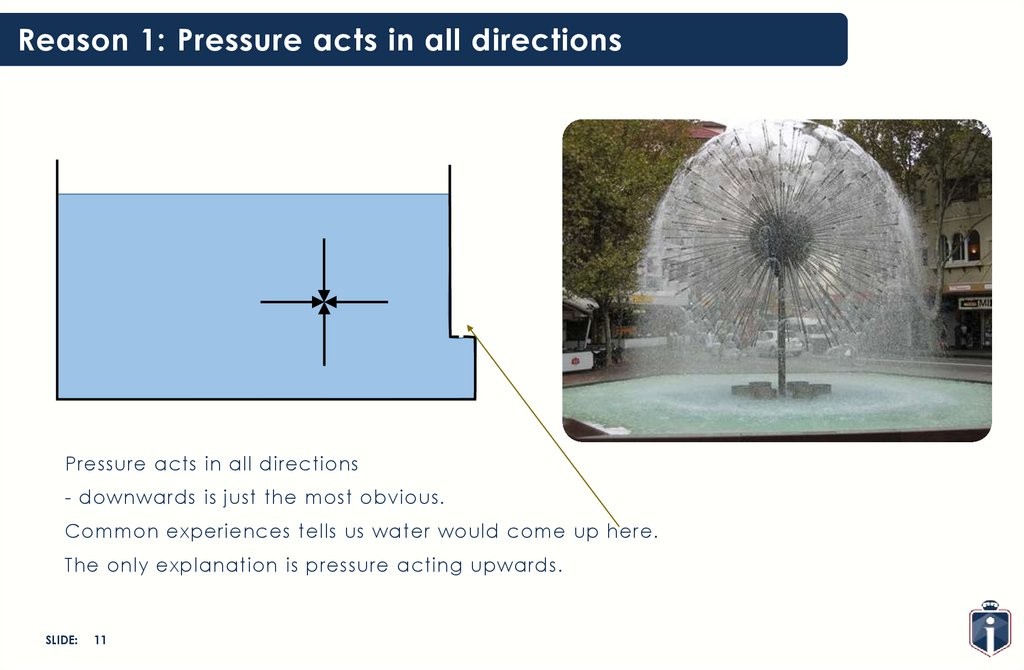
Reason 1: Pressure acts in all directionsPressure acts in all directions
- downwards is just the most obvious.
Common experiences tells us water would come up here.
The only explanation is pressure acting upwards.
SLIDE:
11
12.
Reason 2…..Which bubbles contain most air?
• The bubbles contain roughly the
same mass of air.
ΔP = hρg
• Their volume increases because the
pressure decreases as they rise
(Reason 2).
ΔP = hρg
SLIDE:
12
13.
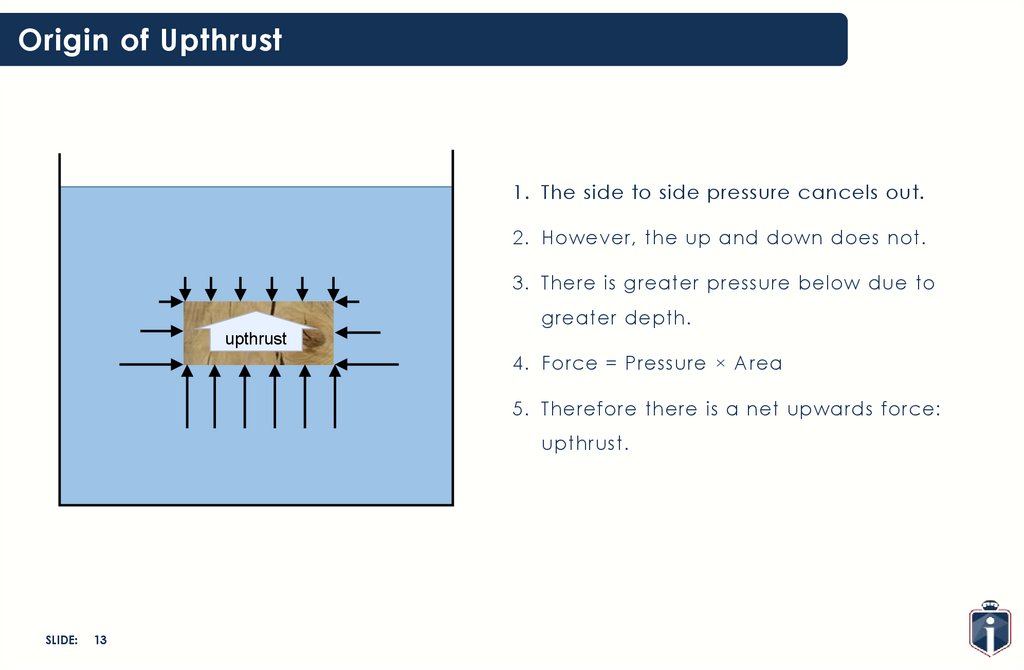
Origin of Upthrust1. The side to side pressure cancels out.
2. However, the up and down does not.
3. There is greater pressure below due to
greater depth.
upthrust
4. Force = Pressure × Area
5. Therefore there is a net upwards force:
upthrust.
SLIDE:
13
14.
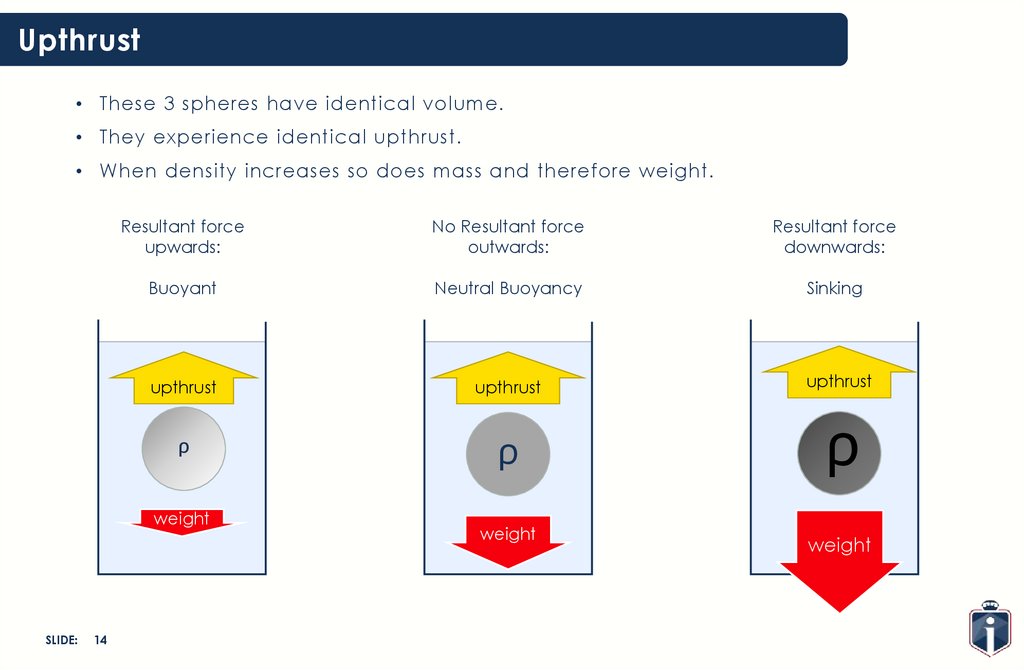
Upthrust• These 3 spheres have identical volume.
• They experience identical upthrust.
• When density increases so does mass and therefore weight.
Resultant force
upwards:
No Resultant force
outwards:
Resultant force
downwards:
Buoyant
Neutral Buoyancy
Sinking
upthrust
upthrust
upthrust
ρ
ρ
ρ
weight
SLIDE:
14
weight
weight
15.
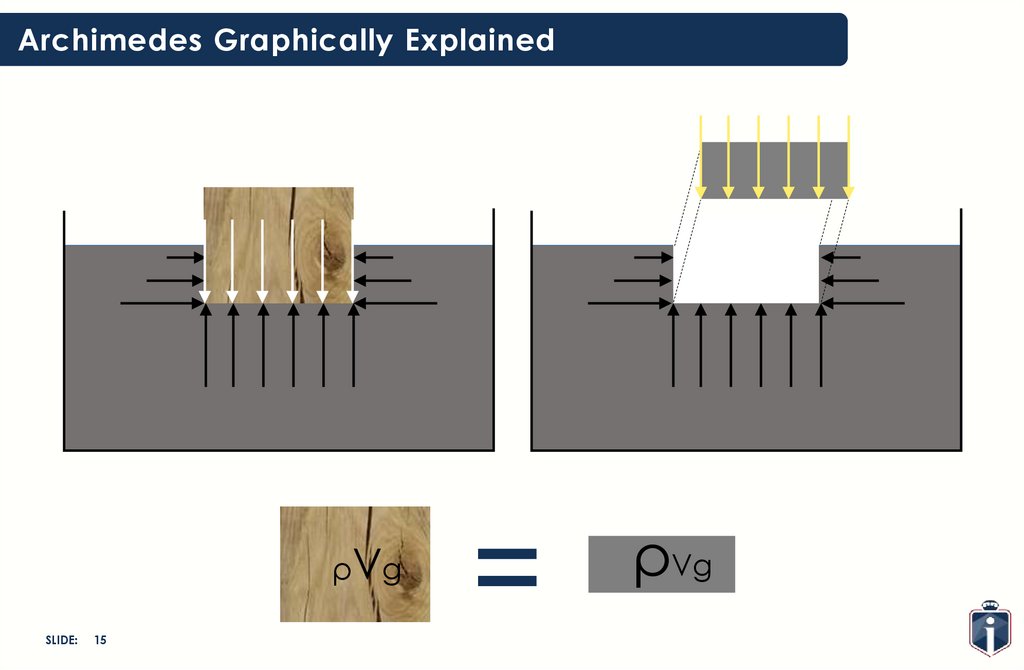
Archimedes Graphically ExplainedρVg
SLIDE:
15
=
ρVg
16.
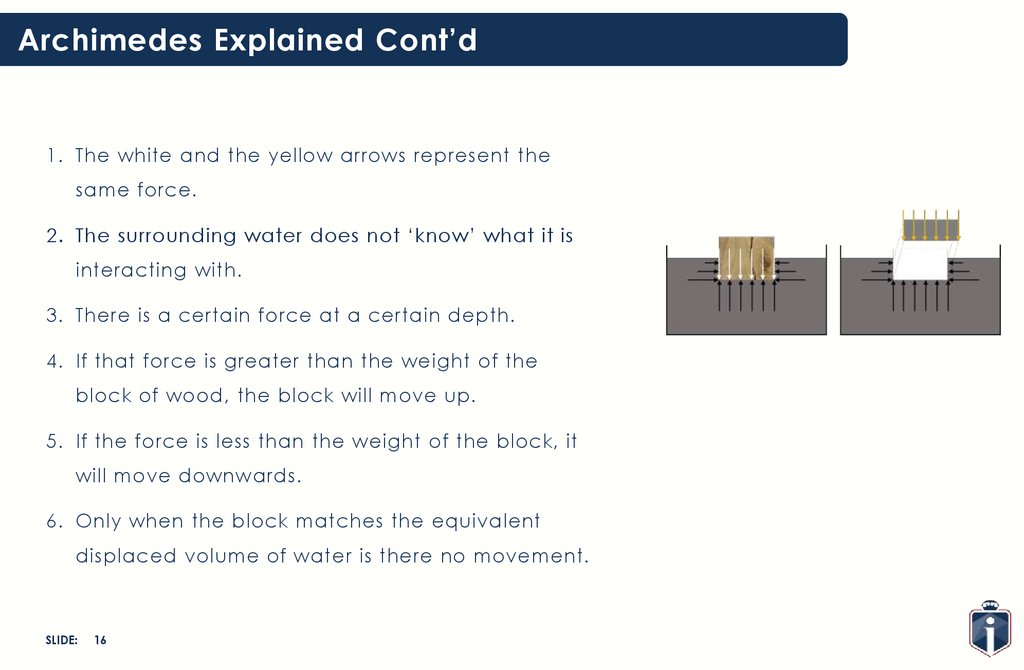
Archimedes Explained Cont’d1. The white and the yellow arrows represent the
same force.
2. The surrounding water does not ‘know’ what it is
interacting with.
3. There is a certain force at a certain depth.
4. If that force is greater than the weight of the
block of wood, the block will move up.
5. If the force is less than the weight of the block, it
will move downwards.
6. Only when the block matches the equivalent
displaced volume of water is there no movement.
SLIDE:
16
17.
Plenary (Answer in Learn 2)A rock is moved from a boat to the bottom of a lake.
What will happen to the water level? Why?
A. Falls
B. Rises
C. Nothing
SLIDE:
17
18.
Learn 2: Viscous DragMaterials – Viscosity
19.
ObjectivesBe able to use the equation for viscous drag (Stokes’s Law):
F = 6πηrv
Understand that this equation applies only to small spherical
objects moving at low speeds with laminar flow (or in the
absence of turbulent flow)…
…and that viscosity is temperature dependent.
SLIDE:
19
20.
Starter (Carried from Learn 1)A rock is moved from a boat to the bottom of a lake.
What will happen to the water level? Why?
A. Falls
B. Rises
C. Nothing
SLIDE:
20
21.
Starter: Using Extreme CasesPhysics Girl: Can you solve the boat puzzle? 4.51 min
What does she mean by:
Extreme case scenario
Why is it useful?
SLIDE:
21
22.
Starter: Numerical ExampleIn Boat
No Rock
In Boat
In Lake
Mass (kg)
10
10 but irrelevant
Volume (dm3)
1 but irrelevant
1
Displacement (dm3)
10
1
Answer: A, water level falls
SLIDE:
22
In Lake
23.
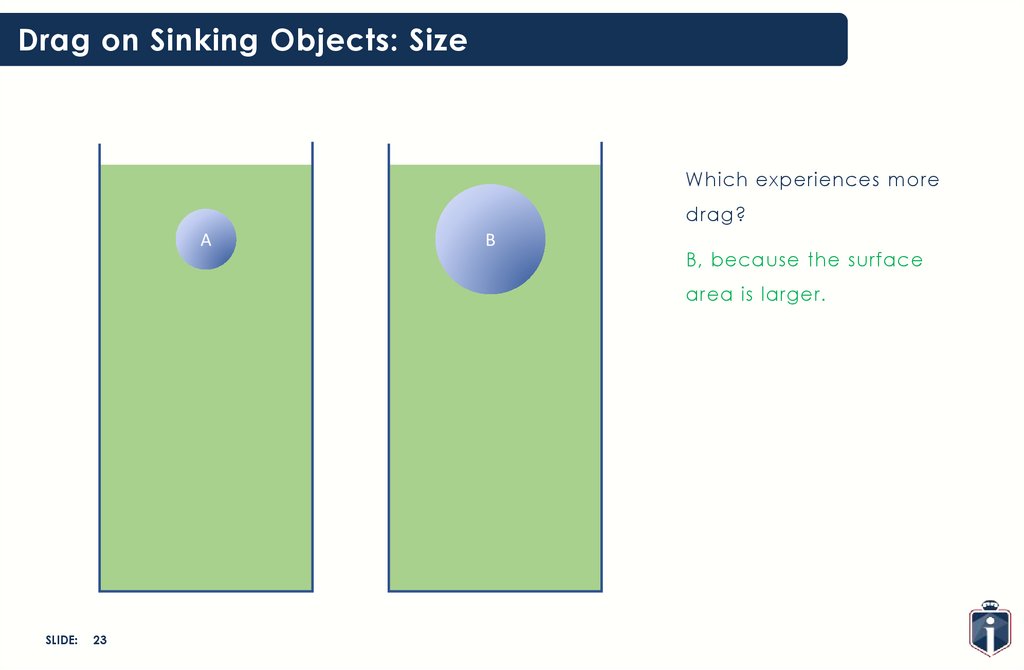
Drag on Sinking Objects: SizeWhich experiences more
drag?
A
B
B, because the surface
area is larger.
SLIDE:
23
24.
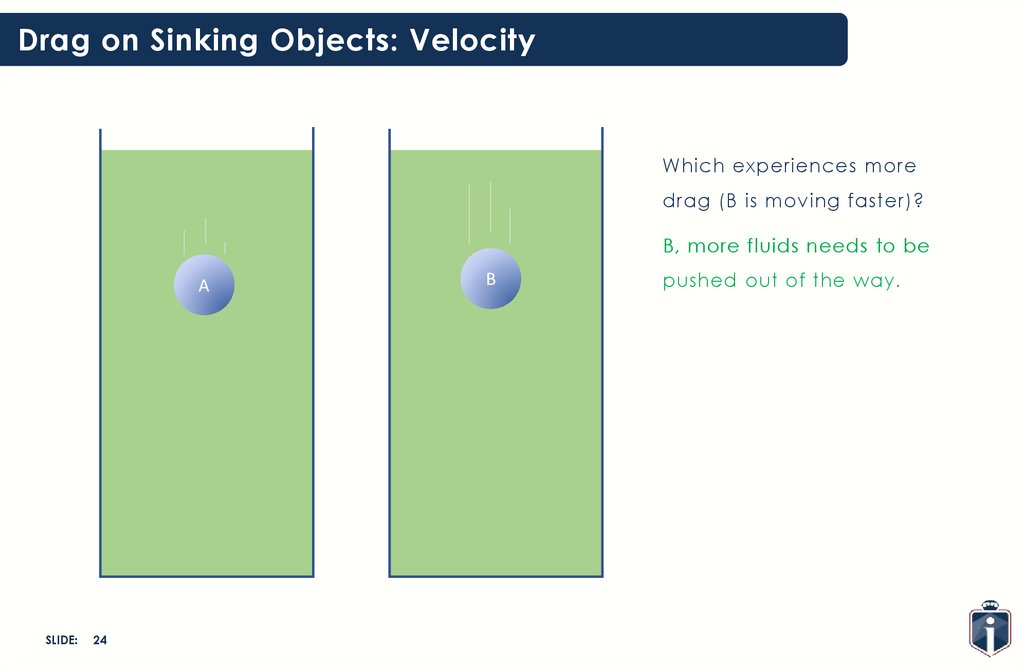
Drag on Sinking Objects: VelocityWhich experiences more
drag (B is moving faster)?
B, more fluids needs to be
A
SLIDE:
24
B
pushed out of the way.
25.
Drag on Sinking Objects: GloopinessWhich experiences more drag (B
is the most gloopy)?
B, more force is needed to push
A
SLIDE:
25
B
the fluid out of the way.
26.
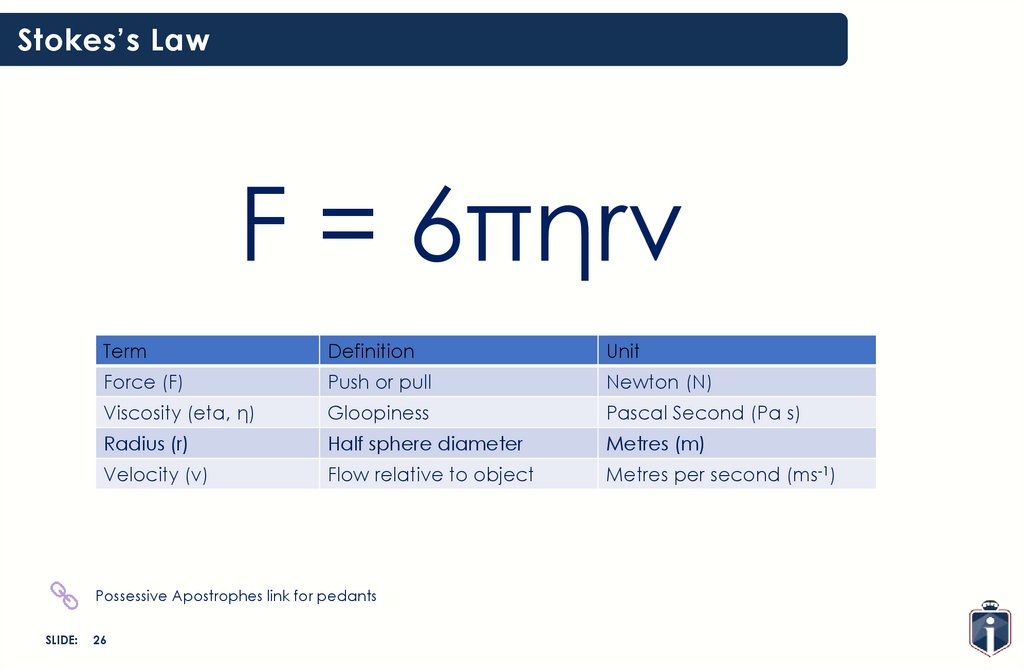
Stokes’s LawF = 6πηrv
Term
Definition
Unit
Force (F)
Push or pull
Newton (N)
Viscosity (eta, η)
Gloopiness
Pascal Second (Pa s)
Radius (r)
Half sphere diameter
Metres (m)
Velocity (v)
Flow relative to object
Metres per second (ms-1)
Possessive Apostrophes link for pedants
SLIDE:
26
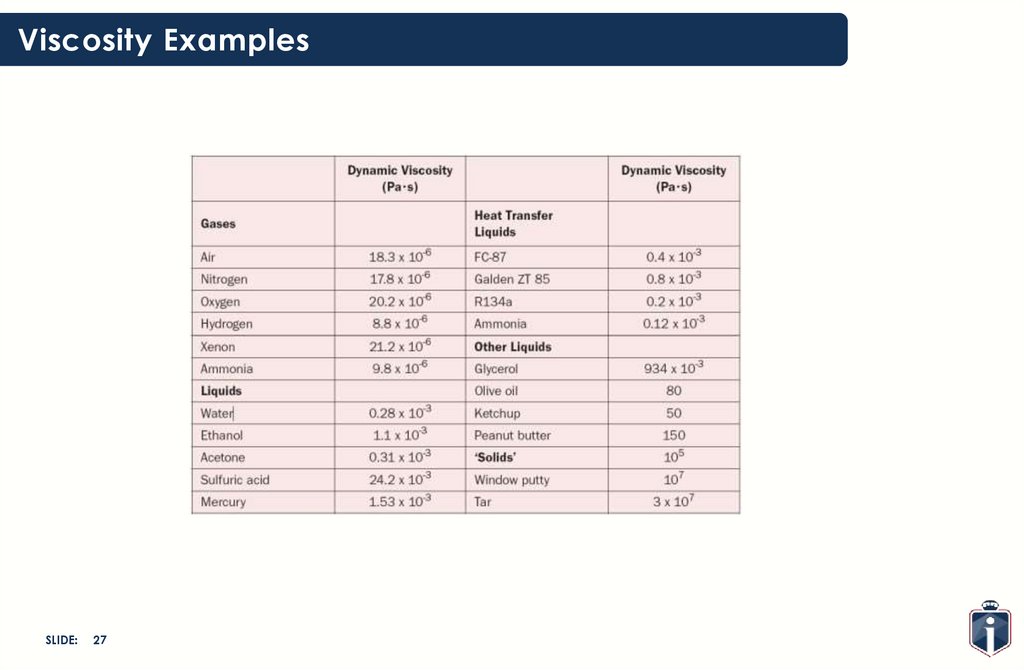
27.
Viscosity ExamplesSLIDE:
27
28.
Laminar vs Turbulent FlowRichard Hammond Engineering connection 40:00 – 45:00 min
SLIDE:
28
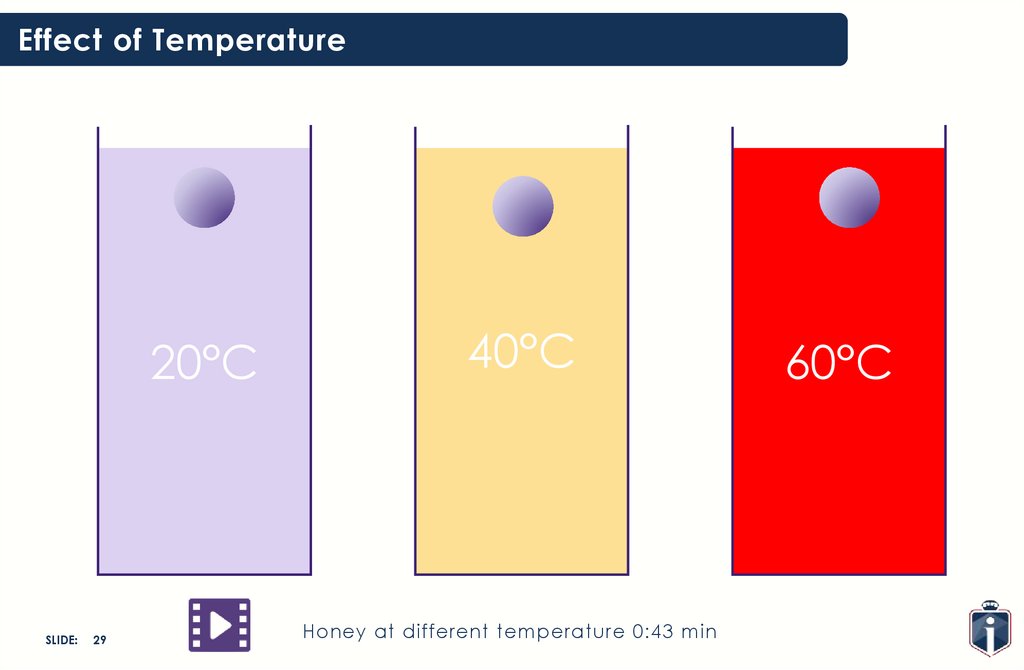
29.
Effect of Temperature20°C
SLIDE:
29
40°C
Honey at different temperature 0:43 min
60°C
30.
DefinitionsTerm
Definition
Density
Mass per unit volume
Mass
Amount of stuff, tendency to resist acceleration
Viscosity
A fluid’s resistance to flowing, stickiness or gloopiness
Volume
Three dimensional enclosed space
Weight
Force on mass in a gravity field
Velocity
Rate of change of displacement
Displacement
1. Distance moved in a given direction.
2. Volume of liquid push aside by a floating or emersed object
Upthrust
Force exerted by a fluid on a object emersed in it or floating on it
SLIDE:
30
31.
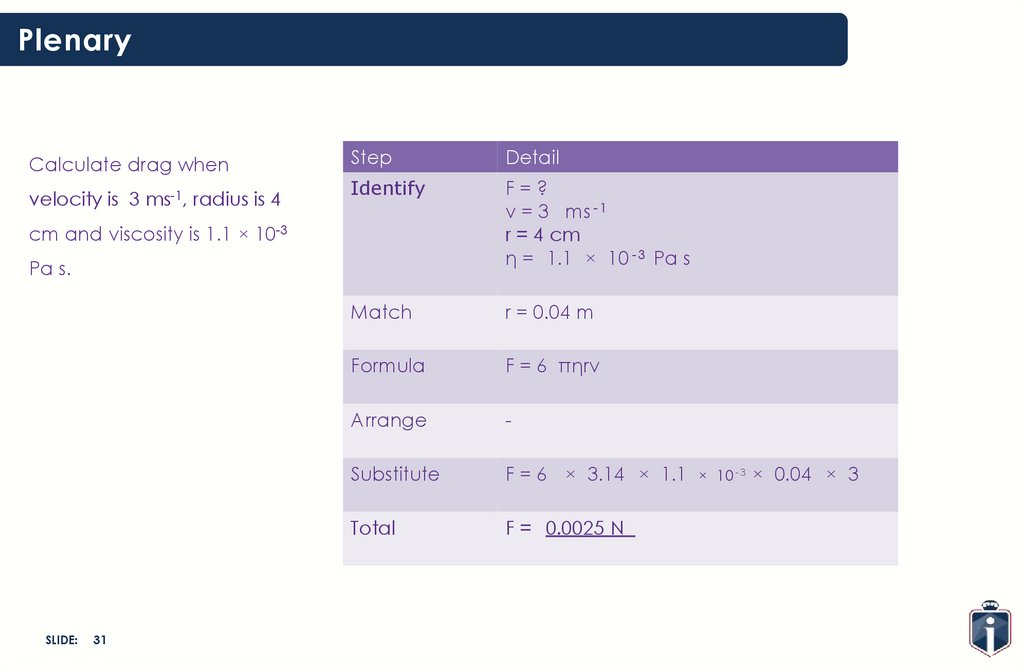
PlenaryCalculate drag when
velocity is 3 ms-1, radius is 4
Step
Detail
Identify
F=?
v = 3 ms - 1
r = 4 cm
η = 1.1 × 10 - 3 Pa s
Match
r = 0.04 m
Formula
F = 6 πηrv
Arrange
-
Substitute
F = 6 × 3.14 × 1.1 × 10 - 3 × 0.04 × 3
Total
F = 0.0025 N
cm and viscosity is 1.1 × 10-3
Pa s.
SLIDE:
31
32.
Learn 3: Measuring ViscosityMaterials – Viscosity
33.
ObjectiveCORE PRACTICAL 4 (INT 2): Use a falling-ball method to
determine the viscosity of a liquid.
SLIDE:
33
34.
StarterCalculate viscosity
when velocity is 7.3
Step
Detail
Identify
η=?
F = 4.3 N
v = 7.3 ms-1
r = 7.8 cm
Match
r = 0.078 m
Formula
F = 6πηrv
Arrange
η = F / 6ϖrv
Substitute
η = 4.3 / (6 × 3.14 × 0.078 × 7.3)
Total
η = 0.4 Pa s
ms-1, radius is 7.8 cm
and force is 4.3 N.
However, the problem is force is not easy to measure for a sphere sinking in fluid.
SLIDE:
34
35.
What we can measureWe can easily measure:
• the diameter of the metal sphere to find radius
• descent time and distance to calculate velocity
Steel ball Dropped in a Viscous Fluid 2.03 min
SLIDE:
35
36.
Volume of a Sphere4 3
V = πr
3
SLIDE:
36
37.
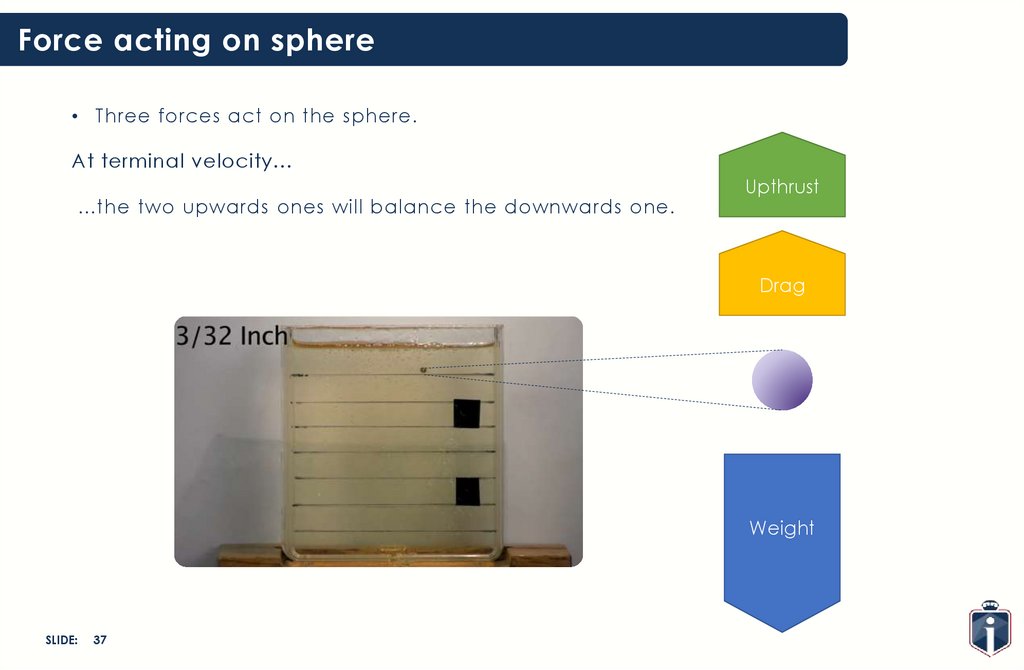
Force acting on sphere• Three forces act on the sphere.
At terminal velocity…
...the two upwards ones will balance the downwards one.
Upthrust
Drag
Weight
SLIDE:
37
38.
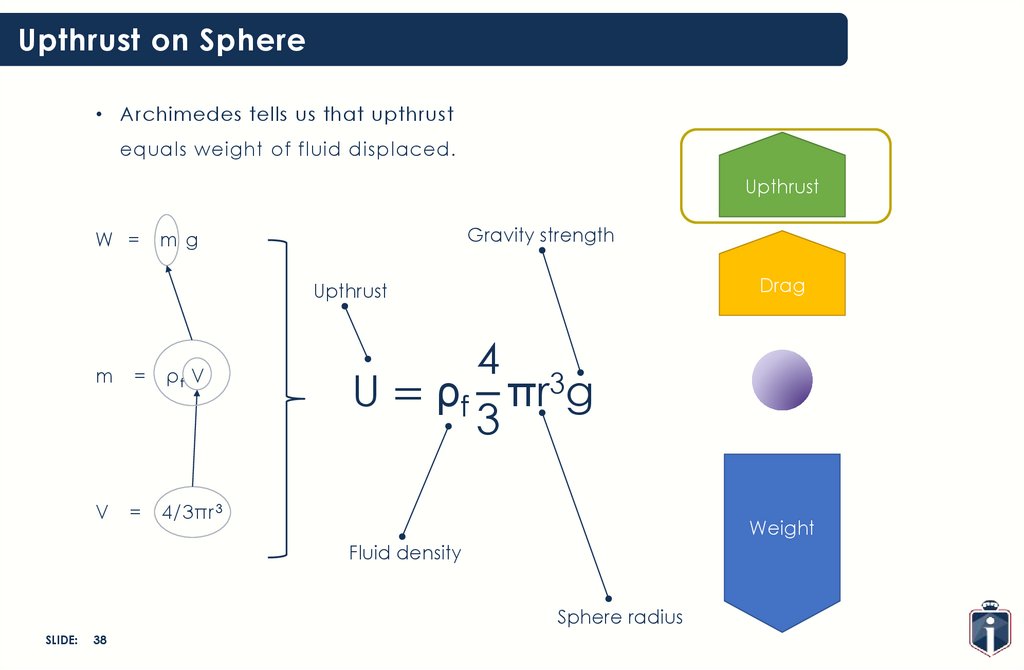
Upthrust on Sphere• Archimedes tells us that upthrust
equals weight of fluid displaced.
Upthrust
W =
Gravity strength
mg
Drag
Upthrust
m
=
ρf V
V
=
4/3πr 3
4 3
U = ρf πr g
3
Weight
Fluid density
Sphere radius
SLIDE:
38
39.
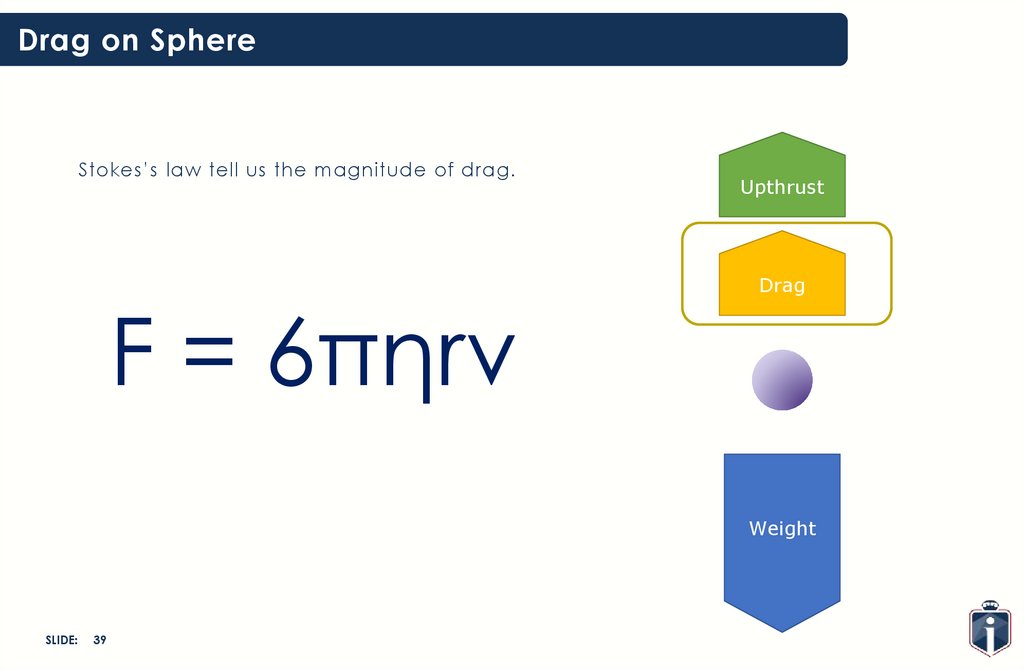
Drag on SphereStokes’s law tell us the magnitude of drag.
F = 6πηrv
Upthrust
Drag
Weight
SLIDE:
39
40.
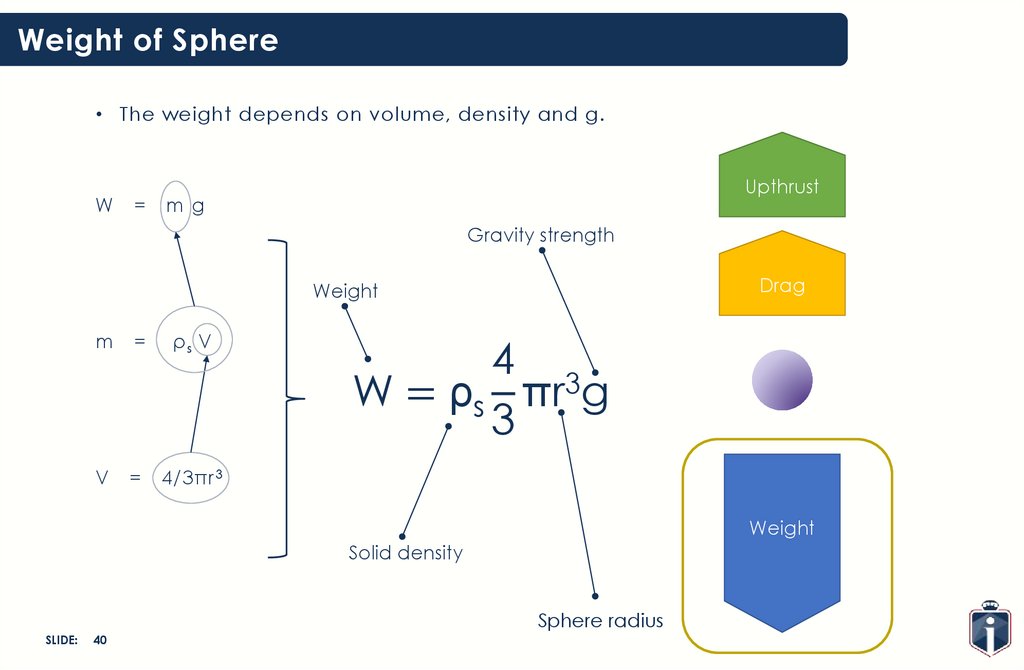
Weight of Sphere• The weight depends on volume, density and g.
W
=
Upthrust
mg
Gravity strength
Drag
Weight
m
=
ρs V
V
=
4/3πr 3
4 3
W = ρs πr g
3
Weight
Solid density
Sphere radius
SLIDE:
40
41.
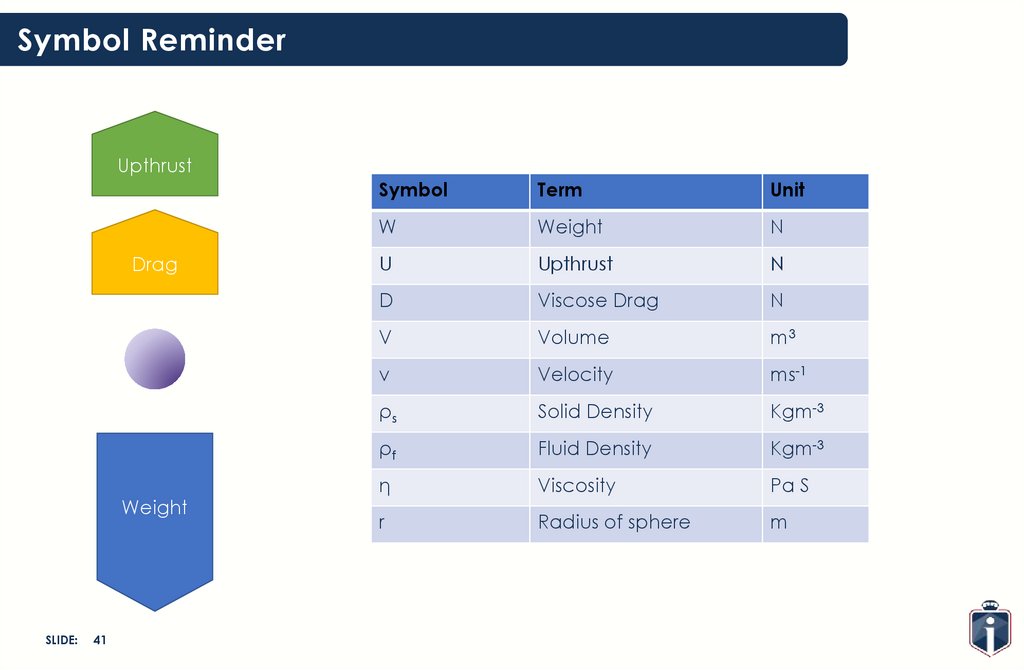
Symbol ReminderUpthrust
Drag
Weight
SLIDE:
41
Symbol
Term
Unit
W
Weight
N
U
Upthrust
N
D
Viscose Drag
N
V
Volume
m3
v
Velocity
ms-1
ρs
Solid Density
Kgm-3
ρf
Fluid Density
Kgm-3
η
Viscosity
Pa S
r
Radius of sphere
m
42.
Measuring ViscosityW
Upthrust
Drag
m
=
g
=
D
+
U
ρs
V
g
=
ρs
4/3 πr3
g
=
6πηrv
+
ρf 4/3 πr3g
ρs4/3 πr3g
=
6πηrv
+
ρf 4/3 πr3g
6πηrv
=
ρs4/3 πr3g
-
ρf 4/3 πr3g
6πηrv
=
η
=
4/3 πr3g (ρs - ρf)
4 πr3g (ρs - ρf)
3 × 6πrv
Weight
η
SLIDE:
42
=
2 r2g (ρs - ρf)
9v
43.
PlenaryWhy do large spheres sink faster than small ones?
• Area = πr 2
• Volume = 4/3πr 3
• If you double radius, you quadruple area.
• But you × 8 volume and so weight.
SLIDE:
43
44.
Lesson complete!See you next lesson


























 Английский язык
Английский язык








