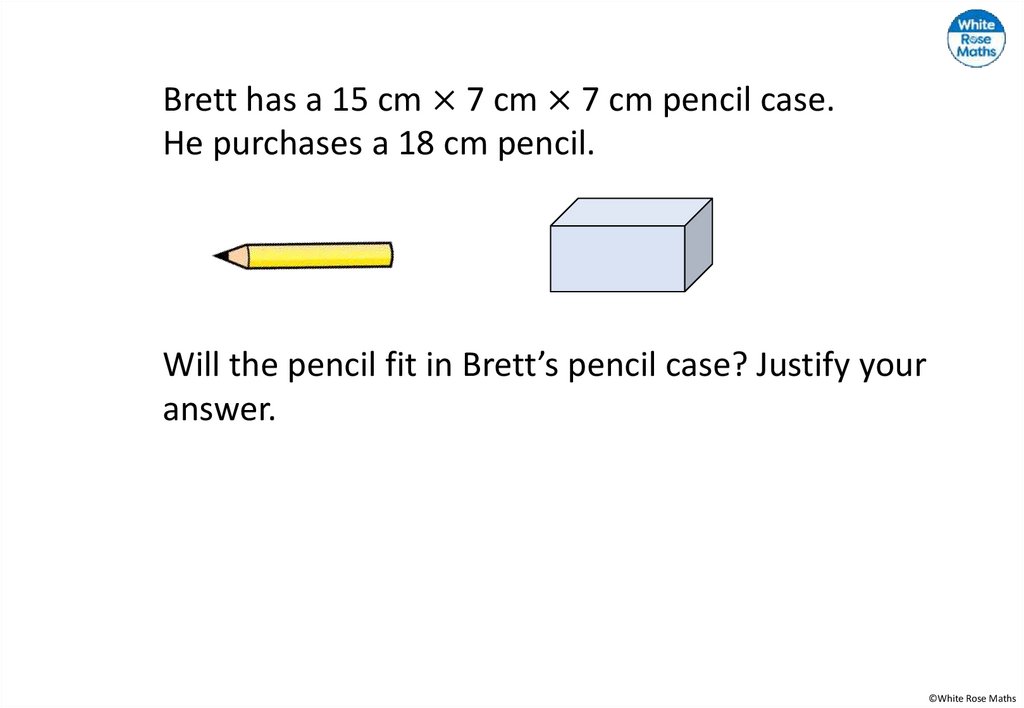
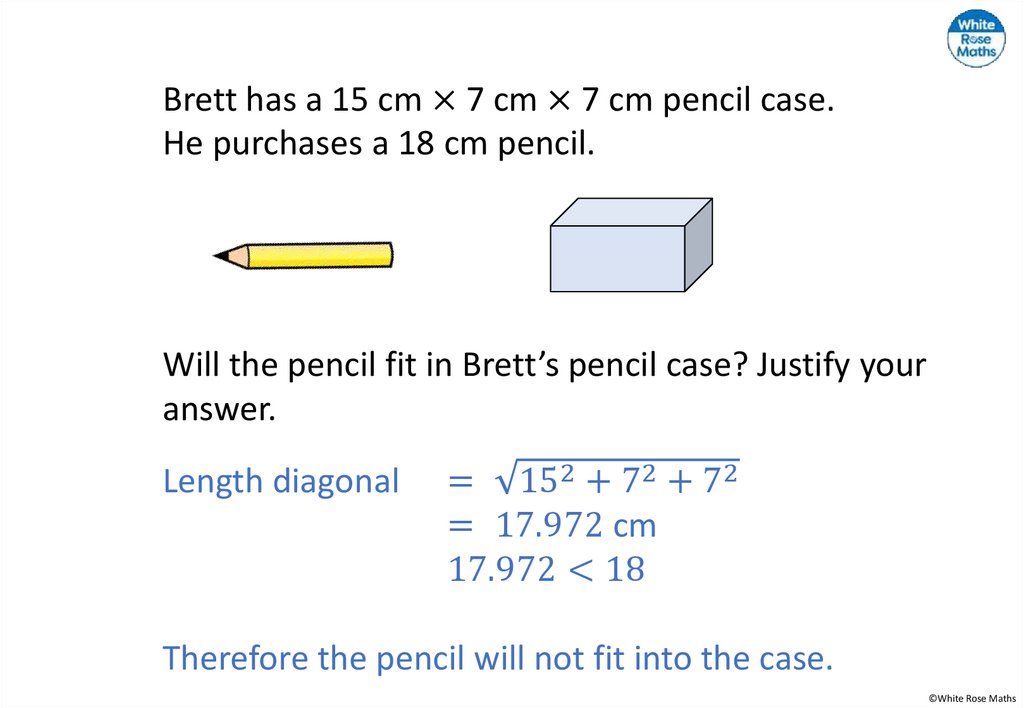
Похожие презентации:
Pythagoras’ theorem
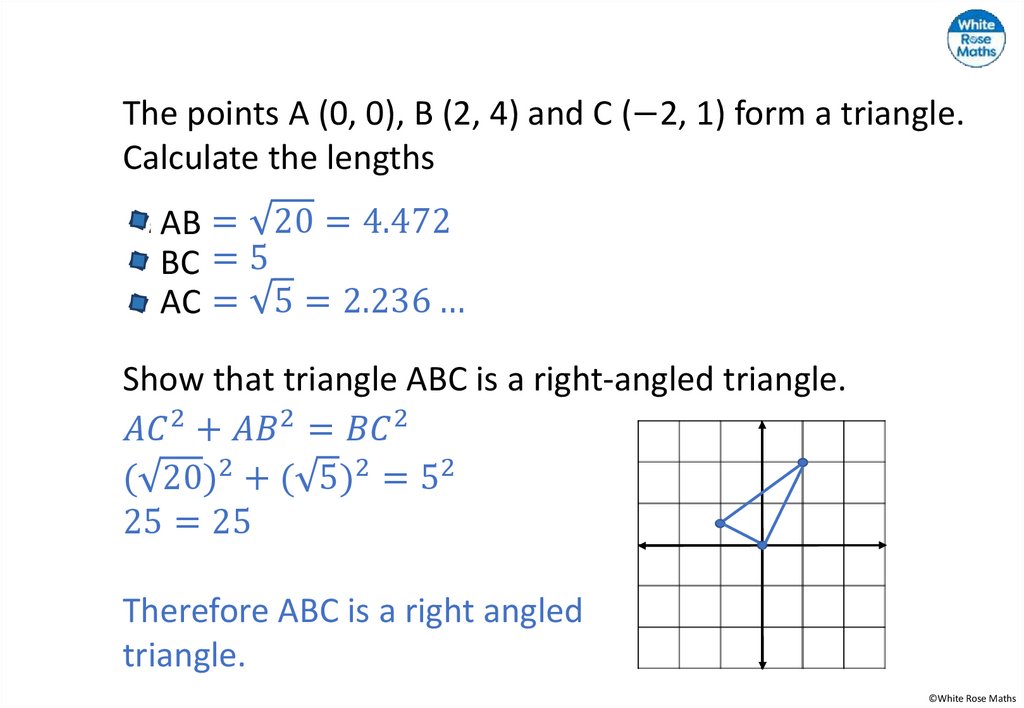
1.
Pythagoras’ TheoremYear 9
#MathsEveryoneCan
2.
Teddy has 30 counters.How many different square numbers can he make?
©White Rose Maths
3.
Teddy has 30 counters.How many different square numbers can he make?
5 different squares
1×1
2×2
3×3
4×4
5×5
©White Rose Maths
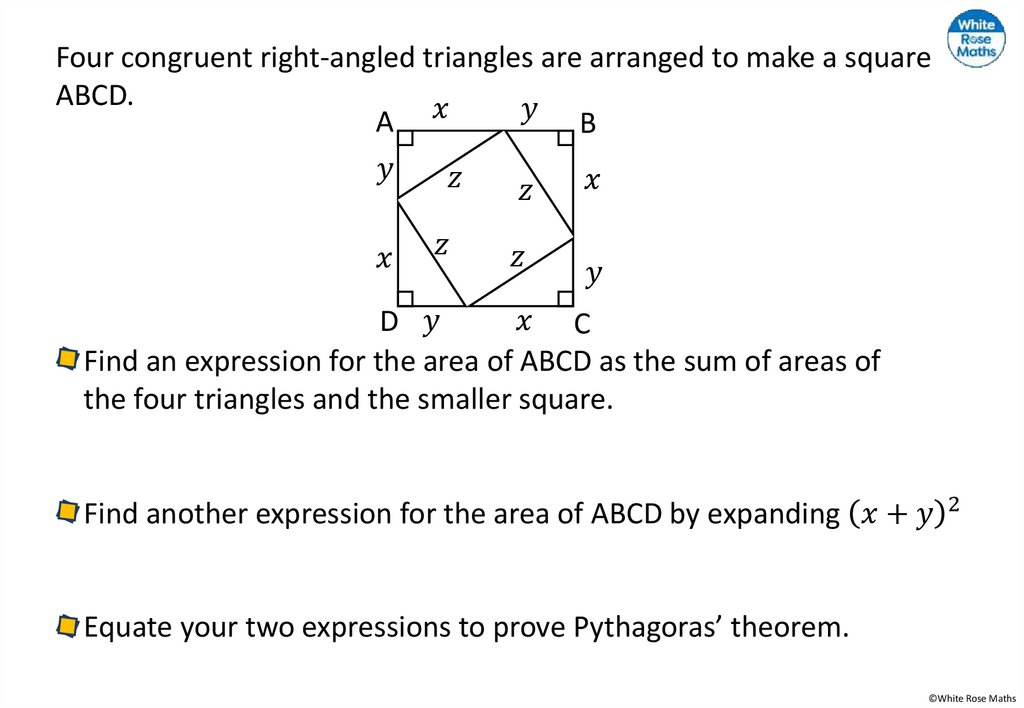
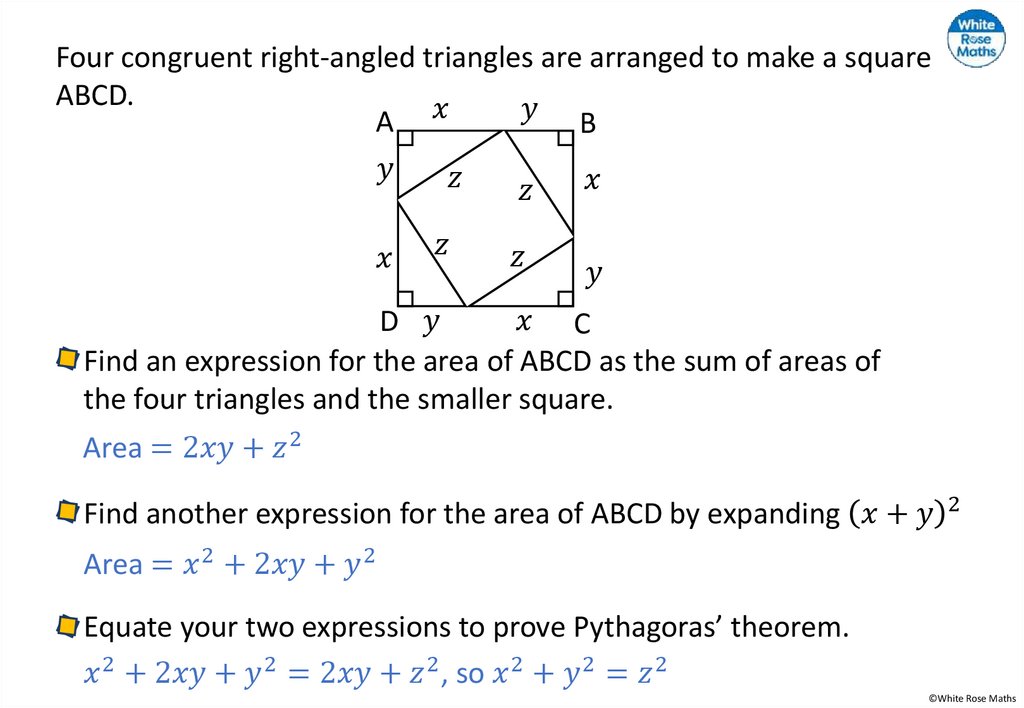
4.
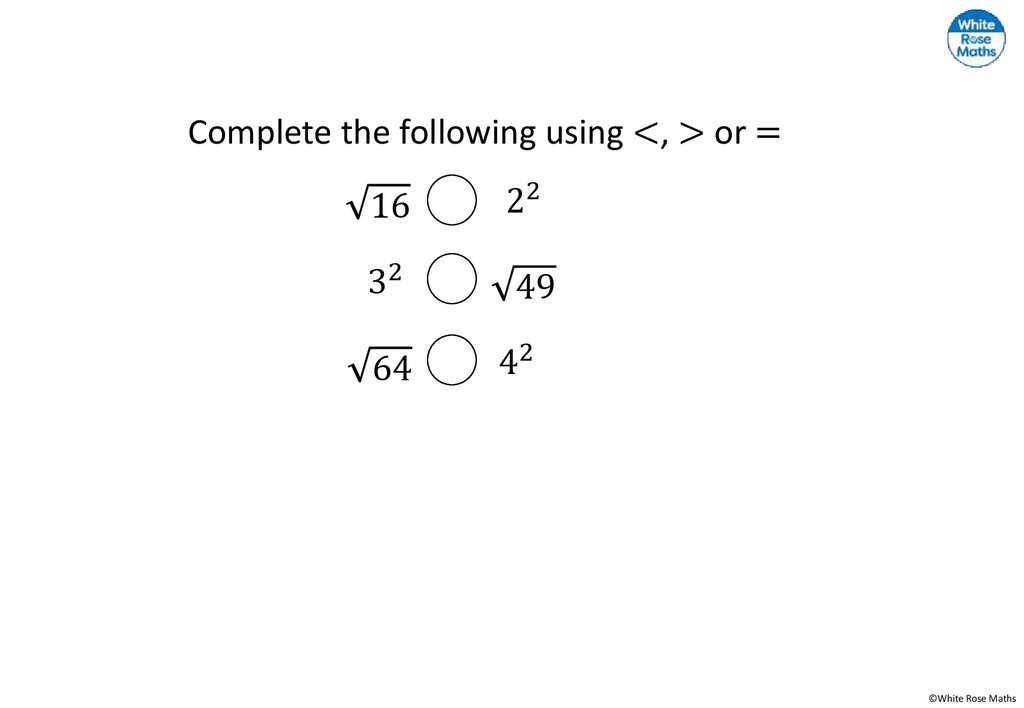
Complete the following using <, > or =16
22
32
49
64
42
©White Rose Maths
5.
Complete the following using <, > or =16 =
22
32 >
49
2
<
4
64
©White Rose Maths
6.
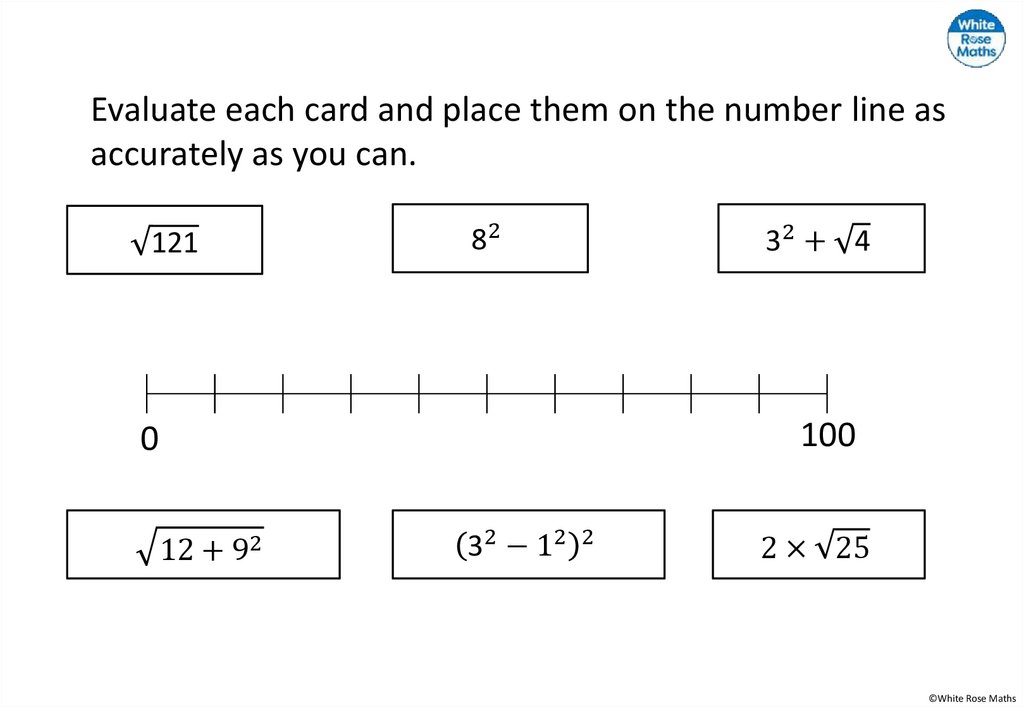
Evaluate each card and place them on the number line asaccurately as you can.
121
82
32 + 4
100
0
12 + 92
32 − 12 2
2 × 25
©White Rose Maths
7.
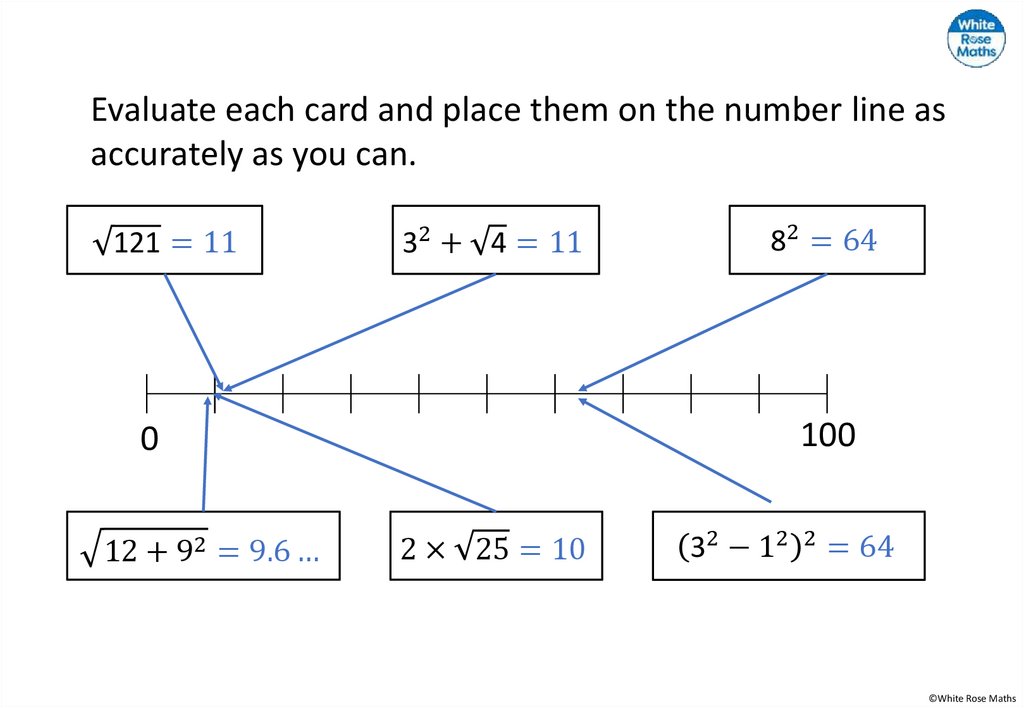
Evaluate each card and place them on the number line asaccurately as you can.
121 = 11
32 + 4 = 11
100
0
12 + 92 = 9.6 …
82 = 64
2 × 25 = 10
32 − 12 2 = 64
©White Rose Maths
8.
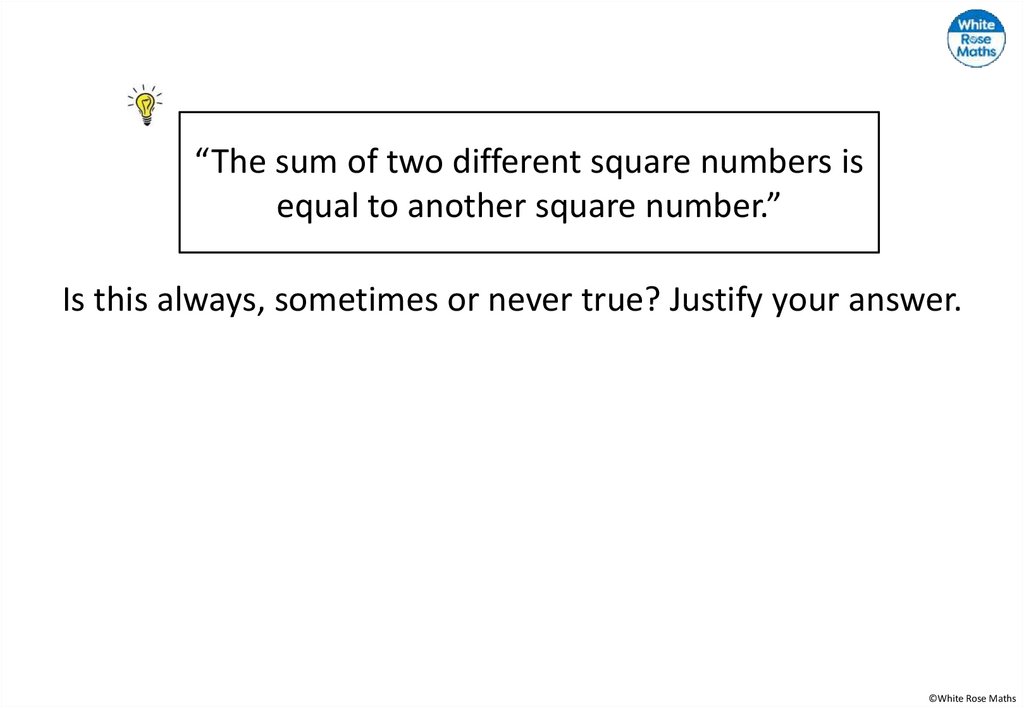
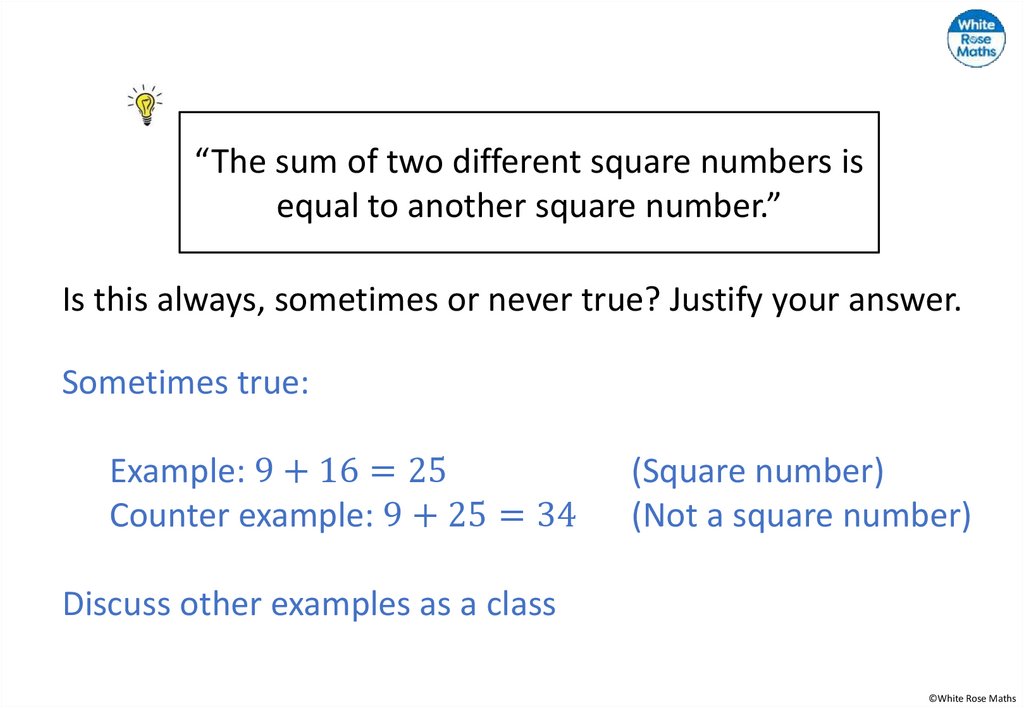
“The sum of two different square numbers isequal to another square number.”
Is this always, sometimes or never true? Justify your answer.
©White Rose Maths
9.
“The sum of two different square numbers isequal to another square number.”
Is this always, sometimes or never true? Justify your answer.
Sometimes true:
Example: 9 + 16 = 25
Counter example: 9 + 25 = 34
(Square number)
(Not a square number)
Discuss other examples as a class
©White Rose Maths
10.
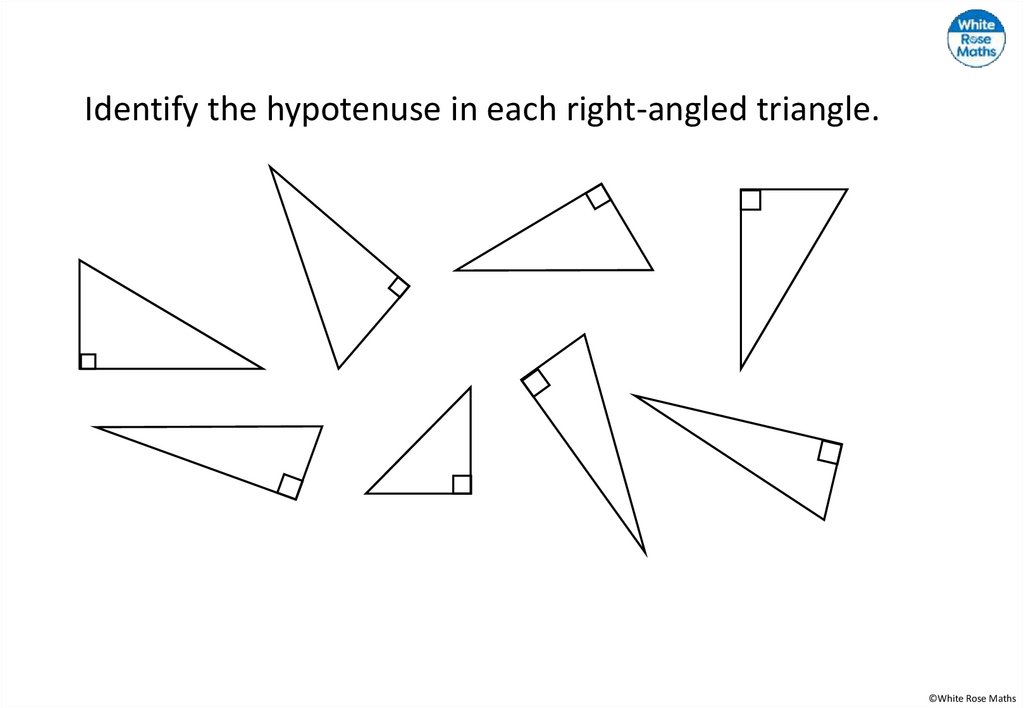
Identify the hypotenuse in each right-angled triangle.©White Rose Maths
11.
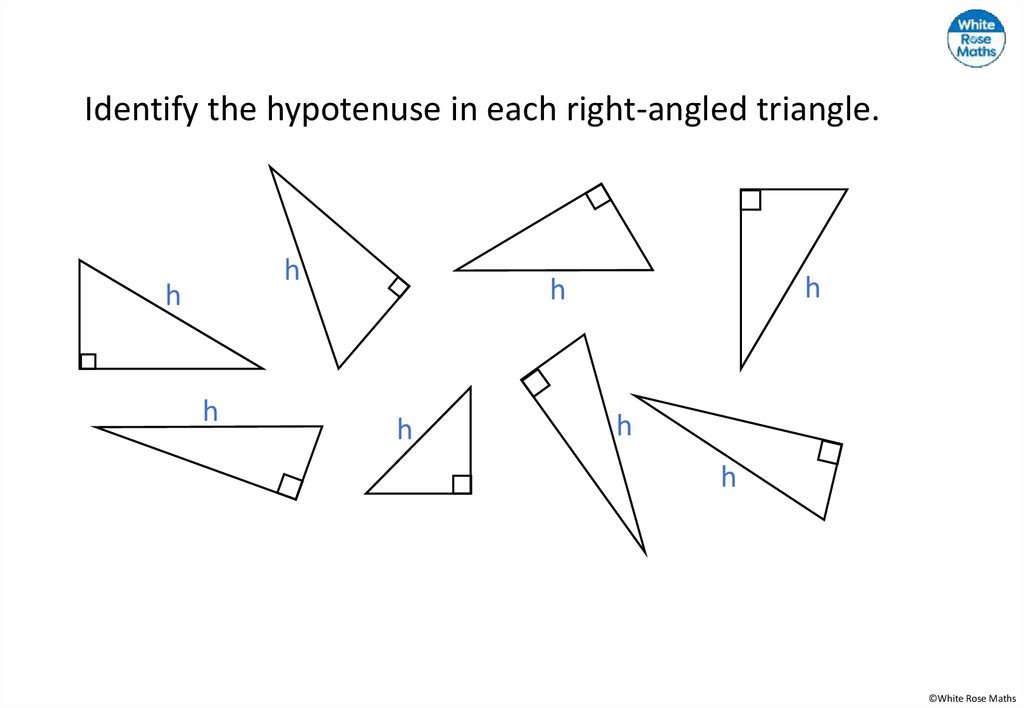
Identify the hypotenuse in each right-angled triangle.h
h
h
h
h
h
h
h
©White Rose Maths
12.
Amir says, “It is not possible to have a hypotenuse in asquare as it is a quadrilateral.”
Do you agree?
©White Rose Maths
13.
Amir says, “It is not possible to have a hypotenuse in asquare as it is a quadrilateral.”
Do you agree?
A square does not have a hypotenuse, but drawing the
diagonal of a square divides it into two congruent rightangled triangles. The diagonal is the hypotenuse of both
the triangles formed.
©White Rose Maths
14.
The line shown is the hypotenuse ofa right-angled triangle.
Complete the triangle.
How many possibilities can you find?
©White Rose Maths
15.
The line shown is the hypotenuse ofa right-angled triangle.
Complete the triangle.
How many possibilities can you find?
Possible answers include:
©White Rose Maths
16.
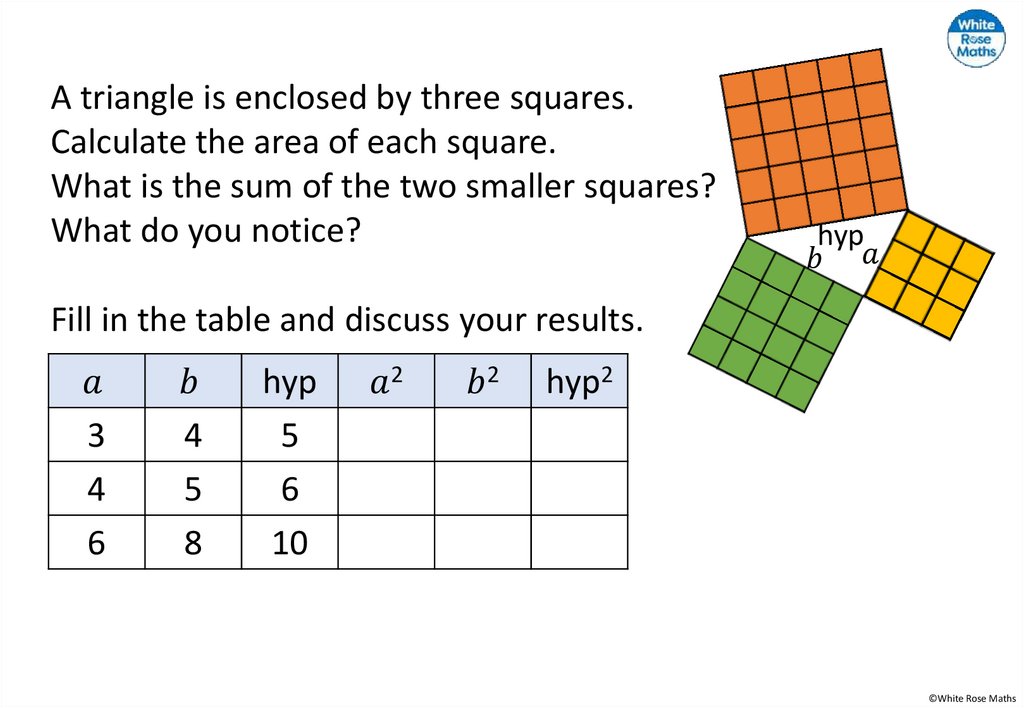
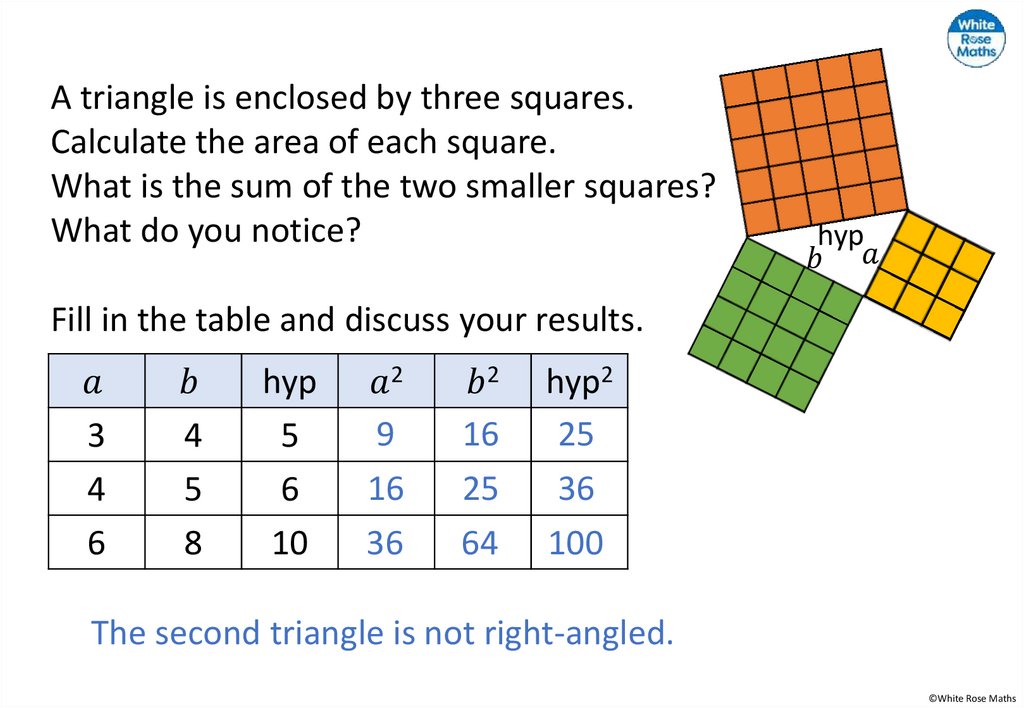
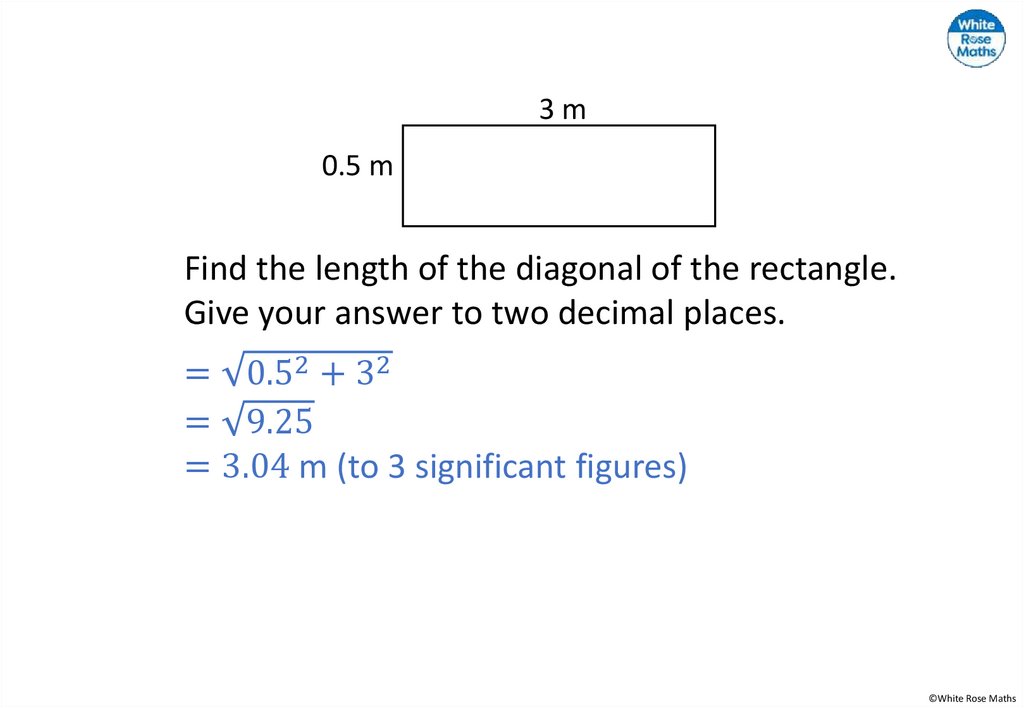
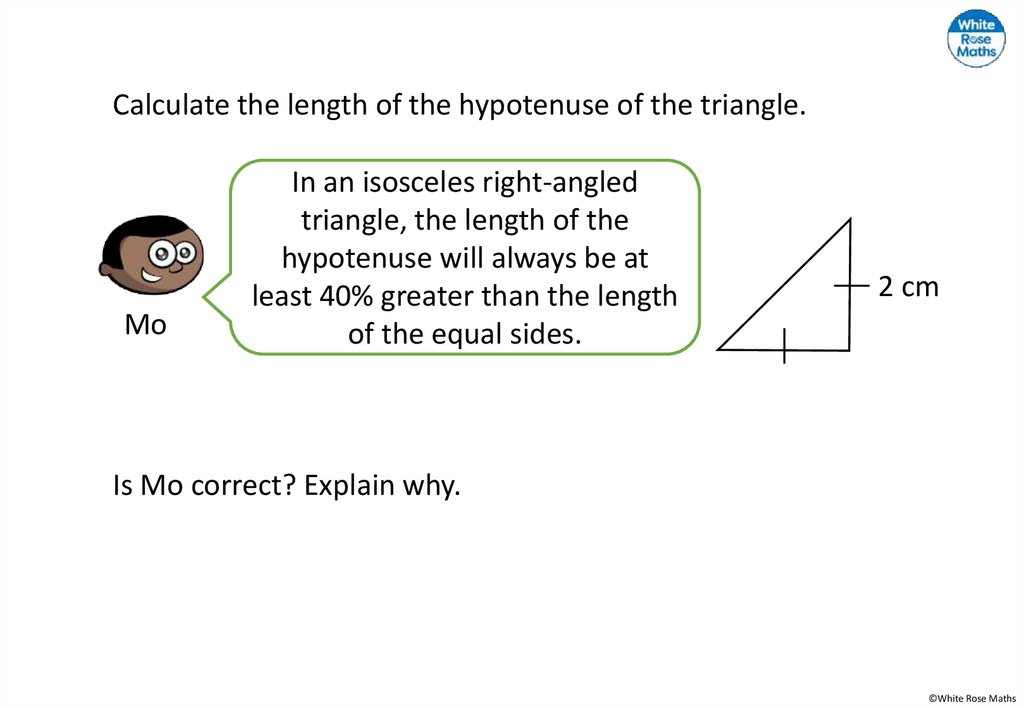
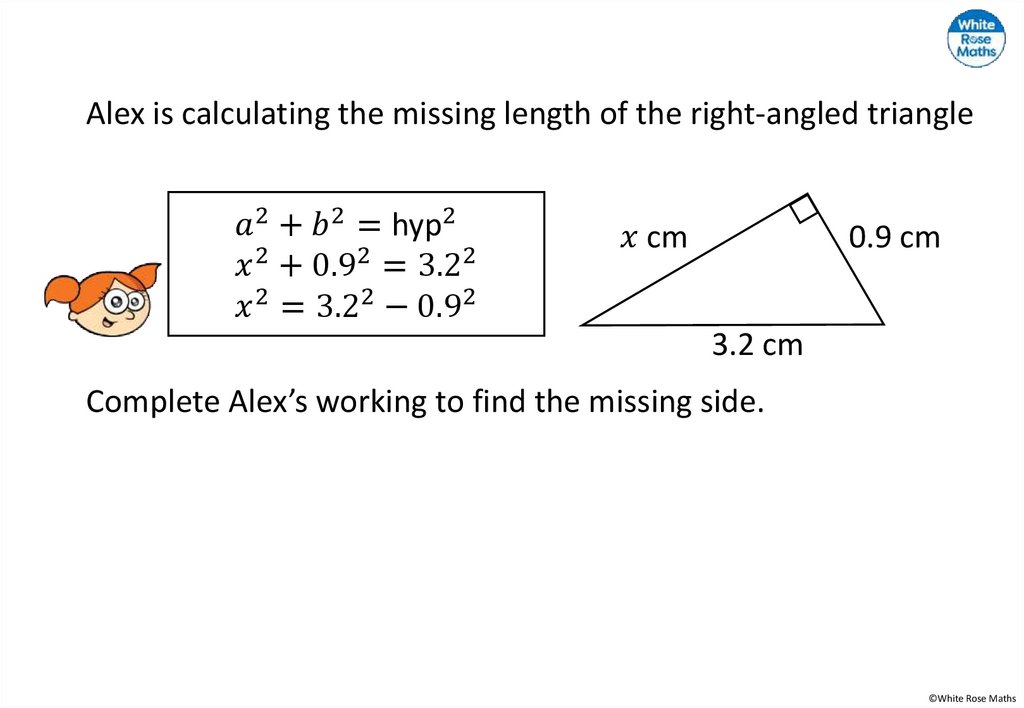
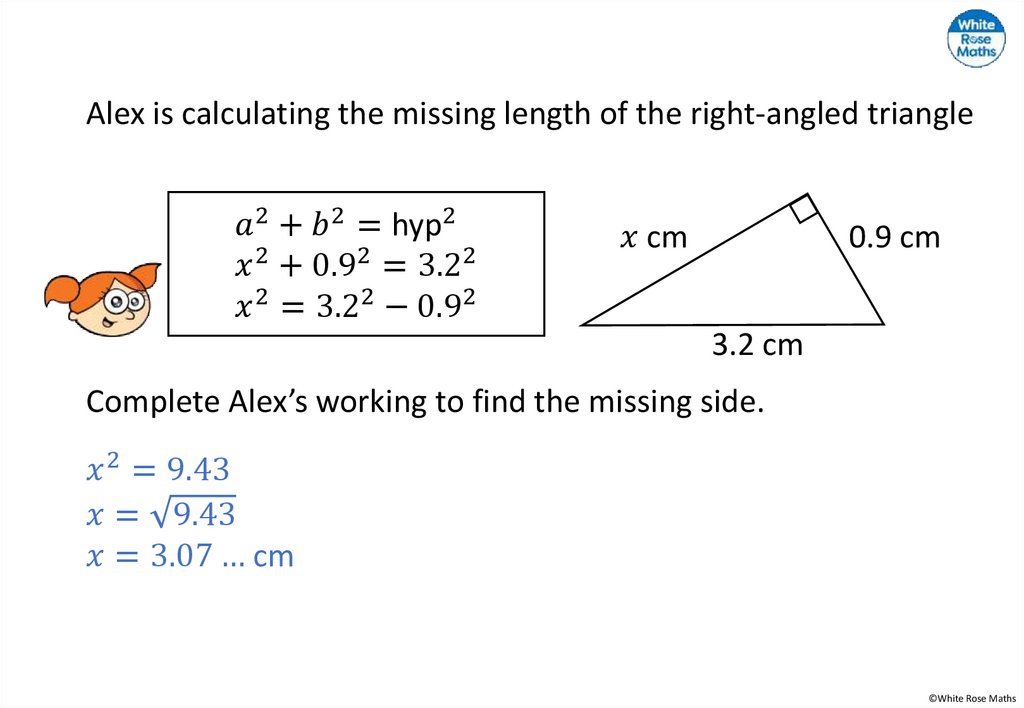
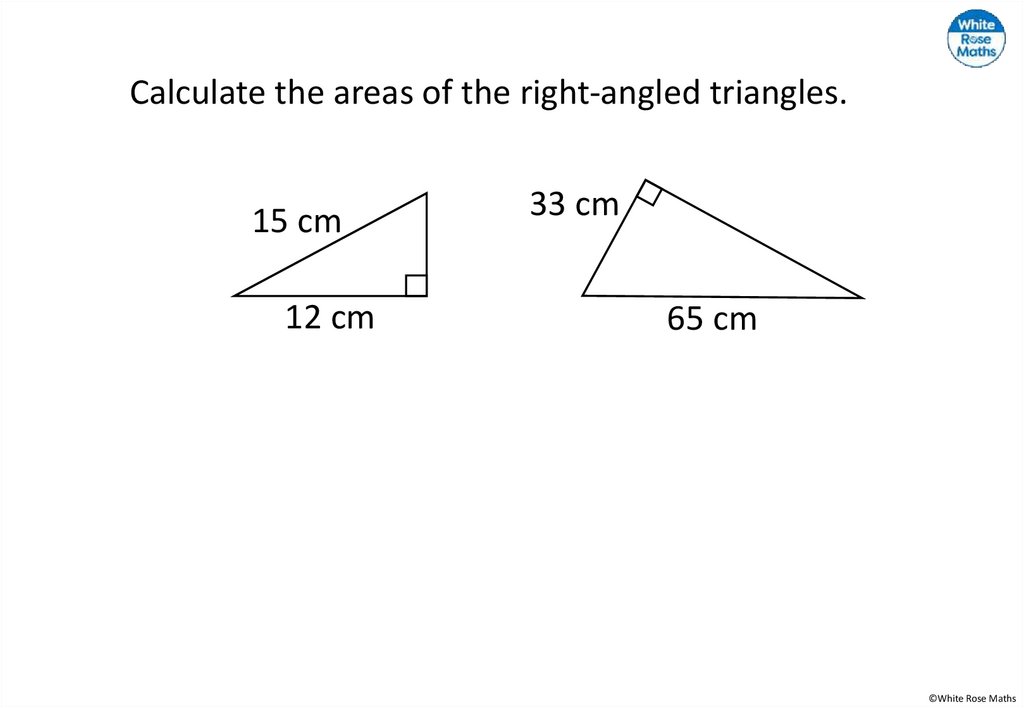
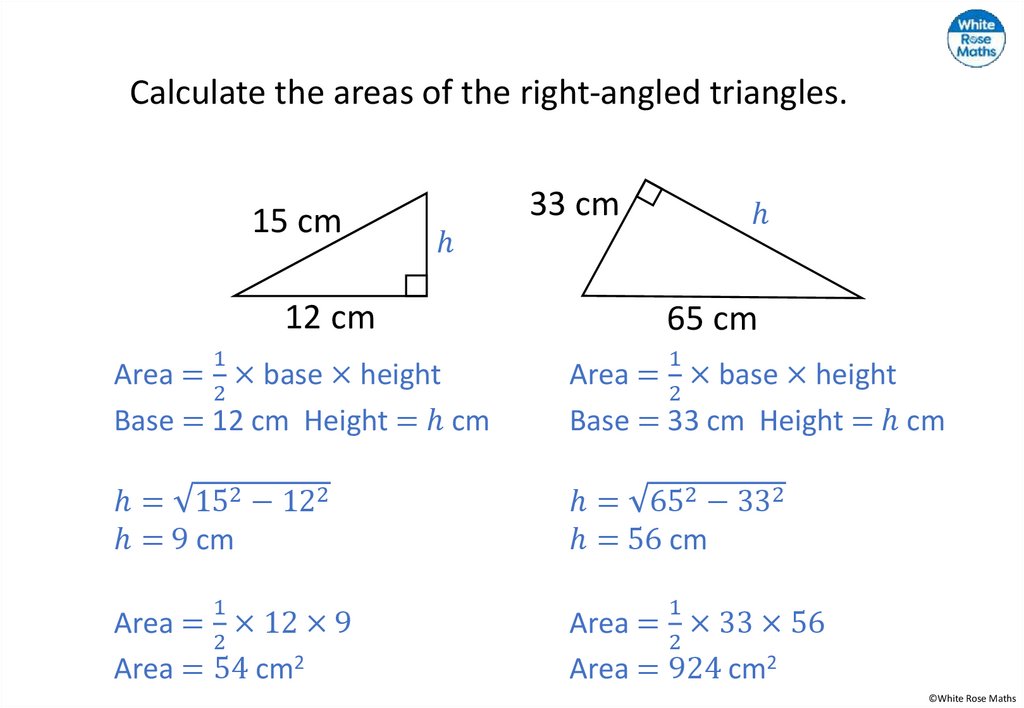
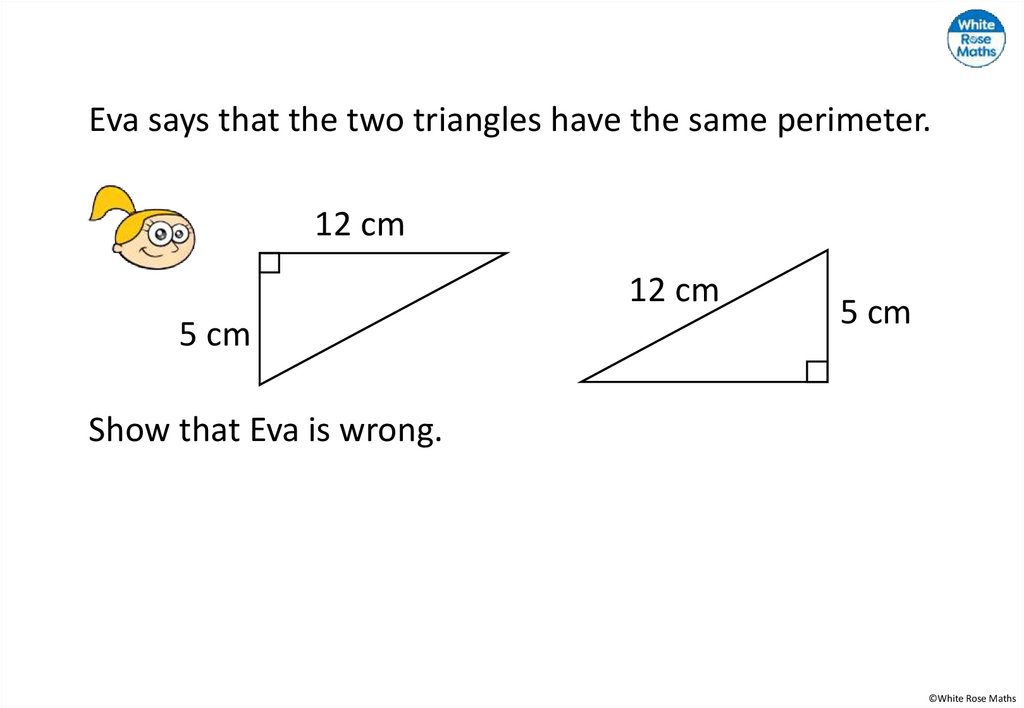
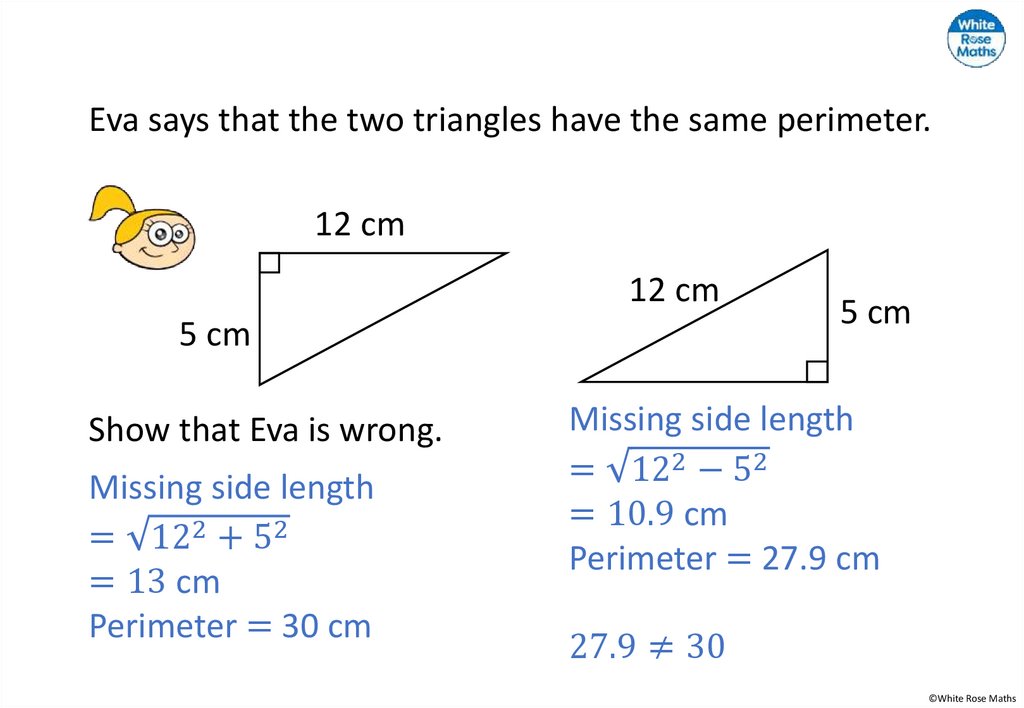
A triangle is enclosed by three squares.Calculate the area of each square.
What is the sum of the two smaller squares?
What do you notice?
hyp













 Математика
Математика








