Похожие презентации:
Inverse Trigonometric Functions
1. Inverse Trigonometric Functions
Basicdefinitions and
examples
2. Inverse Sine Function
Recall that for a function to have an inverse, it must be aone-to-one function and pass the Horizontal Line Test.
f(x) = sin x does not pass the Horizontal Line Test
and must be restricted to find its inverse.
y
y = sin x
1
2
x
1
Sin x has an inverse
function on this interval.
Algebra IGCSE 09/10/2024
2
3. Inverse Sine Function
The inverse sine function is defined byy = arcsin x
if and only if
sin y = x.
Angle whose sine is x
The domain of y = arcsin x is [–1, 1].
The range of y = arcsin x is [– /2 , /2].
Example:
a. arcsin 1
2 6
b. sin 1 3
2
3
is the angle whose sine is 1 .
6
2
sin 3
3
2
This is another way to write arcsin x.
3
4. Inverse Cosine Function
f(x) = cos x must be restricted to find its inverse.y
1
y = cos x
2
x
1
Cos x has an inverse
function on this interval.
4
5. Inverse Cosine Function
The inverse cosine function is defined byy = arccos x if and only if
cos y = x.
Angle whose cosine is x
The domain of y = arccos x is [–1, 1].
The range of y = arccos x is [0 , ].
Example:
is the angle whose cosine is 1 .
a.) arccos 1
3
2
2 3
5
1
3
5 3
b.) cos
cos
6
6
2
2
This is another way to write arccos x.
5
6. Inverse Tangent Function
f(x) = tan x must be restricted to find its inverse.y
y = tan x
2
3
2
3
2
x
2
Tan x has an inverse
function on this interval.
6
7. Inverse Tangent Function
The inverse tangent function is defined byy = arctan x
if and only if
tan y = x.
Angle whose tangent is x
The domain of y = arctan x is ( , ) .
The range of y = arctan x is [– /2 , /2].
Example:
a.) arctan 3
3
6
is the angle whose tangent is
b.) tan 1 3
3
tan 3
3
6
3.
3
This is another way to write arctan x.
7
8. Graphing Utility: Inverse Functions
Graphing Utility: Approximate the value of each expression.Set calculator to radian mode.
a. cos–1 0.75
b. arcsin 0.19
c. arctan 1.32
d. arcsin 2.5
8
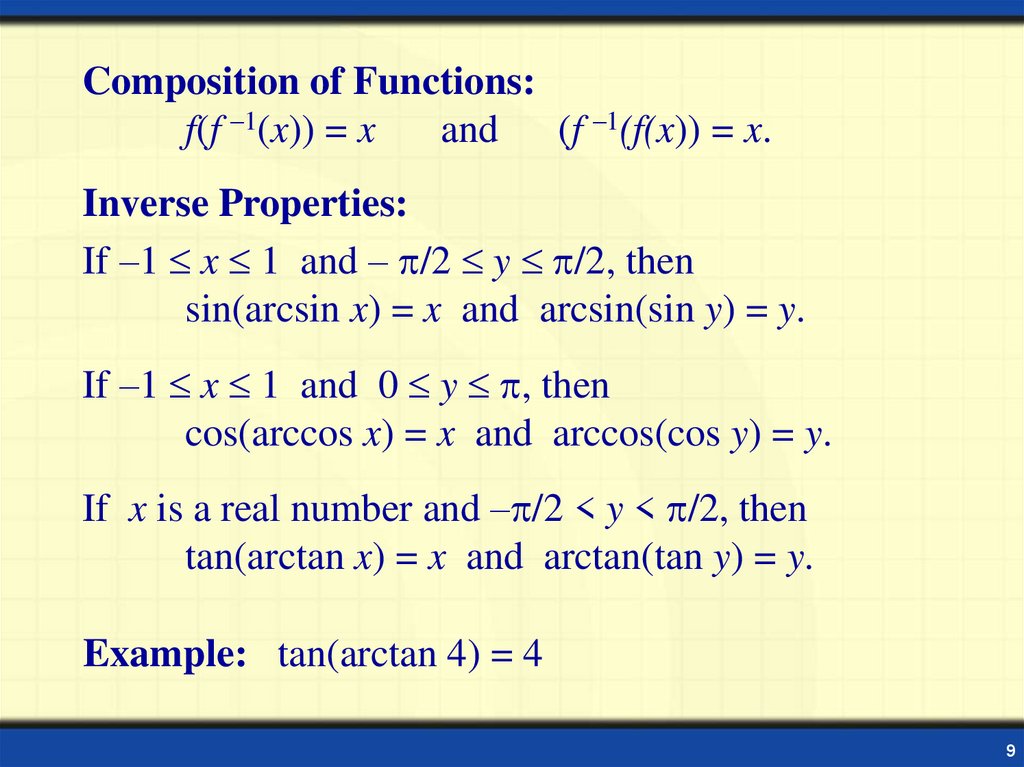
9. Composition of Functions
Composition of Functions:f(f –1(x)) = x
and (f –1(f(x)) = x.
Inverse Properties:
If –1 x 1 and – /2 y /2, then
sin(arcsin x) = x and arcsin(sin y) = y.
If –1 x 1 and 0 y , then
cos(arccos x) = x and arccos(cos y) = y.
If x is a real number and – /2 < y < /2, then
tan(arctan x) = x and arctan(tan y) = y.
Example: tan(arctan 4) = 4
9
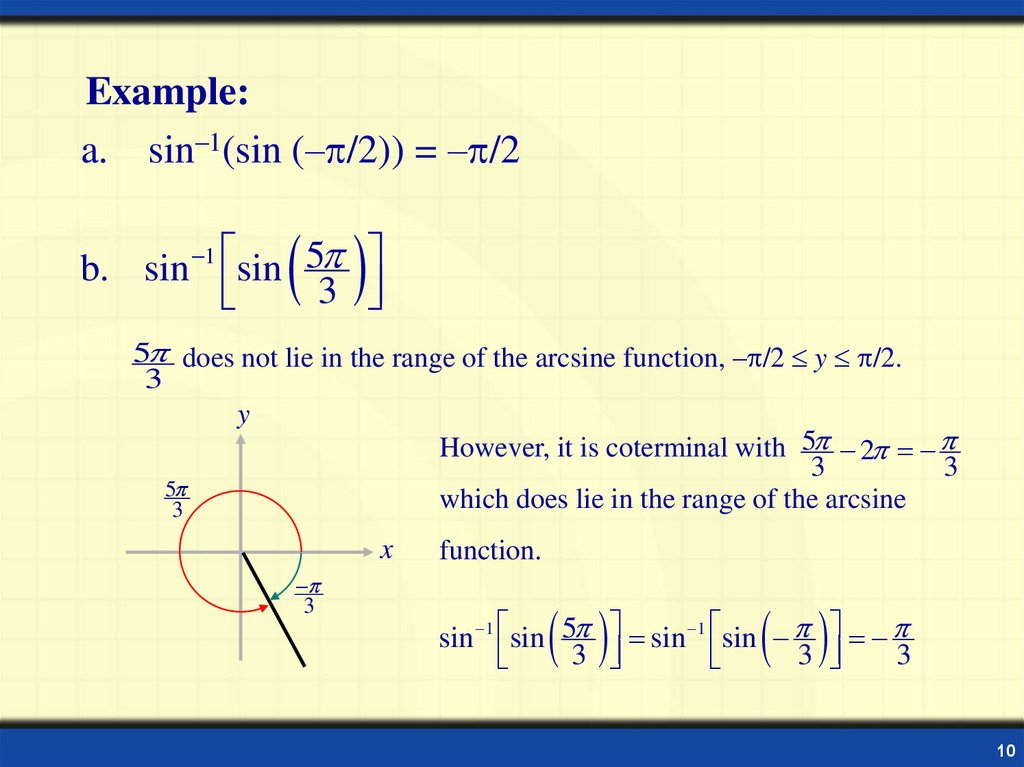
10. Composition of Functions
Example:a. sin–1(sin (– /2)) = – /2
b. sin 1 sin 5
3
5 does not lie in the range of the arcsine function, – /2 y /2.
3
y
However, it is coterminal with 5 2
3
3
5
which does lie in the range of the arcsine
3
x
3
function.
sin 1 sin 5 sin 1 sin
3
3
3
10
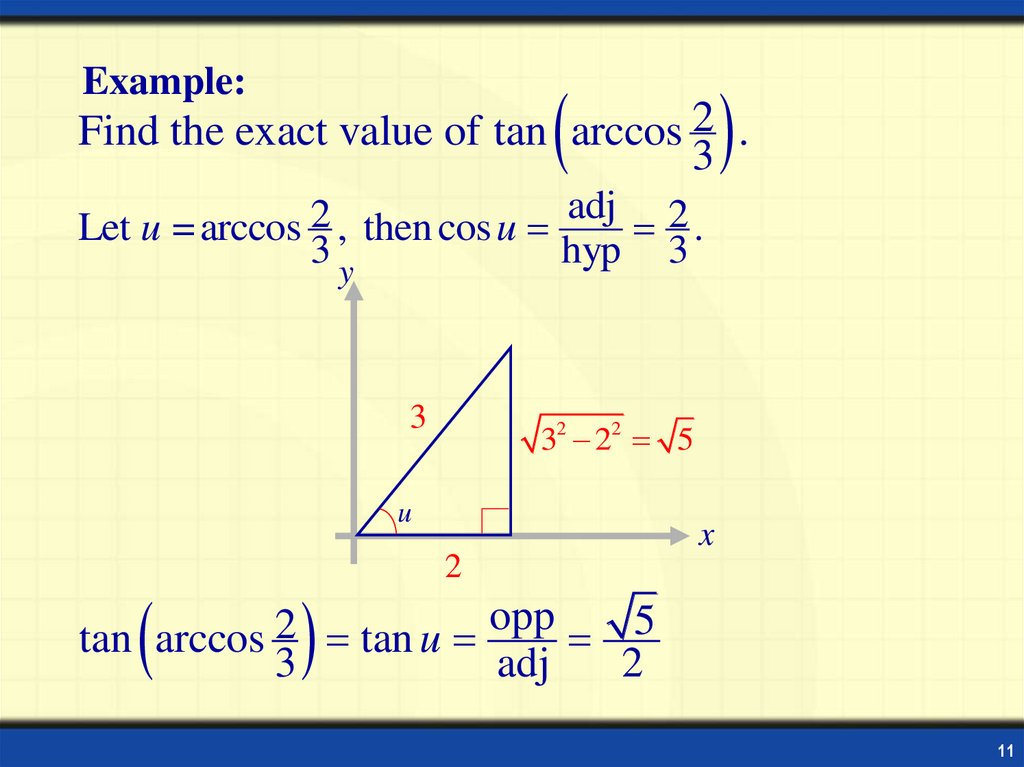
11. Example: Evaluating Composition of Functions
Example:Find the exact value of tan arccos 2 .
3
adj 2
2
Let u = arccos , then cos u
.
3y
hyp 3
3
32 22 5
u
x
2
opp
2
tan arccos tan u
5
3
adj
2
11
12.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.12
13.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.13
14.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.14
15.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.15
16.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.16
17.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.17
18.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.18
19.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.19
20.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.20
21.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.21
22.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.22
23.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.23
24.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.24
25.
Copyright © by Houghton Mifflin Company, Inc. All rights reserved.25








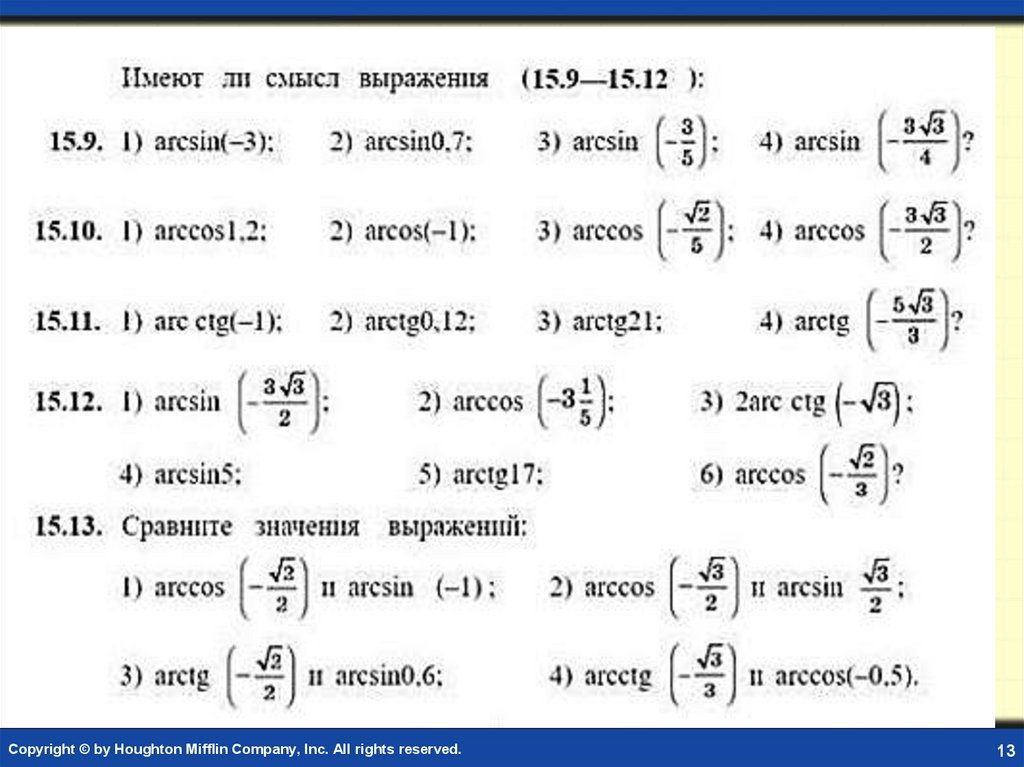










 Математика
Математика








