Похожие презентации:
Ch8: Hypothesis Testing (2 Samples)
1. Ch8: Hypothesis Testing (2 Samples)
8.1 Testing the Difference Between Means
(Independent Samples, 1 and 2 Known)
8.2 Testing the Difference Between Means
(Independent Samples, 1 and 2 Unknown)
8.3 Testing the Difference Between Means
(Dependent Samples)
8.4 Testing the Difference Between Proportions
Larson/Farber
1
2. 8.1 Two Sample Hypothesis Test
Compares two parameters from two populations.
Two types of sampling methods:
Independent (unrelated) Samples
•Sample 1: Test scores for 35 statistics students.
•Sample 2: Test scores for 42 biology students who do not study statistics.
Dependent Samples (paired or matched samples)
Each member of one sample corresponds to a member of the other sample.
•Sample 1: Resting heart rates of 35 individuals before drinking coffee.
•Sample 2: Resting heart rates of the same individuals after drinking two cups of coffee.
Larson/Farber
2
3. Stating a Hypotheses in 2-Sample Hypothesis Test
Null hypothesis• A statistical hypothesis H0
• Statement of equality ( , =, or ).
• No difference between the
parameters of two populations.
H0: μ1 = μ2
Ha: μ1 ≠ μ2
OR
Alternative Hypothesis (Ha)
(Complementary to Null Hypothesis)
• A statement of inequality (>, , or <).
• True when H0 is false.
H0: μ1 ≤ μ2
Ha: μ1 > μ2
OR
H0: μ1 ≥ μ2
Ha: μ1 < μ2
Regardless of which hypotheses you use, you always assume there is no
difference between the population means, or μ1 = μ2.
Larson/Farber
3
4. Two Sample z-Test for the Difference Between Means (μ1 and μ2.)
Three conditions are necessary1. The samples must be randomly selected.
2.
The samples must be independent.
3.
Each population must have a normal distribution with a known
population standard deviation OR each sample size must be at least 30.
Sampling distribution for x1 x2 (difference of sample means) is a normal with:
Mean: x x x x 1 2
1
2
1
Standard error:
2
x x
1
Test Statistic:
x1 x2
Sampling
distribution
1
x2
1
1
2
1 2
σ x x
1
2
2
12 22
n1
n2
Standardized Test Statistic:
x1 x2 1 2
12 22
z
where x x
x x
n1 n2
1
σ x x
Larson/Farber
2
x2
2
2
•For large samples: use s1 and s2 in place of 1 and 2.
x x •For small samples: use a two-sample z-test if populations
are normally distributed & pop. std deviations are known.
1
2
4
5. Using a Two-Sample z-Test for the Difference Between Means (Independent Samples 1 and 2 known or n1 and n2 30 )
Using a Two-Sample z-Test for theDifference Between Means (Independent
Samples 1 and 2 known or n1 and n2 30 )
In Words
In Symbols
1.
State the claim mathematically. Identify the
null and alternative hypotheses.
State H0 and Ha.
2.
Specify the level of significance.
Identify .
3.
Sketch the sampling distribution.
4.
Determine the critical value(s).
Use Table 4 in Appendix B.
5.
6.
Determine the rejection region(s).
Find the standardized test statistic.
z
7.
Make a decision to reject or fail to
reject the null hypothesis.
8.
Interpret the decision in the context of
the original claim.
Larson/Farber
x1 x2 1 2
x x
1
2
If z is in the rejection region,
reject H0.
5
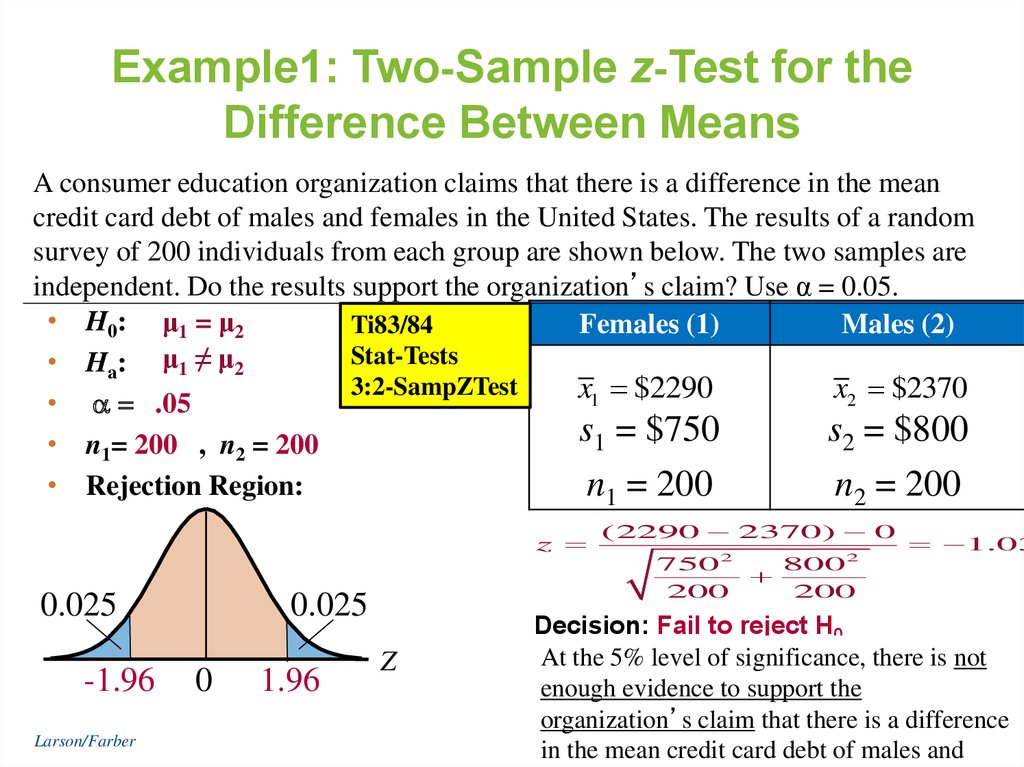
6. Example1: Two-Sample z-Test for the Difference Between Means
A consumer education organization claims that there is a difference in the meancredit card debt of males and females in the United States. The results of a random
survey of 200 individuals from each group are shown below. The two samples are
independent. Do the results support the organization’s claim? Use α = 0.05.
• H0 : μ 1 = μ 2
Females (1)
Males (2)
Ti83/84
Stat-Tests
• Ha: μ1 ≠ μ2
3:2-SampZTest
x1 $2290
x2 $2370
• .05
s1 = $750
s2 = $800
• n1= 200 , n2 = 200
• Rejection Region:
n1 = 200
n2 = 200
z
0.025
-1.96
Larson/Farber
1.96
2
750
200
0.025
0
(2290 2370) 0
Z
2
1.03
800
200
Decision: Fail to reject H0
At the 5% level of significance, there is not
enough evidence to support the
organization’s claim that there is a difference
in the mean credit card debt of males and 6
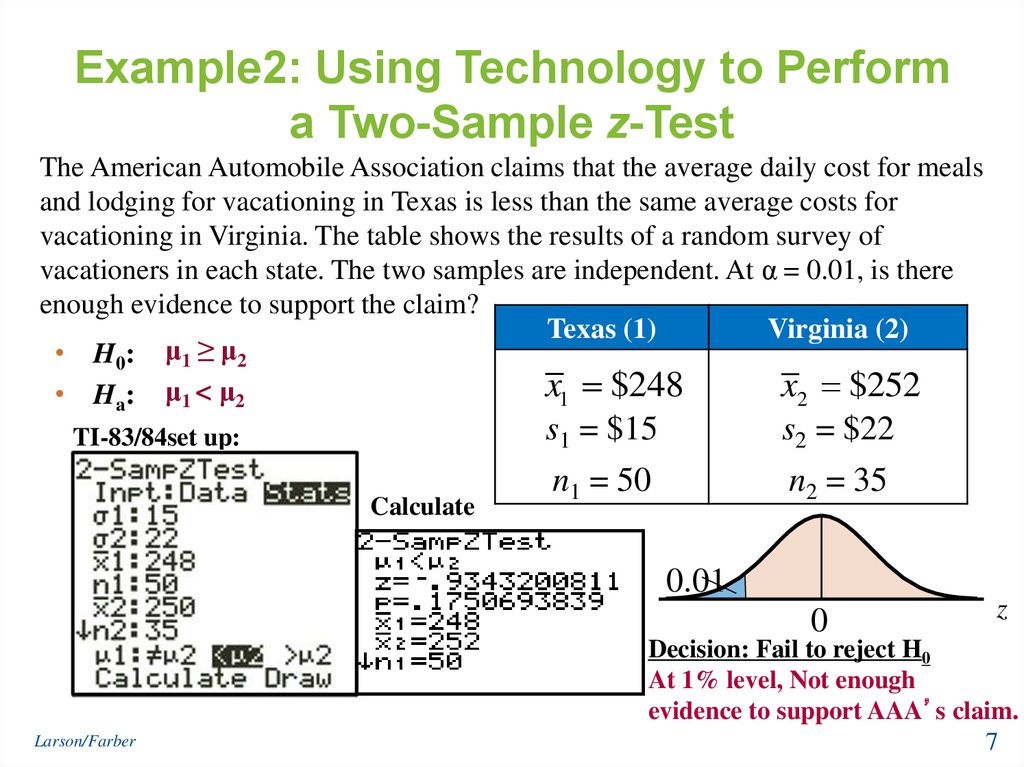
7. Example2: Using Technology to Perform a Two-Sample z-Test
The American Automobile Association claims that the average daily cost for mealsand lodging for vacationing in Texas is less than the same average costs for
vacationing in Virginia. The table shows the results of a random survey of
vacationers in each state. The two samples are independent. At α = 0.01, is there
enough evidence to support the claim?
Texas (1)
Virginia (2)
• H0: μ1 ≥ μ2
x2 $252
x1 $248
• Ha : μ 1 < μ 2
TI-83/84set up:
Calculate
s1 = $15
n1 = 50
s2 = $22
n2 = 35
0.01
0
Larson/Farber
z
Decision: Fail to reject H0
At 1% level, Not enough
evidence to support AAA’s claim.
7
8. 8.2 Two Sample t-Test for the Difference Between Means (1 or 2 unknown)
8.2 Two Sample t-Test for the DifferenceBetween Means ( 1 or 2 unknown)
• If ( 1 or 2 is unknown and samples are taken from normally-distributed) OR
If ( 1 or 2 is unknown and both sample sizes are greater than or equal to 30)
THEN a t-test may be used to test the difference between the population
means μ1 and μ2.
• Three conditions are necessary to use a t-test for small independent samples.
1.
The samples must be randomly selected.
2.
The samples must be independent.
3.
Each population must have a normal distribution.
Test Statistic:
t
x1 x2 1 2
x x
1
Larson/Farber
2
8
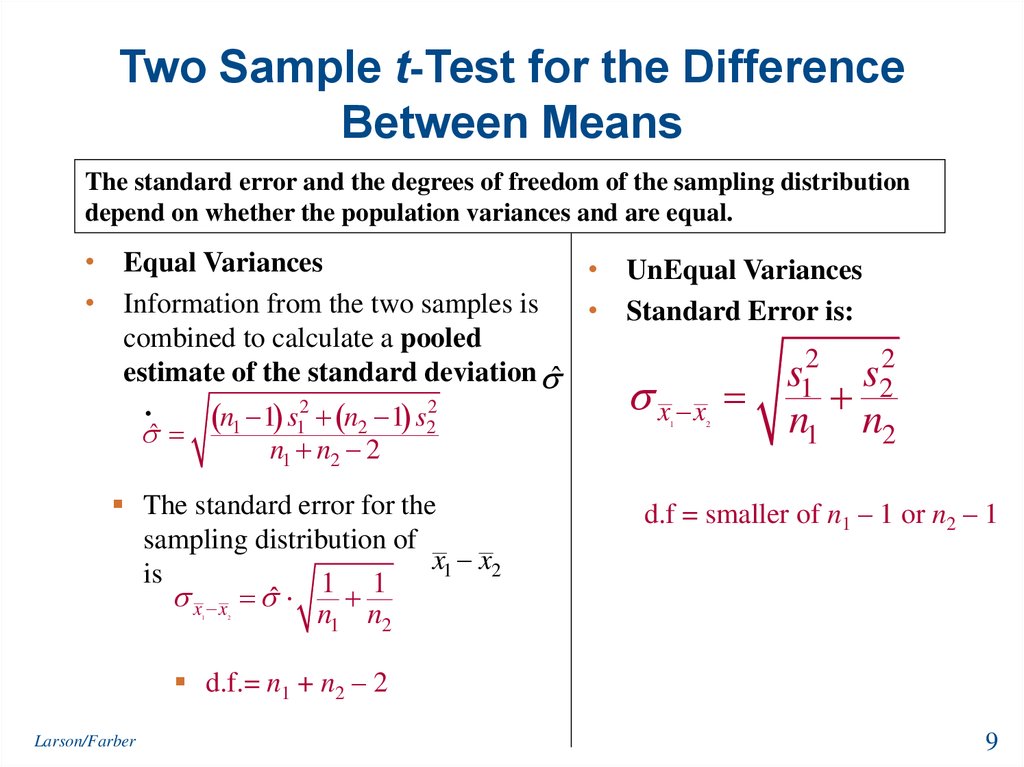
9. Two Sample t-Test for the Difference Between Means
The standard error and the degrees of freedom of the sampling distributiondepend on whether the population variances and are equal.
• Equal Variances
• UnEqual Variances
• Information from the two samples is
• Standard Error is:
combined to calculate a pooled
estimate of the standard deviation ˆ
s12 s22
2
2
.
x
x
n
1
s
n
1
s
1 1 2 2
n1 n2
ˆ
n1 n2 2
1
The standard error for the
sampling distribution of
x1 x2
is
x x ˆ 1 1
n1 n2
1
2
d.f = smaller of n1 – 1 or n2 – 1
2
d.f.= n1 + n2 – 2
Larson/Farber
9
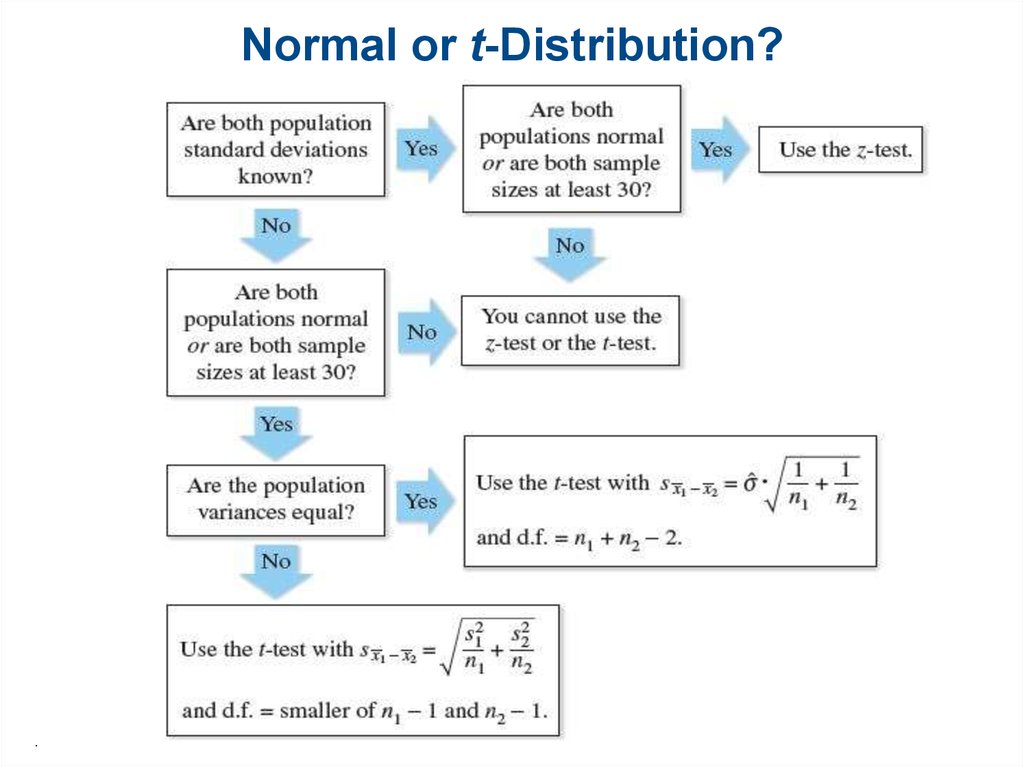
10. Normal or t-Distribution?
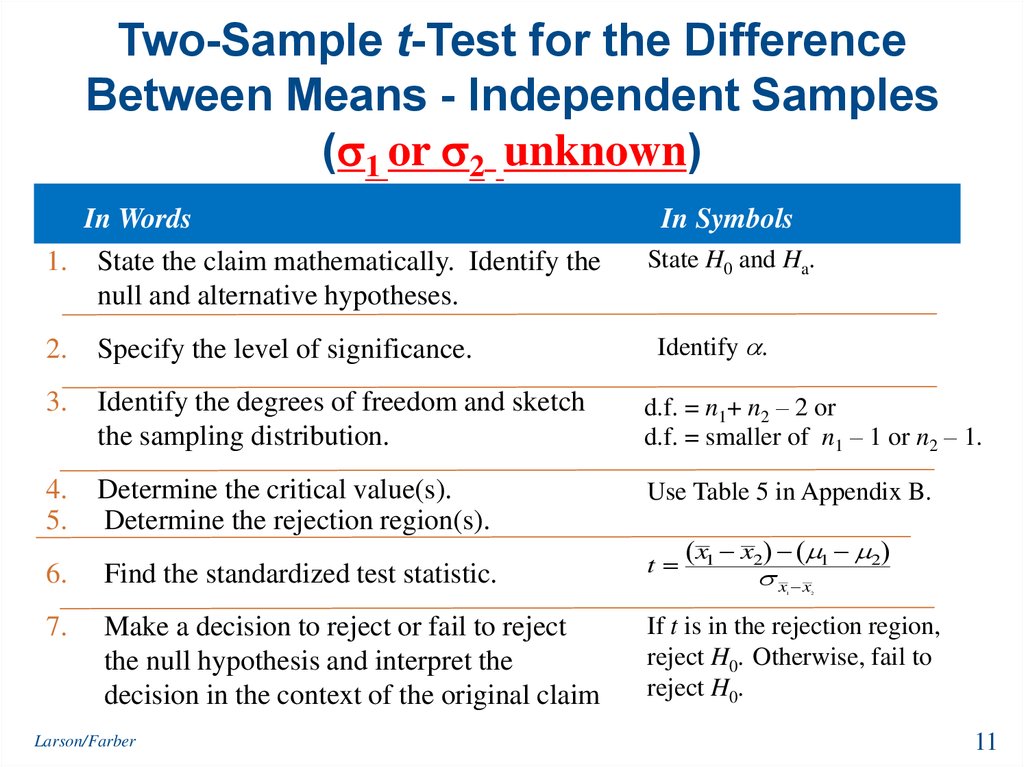
.11. Two-Sample t-Test for the Difference Between Means - Independent Samples (1 or 2 unknown)
Two-Sample t-Test for the DifferenceBetween Means - Independent Samples
( 1 or 2 unknown)
In Words
In Symbols
State H0 and Ha.
1.
State the claim mathematically. Identify the
null and alternative hypotheses.
2.
Specify the level of significance.
3.
Identify the degrees of freedom and sketch
the sampling distribution.
d.f. = n1+ n2 – 2 or
d.f. = smaller of n1 – 1 or n2 – 1.
4.
5.
Determine the critical value(s).
Determine the rejection region(s).
Use Table 5 in Appendix B.
6.
Find the standardized test statistic.
Identify .
t
x1 x2 1 2
x x
1
7.
Make a decision to reject or fail to reject
the null hypothesis and interpret the
decision in the context of the original claim
Larson/Farber
2
If t is in the rejection region,
reject H0. Otherwise, fail to
reject H0.
11
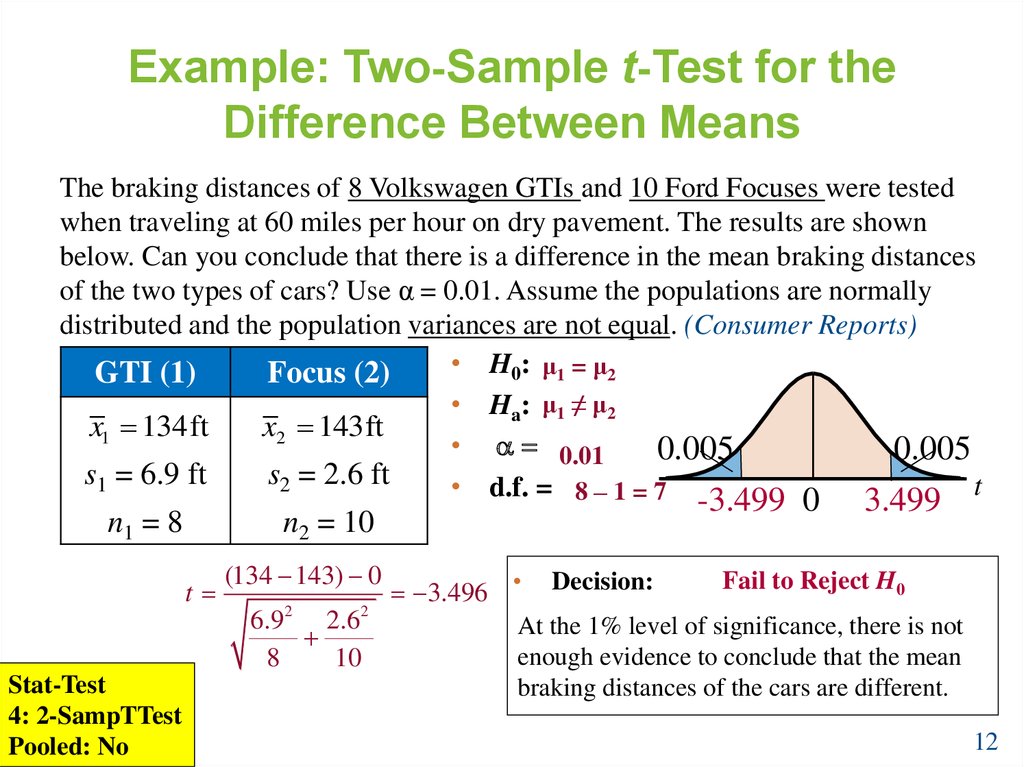
12. Example: Two-Sample t-Test for the Difference Between Means
The braking distances of 8 Volkswagen GTIs and 10 Ford Focuses were testedwhen traveling at 60 miles per hour on dry pavement. The results are shown
below. Can you conclude that there is a difference in the mean braking distances
of the two types of cars? Use α = 0.01. Assume the populations are normally
distributed and the population variances are not equal. (Consumer Reports)
• H0: μ1 = μ2
GTI (1)
Focus (2)
• Ha: μ1 ≠ μ2
x2 143ft
x1 134ft
• 0.01
0.005
0.005
s1 = 6.9 ft
s2 = 2.6 ft
t
• d.f. = 8 – 1 = 7
n1 = 8
n2 = 10
t
Stat-Test
4: 2-SampTTest
Larson/Farber
Pooled:
No 4th ed
(134 143) 0
6.92 2.62
8
10
-3.499 0
3.499
Fail to Reject H0
3.496 • Decision:
At the 1% level of significance, there is not
enough evidence to conclude that the mean
braking distances of the cars are different.
12
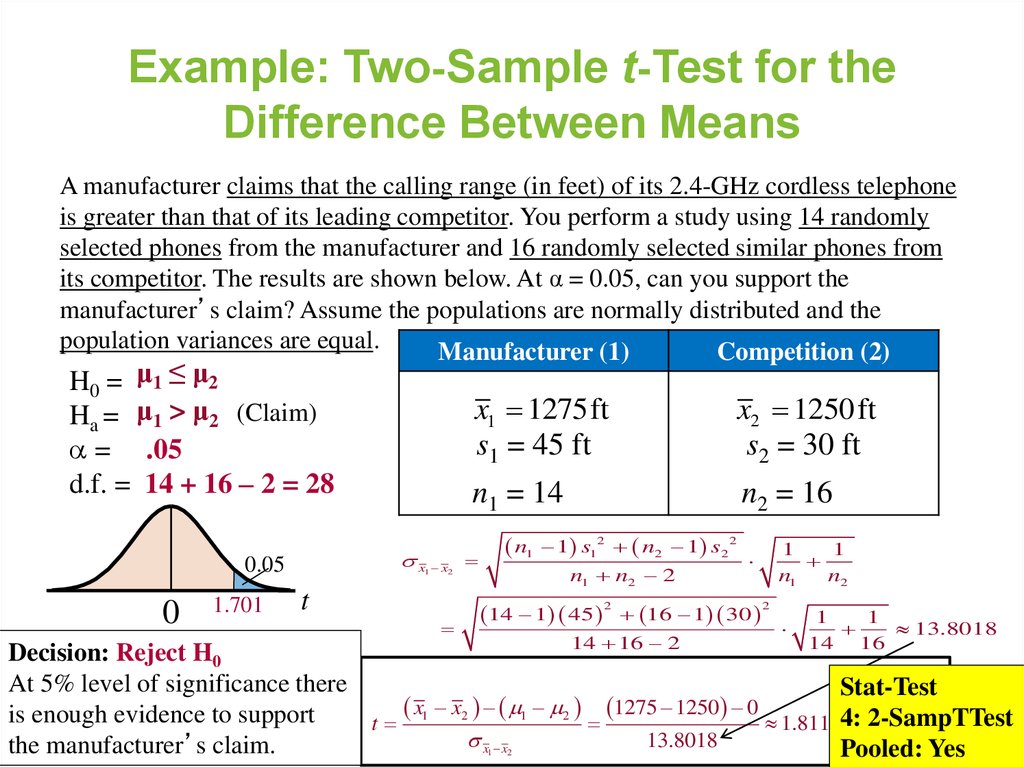
13. Example: Two-Sample t-Test for the Difference Between Means
A manufacturer claims that the calling range (in feet) of its 2.4-GHz cordless telephoneis greater than that of its leading competitor. You perform a study using 14 randomly
selected phones from the manufacturer and 16 randomly selected similar phones from
its competitor. The results are shown below. At α = 0.05, can you support the
manufacturer’s claim? Assume the populations are normally distributed and the
population variances are equal.
Manufacturer (1)
Competition (2)
H 0 = μ 1 ≤ μ2
Ha = μ1 > μ2 (Claim)
= .05
d.f. = 14 + 16 – 2 = 28
1.701
x2 1250ft
s2 = 30 ft
n1 = 14
n2 = 16
n1
x x
0.05
0
x1 1275ft
s1 = 45 ft
1
n1 n2 2
2
t
1 s12 n2 1 s2 2
14 1 45
2
1
1
n1
n2
16 1 30
2
1
1
13.8018
14 16
14 16 2
Decision: Reject H0
At 5% level of significance there
Stat-Test
x
x
1275
1250
0
1
2
1
2
is enough evidence to support
t
1.811 4: 2-SampTTest
4th ed
x x
13.8018
theLarson/Farber
manufacturer’s
claim.
Pooled: Yes 13
1
2
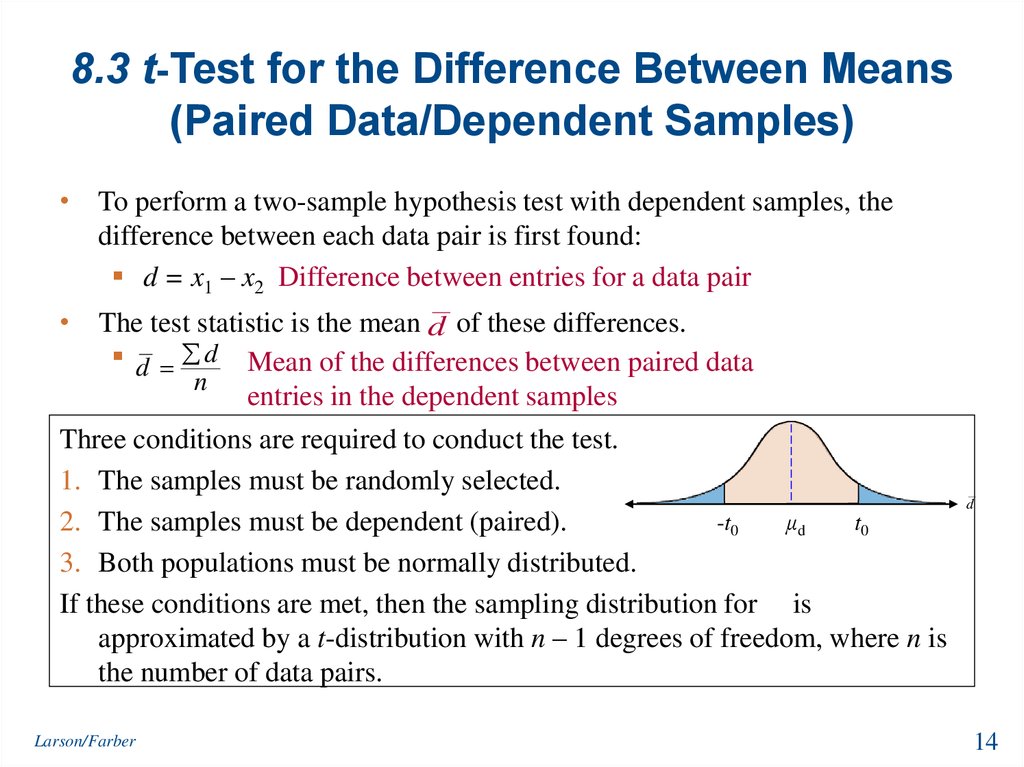
14. 8.3 t-Test for the Difference Between Means (Paired Data/Dependent Samples)
• To perform a two-sample hypothesis test with dependent samples, thedifference between each data pair is first found:
d = x1 – x2 Difference between entries for a data pair
The test statistic is the mean d of these differences.
d d Mean of the differences between paired data
n
entries in the dependent samples
Three conditions are required to conduct the test.
1. The samples must be randomly selected.
-t0
μd
t0
2. The samples must be dependent (paired).
3. Both populations must be normally distributed.
If these conditions are met, then the sampling distribution for is
approximated by a t-distribution with n – 1 degrees of freedom, where n is
the number of data pairs.
Larson/Farber
d
14
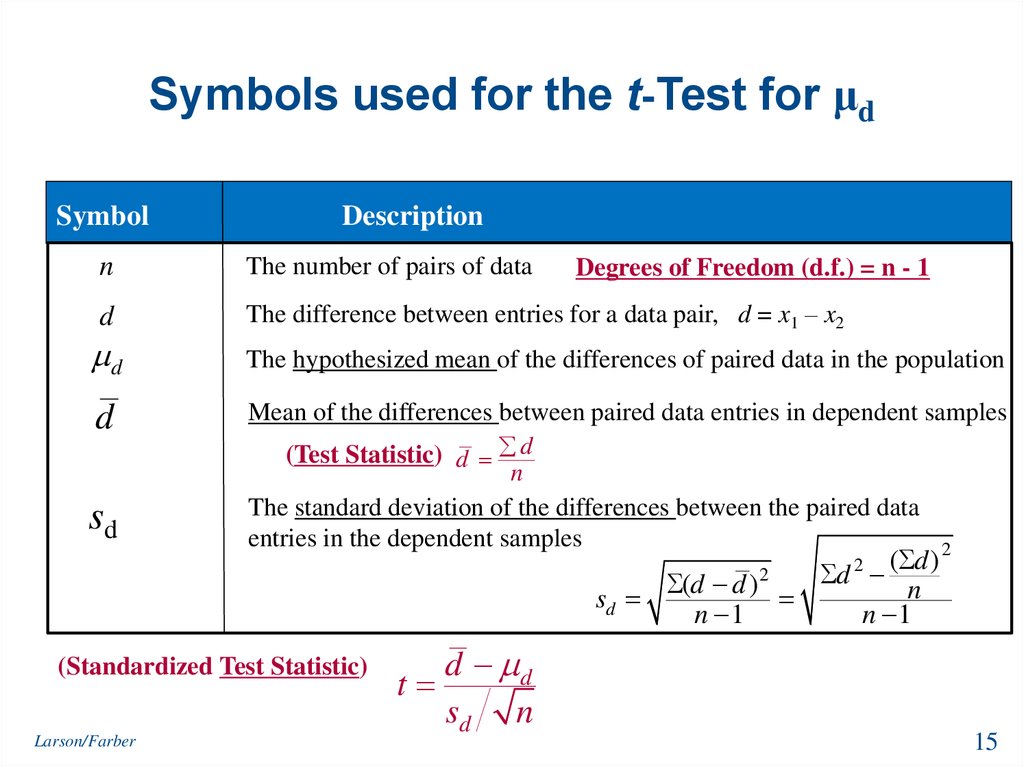
15. Symbols used for the t-Test for μd
SymbolDescription
n
The number of pairs of data
d
The difference between entries for a data pair, d = x1 – x2
d
d
sd
Degrees of Freedom (d.f.) = n - 1
The hypothesized mean of the differences of paired data in the population
Mean of the differences between paired data entries in dependent samples
(Test Statistic) d d
n
The standard deviation of the differences between the paired data
entries in the dependent samples
2
2 ( d )
d
(d d )
n
sd
n 1
n 1
2
(Standardized Test Statistic)
Larson/Farber
d d
t
sd n
15
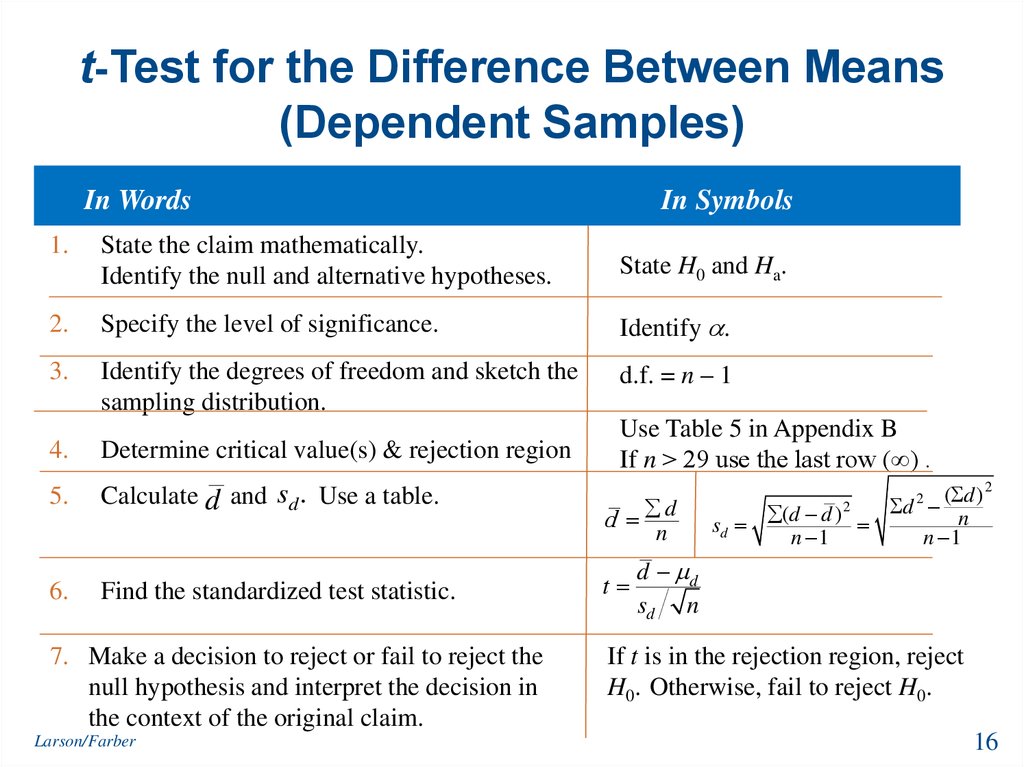
16. t-Test for the Difference Between Means (Dependent Samples)
In WordsIn Symbols
1.
State the claim mathematically.
Identify the null and alternative hypotheses.
State H0 and Ha.
2.
Specify the level of significance.
Identify .
3.
Identify the degrees of freedom and sketch the
sampling distribution.
d.f. = n – 1
4.
Determine critical value(s) & rejection region
5.
Calculate d and sd . Use a table.
Use Table 5 in Appendix B
If n > 29 use the last row (∞) .
d d
n
6.
Find the standardized test statistic.
7. Make a decision to reject or fail to reject the
null hypothesis and interpret the decision in
the context of the original claim.
Larson/Farber
t
( d )
d
(d d )
n
sd
n 1
n 1
2
2
2
d d
sd n
If t is in the rejection region, reject
H0. Otherwise, fail to reject H0.
16
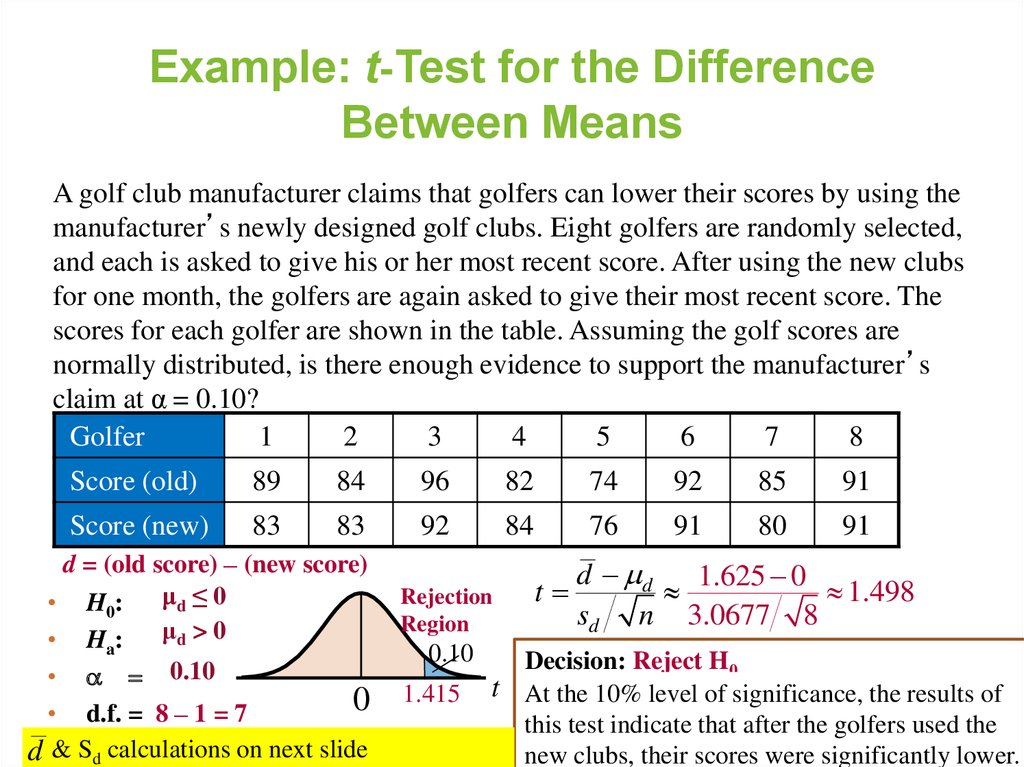
17. Example: t-Test for the Difference Between Means
A golf club manufacturer claims that golfers can lower their scores by using themanufacturer’s newly designed golf clubs. Eight golfers are randomly selected,
and each is asked to give his or her most recent score. After using the new clubs
for one month, the golfers are again asked to give their most recent score. The
scores for each golfer are shown in the table. Assuming the golf scores are
normally distributed, is there enough evidence to support the manufacturer’s
claim at α = 0.10?
Golfer
1
2
3
4
5
6
7
8
Score (old)
89
84
96
82
74
92
85
91
Score (new)
83
83
92
84
76
91
80
91
d = (old score) – (new score)
μd ≤ 0
• H 0:
μd > 0
• H a:
• 0.10
0
• d.f. = 8 – 1 = 7
4th ed
& Sd calculations
on next slide
dLarson/Farber
Rejection
Region
0.10
1.415
t
d d
1.625 0
1.498
sd n 3.0677 8
Decision: Reject H0
t At the 10% level of significance, the results of
this test indicate that after the golfers used the
17
new clubs, their scores were significantly lower.
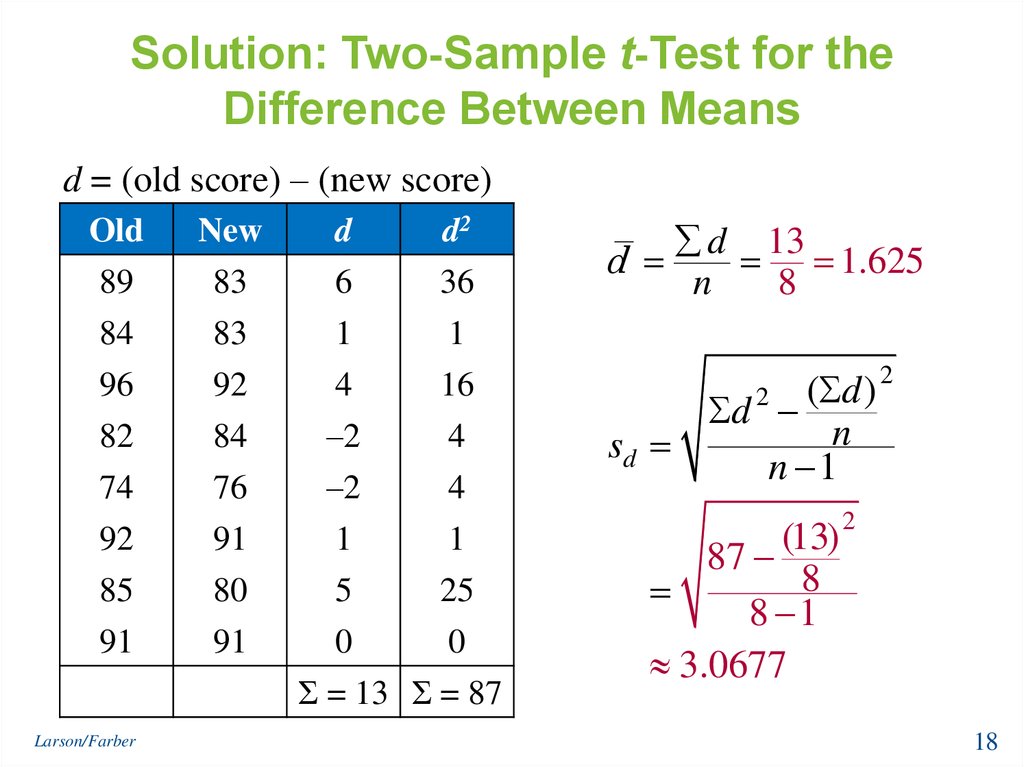
18. Solution: Two-Sample t-Test for the Difference Between Means
d = (old score) – (new score)Old
89
New
83
d
6
d2
36
84
96
82
83
92
84
1
4
–2
1
16
4
74
92
85
76
91
80
–2
1
5
4
1
25
91
91
Larson/Farber
0
0
Σ = 13 Σ = 87
d 13
d
1.625
n
8
2 ( d )
d
n
sd
n 1
2
(13) 2
87
8
8 1
3.0677
18
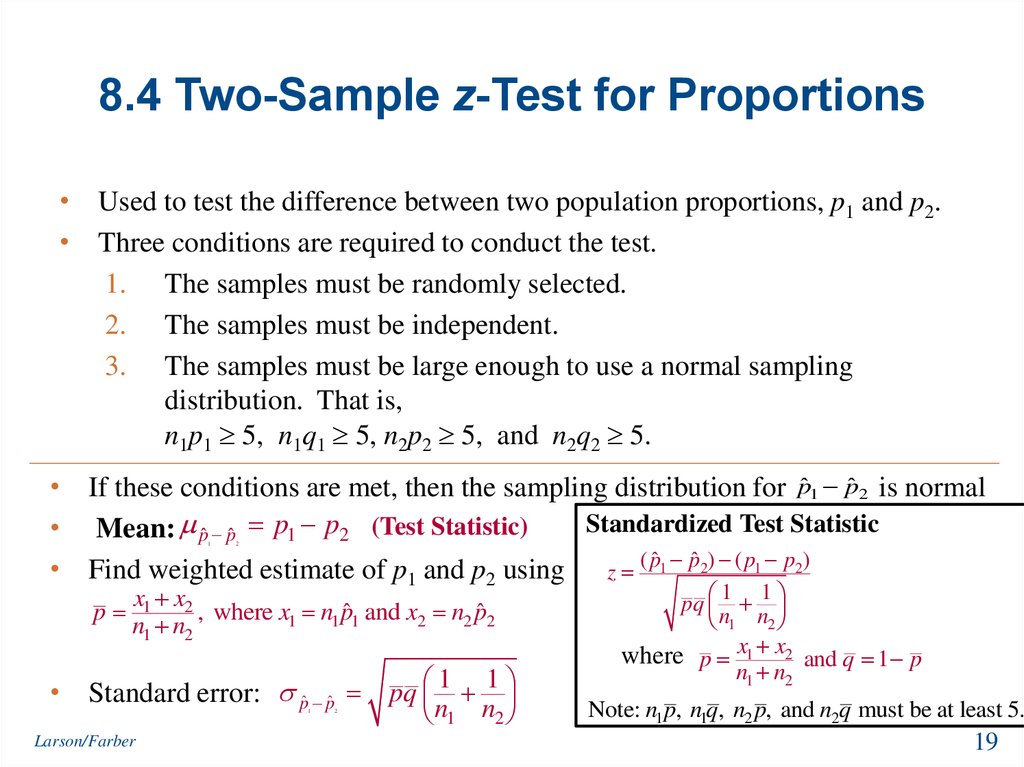
19. 8.4 Two-Sample z-Test for Proportions
• Used to test the difference between two population proportions, p1 and p2.• Three conditions are required to conduct the test.
1. The samples must be randomly selected.
2. The samples must be independent.
3. The samples must be large enough to use a normal sampling
distribution. That is,
n1p1 5, n1q1 5, n2p2 5, and n2q2 5.
• If these conditions are met, then the sampling distribution for pˆ1 pˆ 2 is normal
Standardized Test Statistic
• Mean: pˆ pˆ p1 p2 (Test Statistic)
• Find weighted estimate of p1 and p2 using z ( pˆ1 pˆ 2) ( p1 p2)
1
p
2
x1 x2
, where x1 n1 pˆ1 and x2 n2 pˆ 2
n1 n2
1 1
• Standard error: pˆ pˆ pq
n1 n2
1
Larson/Farber
2
1 1
pq
n1 n2
where p x1 x2 and q 1 p
n1 n2
Note: n1 p, n1q, n2 p, and n2q must be at least 5.
19
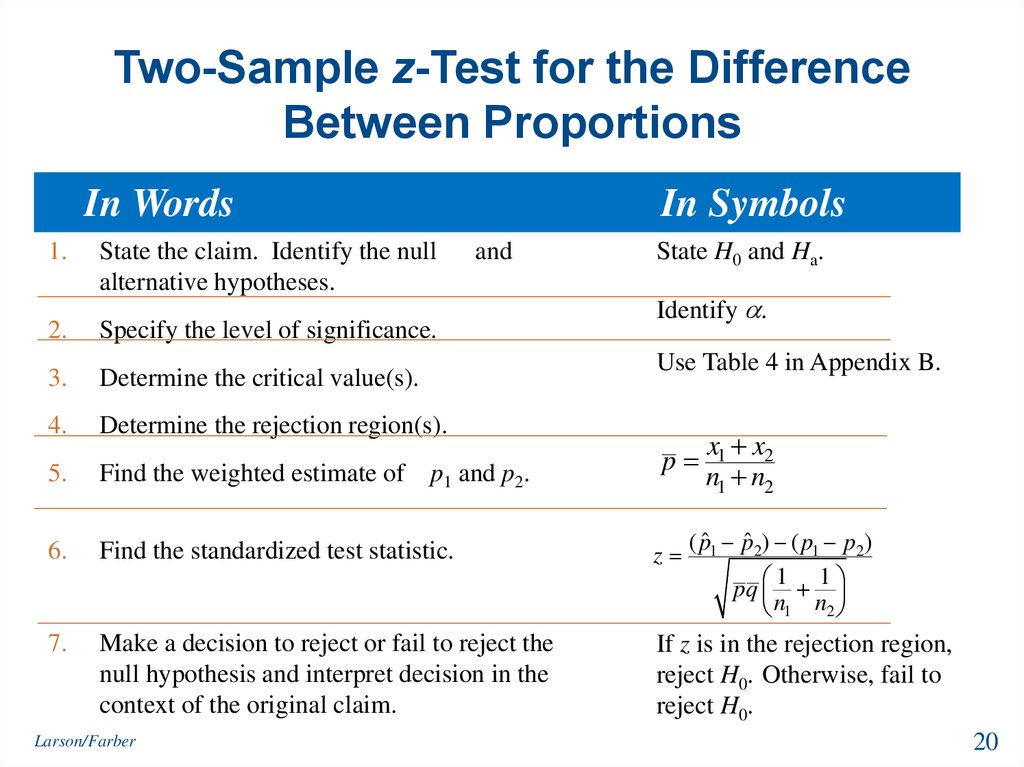
20. Two-Sample z-Test for the Difference Between Proportions
In WordsIn Symbols
1.
State the claim. Identify the null
alternative hypotheses.
2.
Specify the level of significance.
3.
Determine the critical value(s).
4.
Determine the rejection region(s).
and
State H0 and Ha.
Identify .
Use Table 4 in Appendix B.
x1 x2
n1 n2
5.
Find the weighted estimate of
6.
Find the standardized test statistic.
z
7.
Make a decision to reject or fail to reject the
null hypothesis and interpret decision in the
context of the original claim.
If z is in the rejection region,
reject H0. Otherwise, fail to
reject H0.
Larson/Farber
p1 and p2.
p
( pˆ1 pˆ 2) ( p1 p2)
1 1
pq
n1 n2
20
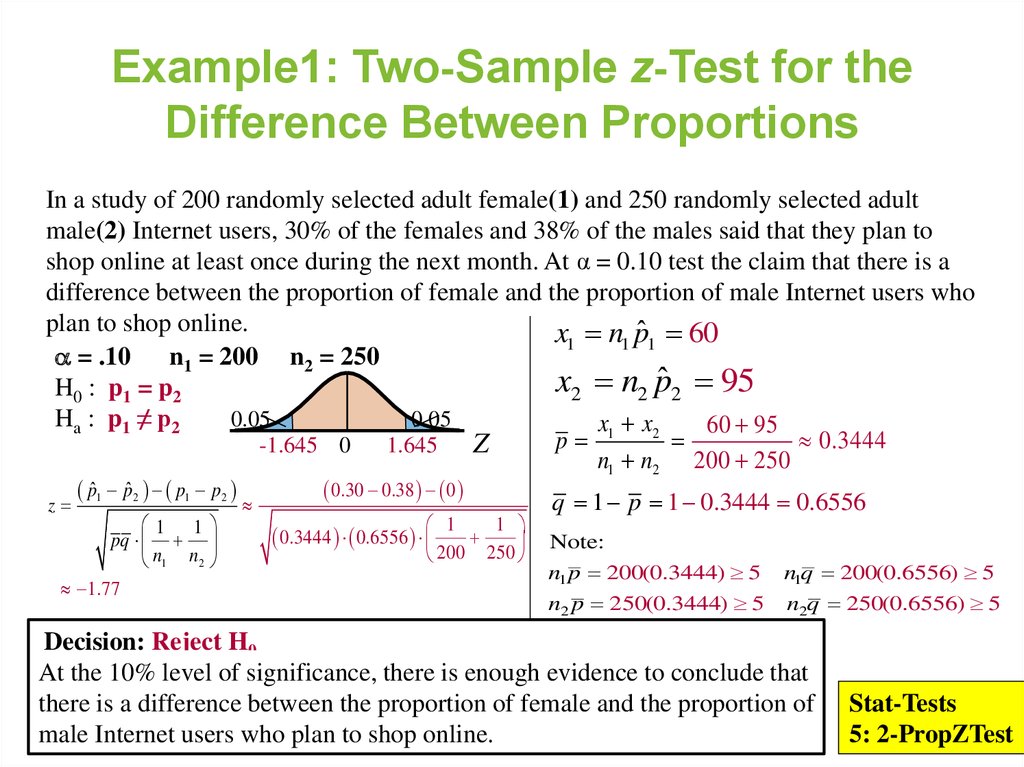
21. Example1: Two-Sample z-Test for the Difference Between Proportions
In a study of 200 randomly selected adult female(1) and 250 randomly selected adultmale(2) Internet users, 30% of the females and 38% of the males said that they plan to
shop online at least once during the next month. At α = 0.10 test the claim that there is a
difference between the proportion of female and the proportion of male Internet users who
plan to shop online.
x1 n1 pˆ1 60
= .10
n1 = 200 n2 = 250
x2 n2 pˆ 2 95
H0 : p1 = p2
0.05
0.05
Ha : p1 ≠ p2
x1 x2
60 95
p
0.3444
Z
-1.645 0
1.645
n1 n2 200 250
pˆ1 pˆ 2 p1 p2
0.30 0.38 0
q 1 p 1 0.3444 0.6556
z
1 1
pq
n1 n2
1.77
0.3444 0.6556
1
1
200 250
Note:
n1 p 200(0.3444) 5
n1q 200(0.6556) 5
n2 p 250(0.3444) 5
n2q 250(0.6556) 5
Decision: Reject H0
At the 10% level of significance, there is enough evidence to conclude that
there is a difference between the proportion of female and the proportion of
male Internet
Larson/Farber
4th edusers who plan to shop online.
Stat-Tests
5: 2-PropZTest
21
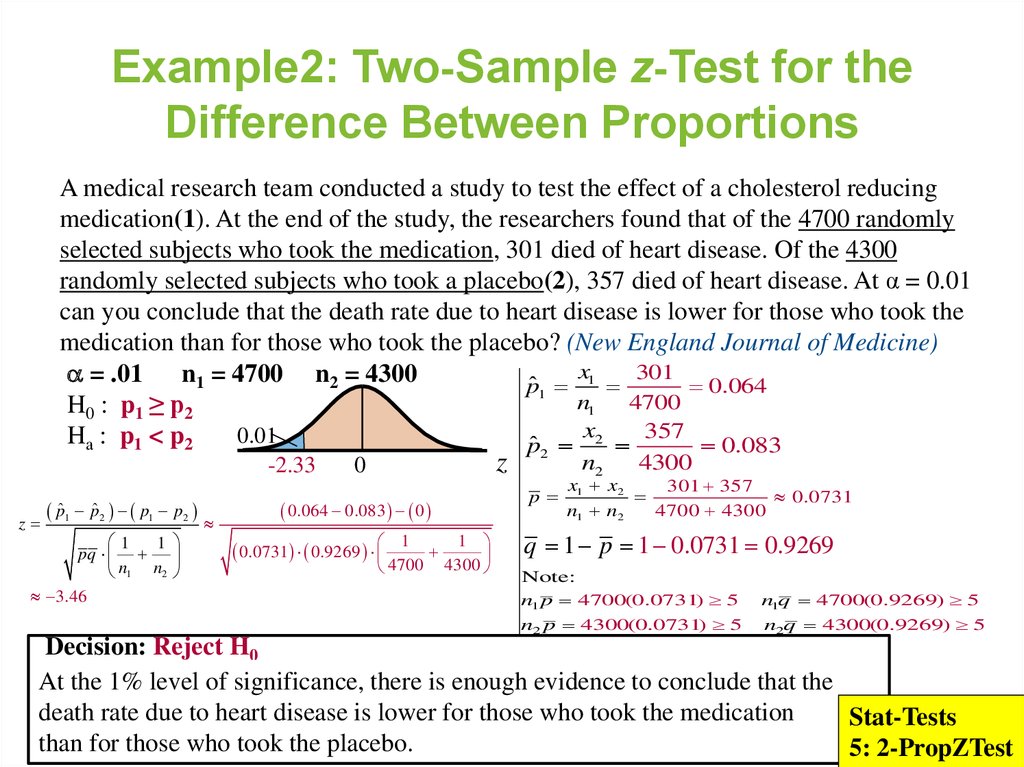
22. Example2: Two-Sample z-Test for the Difference Between Proportions
A medical research team conducted a study to test the effect of a cholesterol reducingmedication(1). At the end of the study, the researchers found that of the 4700 randomly
selected subjects who took the medication, 301 died of heart disease. Of the 4300
randomly selected subjects who took a placebo(2), 357 died of heart disease. At α = 0.01
can you conclude that the death rate due to heart disease is lower for those who took the
medication than for those who took the placebo? (New England Journal of Medicine)
x
301
= .01
n1 = 4700 n2 = 4300
ˆ1 1
p
0.064
n1
4700
H0 : p1 ≥ p2
x
357
0.01
Ha : p1 < p2
ˆ 2
p
0.083
-2.33
z
pˆ1 pˆ 2 p1 p2
1
1
pq
n1 n2
3.46
z
0
0.064 0.083 0
0.0731 0.9269
1
1
4700 4300
2
p
n2
4300
x1 x2
301 357
0.0731
n1 n2
4700 4300
q 1 p 1 0.0731 0.9269
Note:
n1 p 4700(0.0731) 5
n1q 4700(0.9269) 5
n2 p 4300(0.0731) 5
n2q 4300(0.9269) 5
Decision: Reject H0
At the 1% level of significance, there is enough evidence to conclude that the
death rate due to heart disease is lower for those who took the medication
Stat-Tests
Larson/Farber
4th ed who took the placebo.
22
than for those
5: 2-PropZTest


 Математика
Математика








