Введение в техническое зрение

1. Введение в техническое зрение

Степанов Дмитрий Николаевич
Начальник лаборатории систем технического зрения
[email protected]
Image by
kirkh.deviantart.com

2.

Дальше
Модель камеры
Камера и человеческий глаз
Восприятие света и цвета
Пиксели
Фильтрация изображений
Практическое задание: линейные фильтры

3. Проективная геометрия и модели камеры

Сегодняшнее занятие
Проективная геометрия и
модели камеры
Slides from Derek Hoiem,
Alexei Efros, Steve Seitz, and
David Forsyth

4.

Что нужно, чтобы сделать камеру?

5.

Камера и реальный мир
Какого роста женщина?
Как высоко установлена камера?
Как камера повёрнута?
Каково фокусное расстояние?
Который мяч ближе?

6.

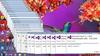
Формирование изображения
Давайте сделаем камеру
– Идея 1: поместим плёнку перед объектом
– Получим ли мы изображение? Почему?
Slide source: Seitz

7.

Камера-обскура
Идея 2: добавим барьер и отсечем почти
все лучи
– Это уменьшит размытие
– Отверстие известно как диафрагма (aperture)
Slide source: Seitz

8.

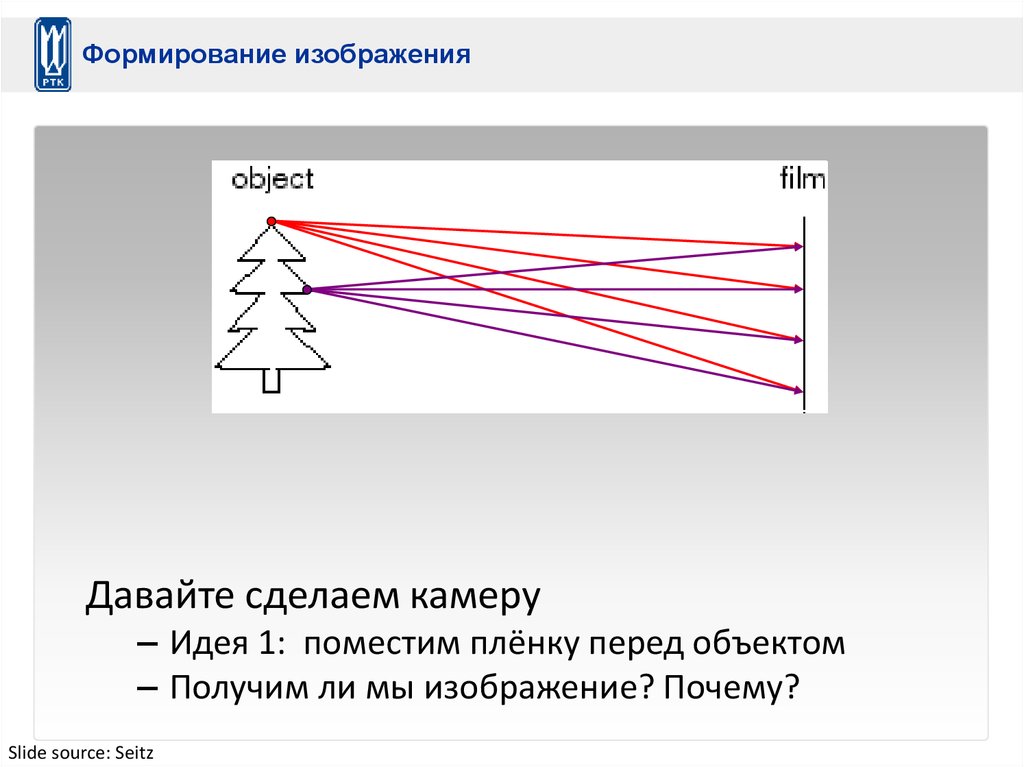
Камера-обскура: модель
f
c
f = фокусное расстояние
c = центр камеры (центр проекции)
Image plane = картинная плоскость
Figure from Forsyth

9.

Камера-обскура: пре-камера
• Известна со времен Древнего Китая и Греции
(Mo-Ti, China, 470BC - 390BC)
Иллюстрация Camera obscura
Настоящая камера обскура
в Университете Северной Каролины
Photo by Seth Ilys
(лат. camera obscūra — «тёмная комната»)

10.

Камера-обскура: применение (обводка)
Lens Based Camera Obscura, 1568

11.

Первые фотографии: Ньепс, 8 часов
Самая старая сохранившаяся
фотография
Фотография первой фотографии
– 8 часов, пьютерная (оловянная)
пластина
Жозеф Ньепс, 1826
Университет Техаса, Остин
Ньепс позже работал с Луи Дагером над созданием дагеротипов

12.

Первые фотографии: дагерротип, 20 минут
1837
1864

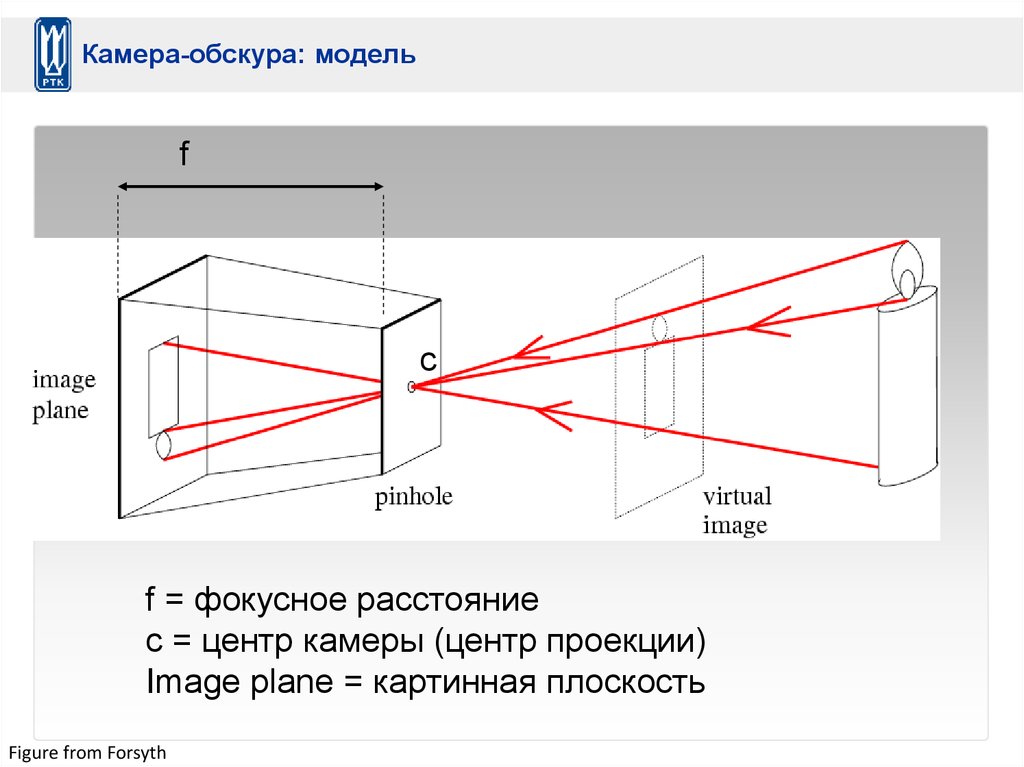
13. Изображение – отображение сцены на плоскости

Машина для уменьшения размерности
Изображение – отображение
сцены на плоскости
Трехмерный мир
Двумерное изображение
Point of observation
Figures © Stephen E. Palmer, 2002

14.

Slide source: Seitz
Проекция может быть обманчива

15.

Slide source: Seitz
Проекция может быть обманчива

16.

Проективная геометрия
Что теряется?
• Длина (расстояние)
Кто выше?
Которые ближе?

17.

Расстояние не сохраняется
A’
C’
B’
Figure by David Forsyth

18.

Проективная геометрия
Что теряется?
• Длина
• Углы
Параллельные?
Перпендикулярные?

19.

Проективная геометрия
Что сохраняется?
• Прямые линии остаются прямыми

20.

Точки схода
Параллельные в реальности линии пересекаются на
кадре в точках схода, образующих…

21.

Точки схода и линия горизонта
Параллельные в реальности линии пересекаются на
кадре в точках схода, образующих…
линию горизонта

22.

Линия горизонта
Точка схода
Линия горизонта
o
Точка схода
o

23.

Линия горизонта
Вертикальная
точка схода
(в бесконечности)
Линия
горизонта
Точка
схода
Slide from Efros, Photo from Criminisi
Точка схода

24.

Линия горизонта

25.

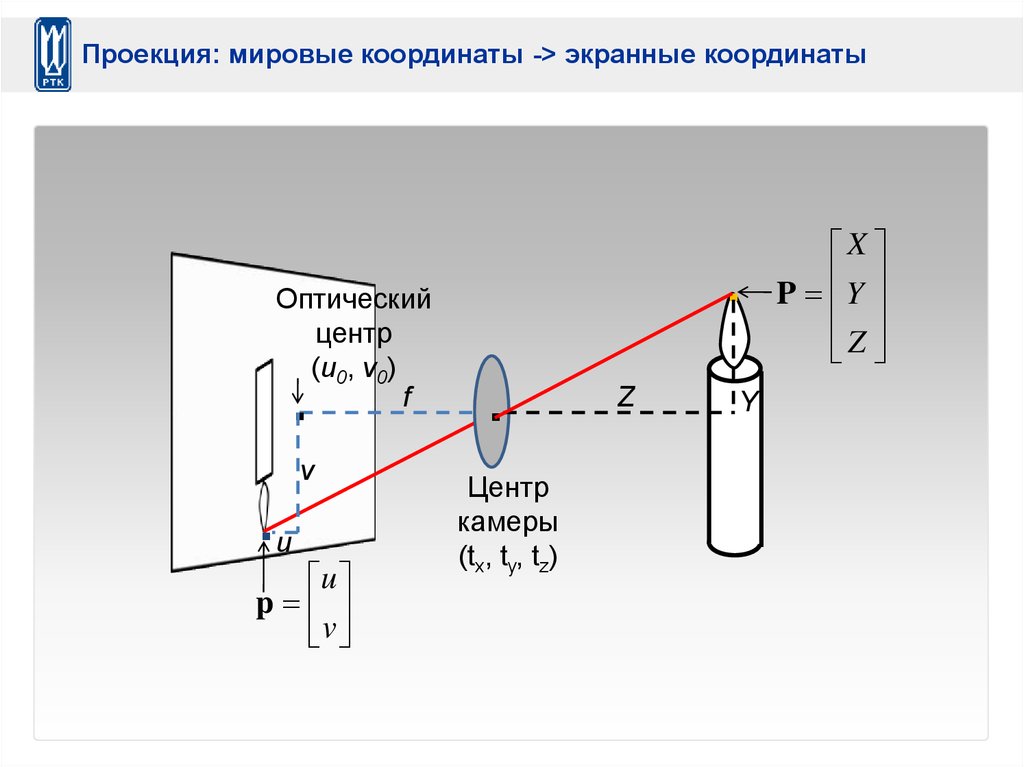
Проекция: мировые координаты -> экранные координаты
Оптический
центр
(u0, v0)
f
.
.
v
u
u
p
v
.
.
Центр
камеры
(tx, ty, tz)
Z
Y
X
P Y
Z

26.

Однородные координаты
Преобразование к однородным координатам
Однородные
экранные координаты
Однородные
мировые координаты
Преобразование ИЗ однородных координат

27.

Однородные координаты
Инвариантность к масштабу
x kx
kx
x
kw
w
k y ky ky y
w kw kw w
Однородные
координаты
Декартовы
координаты
Точке в декартовых координатах соответствует
луч в однородных

28.

Базовая геометрия однородных координат
• Уравнение прямой:
ax + by + c = 0
• Добавить «1» для перехода
в однородные координаты
ai
line i bi
ci
ui
pi vi
1
• Прямая получается как векторное
произведение двух точек
line ij pi p j
• Пересечение прямых получается как
векторное произведение прямых
qij linei line j

29.

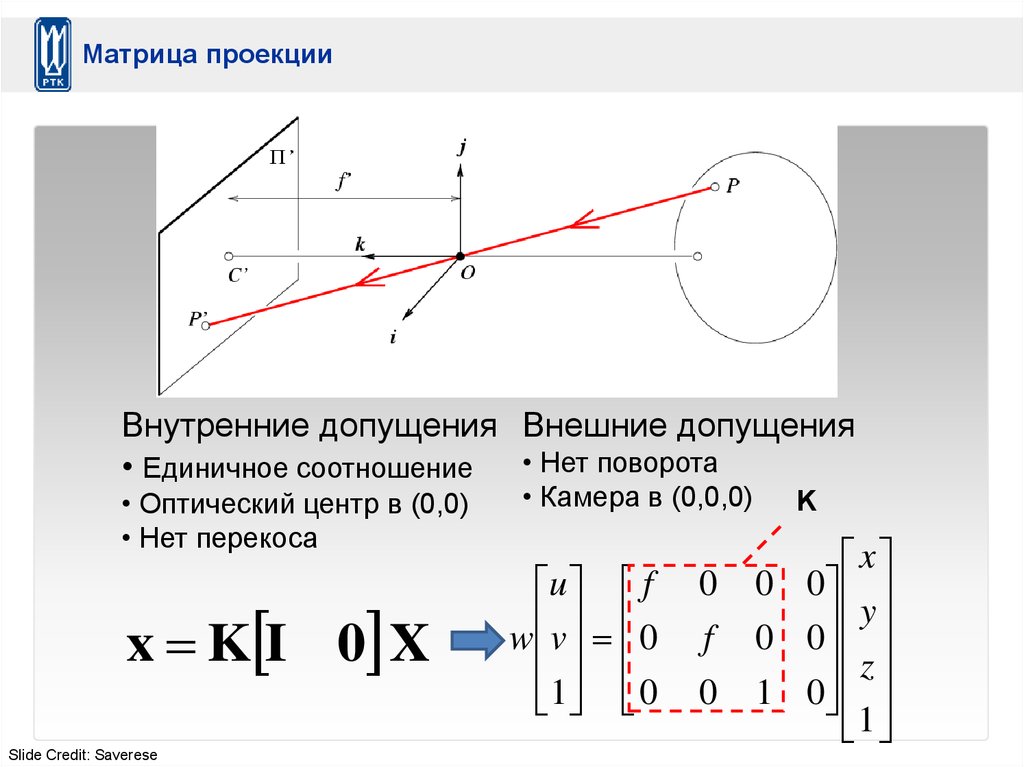
Slide Credit: Saverese
Матрица проекции
R,T
jw
kw
Ow
iw
x K R t X
x: Экранные координаты: (u,v,1)
K: Внутренняя матрица (3x3)
R: Вращение (3x3)
t: Перенос (3x1)
X: Мировые координаты: (X,Y,Z,1)

30.

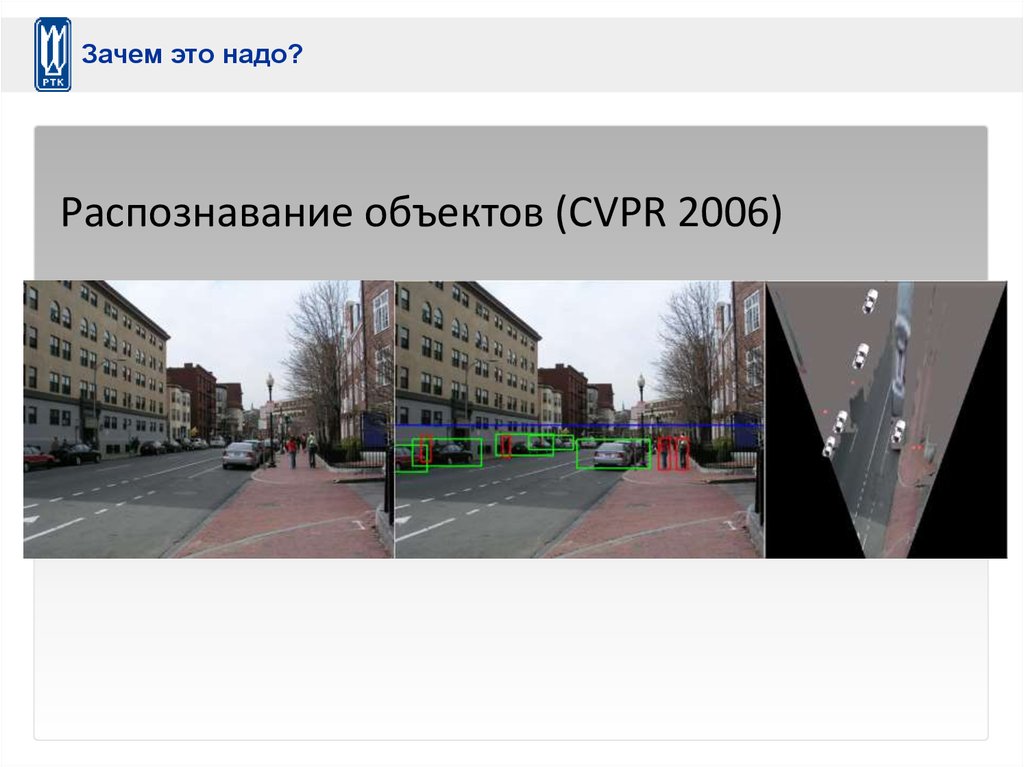
Зачем это надо?
Соотнесение множества видов

31.

Зачем это надо?
Распознавание объектов (CVPR 2006)

32. Соотнесение множества видов

Зачем это надо?
Дополненная реальность (SIGGRAPH 2007)
Оригинал
Дополнение

33.

Матрица проекции
Внутренние допущения Внешние допущения
• Единичное соотношение • Нет поворота
• Оптический центр в (0,0)
• Нет перекоса
x K I 0 X
Slide Credit: Saverese
• Камера в (0,0,0)
u f
w v 0
1 0
0
f
0
K
x
0 0
y
0 0
z
1 0
1

34.

Убираем допущения: известный оптический центр
Внутренние допущения Внешние допущения
• Единичное соотношение
• Нет перекоса
x K I 0 X
• Нет поворота
• Камера в (0,0,0)
u f
w v 0
1 0
0
u0
f
v0
0
1
x
0
y
0
z
0
1

35.

Убираем допущения: квадратные пиксели
Внутренние допущения Внешние допущения
• Нет перекоса
x K I 0 X
• Нет поворота
• Камера в (0,0,0)
u
w v 0
1 0
0
u0
v0
0
1
x
0
y
0
z
0
1

36.

Убираем допущения: прямые пиксели
Внутренние допущения Внешние допущения
• Нет поворота
• Камера в (0,0,0)
x K I 0 X
u
w v 0
1 0
s
u0
v0
0
1
x
0
y
0
z
0
1
Note: different books use different notation for parameters

37.

Убираем допущения: перенос и поворот камеры
R
jw
t
kw
Ow
iw

38.

Убираем допущения: перенос камеры
Внутренние допущения Внешние допущения
• Нет поворота
x K I t X
u
w v 0
1 0
0
0
x
u0 1 0 0 t x
y
v0 0 1 0 t y
z
1 0 0 1 t z
1

39.

Slide Credit: Saverese
Трехмерный поворот точек
Поворот вокруг координатных осей, против час.стрелки:
0
1
Rx ( ) 0 cos
0 sin
p’
y
z
p
0
sin
cos
cos 0 sin
R y ( ) 0
1
0
sin 0 cos
cos sin 0
Rz ( ) sin cos 0
0
0
1

40.

Убираем допущения: разрешаем поворот камеры
x K R t X
u
w v 0
1 0
s
0
u0 r11 r12
v0 r21 r22
1 r31 r32
r13
r23
r33
x
tx
y
ty
z
t z
1

41.

Степени свободы
x K R t X
5
u
w v 0
1 0
s
0
6
u0 r11 r12
v0 r21 r22
1 r31 r32
r13
r23
r33
x
tx
y
ty
z
t z
1

42.

Ортографическая проекция
• Особый случай перспективной проекции
Image
World
x
– Также называется параллельной u 1 0 0 0
y
проекцией
w v 0 1 0 0
z
– Какова матрица проекции?
1 0 0 0 1
1
Slide by Steve Seitz

43.

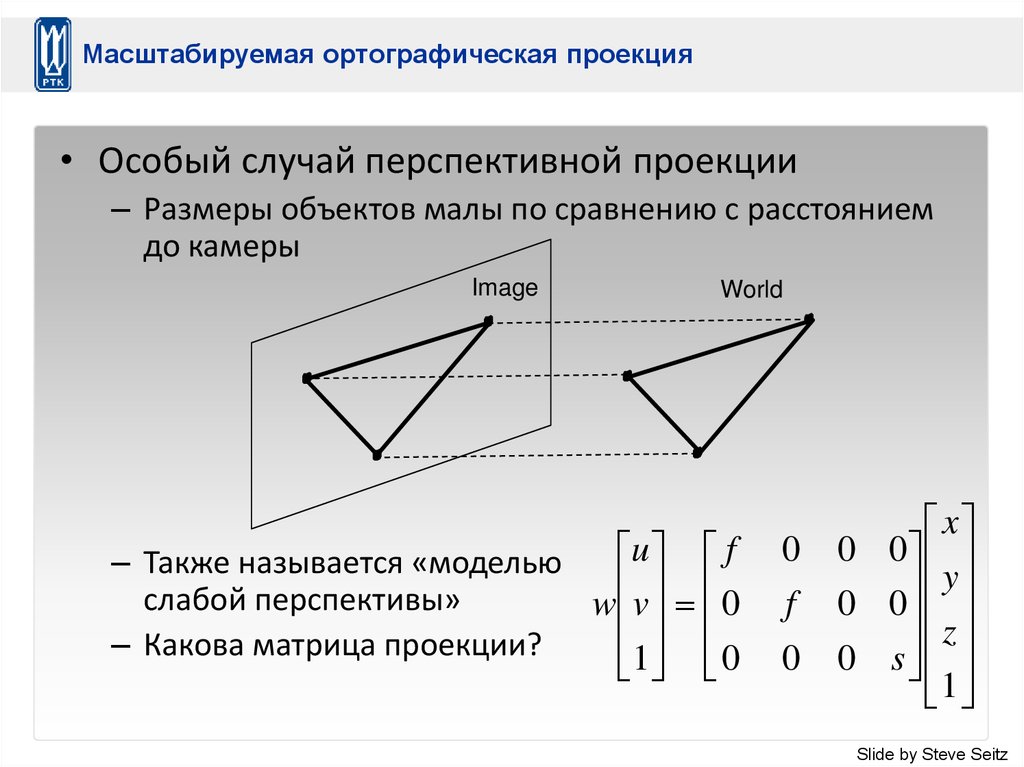
Масштабируемая ортографическая проекция
• Особый случай перспективной проекции
– Размеры объектов малы по сравнению с расстоянием
до камеры
Image
World
u f
– Также называется «моделью
слабой перспективы»
w v 0
– Какова матрица проекции?
1 0
0
f
0
x
0 0
y
0 0
z
0 s
1
Slide by Steve Seitz

44. Vanishing Point = Projection from Infinity

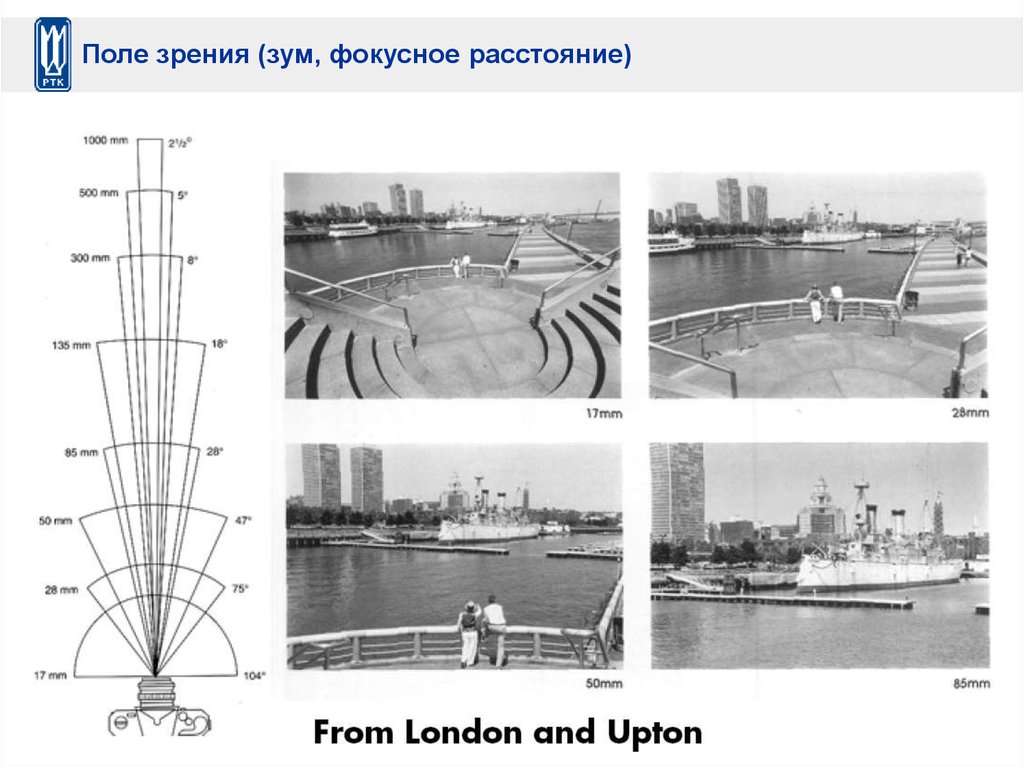
Поле зрения (зум, фокусное расстояние)

45.

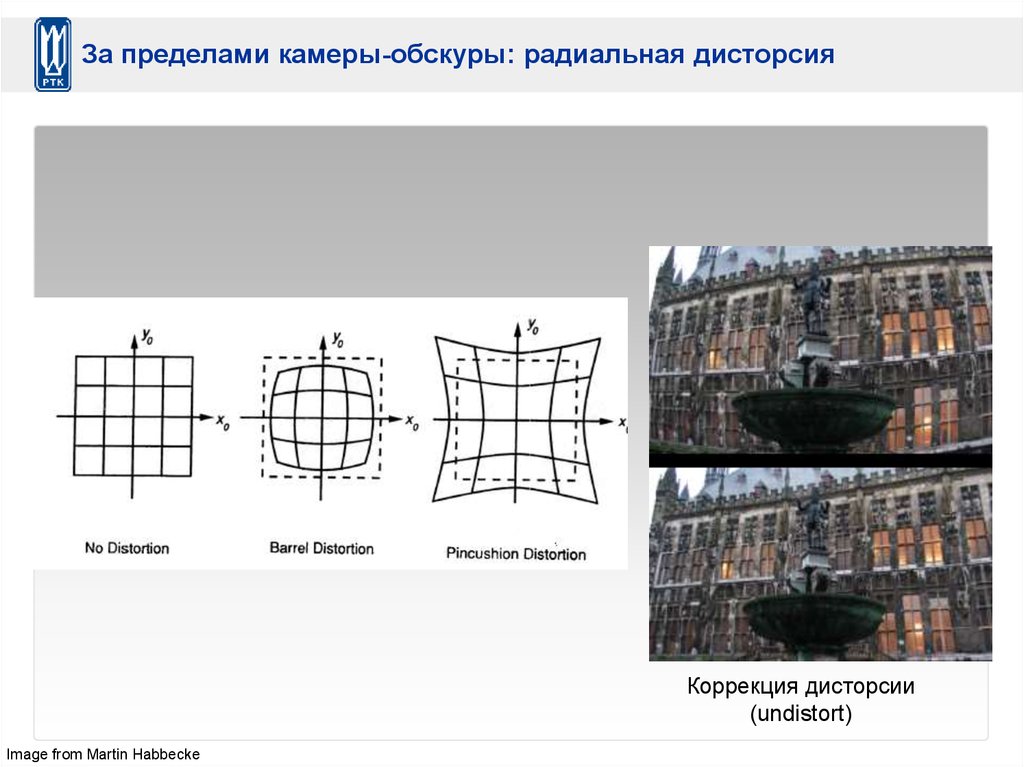
За пределами камеры-обскуры: радиальная дисторсия
Коррекция дисторсии
(undistort)
Image from Martin Habbecke

46.

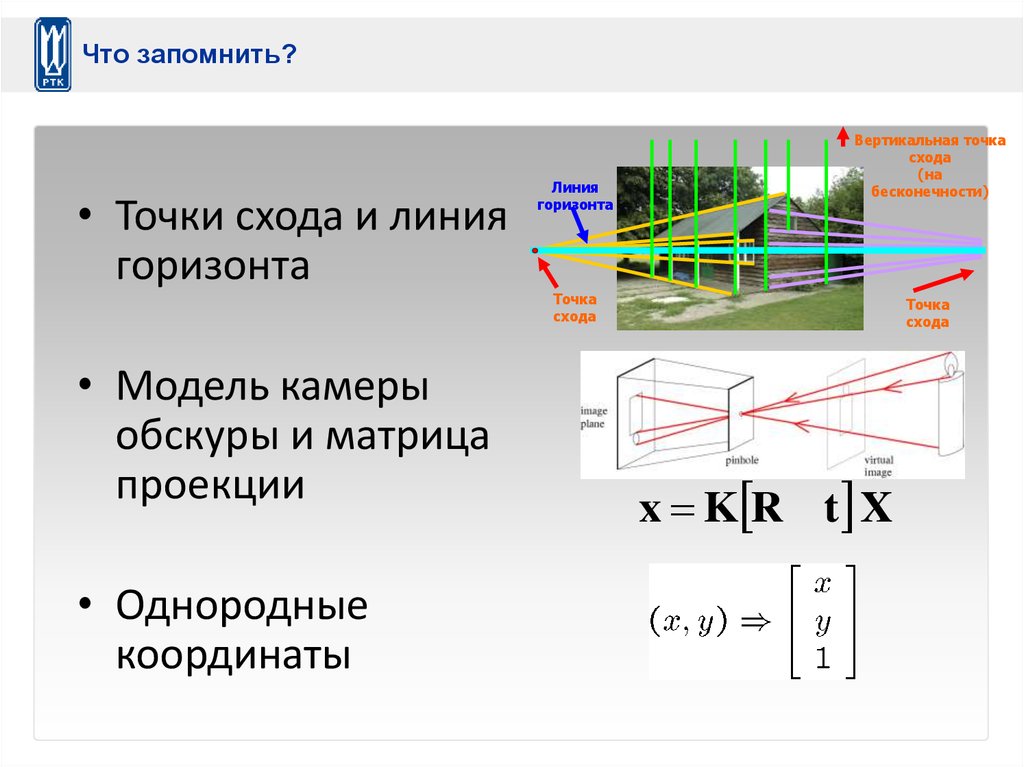
Что запомнить?
• Точки схода и линия
горизонта
• Модель камеры
обскуры и матрица
проекции
• Однородные
координаты
Линия
горизонта
Вертикальная точка
схода
(на
бесконечности)
Точка
схода
Точка
схода
x K R t X

47.

Домашнее задание
Сделать из «зеркалки» камеру обскура
Измерить угол зрения камеры

48.

Adding a lens
“circle of
confusion”
• A lens focuses light onto the film
– There is a specific distance at which objects are
“in focus”
• other points project to a “circle of confusion” in the
image

49.

Focal length, aperture, depth of
field
F
focal point
optical center
(Center Of Projection)
• A lens focuses parallel rays onto a single focal
point
– focal point at a distance f beyond the plane of the lens
– Aperture of diameter D restricts the range of rays
Slide source: Seitz

50.

Slide source: Seitz
Depth of field
f / 5.6
f / 32
Changing the aperture size or focal
length affects depth of field
Flower images from Wikipedia
http://en.wikipedia.org/wiki/Depth_of_field

51.

Shrinking the aperture
• Why not make the aperture as small as
possible?
– Less light gets through
Slide by Steve Seitz

52. Adding a lens

Shrinking the aperture
Slide by Steve Seitz

53. Focal length, aperture, depth of field

Capturing Light… in man and
machine
Many slides by
Alexei A. Efros
CS 143: Computer Vision
James Hays, Brown, Fall 2013

54. Depth of field

Image Formation
Digital Camera
Film
The Eye

55. Shrinking the aperture

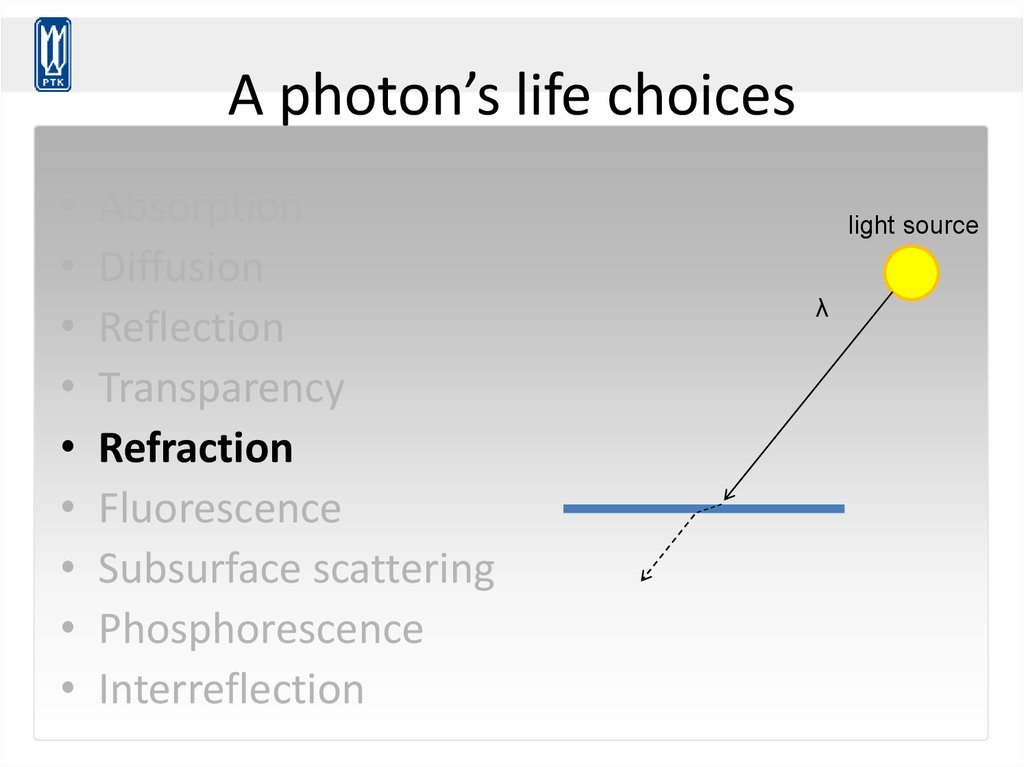
A photon’s life choices
Absorption
Diffusion
Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
λ
?

56. Shrinking the aperture

A photon’s life choices
Absorption
Diffusion
Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
λ

57. Capturing Light… in man and machine

A photon’s life choices
Absorption
Diffuse Reflection
Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
λ

58. Image Formation

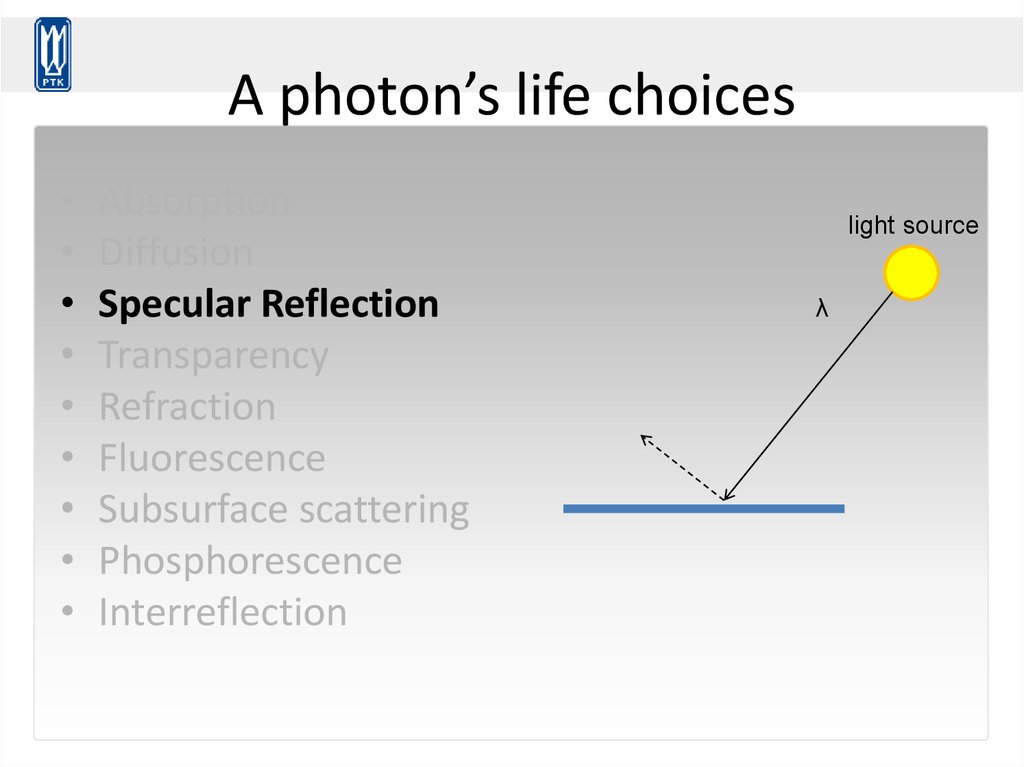
A photon’s life choices
Absorption
Diffusion
Specular Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
λ

59. A photon’s life choices

Absorption
Diffusion
Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
λ

60. A photon’s life choices

Absorption
Diffusion
Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
λ

61. A photon’s life choices

Absorption
Diffusion
Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
λ1
λ2

62. A photon’s life choices

Absorption
Diffusion
Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
λ

63. A photon’s life choices

Absorption
Diffusion
Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
t=1
t=n

64. A photon’s life choices

Absorption
Diffusion
Reflection
Transparency
Refraction
Fluorescence
Subsurface scattering
Phosphorescence
Interreflection
light source
λ
(Specular Interreflection)

65. A photon’s life choices

Lambertian Reflectance
• In computer vision, surfaces are often
assumed to be ideal diffuse reflectors with
know dependence on viewing direction.

66. A photon’s life choices

Обратная трассировка лучей

67. A photon’s life choices

Digital camera
• A digital camera replaces film with a sensor
array
– Each cell in the array is light-sensitive diode that converts photons to electrons
– Two common types
• Charge Coupled Device (CCD)
• CMOS
– http://electronics.howstuffworks.com/digital-camera.htm
Slide by Steve Seitz

68. A photon’s life choices

Sensor Array
CMOS sensor

69. Lambertian Reflectance

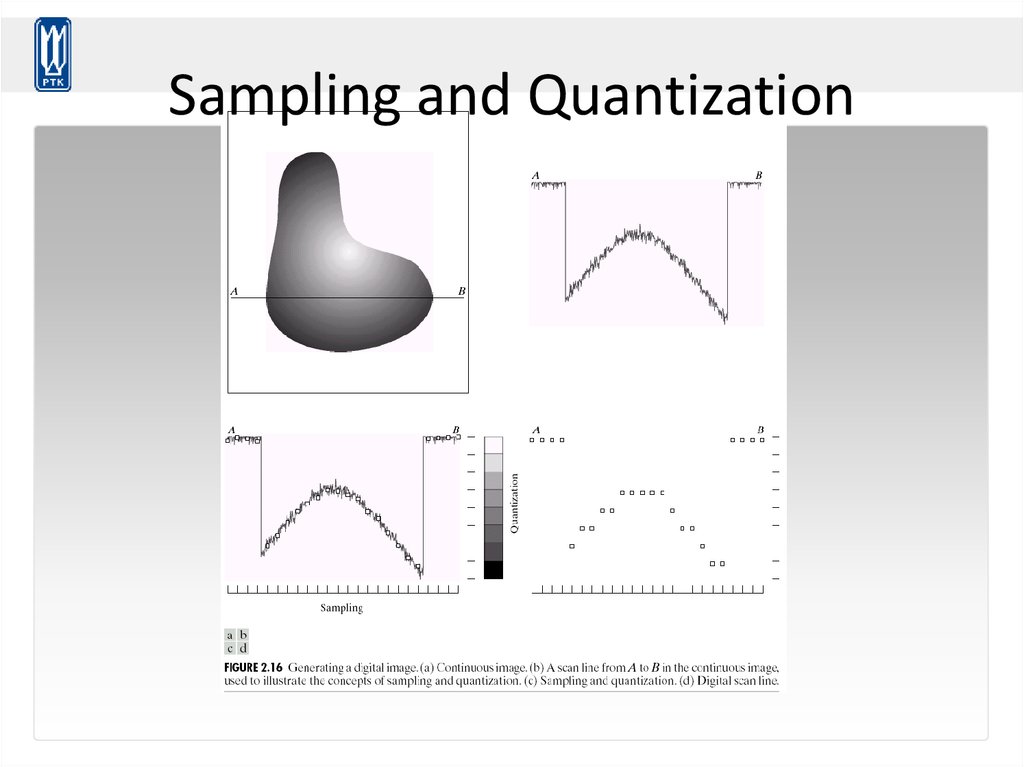
Sampling and Quantization

70. Обратная трассировка лучей

Interlace vs. progressive scan
http://www.axis.com/products/video/camera/progressive_scan.htm
Slide by Steve Seitz

71. Digital camera

Progressive scan
http://www.axis.com/products/video/camera/progressive_scan.htm
Slide by Steve Seitz

72. Sensor Array

Interlace
http://www.axis.com/products/video/camera/progressive_scan.htm
Slide by Steve Seitz

73. Sampling and Quantization

Rolling Shutter

74. Interlace vs. progressive scan

The Eye
• The human eye is a camera!
– Iris - colored annulus with radial muscles
– Pupil - the hole (aperture) whose size is controlled by the iris
– photoreceptor cells (rods and cones) in the retina
– What’s the “film”?
Slide by Steve Seitz

75. Progressive scan

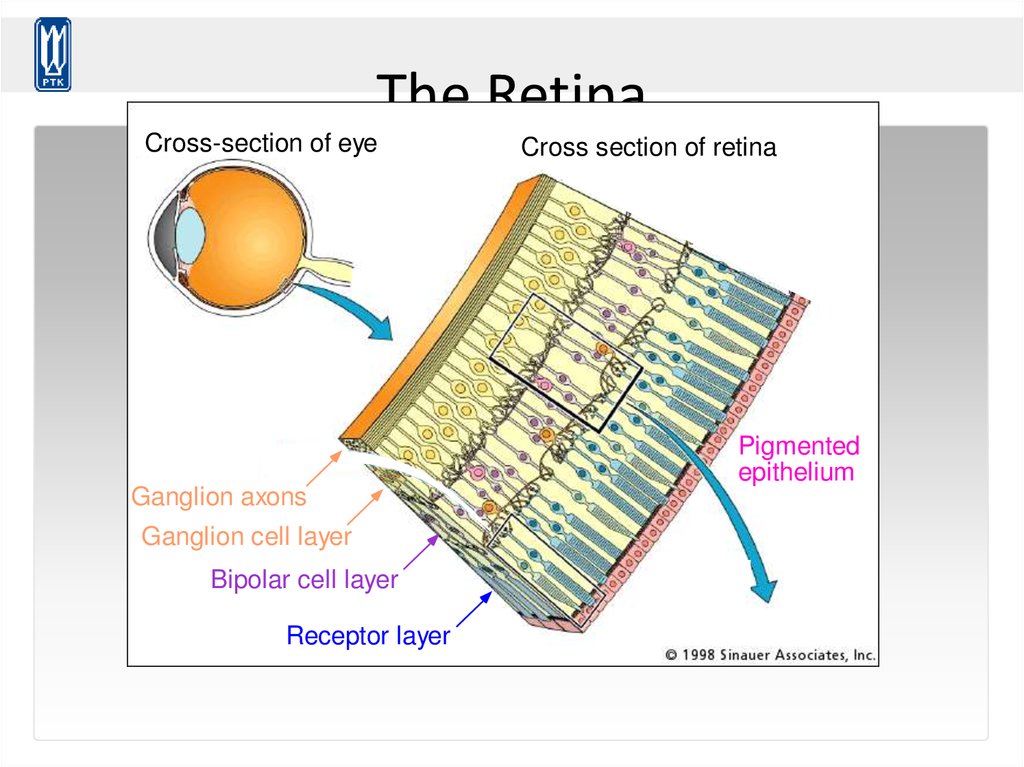
The Retina
Cross-section of eye
Cross section of retina
Pigmented
epithelium
Ganglion axons
Ganglion cell layer
Bipolar cell layer
Receptor layer

76. Interlace

What humans don’t have: tapetum
lucidum

77. Rolling Shutter

Two types of light-sensitive receptors
Cones
cone-shaped
less sensitive
operate in high light
color vision
Rods
rod-shaped
highly sensitive
operate at night
gray-scale vision
© Stephen E. Palmer, 2002

78. The Eye

Rod / Cone sensitivity

79. The Retina

Distribution of Rods and Cones
# Receptors/mm2
.
Fovea
150,000
Rods
Blind
Spot
Rods
100,000
50,000
0
Cones
Cones
80 60 40 20 0
20 40 60 80
Visual Angle (degrees from fovea)
Night Sky: why are there more stars off-center?
Averted vision: http://en.wikipedia.org/wiki/Averted_vision
© Stephen E. Palmer, 2002

80. Retina up-close

Eye Movements
• Saccades
Can be consciously controlled. Related to perceptual attention.
200ms to initiation, 20 to 200ms to carry out. Large amplitude.
• Microsaccades
Involuntary. Smaller amplitude. Especially evident during
prolonged fixation. Function debated.
• Ocular microtremor (OMT)
involuntary. high frequency (up to 80Hz), small amplitude.

81. What humans don’t have: tapetum lucidum

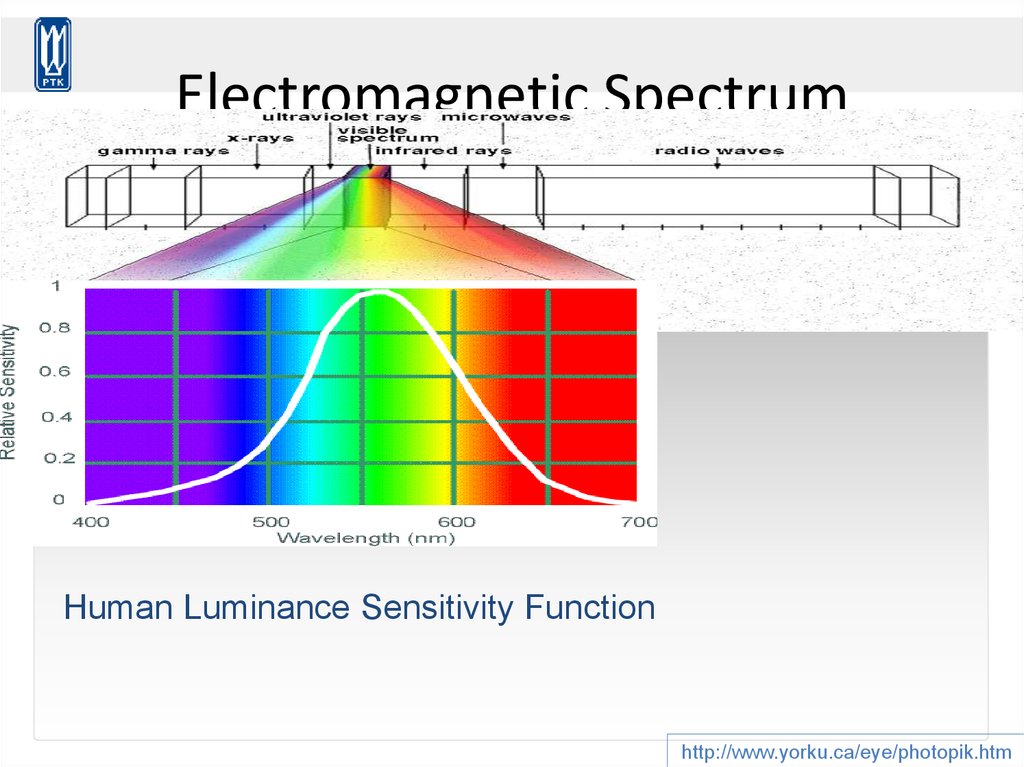
Electromagnetic Spectrum
Human Luminance Sensitivity Function
http://www.yorku.ca/eye/photopik.htm

82.

Visible Light
Why do we see light of these wavelengths?
…because that’s where the
Sun radiates EM energy
© Stephen E. Palmer, 2002

83. Rod / Cone sensitivity

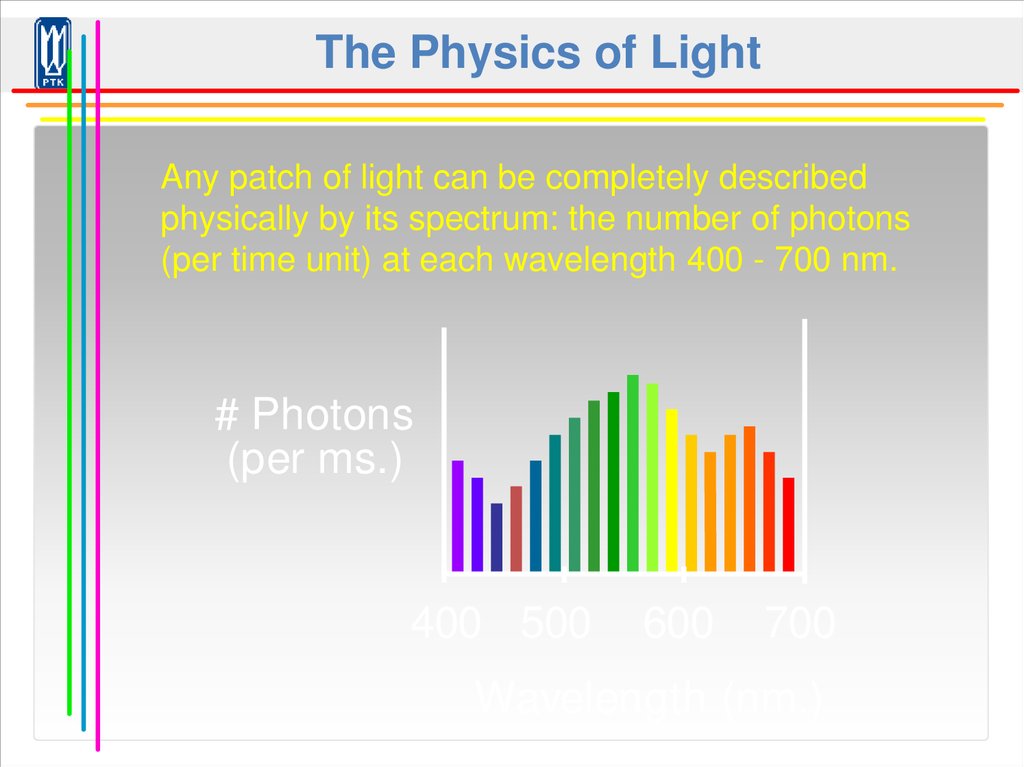
The Physics of Light
Any patch of light can be completely described
physically by its spectrum: the number of photons
(per time unit) at each wavelength 400 - 700 nm.
# Photons
(per ms.)
400 500
600
700
Wavelength (nm.)
© Stephen E. Palmer, 2002

84.

The Physics of Light
Some examples of the spectra of light sources
.
B. Gallium Phosphide Crystal
# Photons
# Photons
A. Ruby Laser
400 500
600
700
400 500
Wavelength (nm.)
700
Wavelength (nm.)
D. Normal Daylight
# Photons
C. Tungsten Lightbulb
# Photons
600
400 500
600
700
400 500
600
700
© Stephen E. Palmer, 2002

85. Eye Movements

The Physics of Light
% Photons Reflected
Some examples of the reflectance spectra of surfaces
Red
400
Yellow
700 400
Blue
700 400
Wavelength (nm)
Purple
700 400
700
© Stephen E. Palmer, 2002

86. Electromagnetic Spectrum

The Psychophysical Correspondence
There is no simple functional description for the perceived
color of all lights under all viewing conditions, but …...
A helpful constraint:
Consider only physical spectra with normal distributions
mean
area
# Photons
400
500
variance
600
700
Wavelength (nm.)
© Stephen E. Palmer, 2002

87.

The Psychophysical Correspondence
# Photons
Mean
blue
Hue
green yellow
Wavelength
© Stephen E. Palmer, 2002

88.

The Psychophysical Correspondence
# Photons
Variance
Saturation
hi. high
med. medium
low
low
Wavelength
© Stephen E. Palmer, 2002

89.

The Psychophysical Correspondence
Area
Brightness
# Photons
B. Area
Lightness
bright
dark
Wavelength
© Stephen E. Palmer, 2002

90.

Physiology of Color Vision
Three kinds of cones:
440
RELATIVE ABSORBANCE (%)
.
530 560 nm.
100
S
M
L
50
400
450
500
550
600 650
WAVELENGTH (nm.)
• Why are M and L cones so close?
• Why are there 3?
© Stephen E. Palmer, 2002

91.

Impossible Colors
Can you make the cones respond in ways that typical
light spectra never would?
http://en.wikipedia.org/wiki/Impossible_colors

92.

Tetrachromatism
Bird cone
responses
• Most birds, and many other animals, have
cones for ultraviolet light.
• Some humans, mostly female, seem to
have slight tetrachromatism.

93.

More Spectra
metamers

94.

Practical Color Sensing: Bayer Grid
• Estimate RGB
at ‘G’ cells
from
neighboring
values
Slide by Steve Seitz

95.

Color Image
R
G
B

96. Impossible Colors

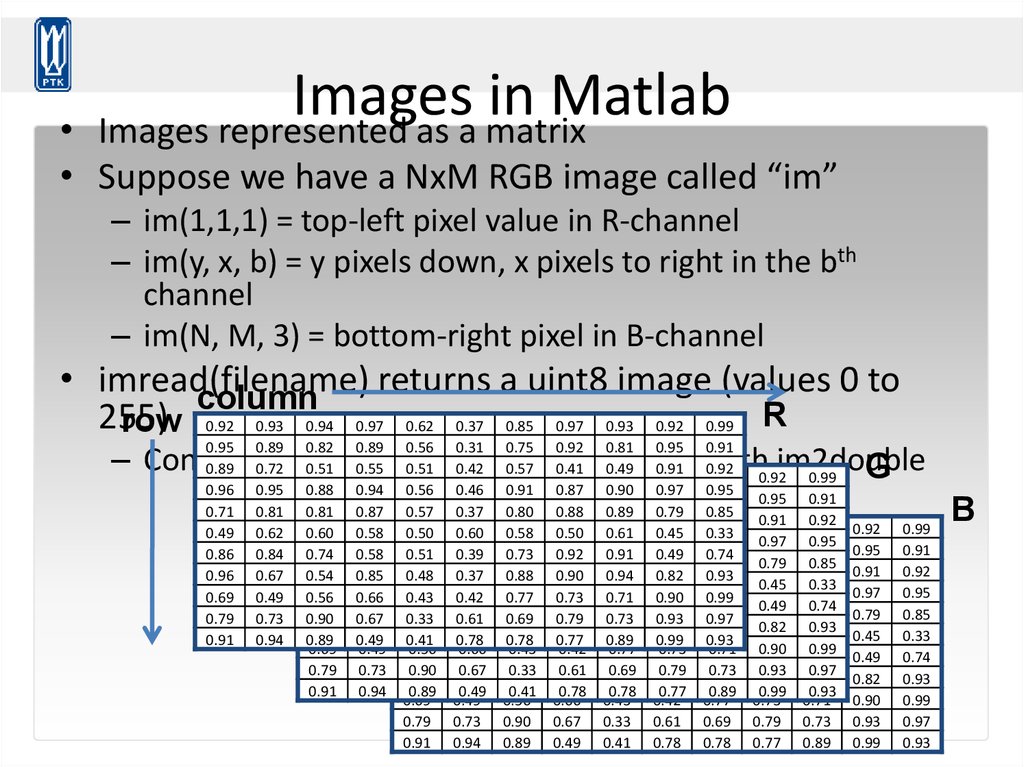
Images
in
Matlab
Images represented as a matrix
• Suppose we have a NxM RGB image called “im”
– im(1,1,1) = top-left pixel value in R-channel
– im(y, x, b) = y pixels down, x pixels to right in the bth
channel
– im(N, M, 3) = bottom-right pixel in B-channel
• imread(filename)
returns
a
uint8
image
(values
0
to
column
255)
row 0.92 0.93 0.94 0.97 0.62 0.37 0.85 0.97 0.93 0.92 0.99 R
– Convert
to double
0 to0.911) 0.92
with im2double
0.89 0.72
0.51 0.55 format
0.51 0.42 (values
0.57 0.41 0.49
G
0.92 0.93 0.94 0.97 0.62 0.37 0.85 0.97 0.93 0.92 0.99
0.95
0.89
0.82
0.89
0.56
0.31
0.75
0.92
0.81
0.95
0.91
0.96
0.71
0.49
0.86
0.96
0.69
0.79
0.91
0.95
0.81
0.62
0.84
0.67
0.49
0.73
0.94
0.88
0.95
0.81
0.89
0.60
0.96
0.74
0.71
0.54
0.49
0.56
0.86
0.90
0.96
0.89
0.69
0.79
0.91
0.94
0.89
0.87
0.72
0.58
0.95
0.58
0.81
0.85
0.62
0.66
0.84
0.67
0.67
0.49
0.49
0.73
0.94
0.56
0.82
0.57
0.51
0.92
0.50
0.88
0.95
0.51
0.81
0.89
0.48
0.60
0.96
0.43
0.74
0.71
0.33
0.54
0.49
0.41
0.56
0.86
0.90
0.96
0.89
0.69
0.79
0.91
0.46
0.89
0.37
0.55
0.93
0.60
0.94
0.89
0.39
0.87
0.72
0.37
0.58
0.95
0.42
0.58
0.81
0.61
0.85
0.62
0.78
0.66
0.84
0.67
0.67
0.49
0.49
0.73
0.94
0.91
0.56
0.80
0.51
0.94
0.58
0.56
0.82
0.73
0.57
0.51
0.88
0.50
0.88
0.77
0.51
0.81
0.69
0.48
0.60
0.78
0.43
0.74
0.33
0.54
0.41
0.56
0.90
0.89
0.87
0.31
0.88
0.42
0.97
0.50
0.46
0.89
0.92
0.37
0.55
0.90
0.60
0.94
0.73
0.39
0.87
0.79
0.37
0.58
0.77
0.42
0.58
0.61
0.85
0.78
0.66
0.67
0.49
0.90
0.75
0.89
0.57
0.62
0.61
0.91
0.56
0.91
0.80
0.51
0.94
0.58
0.56
0.71
0.73
0.57
0.73
0.88
0.50
0.89
0.77
0.51
0.69
0.48
0.78
0.43
0.33
0.41
0.97
0.92
0.79
0.41
0.37
0.45
0.87
0.31
0.49
0.88
0.42
0.82
0.50
0.46
0.90
0.92
0.37
0.93
0.90
0.60
0.99
0.73
0.39
0.79
0.37
0.77
0.42
0.61
0.78
0.95
0.81
0.85
0.49
0.85
0.33
0.90
0.75
0.74
0.89
0.57
0.93
0.61
0.91
0.99
0.91
0.80
0.97
0.94
0.58
0.93
0.71
0.73
0.73
0.88
0.89
0.77
0.69
0.78
0.95
0.91
0.97
0.97
0.92
0.79
0.41
0.45
0.87
0.49
0.88
0.82
0.50
0.90
0.92
0.93
0.90
0.99
0.73
0.79
0.77
0.91
0.92
0.93
0.95
0.81
0.85
0.49
0.33
0.90
0.74
0.89
0.93
0.61
0.99
0.91
0.97
0.94
0.93
0.71
0.73
0.89
0.92
0.95
0.91
0.97
0.79
0.45
0.49
0.82
0.90
0.93
0.99
0.99
0.91
0.92
0.95
0.85
0.33
0.74
0.93
0.99
0.97
0.93
B

97. Tetrachromatism

Color spaces
• How can we represent color?
http://en.wikipedia.org/wiki/File:RGB_illumination.jpg

98. More Spectra

Color spaces: RGB
Default color space
0,1,0
R
(G=0,B=0)
G
1,0,0
(R=0,B=0)
0,0,1
Some drawbacks
B
(R=0,G=0)
• Strongly correlated channels
• Non-perceptual
Image from: http://en.wikipedia.org/wiki/File:RGB_color_solid_cube.png

99. Color Sensing in Camera (RGB)

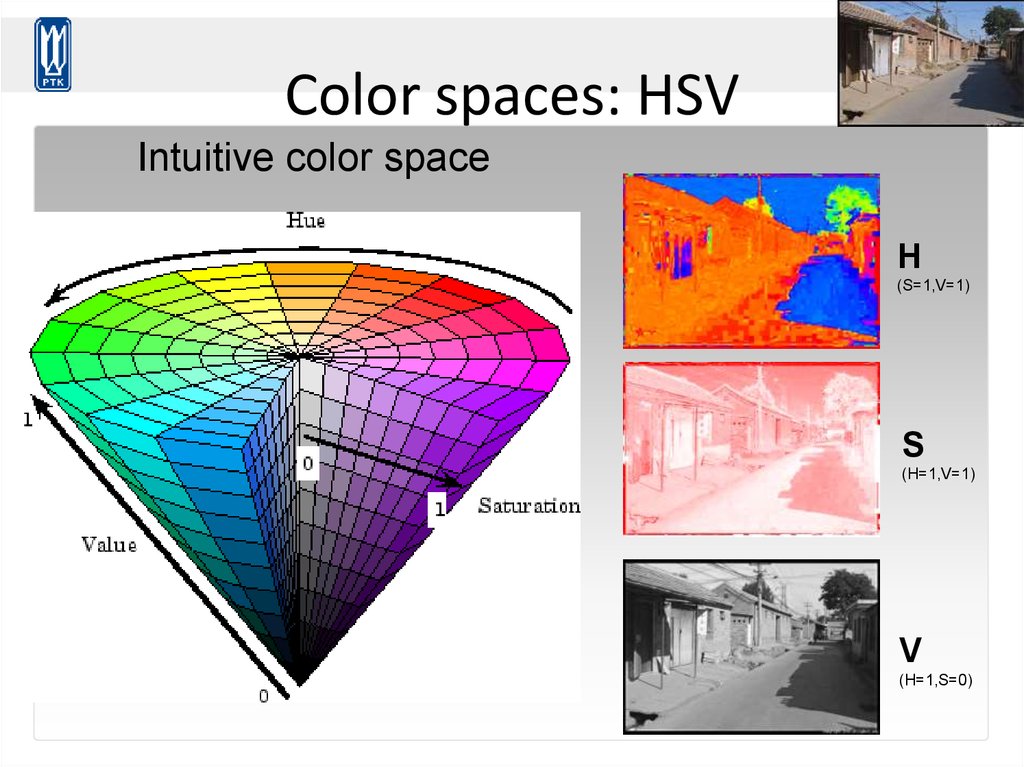
Color spaces: HSV
Intuitive color space
H
(S=1,V=1)
S
(H=1,V=1)
V
(H=1,S=0)

100. Practical Color Sensing: Bayer Grid

Color spaces: YCbCr
Fast to compute, good for
compression, used by TV
Y=0
Y=0.5
Y
(Cb=0.5,Cr=0.5)
Cr
Cb
Cb
(Y=0.5,Cr=0.5)
Y=1
Cr
(Y=0.5,Cb=05)

101. Color Image

Color spaces: L*a*b*
“Perceptually uniform”* color space
L
(a=0,b=0)
a
(L=65,b=0)
b
(L=65,a=0)

102. Images in Matlab

If you had to choose, would you rather
go without luminance or
chrominance?

103. Color spaces

If you had to choose, would you rather
go without luminance or
chrominance?

104. Color spaces: RGB

Most information in intensity
Only color shown – constant intensity

105. Color spaces: HSV

Most information in intensity
Only intensity shown – constant color

106. Color spaces: YCbCr

Most information in intensity
Original image

107. Color spaces: L*a*b*

Back to grayscale intensity
0.92
0.95
0.89
0.96
0.71
0.49
0.86
0.96
0.69
0.79
0.91
0.93
0.89
0.72
0.95
0.81
0.62
0.84
0.67
0.49
0.73
0.94
0.94
0.82
0.51
0.88
0.81
0.60
0.74
0.54
0.56
0.90
0.89
0.97
0.89
0.55
0.94
0.87
0.58
0.58
0.85
0.66
0.67
0.49
0.62
0.56
0.51
0.56
0.57
0.50
0.51
0.48
0.43
0.33
0.41
0.37
0.31
0.42
0.46
0.37
0.60
0.39
0.37
0.42
0.61
0.78
0.85
0.75
0.57
0.91
0.80
0.58
0.73
0.88
0.77
0.69
0.78
0.97
0.92
0.41
0.87
0.88
0.50
0.92
0.90
0.73
0.79
0.77
0.93
0.81
0.49
0.90
0.89
0.61
0.91
0.94
0.71
0.73
0.89
0.92
0.95
0.91
0.97
0.79
0.45
0.49
0.82
0.90
0.93
0.99
0.99
0.91
0.92
0.95
0.85
0.33
0.74
0.93
0.99
0.97
0.93

108. If you had to choose, would you rather go without luminance or chrominance?

Дальше
Восприятие света и цвета
Камера и человеческий глаз
Сенсоры и пиксели
Фильтрация изображений
Практическое задание: линейные фильтры

109. If you had to choose, would you rather go without luminance or chrominance?

ОС
СУ
УД
ДА
АР
РС
СТ
ТВ
ВЕ
ЕН
НН
НЫ
ЫЙ
Й
ГГ О
НА
АУ
УЧ
ЧН
НЫ
ЫЙ
Й
Н
ЦЕ
ЕН
Н ТТ Р
Р
Ц
РО
ОС
СС
СИ
ИИ
И
Р
ЦЕНТРАЛЬНЫЙ НАУЧНО-ИССЛЕДОВАТЕЛЬСКИЙ И ОПЫТНО-КОНСТРУКТОРСКИЙ
ИНСТИТУТ РОБОТОТЕХНИКИ И ТЕХНИЧЕСКОЙ КИБЕРНЕТИКИ
Спасибо за внимание!
Россия, 194064, г. Санкт-Петербург, Тихорецкий пр., 21
тел.: (812) 552-0110 (812) 552-1325 факс: (812) 556-3692 http://www.rtc.ru e-mail: [email protected]