Похожие презентации:
Magnetism
1. Prelude to an Exam Allegro con brio
Next Friday – EXAMINATION #2Watch those WebAssigns .. no more
extensions.
Monday will be
• A Quiz on Circuits
• A review of circuits and some other problems.
Wednesday, more on Magnetism. Only day 1
on the exm. Watch for a new Webassign.
Magnetism
1
2. Magnetism
A Whole New TopicMagnetism
2
3. DEMO
Magnetism3
4. Lodestone (Mineral)
• Lodestones attractediron filings.
• Lodestones seemed to
attract each other.
• Used as a compass.
– One end always
pointed north.
• Lodestone is a natural
magnet.
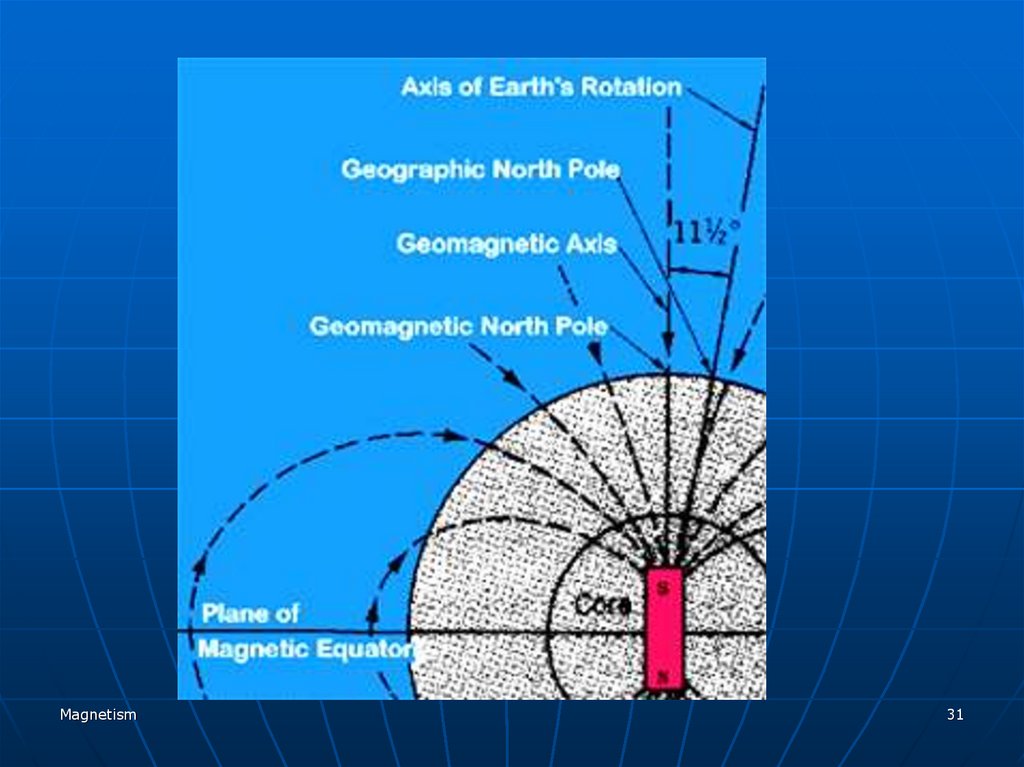
Magnetism
4
5. Magnetism
• Refrigerators are attracted to magnets!Magnetism
5
6. Applications
• Motors• Navigation – Compass
• Magnetic Tapes
– Music, Data
• Television
– Beam deflection Coil
• Magnetic Resonance Imaging
• High Energy Physics Research
Magnetism
6
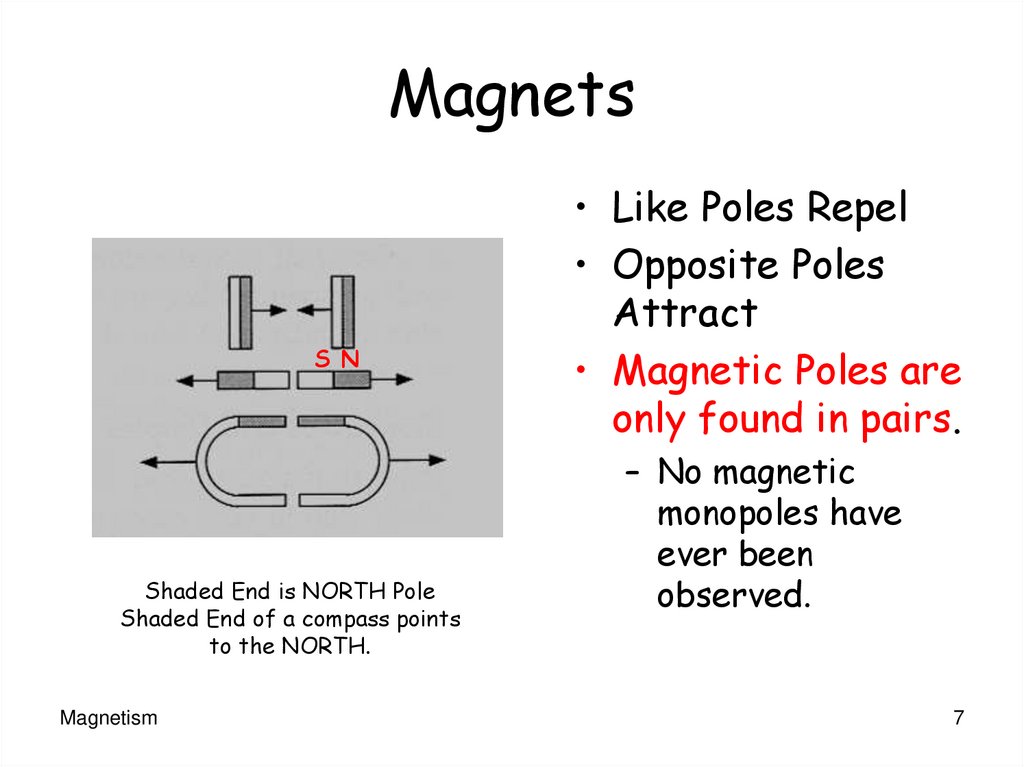
7. Magnets
S NShaded End is NORTH Pole
Shaded End of a compass points
to the NORTH.
Magnetism
• Like Poles Repel
• Opposite Poles
Attract
• Magnetic Poles are
only found in pairs.
– No magnetic
monopoles have
ever been
observed.
7
8. Observations
+Observations
+
+
• Bring a magnet to a charged electroscope and
nothing happens. No forces.
• Bring a magnet near some metals (Co, Fe, Ni
…) and it will be attracted to the magnet.
– The metal will be attracted to both the N and S
poles independently.
– Some metals are not attracted at all.
– Wood is NOT attracted to a magnet.
– Neither is water.
• A magnet will force a compass needle to align
with it. (No big Surprise.)
Magnetism
8
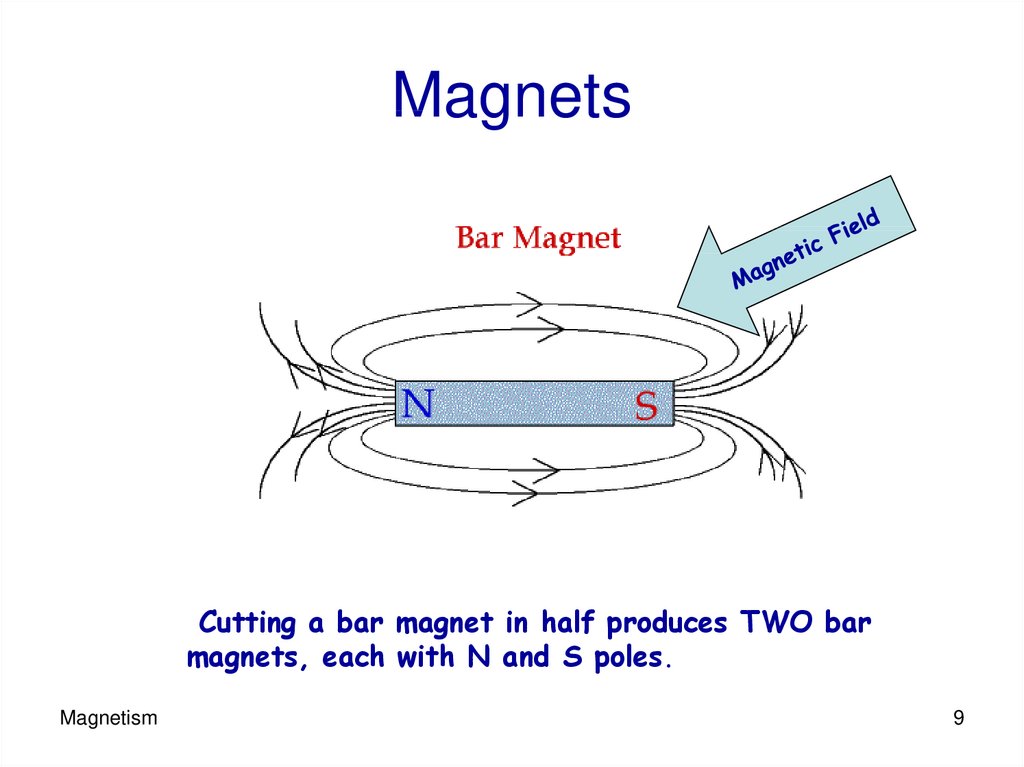
9. Magnets
Cutting a bar magnet in half produces TWO barmagnets, each with N and S poles.
Magnetism
9
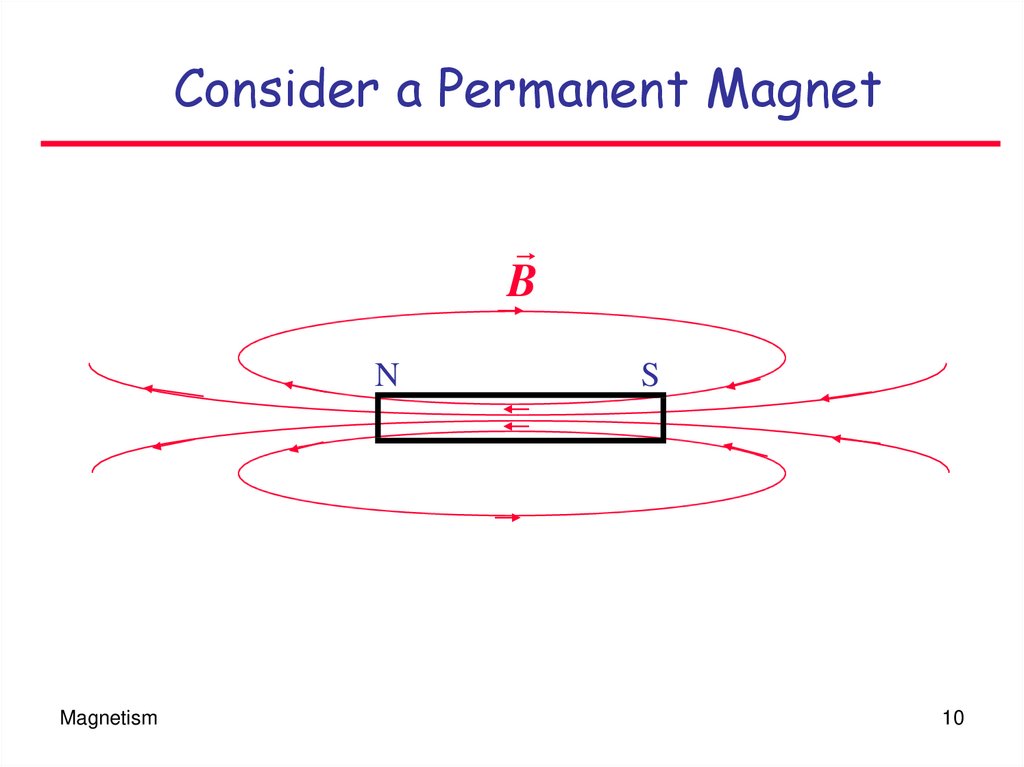
10. Consider a Permanent Magnet
BN
Magnetism
S
10
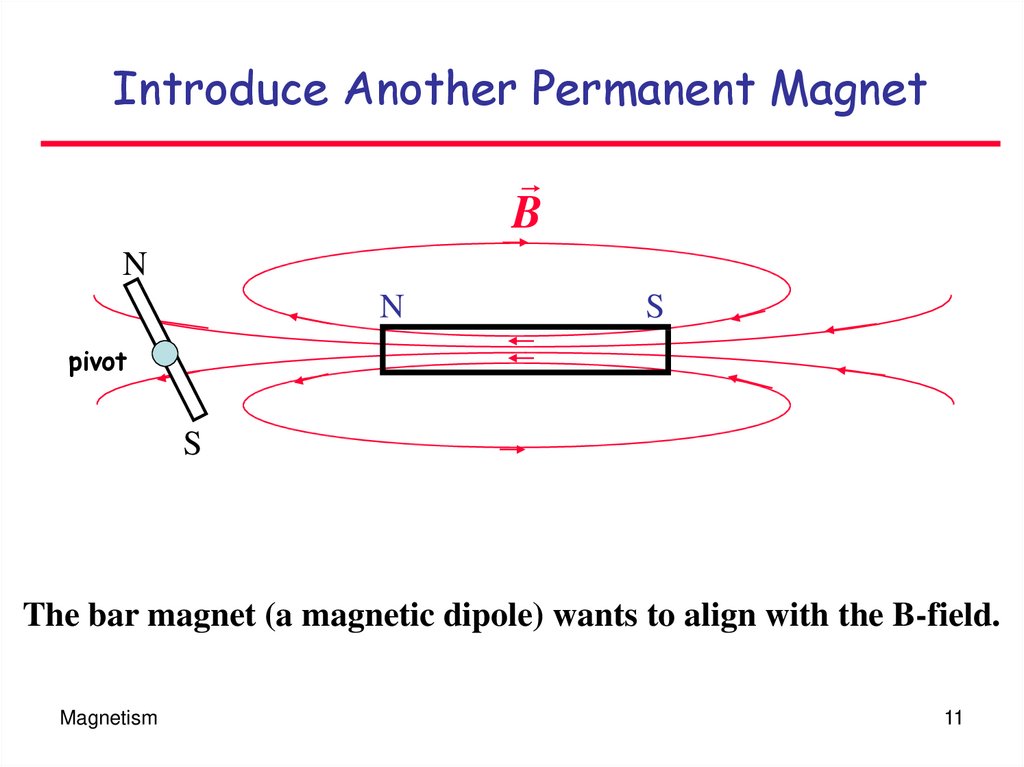
11. Introduce Another Permanent Magnet
BN
N
S
pivot
S
The bar magnet (a magnetic dipole) wants to align with the B-field.
Magnetism
11
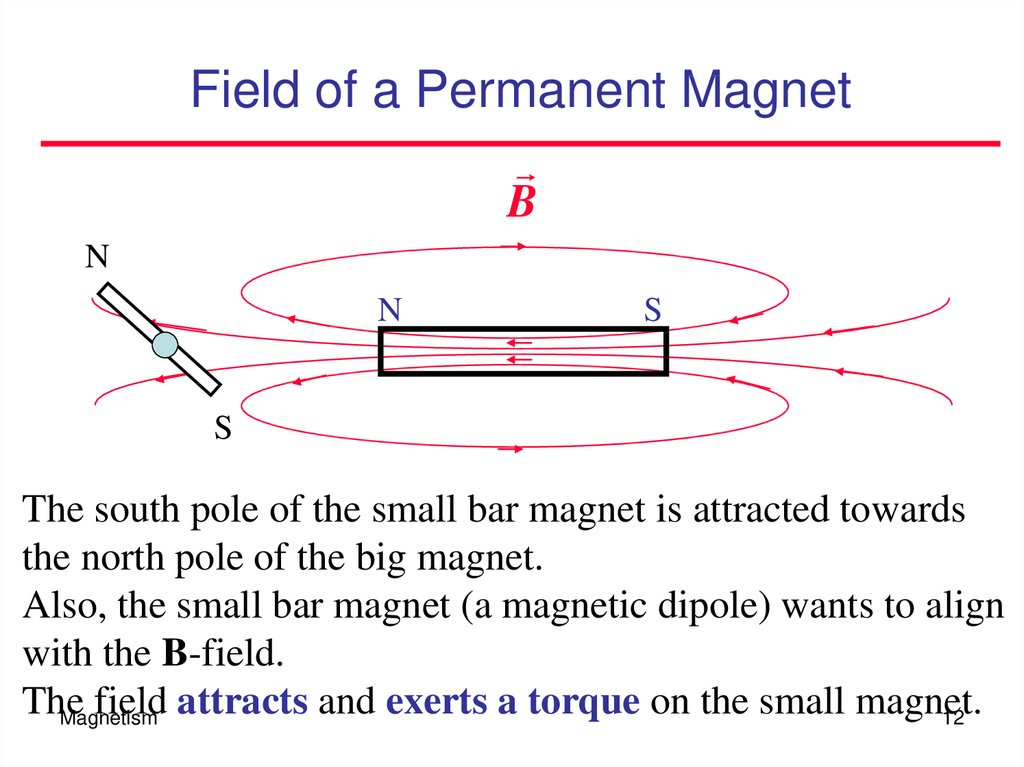
12. Field of a Permanent Magnet
BN
N
S
S
The south pole of the small bar magnet is attracted towards
the north pole of the big magnet.
Also, the small bar magnet (a magnetic dipole) wants to align
with the B-field.
The
field attracts and exerts a torque on the small magnet.
Magnetism
12
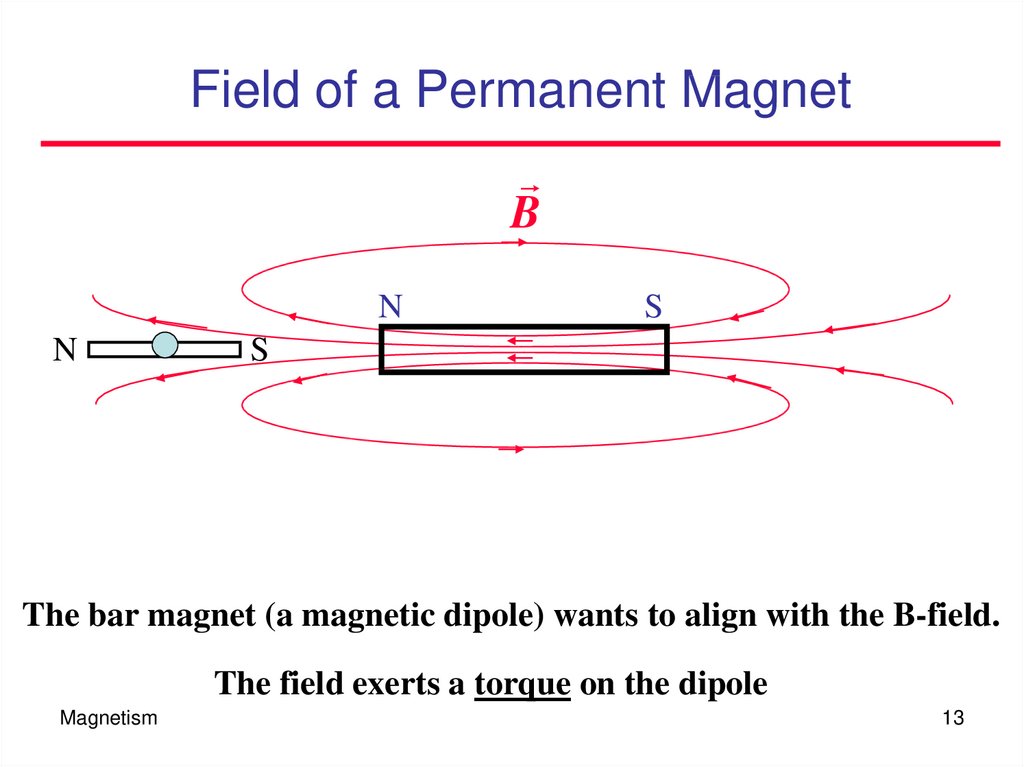
13. Field of a Permanent Magnet
BN
N
S
S
The bar magnet (a magnetic dipole) wants to align with the B-field.
The field exerts a torque on the dipole
Magnetism
13
14. The Magnetic Field
• Similar to Electric Field … exists inspace.
– Has Magnitude AND Direction.
• The “stronger” this field, the greater is
the ability of the field to interact with
a magnet.
Magnetism
14
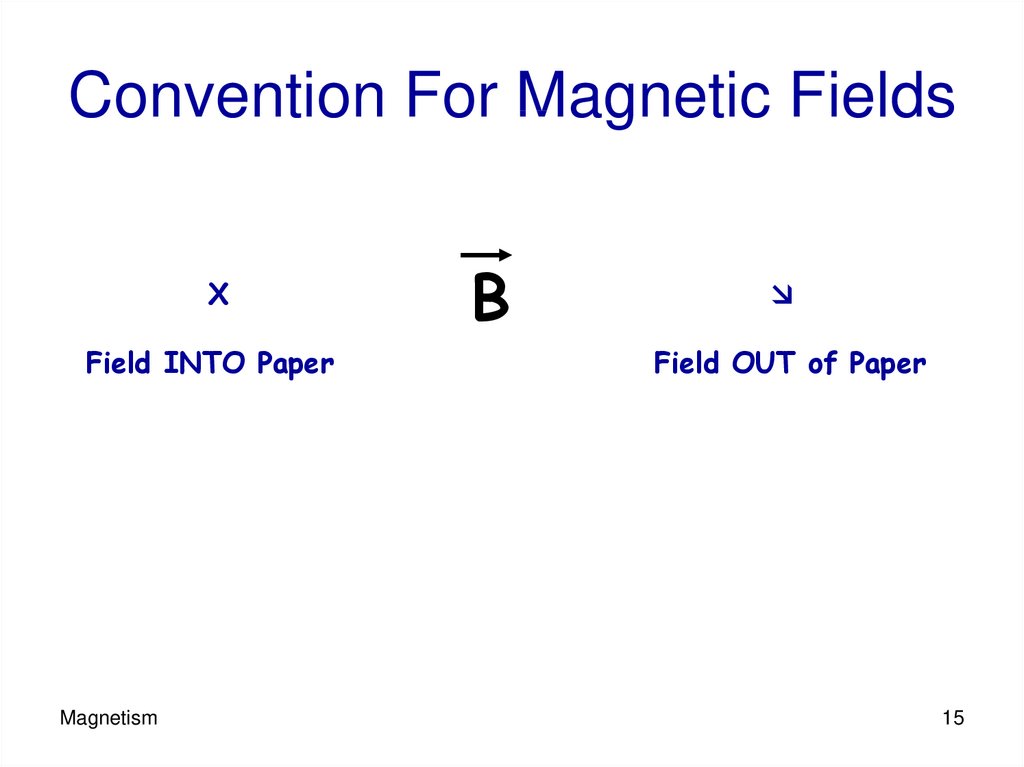
15. Convention For Magnetic Fields
XField INTO Paper
Magnetism
B
Field OUT of Paper
15
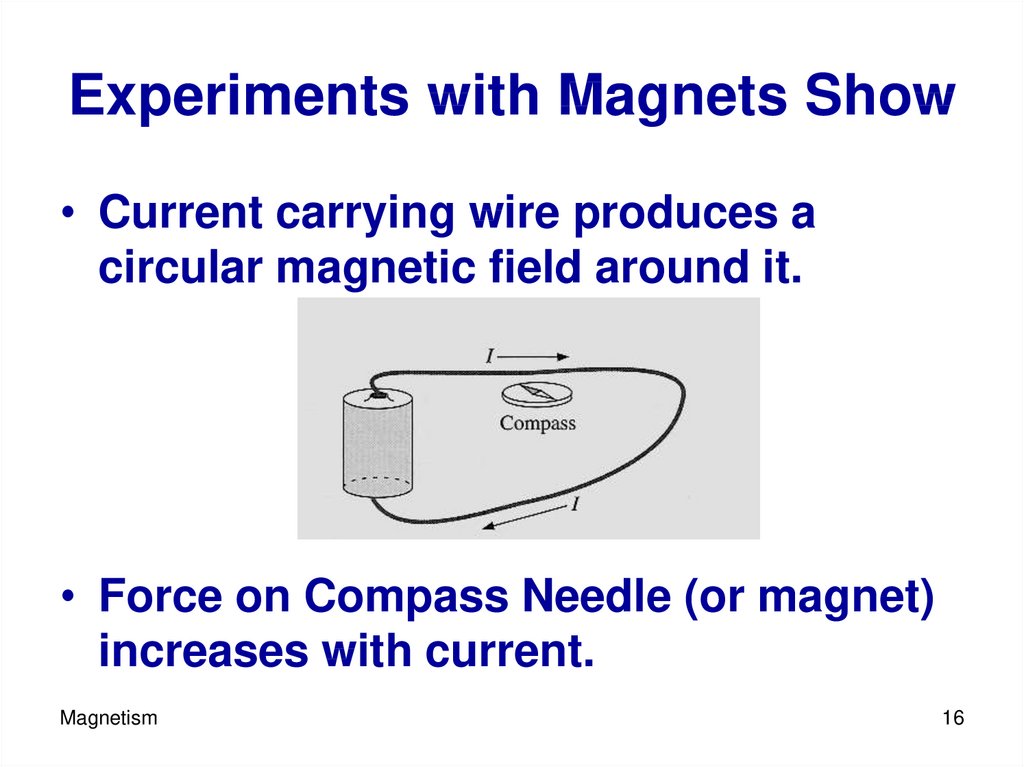
16. Experiments with Magnets Show
• Current carrying wire produces acircular magnetic field around it.
• Force on Compass Needle (or magnet)
increases with current.
Magnetism
16
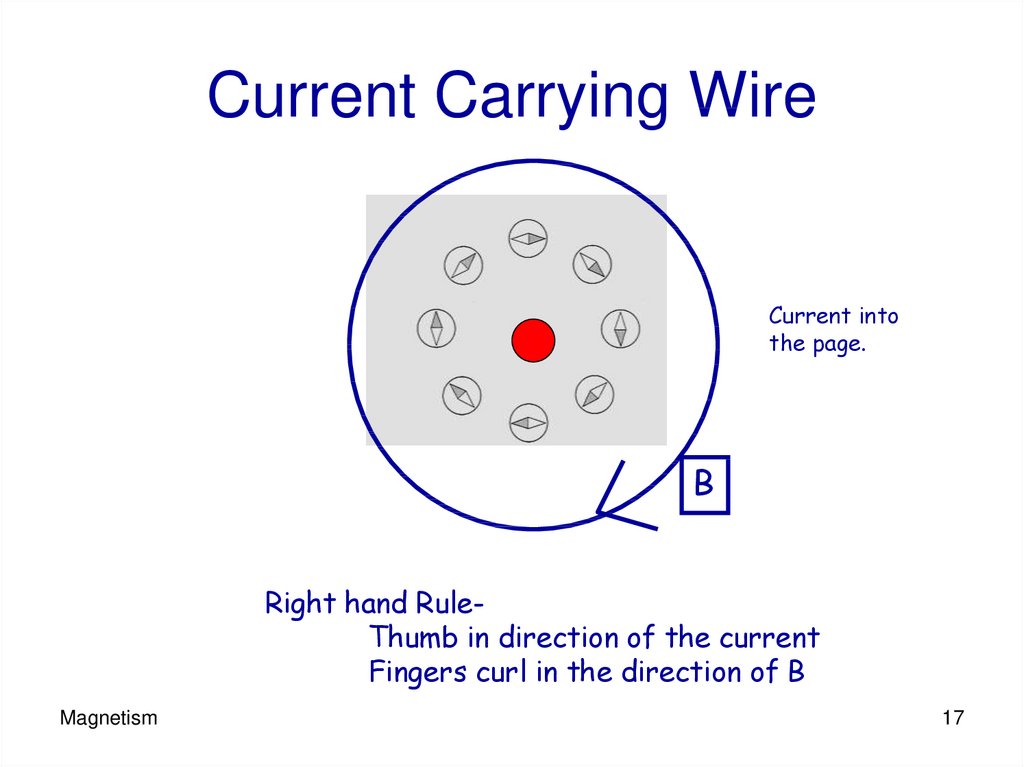
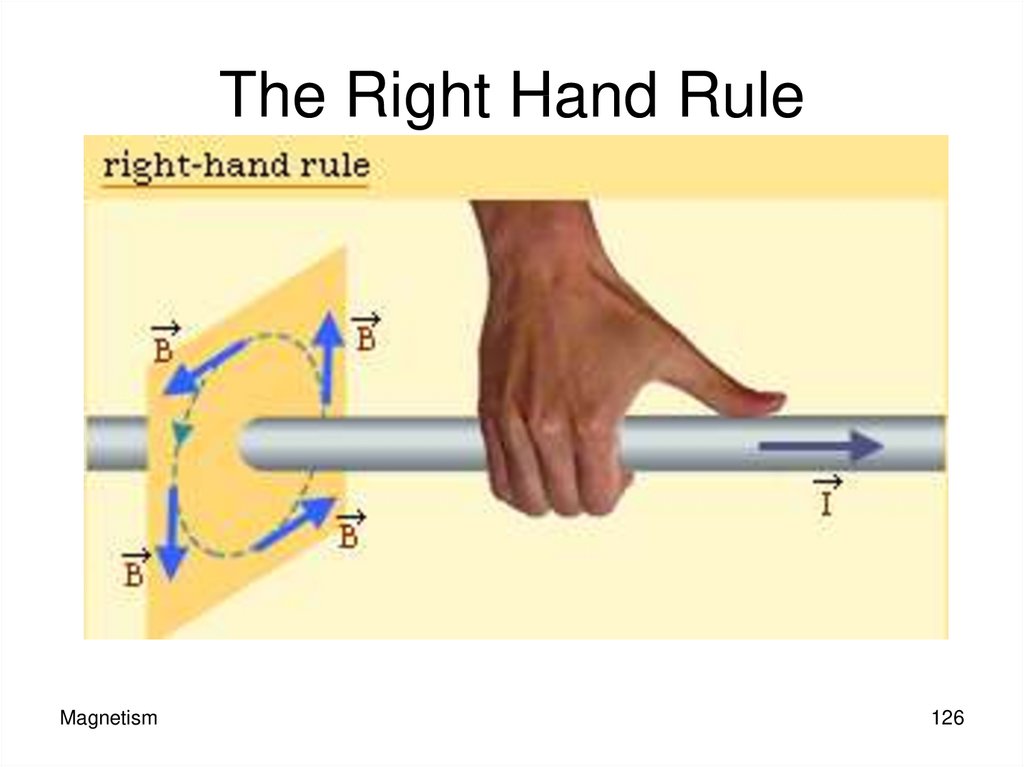
17. Current Carrying Wire
Current intothe page.
B
Right hand RuleThumb in direction of the current
Fingers curl in the direction of B
Magnetism
17
18. Current Carrying Wire
• B field is created at ALL POINTS in spacesurrounding the wire.
• The B field had magnitude and direction.
• Force on a magnet increases with the
current.
• Force is found to vary as ~(1/d) from the
wire.
Magnetism
18
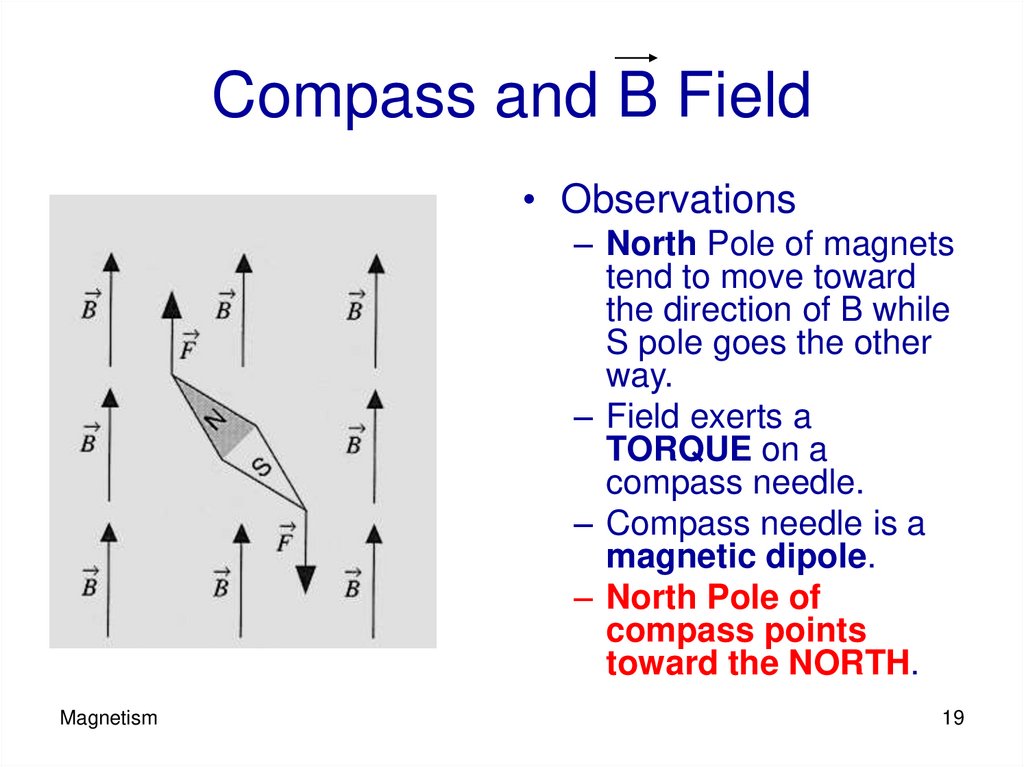
19. Compass and B Field
• Observations– North Pole of magnets
tend to move toward
the direction of B while
S pole goes the other
way.
– Field exerts a
TORQUE on a
compass needle.
– Compass needle is a
magnetic dipole.
– North Pole of
compass points
toward the NORTH.
Magnetism
19
20. Planet Earth
Magnetism20
21. Inside it all.
8000Miles
Magnetism
21
22. On the surface it looks like this..
Magnetism22
23. Inside: Warmer than Floriduh
Magnetism23
24. Much Warmer than Floriduh
Magnetism24
25. Finally
Magnetism25
26. In Between
The molten iron core exists in a magneticfield that had been created from other
sources (sun…).
The fluid is rotating in this field.
This motion causes a current in the molten
metal.
The current causes a magnetic field.
The process is self-sustaining.
The driving force is the heat (energy) that
is generated in the core of the planet.
Magnetism
26
27.
After molten lava emerges from a volcano, it solidifies to arock. In most cases it is a black rock known as basalt, which is
faintly magnetic, like iron emerging from a melt. Its
magnetization is in the direction of the local magnetic force
at the time when it cools down.
Instruments can measure the magnetization of basalt.
Therefore, if a volcano has produced many lava flows over a
past period, scientists can analyze the magnetizations of the
various flows and from them get an idea on how the direction
of the local Earth's field varied in the past. Surprisingly, this
procedure suggested that times existed when the
magnetization had the opposite direction from today's. All
sorts of explanation were proposed, but in the end the only
one which passed all tests was that in the distant past,
indeed, the magnetic polarity of the Earth was sometimes
reversed.
Magnetism
27
28. Ancient Navigation
Magnetism28
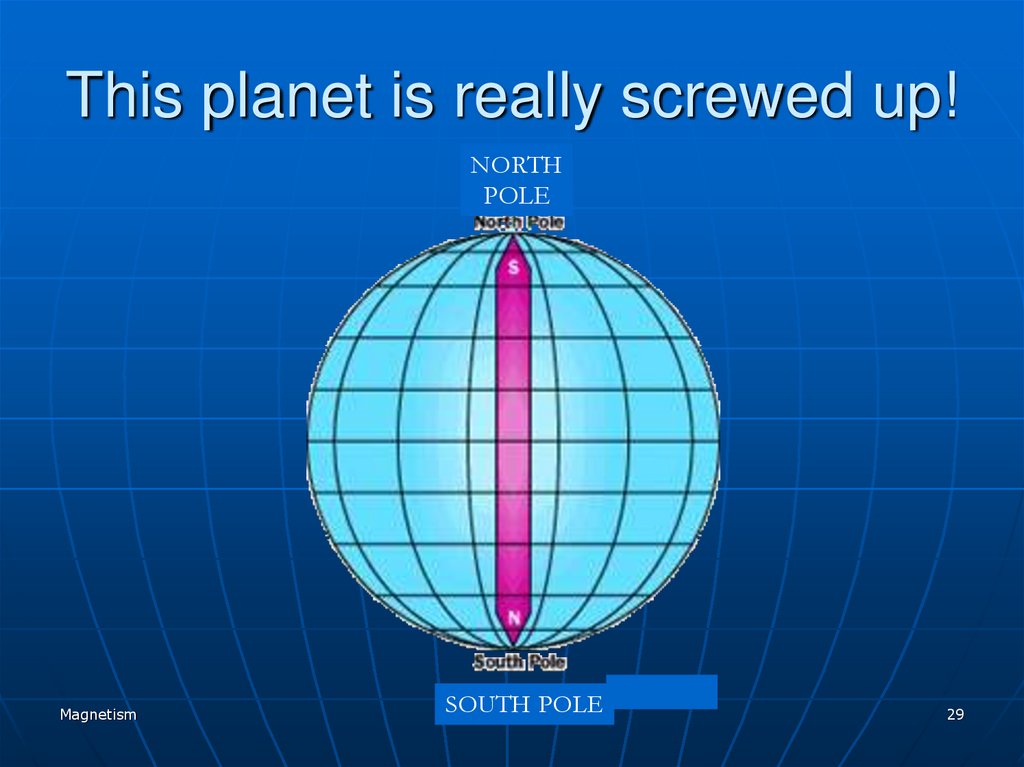
29. This planet is really screwed up!
NORTHPOLE
Magnetism
SOUTH POLE
29
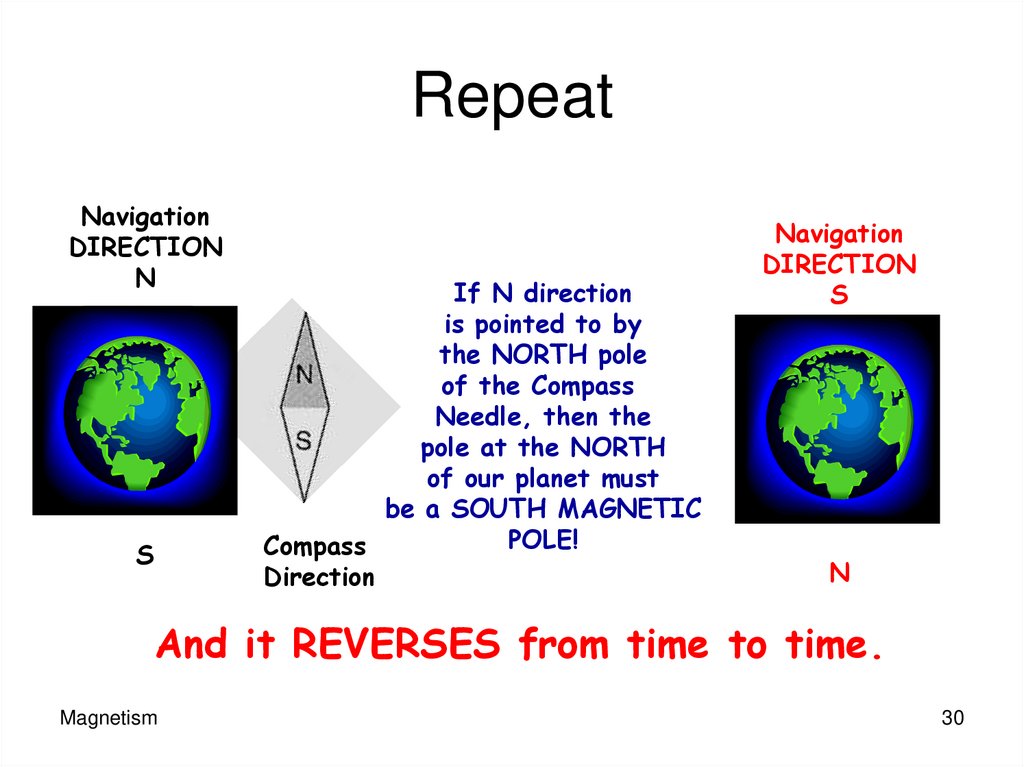
30. Repeat
NavigationDIRECTION
N
S
If N direction
is pointed to by
the NORTH pole
of the Compass
Needle, then the
pole at the NORTH
of our planet must
be a SOUTH MAGNETIC
POLE!
Compass
Direction
Navigation
DIRECTION
S
N
And it REVERSES from time to time.
Magnetism
30
31.
Magnetism31
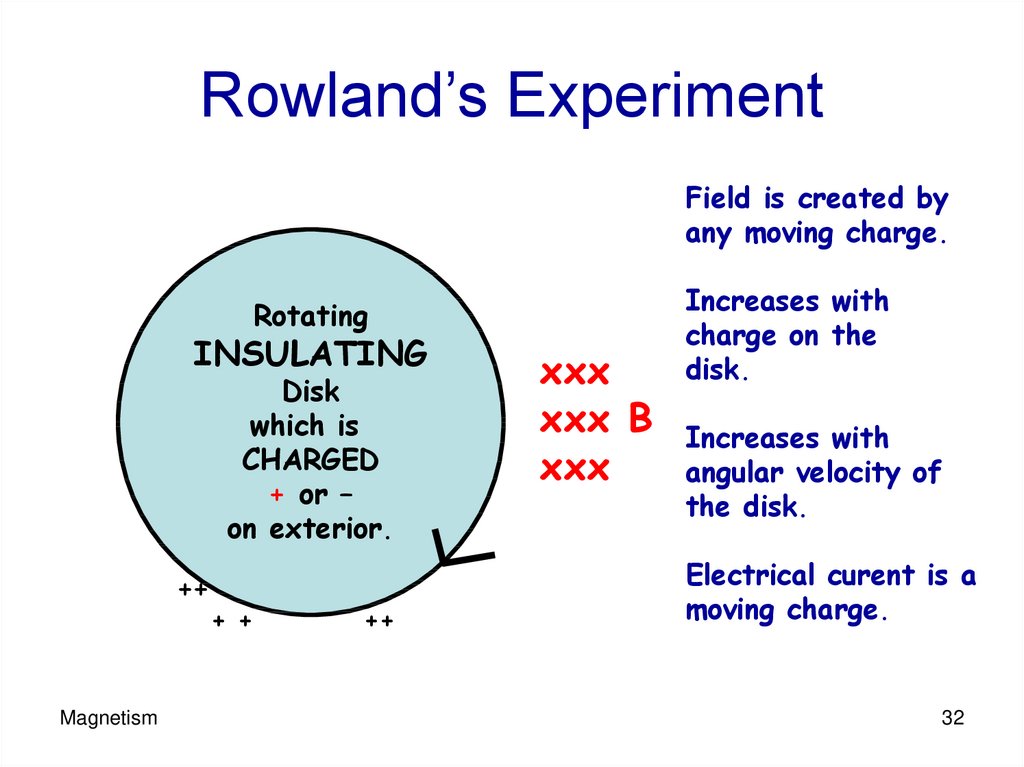
32. Rowland’s Experiment
Field is created byany moving charge.
Rotating
INSULATING
Disk
which is
CHARGED
+ or –
on exterior.
++
Magnetism
+ +
++
xxx
xxx B
xxx
Increases with
charge on the
disk.
Increases with
angular velocity of
the disk.
Electrical curent is a
moving charge.
32
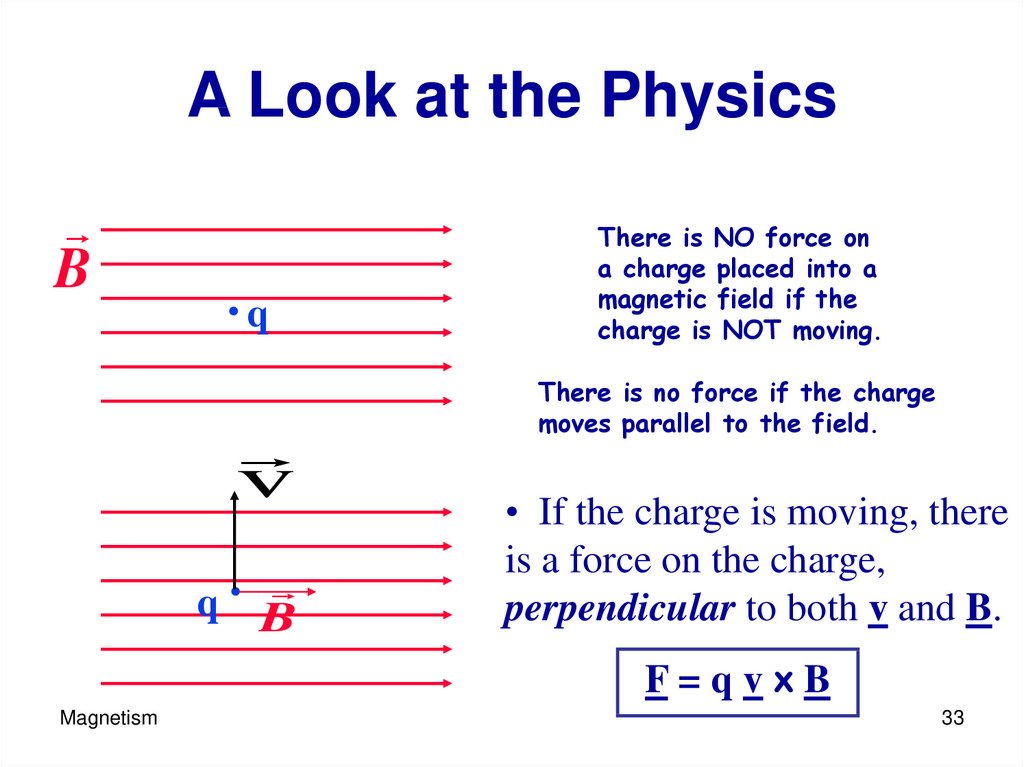
33. A Look at the Physics
Bq
v
q B
There is NO force on
a charge placed into a
magnetic field if the
charge is NOT moving.
There is no force if the charge
moves parallel to the field.
• If the charge is moving, there
is a force on the charge,
perpendicular to both v and B.
F=qvxB
Magnetism
33
34. WHAT THE HECK IS THAT???
• A WHAT PRODUCT?• A CROSS PRODUCT – Like an
angry one??
• Alas, yes ….
• F=qv X B
Magnetism
34
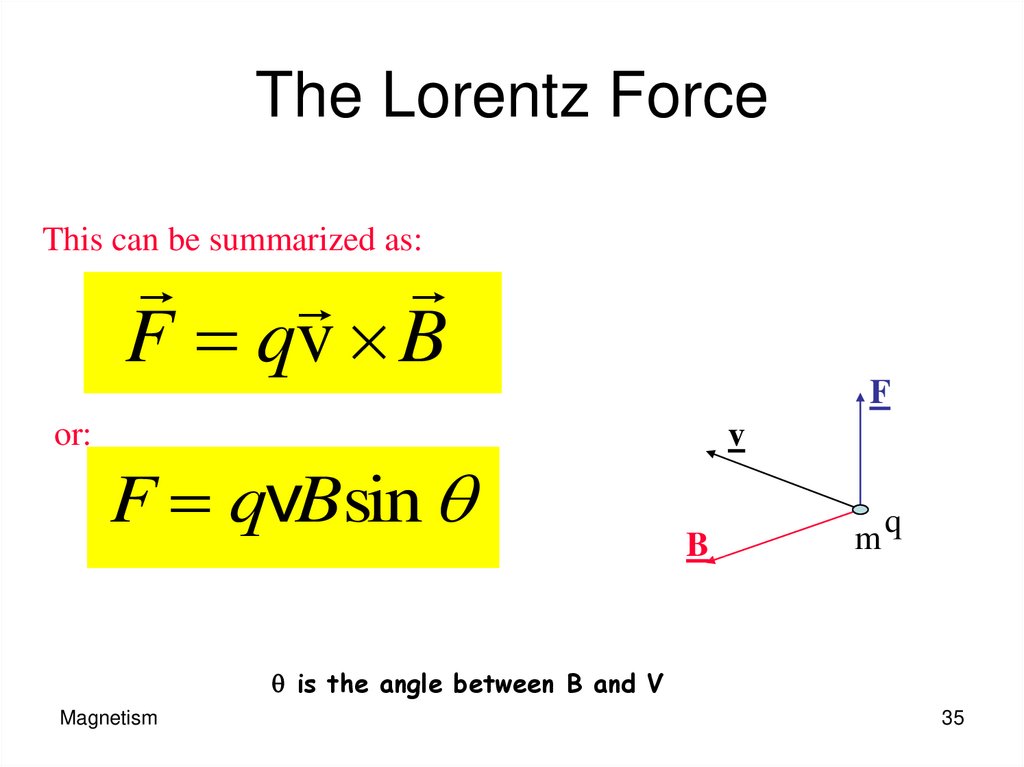
35. The Lorentz Force
This can be summarized as:F qv B
F
or:
F qvBsin
v
B
mq
is the angle between B and V
Magnetism
35
36. Note
B is sort of the Force per unit(charge-velocity)
Whatever that is!!
Magnetism
36
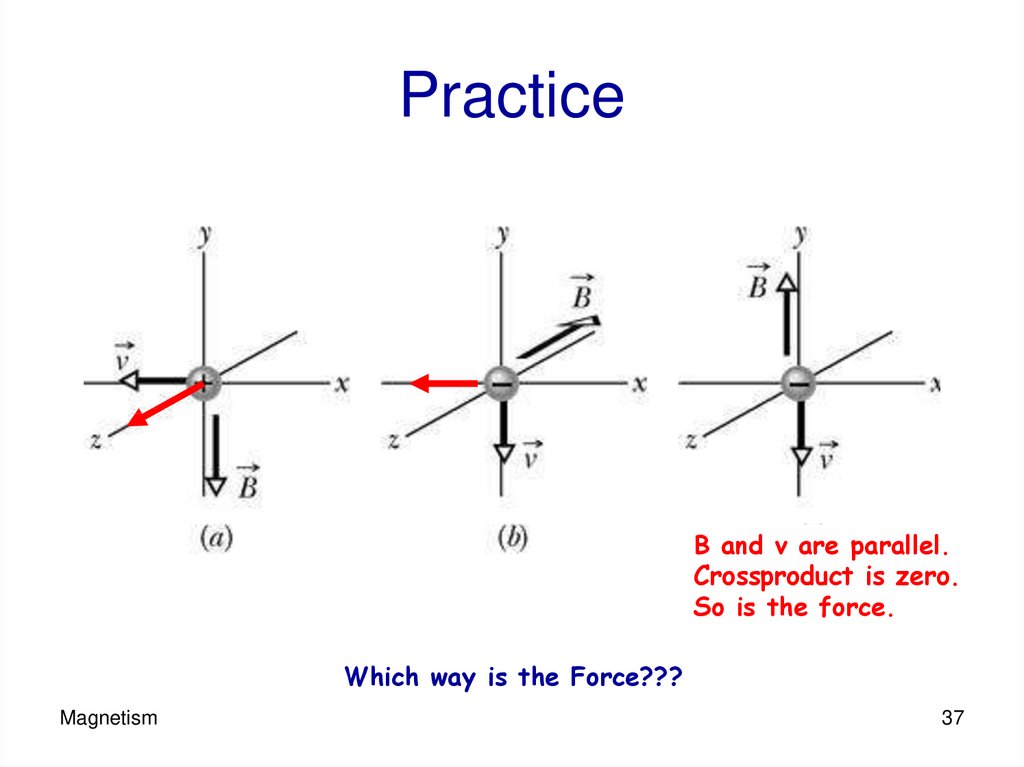
37. Practice
B and v are parallel.Crossproduct is zero.
So is the force.
Which way is the Force???
Magnetism
37
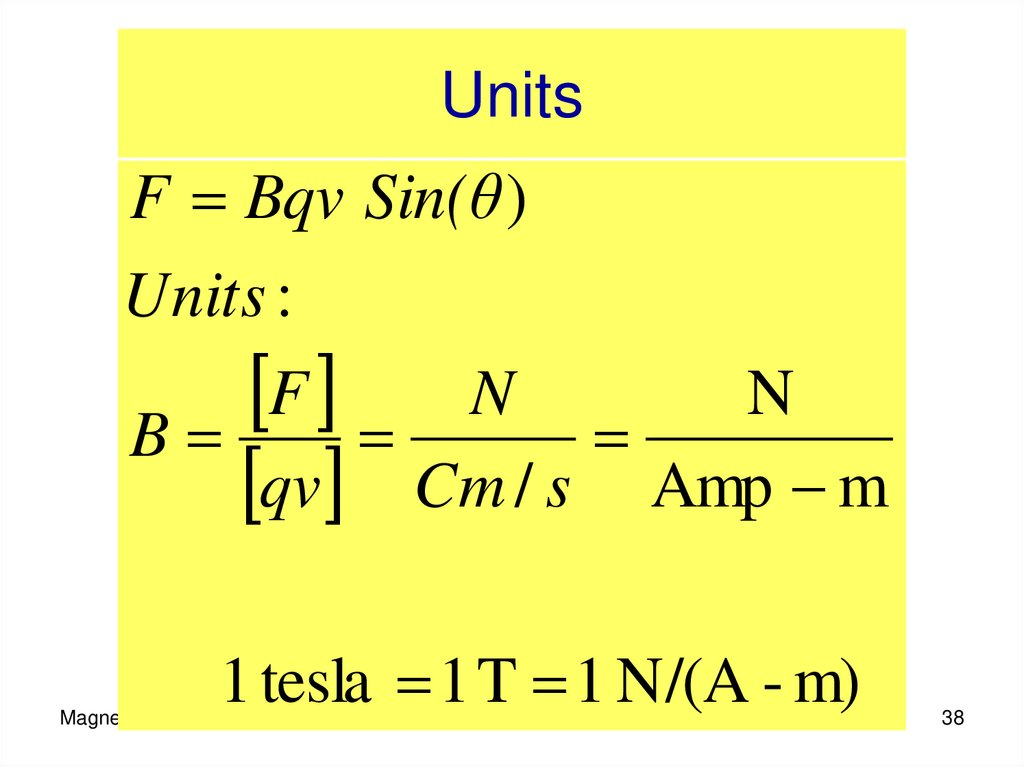
38. Units
F Bqv Sin(θ )Units :
F
N
N
B
qv Cm / s Amp m
Magnetism
1 tesla 1 T 1 N/(A - m)
38
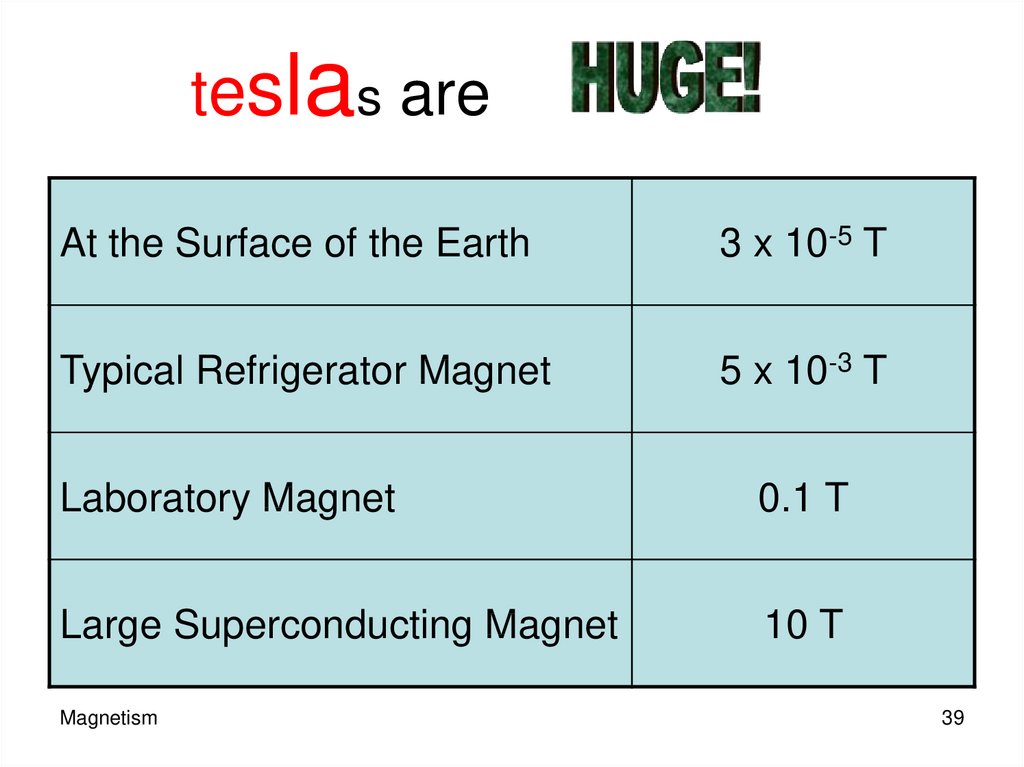
39. teslas are
At the Surface of the Earth3 x 10-5 T
Typical Refrigerator Magnet
5 x 10-3 T
Laboratory Magnet
0.1 T
Large Superconducting Magnet
10 T
Magnetism
39
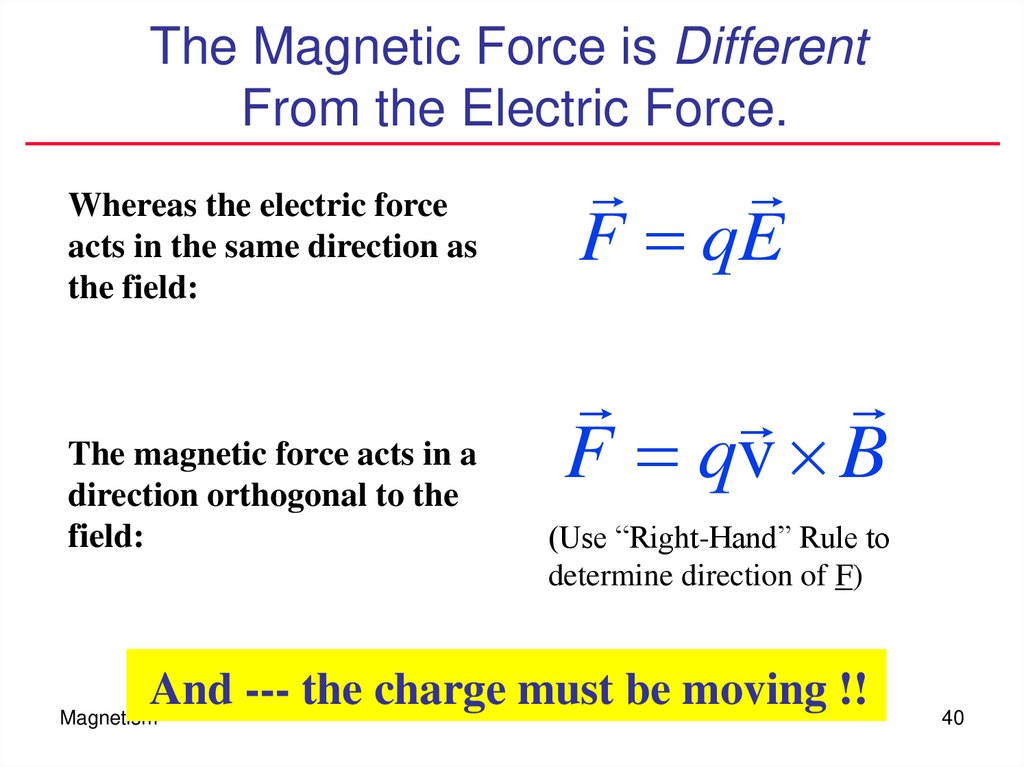
40. The Magnetic Force is Different From the Electric Force.
Whereas the electric forceacts in the same direction as
the field:
The magnetic force acts in a
direction orthogonal to the
field:
F qE
F qv B
(Use “Right-Hand” Rule to
determine direction of F)
And
--the
charge
must
be
moving
!!
Magnetism
40
41. So…
A moving charge can create a magneticfield.
A moving charge is acted upon by a
magnetic field.
In Magnetism, things move.
In the Electric Field, forces and the
field can be created by stationary
charges.
Magnetism
41
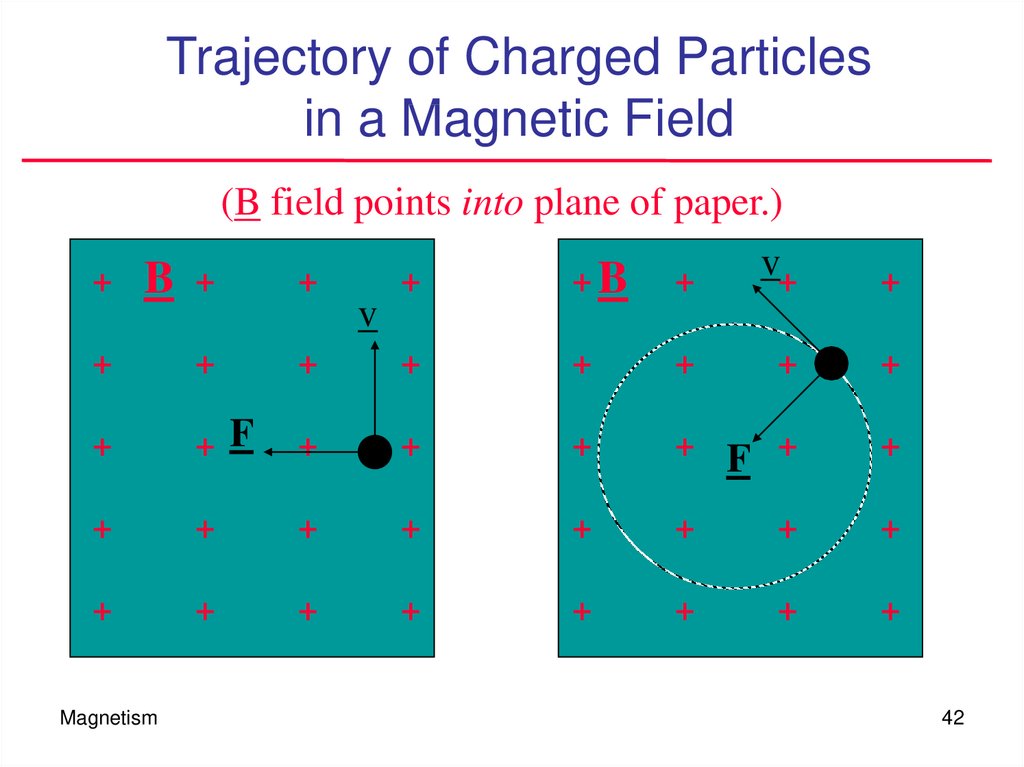
42. Trajectory of Charged Particles in a Magnetic Field
(B field points into plane of paper.)+
+B
+
v+
+
+
+
+
+
+
+
+ F
+
+
+
+ F +
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
B
+
+
+
+
+
Magnetism
v
42
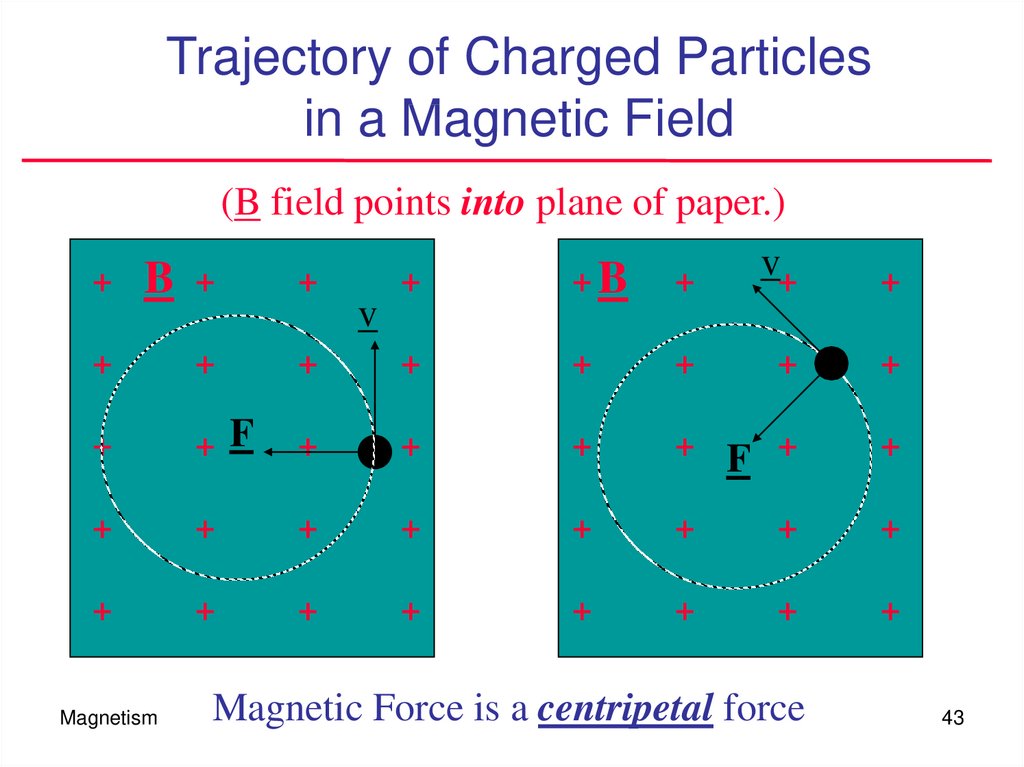
43. Trajectory of Charged Particles in a Magnetic Field
(B field points into plane of paper.)+
+B
+
v+
+
+
+
+
+
+
+
+ F
+
+
+
+ F +
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
+
B
+
+
+
+
+
Magnetism
v
Magnetic Force is a centripetal force
43
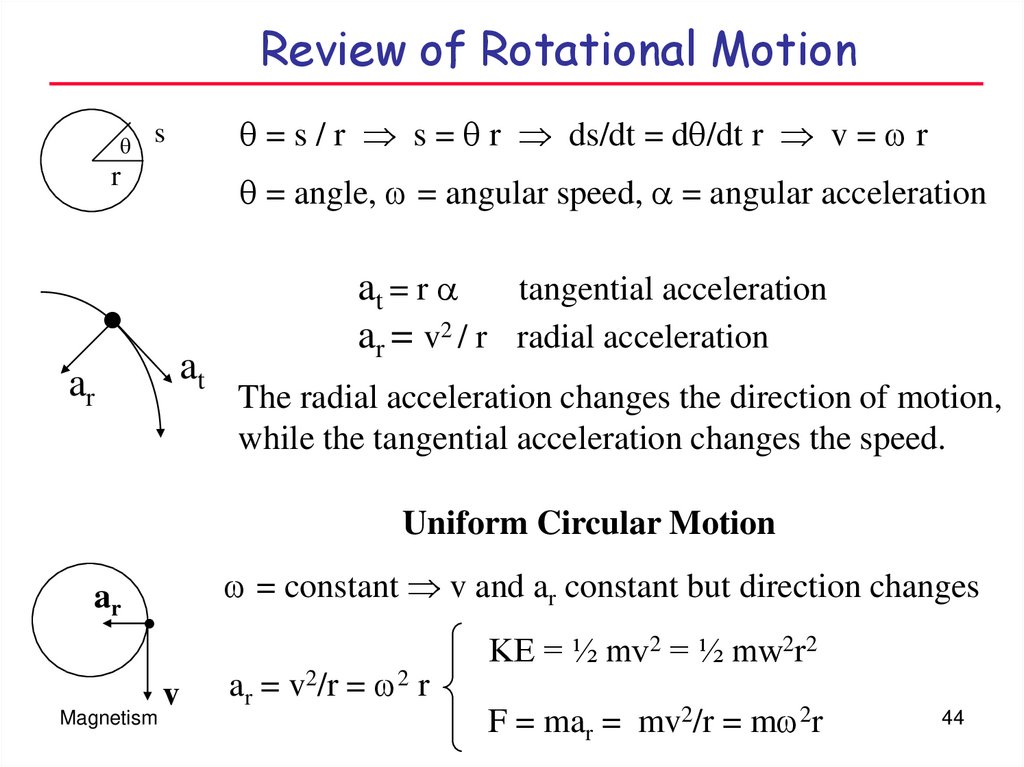
44. Review of Rotational Motion
= s / r s = r ds/dt = d /dt r v = rs
r
= angle, = angular speed, = angular acceleration
at
ar
at = r
tangential acceleration
ar = v2 / r radial acceleration
The radial acceleration changes the direction of motion,
while the tangential acceleration changes the speed.
Uniform Circular Motion
ar
= constant v and ar constant but direction changes
Magnetism
v
ar = v2/r = 2 r
KE = ½ mv2 = ½ mw2r2
F = mar = mv2/r = m 2r
44
45.
Magnetism45
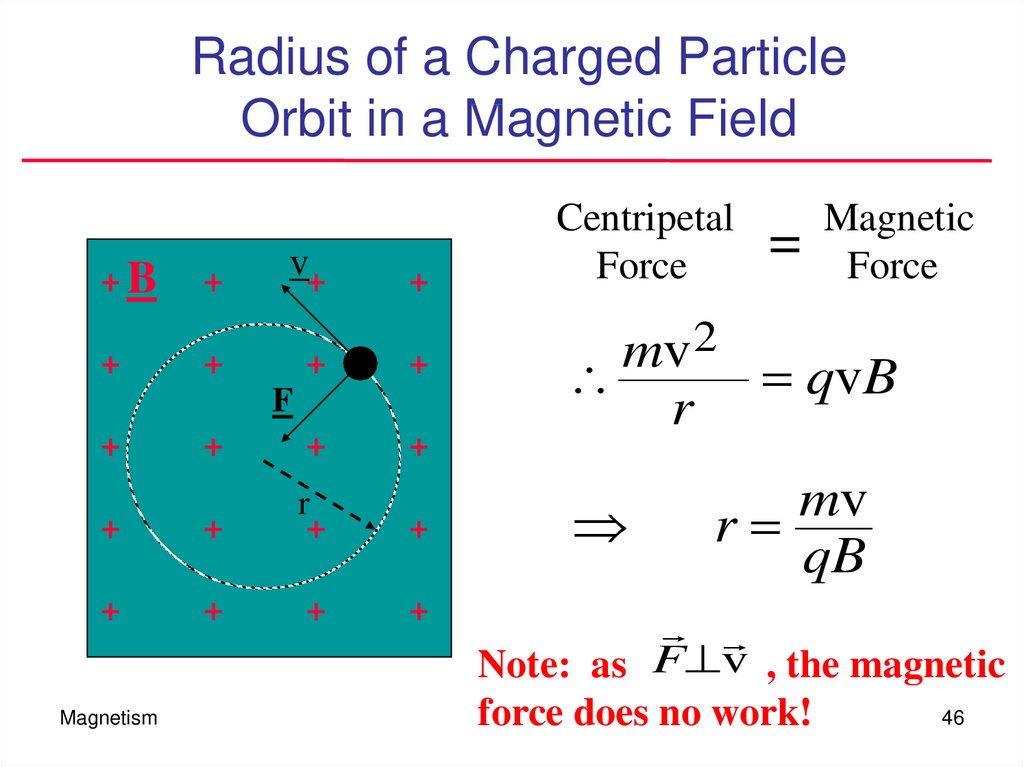
46. Radius of a Charged Particle Orbit in a Magnetic Field
+B+
+
v+
+
+
+
+
+
+
+
+
+
r
+
+
+
+
+
+
F
+
Magnetism
Centripetal
Force
=
Magnetic
Force
mv 2
qvB
r
mv
r
qB
Note: as F v , the magnetic
46
force does no work!
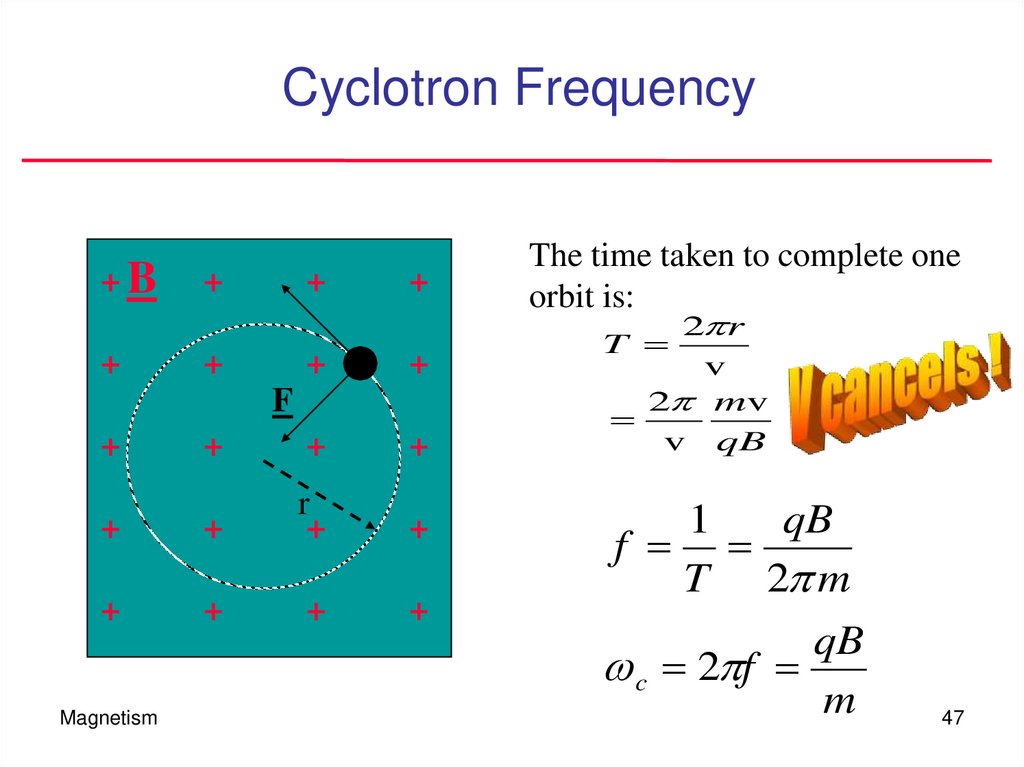
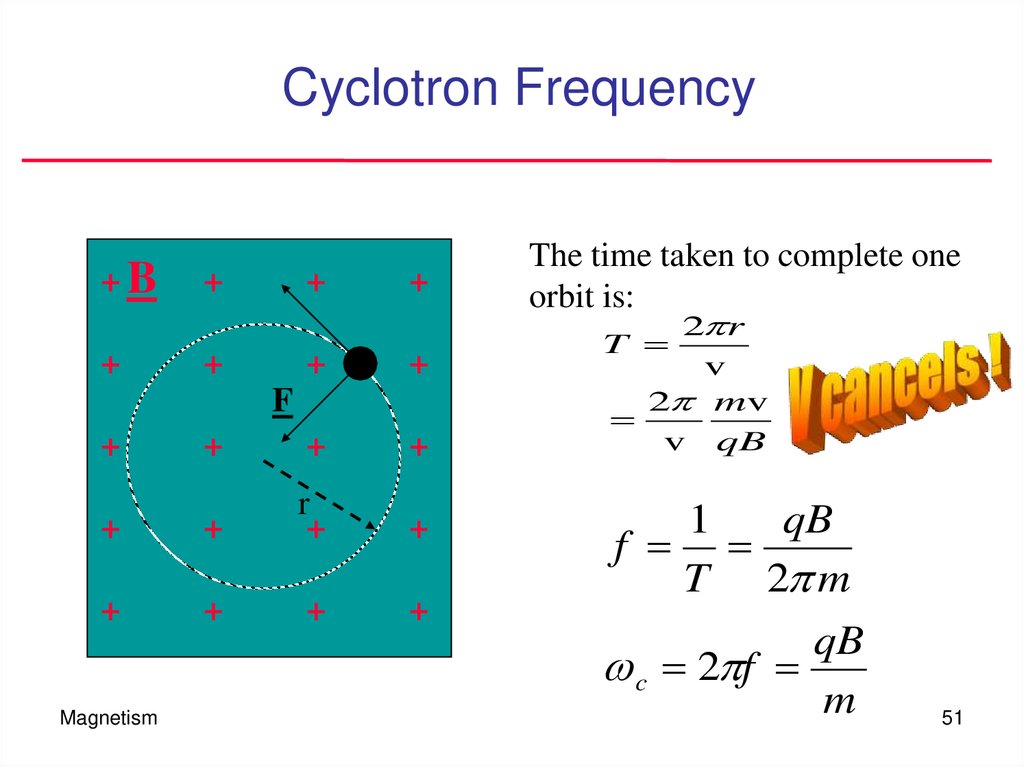
47. Cyclotron Frequency
+B+
+
v+
+
+
+
+
+
+
+
+
+
r
+
+
+
+
+
+
F
+
Magnetism
The time taken to complete one
orbit is:
2 r
v
2 mv
v qB
T
1
qB
f
T 2 m
qB
c 2 f
m
47
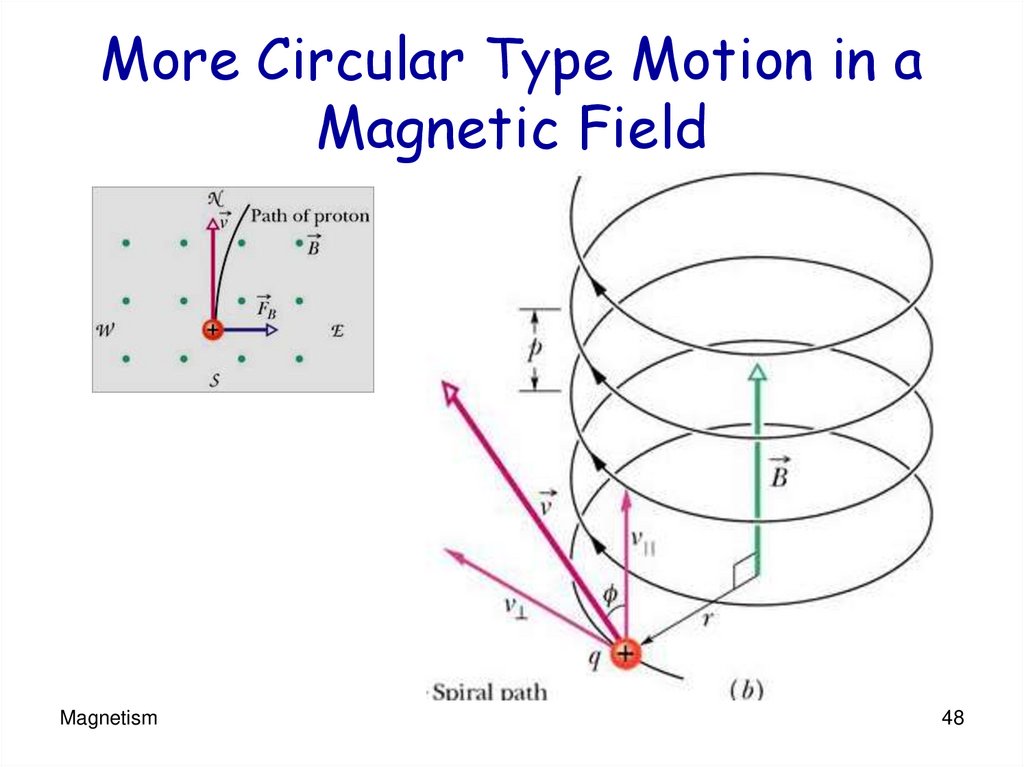
48. More Circular Type Motion in a Magnetic Field
Magnetism48
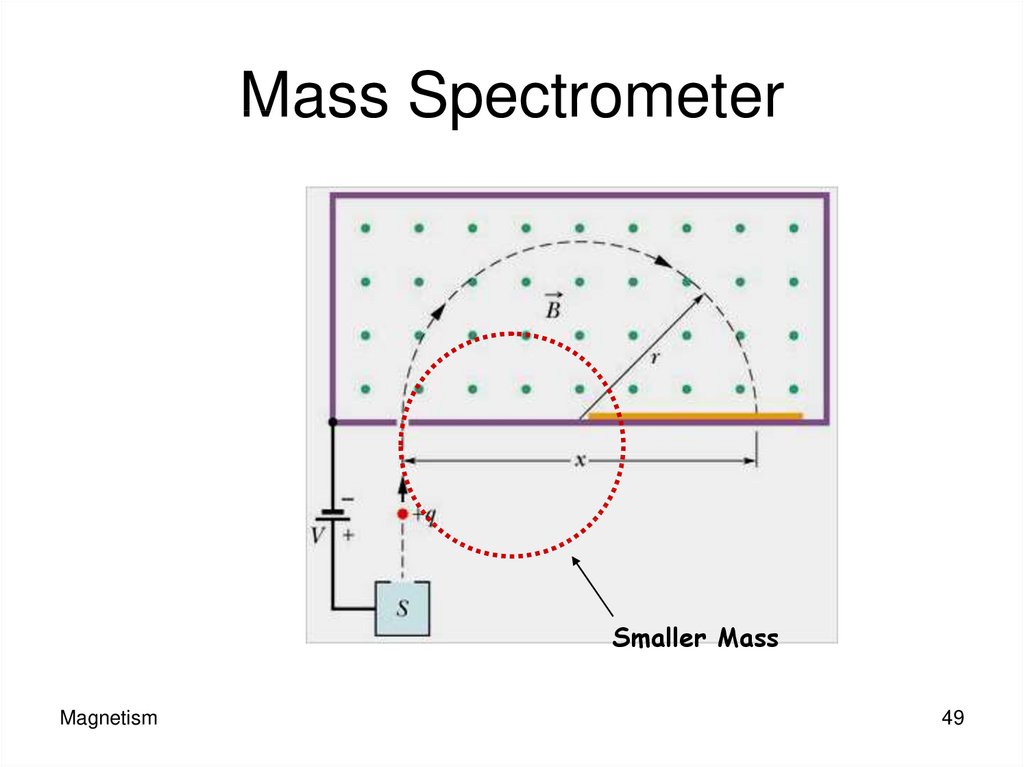
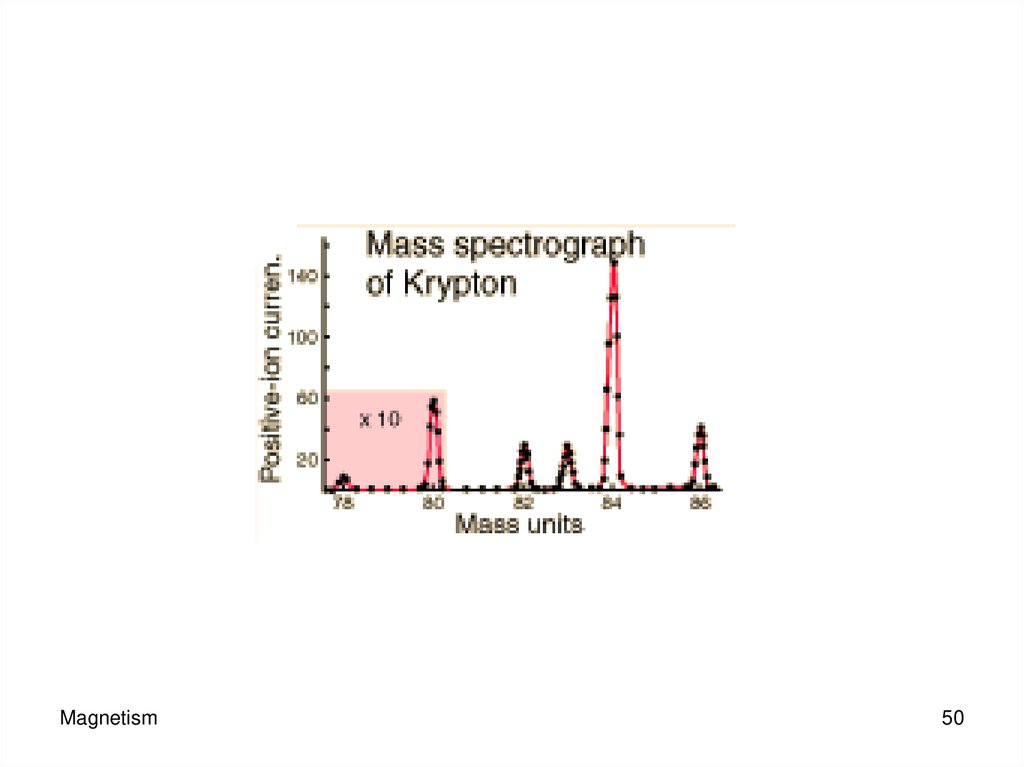
49. Mass Spectrometer
Smaller MassMagnetism
49
50.
Magnetism50
51.
Cyclotron Frequency+B
+
+
v+
+
+
+
+
+
+
+
+
+
r
+
+
+
+
+
+
F
+
Magnetism
The time taken to complete one
orbit is:
2 r
v
2 mv
v qB
T
1
qB
f
T 2 m
qB
c 2 f
m
51
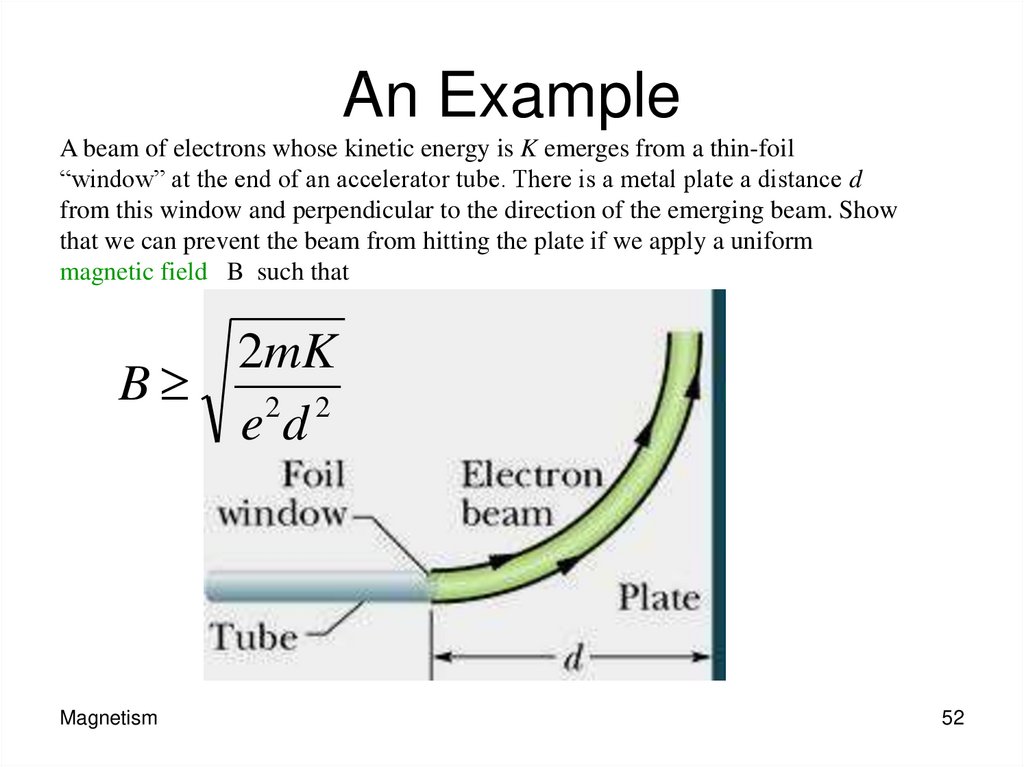
52. An Example
A beam of electrons whose kinetic energy is K emerges from a thin-foil“window” at the end of an accelerator tube. There is a metal plate a distance d
from this window and perpendicular to the direction of the emerging beam. Show
that we can prevent the beam from hitting the plate if we apply a uniform
magnetic field B such that
2mK
B 2 2
ed
Magnetism
52
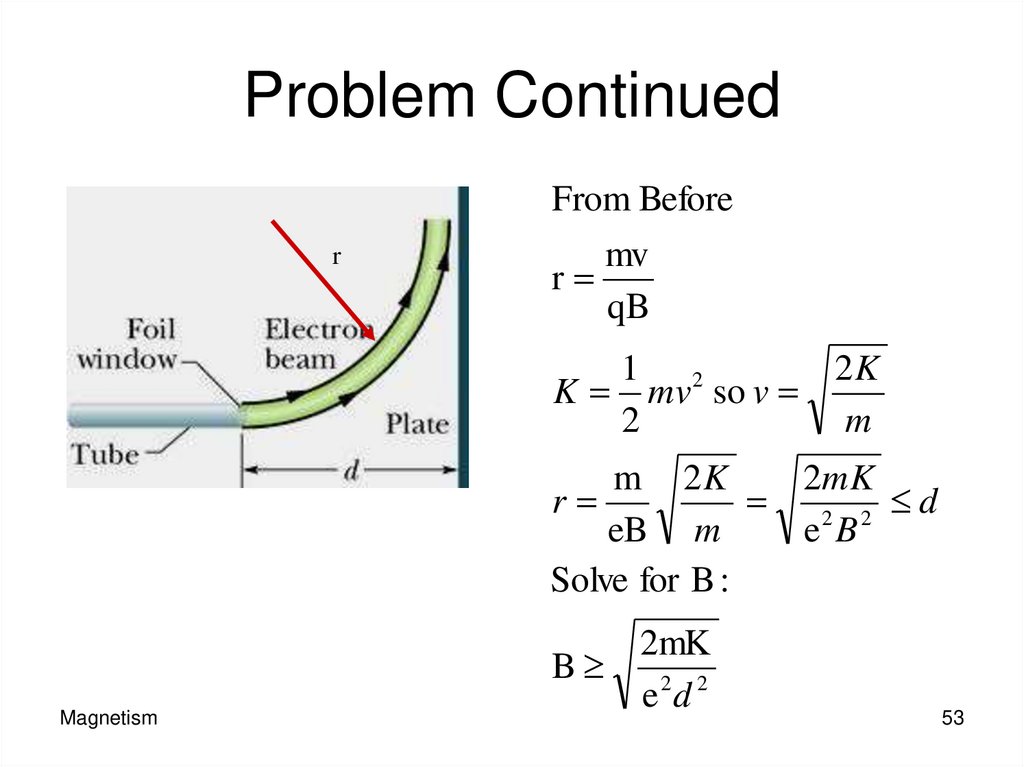
53. Problem Continued
From Beforer
mv
r
qB
1 2
2K
K mv so v
2
m
m 2K
2mK
r
d
2 2
eB m
e B
Solve for B :
Magnetism
2mK
B
e2d 2
53
54. Some New Stuff
Magnetism and ForcesMagnetism
54
55.
Let’s Look at the effect of crossed E and B Fields:x x x B
E
x x x
v
q , m
Magnetism
55
56.
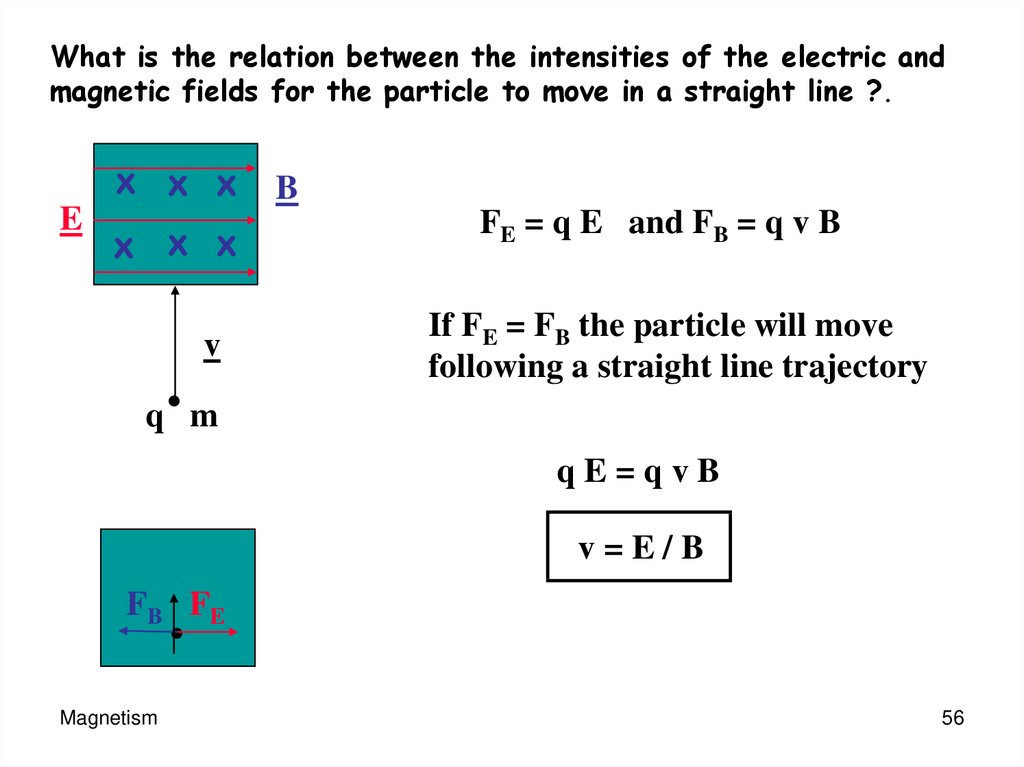
What is the relation between the intensities of the electric andmagnetic fields for the particle to move in a straight line ?.
x x x B
E
x x x
v
q• m
FE = q E and FB = q v B
If FE = FB the particle will move
following a straight line trajectory
qE=qvB
v=E/B
FB FE
Magnetism
56
57. What does this mean??
v=E/BMagnetism
This equation only
contains the E and
B fields in it.
Mass is missing!
Charge is missing!
This configuration
is a velocity filter!
57
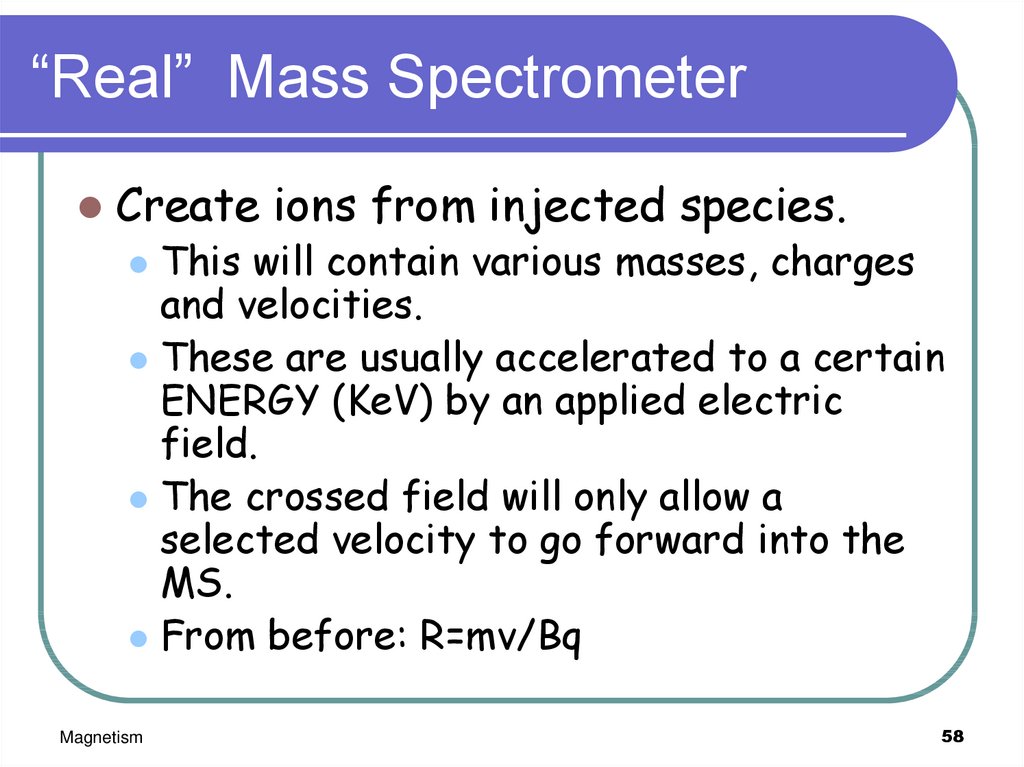
58. “Real” Mass Spectrometer
Create ions from injected species.This will contain various masses, charges
and velocities.
These are usually accelerated to a certain
ENERGY (KeV) by an applied electric
field.
The crossed field will only allow a
selected velocity to go forward into the
MS.
From before: R=mv/Bq
Magnetism
58
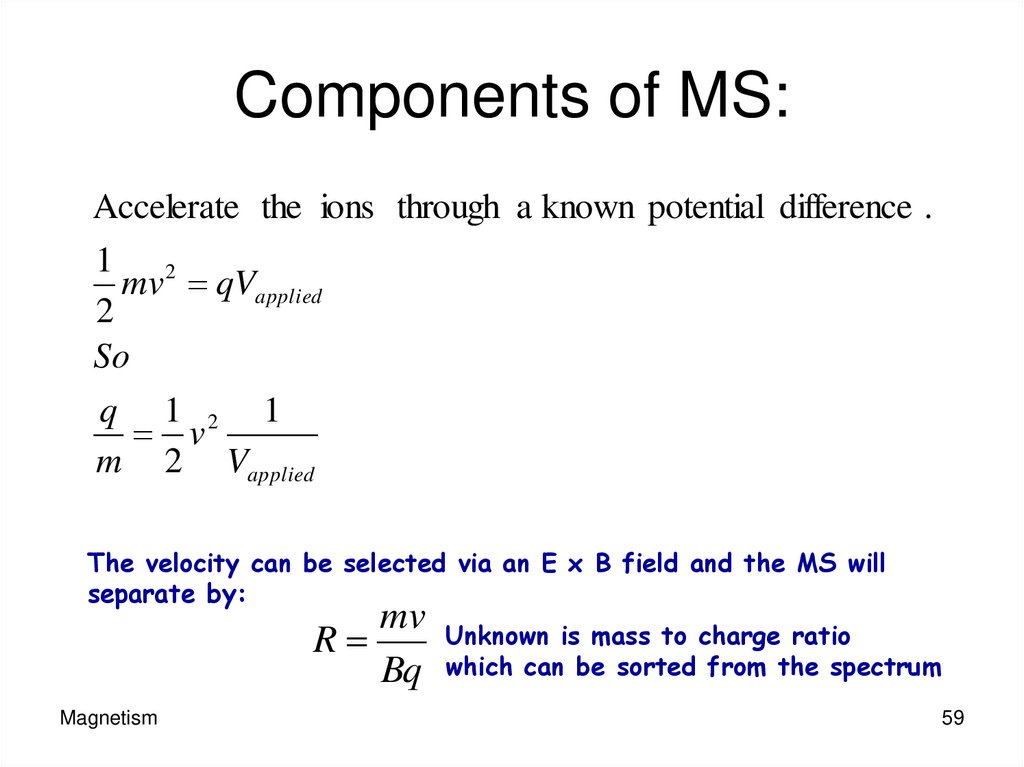
59. Components of MS:
Accelerate the ions through a known potential difference .1 2
mv qVapplied
2
So
q 1 2 1
v
m 2 Vapplied
The velocity can be selected via an E x B field and the MS will
separate by:
mv
R
Bq
Magnetism
Unknown is mass to charge ratio
which can be sorted from the spectrum
59
60.
Magnetism60
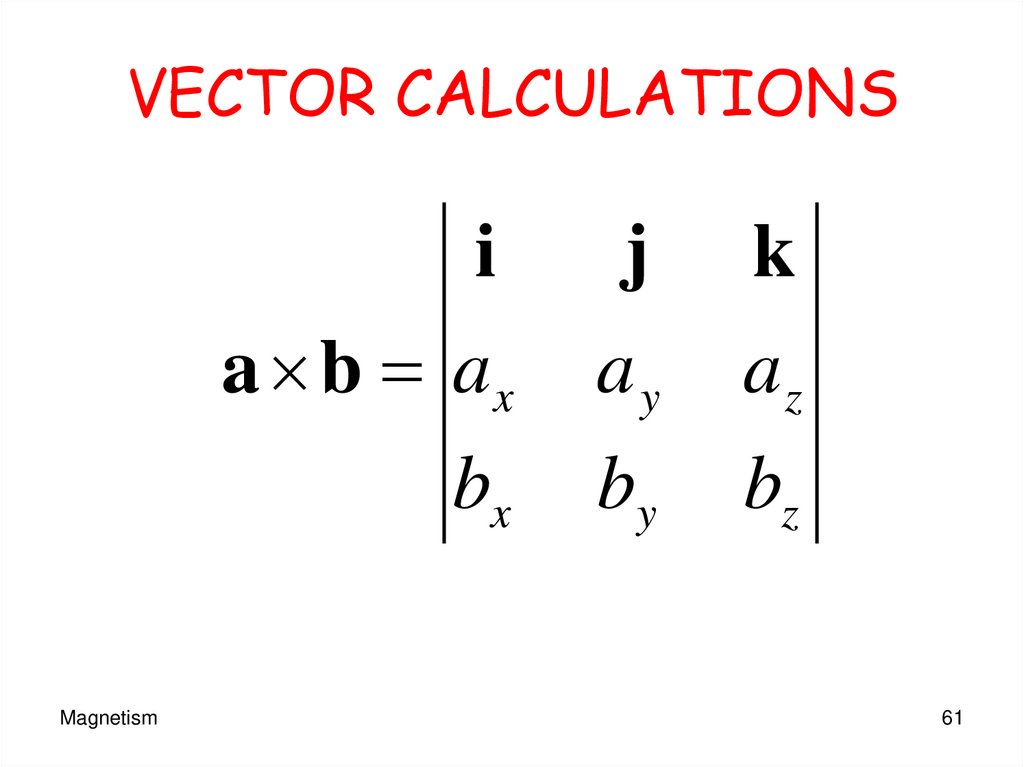
61. VECTOR CALCULATIONS
Magnetismi
a b ax
j
ay
k
az
bx
by
bz
61
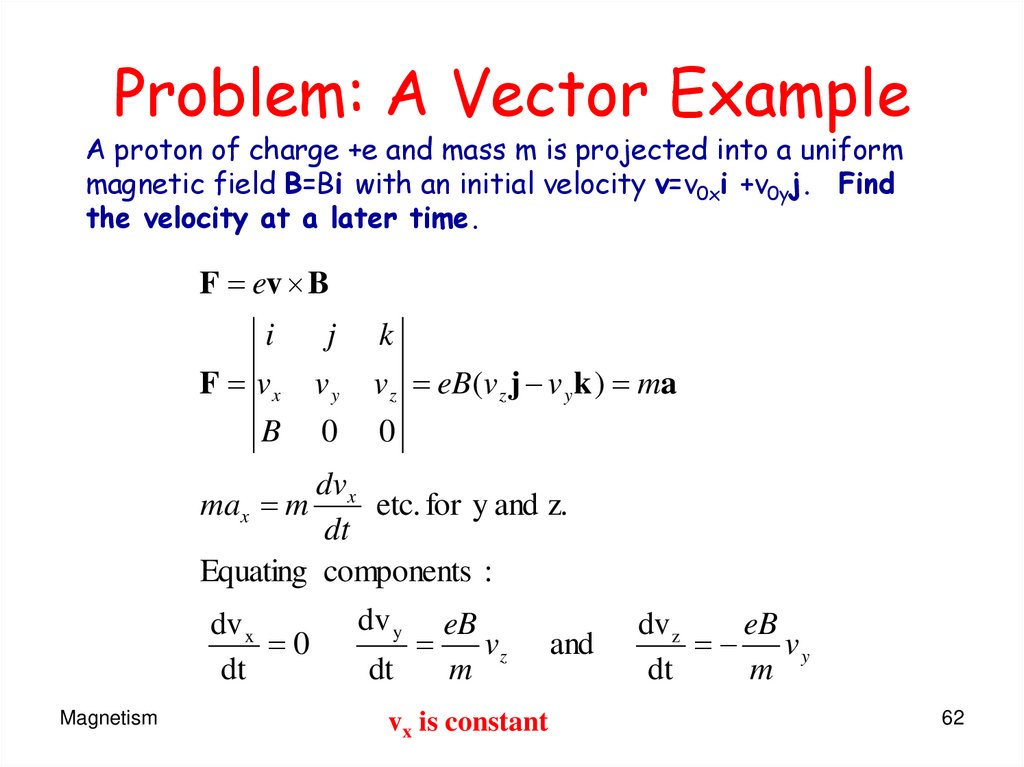
62. Problem: A Vector Example
A proton of charge +e and mass m is projected into a uniformmagnetic field B=Bi with an initial velocity v=v0xi +v0yj. Find
the velocity at a later time.
F ev B
i
F vx
B
j
vy
0
k
vz eB(v z j v y k ) ma
0
dvx
max m
etc. for y and z.
dt
Equating components :
dv y eB
dv x
0
v z and
dt
dt
m
Magnetism
vx is constant
dv z
eB
vy
dt
m
62
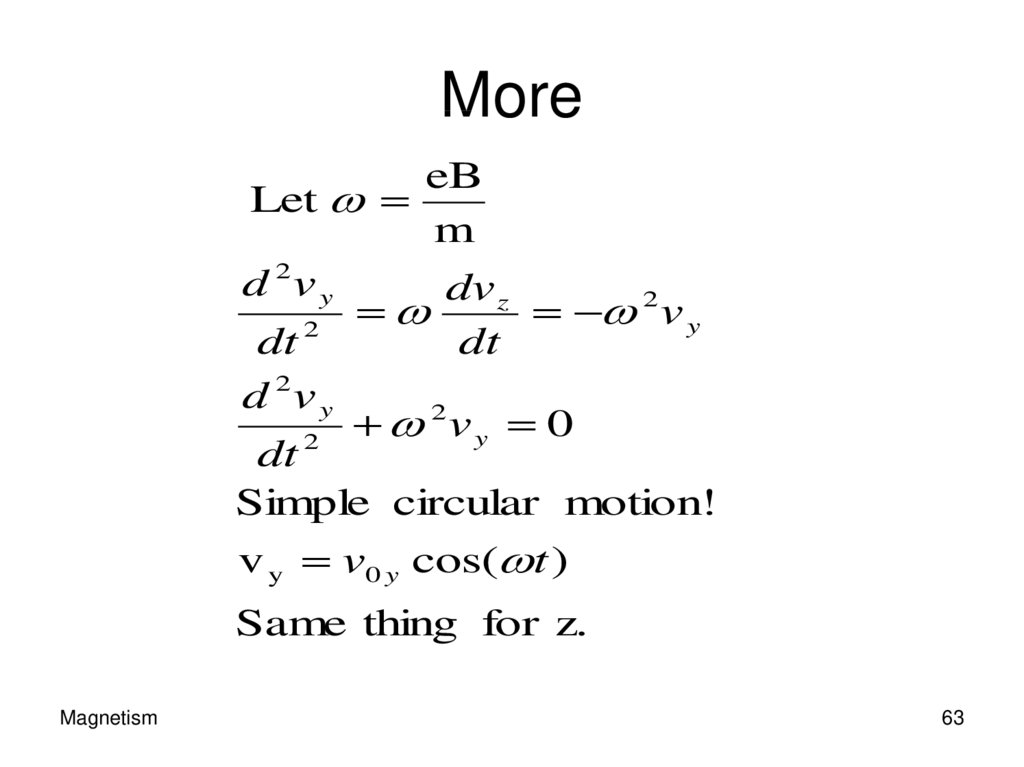
63. More
eBLet
m
d 2v y
dv z
2
vy
2
dt
dt
d 2v y
2
vy 0
2
dt
Simple circular motion!
v y v0 y cos( t )
Same thing for z.
Magnetism
63
64.
Magnetism64
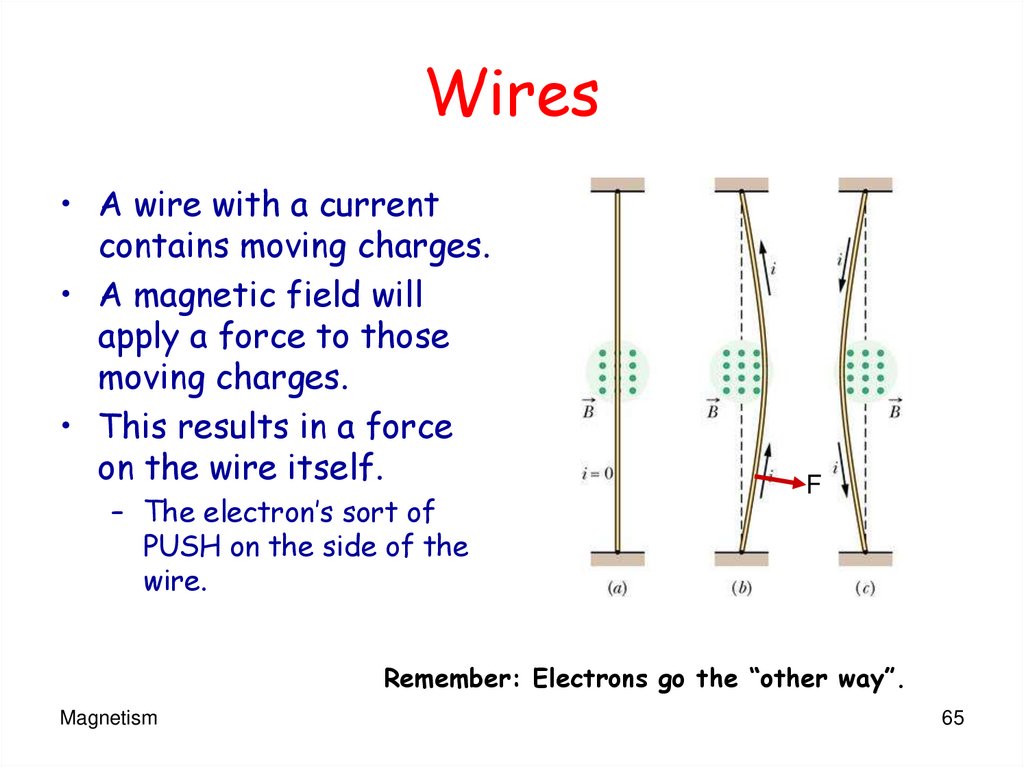
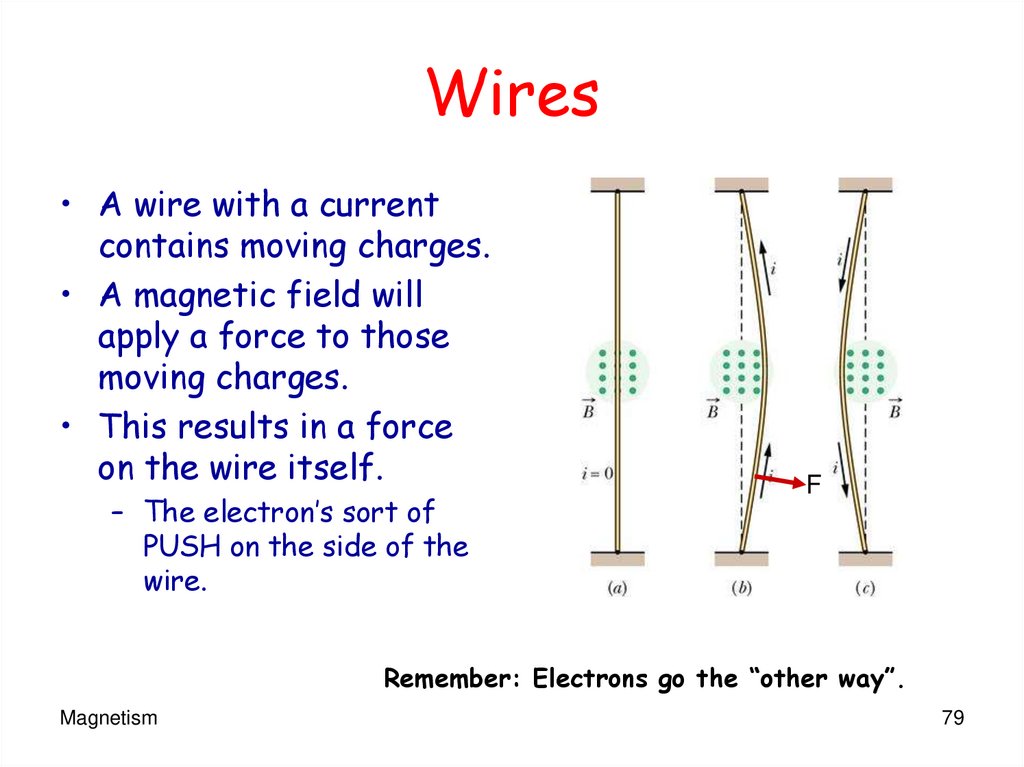
65. Wires
• A wire with a currentcontains moving charges.
• A magnetic field will
apply a force to those
moving charges.
• This results in a force
on the wire itself.
– The electron’s sort of
PUSH on the side of the
wire.
F
Remember: Electrons go the “other way”.
Magnetism
65
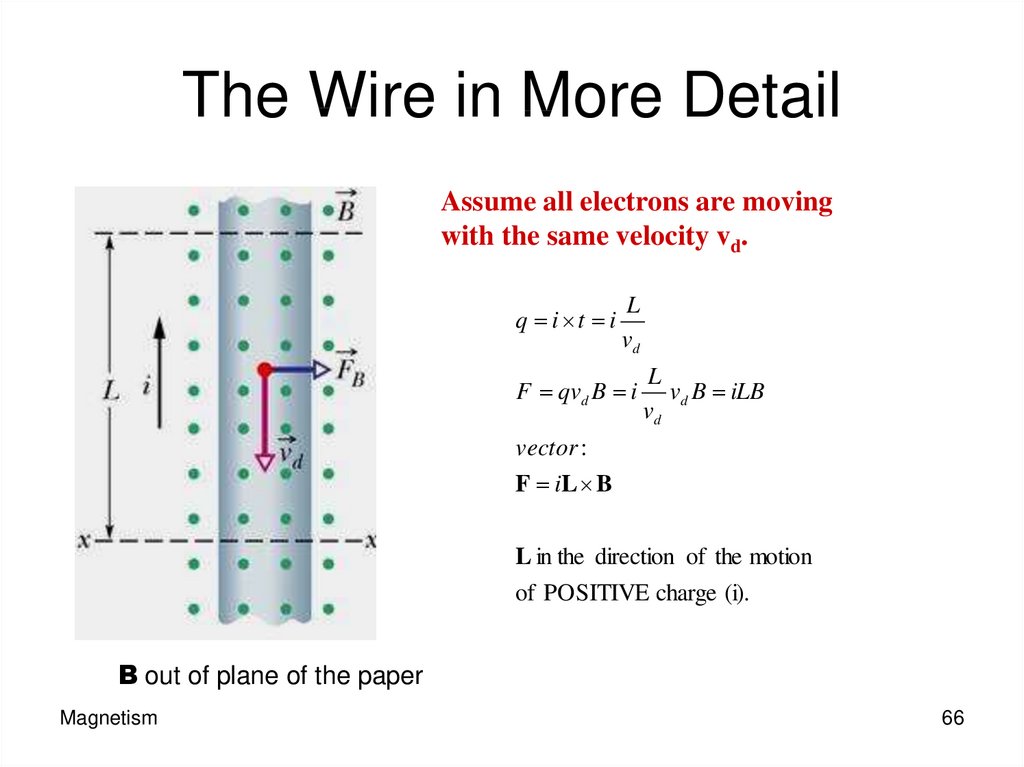
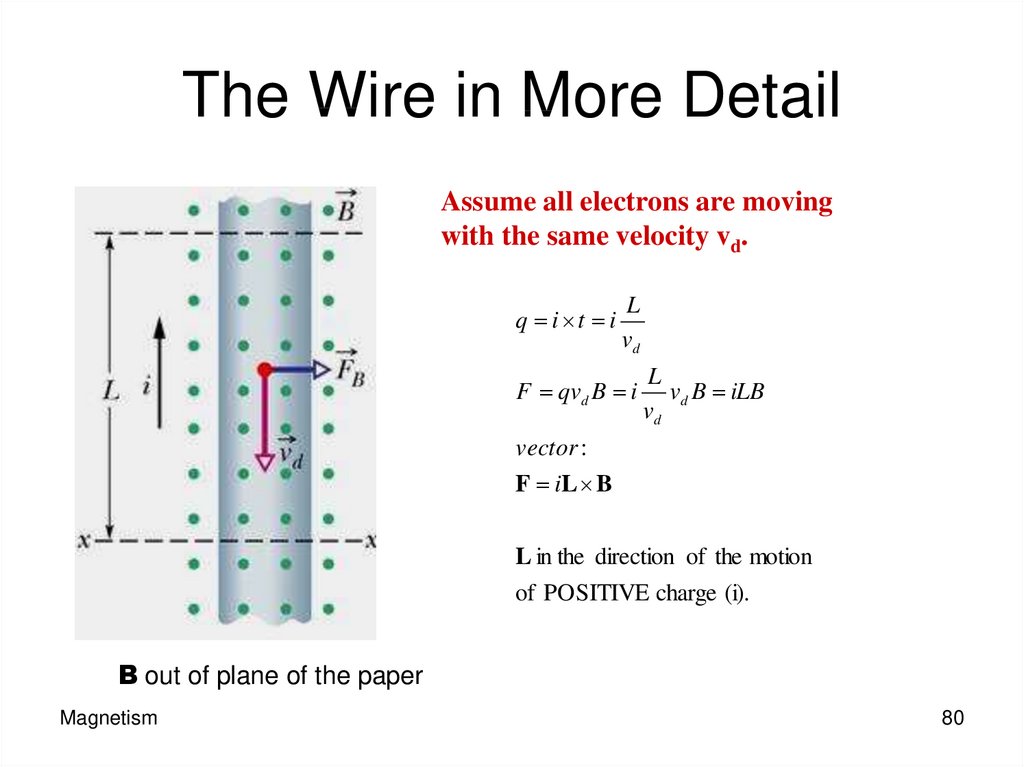
66. The Wire in More Detail
Assume all electrons are movingwith the same velocity vd.
q i t i
L
vd
F qvd B i
L
vd B iLB
vd
vector :
F iL B
L in the direction of the motion
of POSITIVE charge (i).
B out of plane of the paper
Magnetism
66
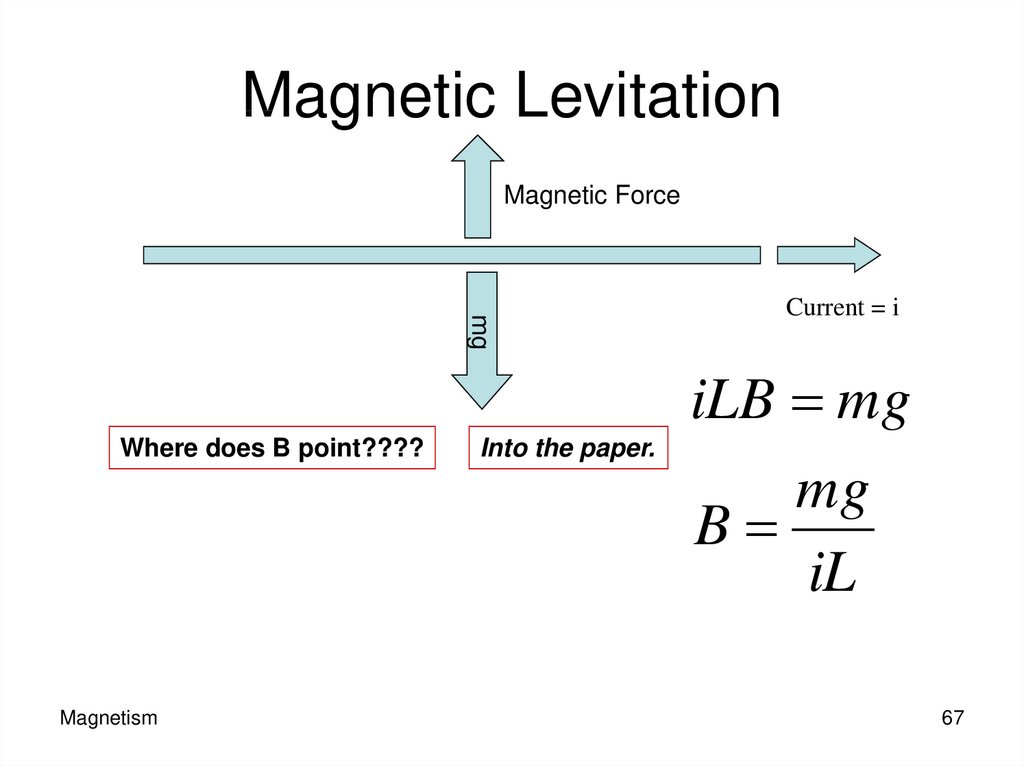
67. Magnetic Levitation
Magnetic Forcemg
Where does B point????
Magnetism
Into the paper.
Current = i
iLB mg
mg
B
iL
67
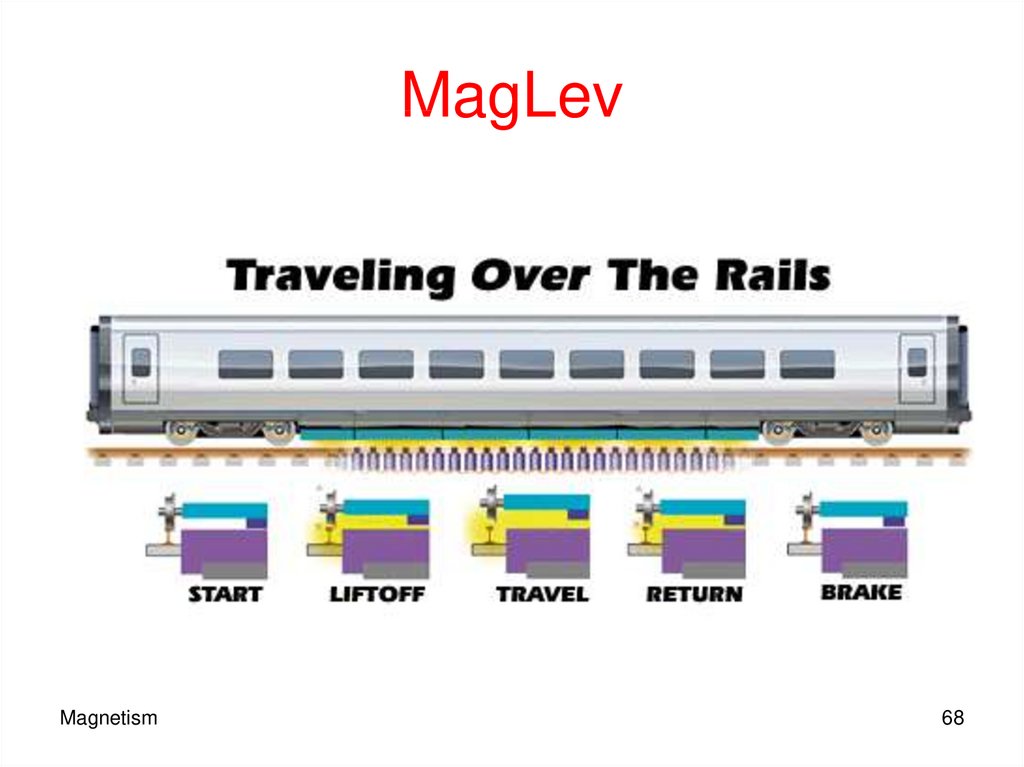
68. MagLev
Magnetism68
69. Magnetic Repulsion
Magnetism69
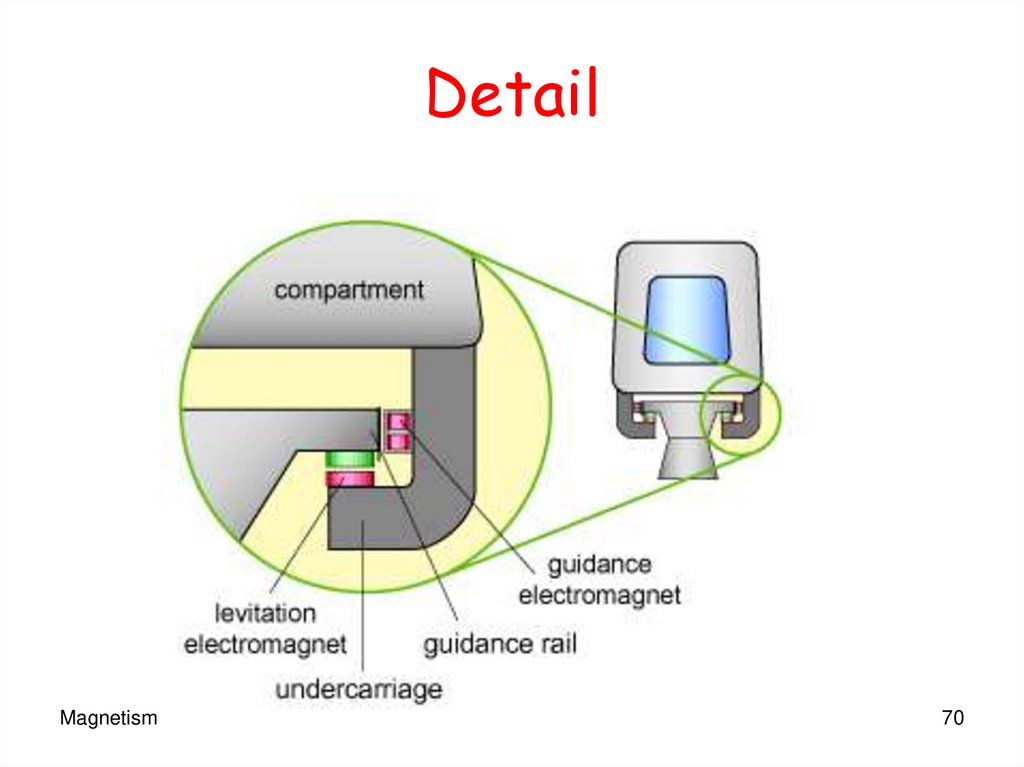
70. Detail
Magnetism70
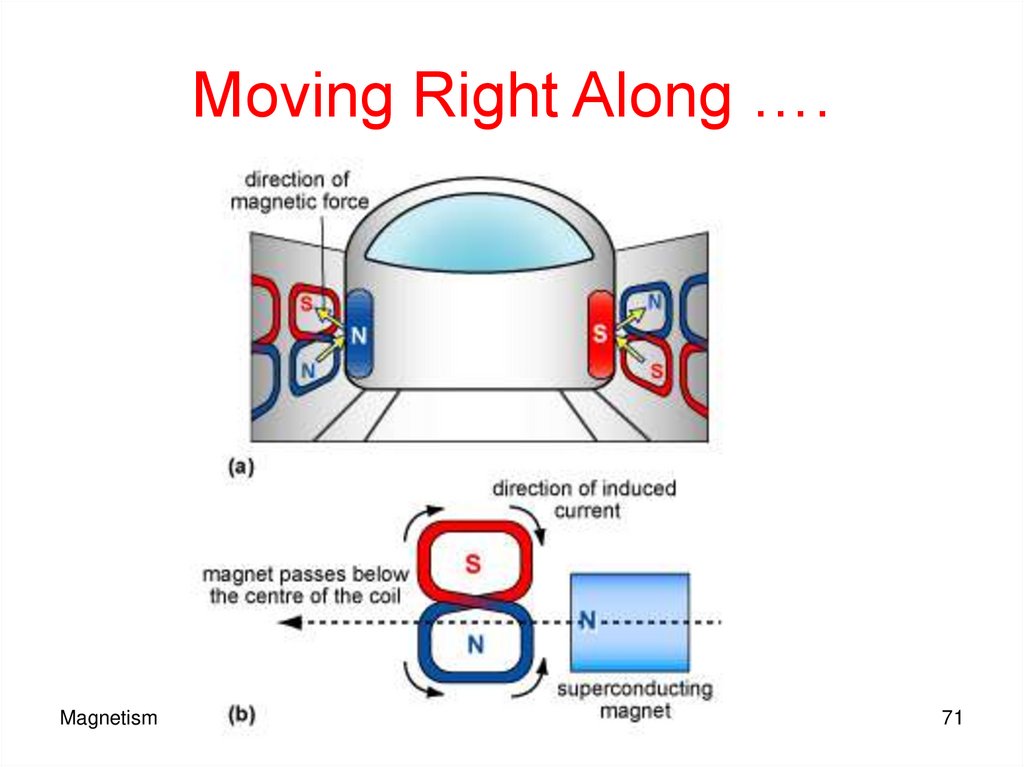
71. Moving Right Along ….
Magnetism71
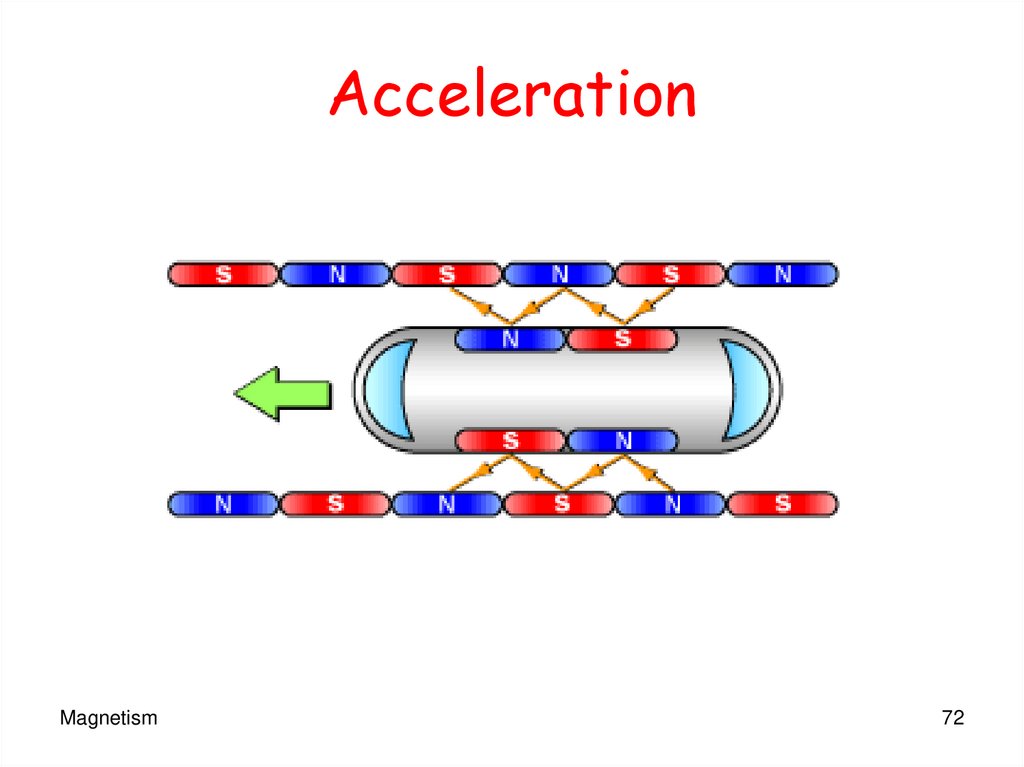
72. Acceleration
Magnetism72
73. Don’t Buy A Ticket Quite Yet..
This is still experimental.Much development still required.
Some of these attempts have been
abandoned because of the high cost
of building a MagLev train.
Probably 10-20 years out.
Or More.
Magnetism
73
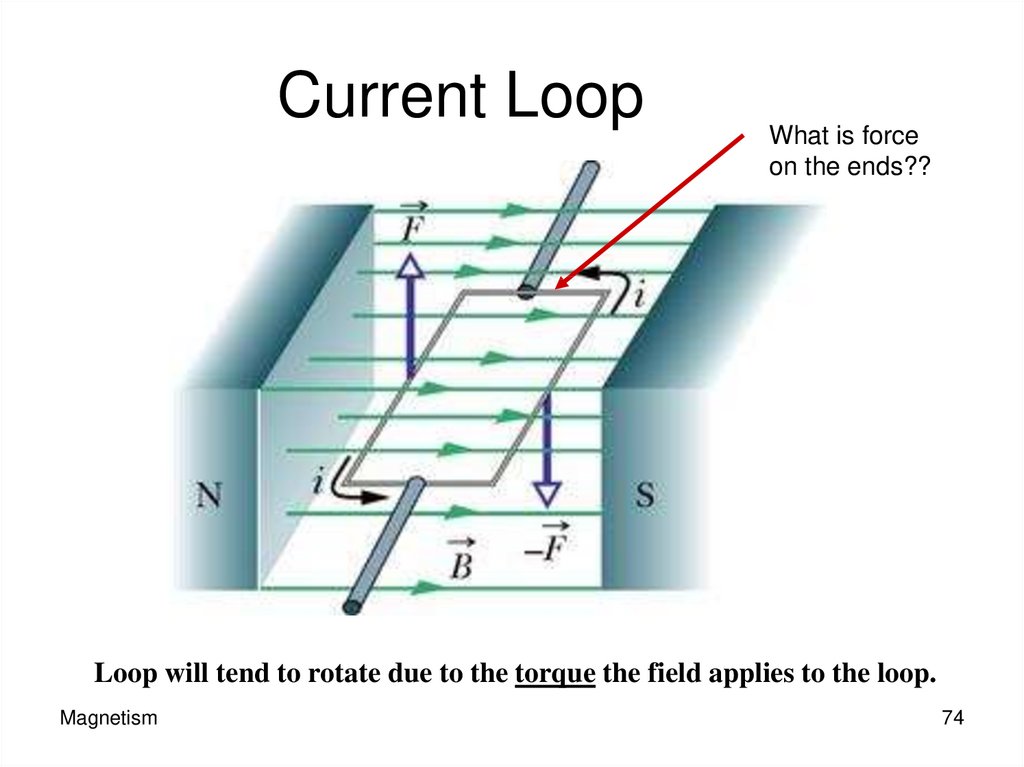
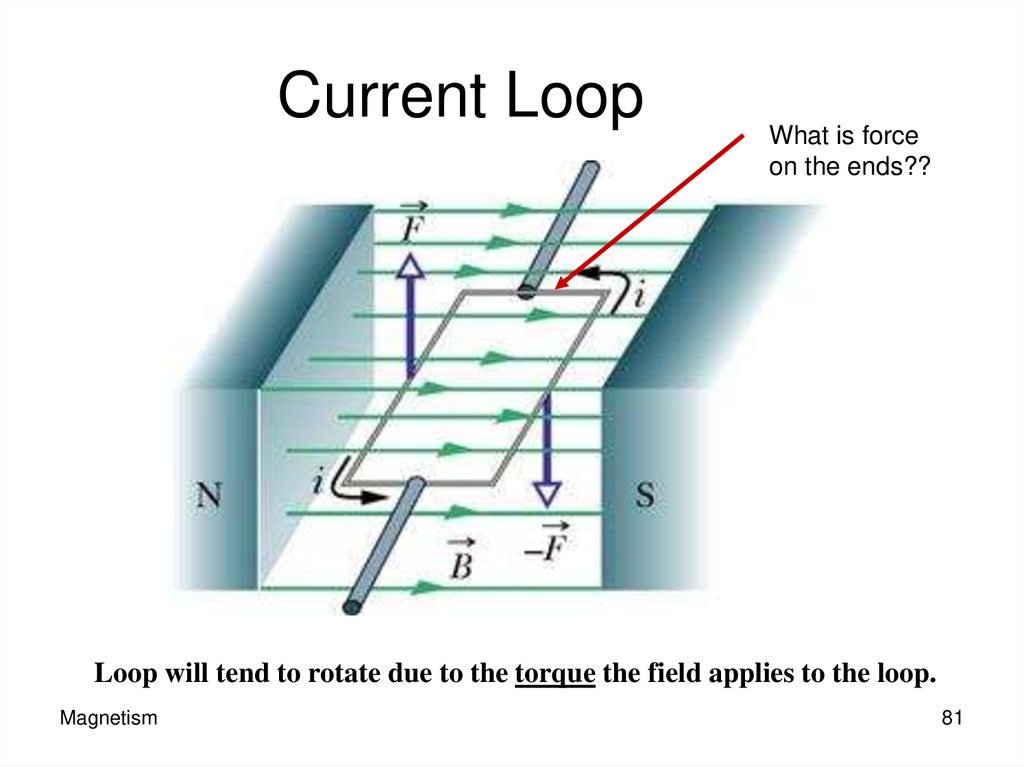
74. Current Loop
What is forceon the ends??
Loop will tend to rotate due to the torque the field applies to the loop.
Magnetism
74
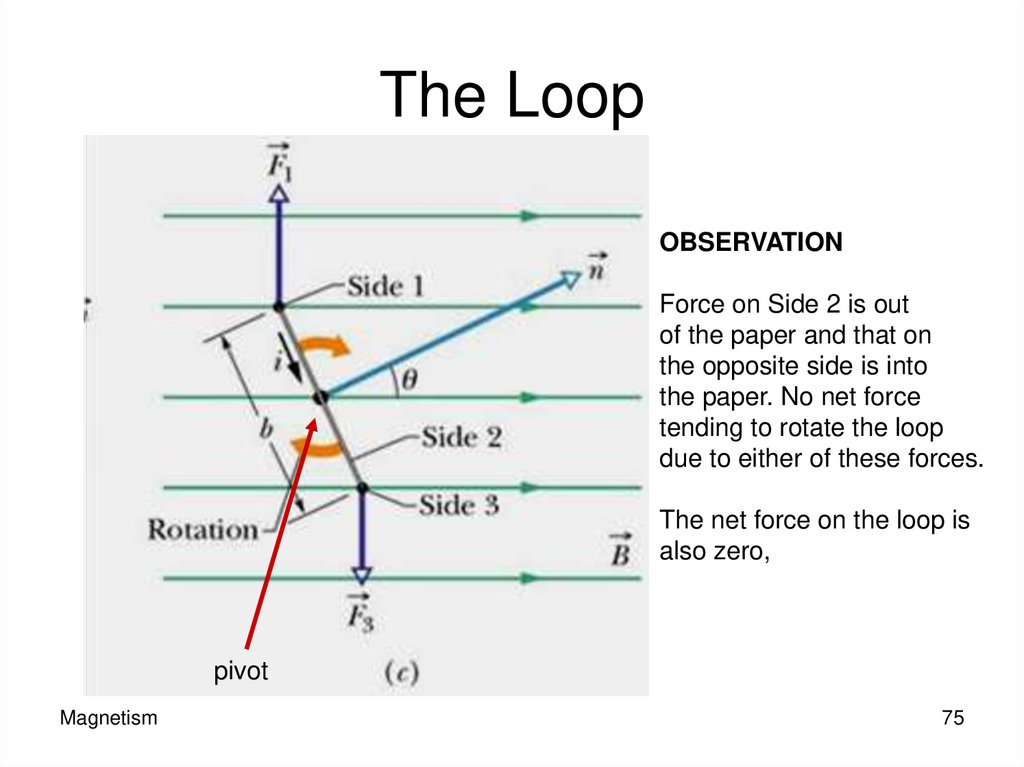
75. The Loop
OBSERVATIONForce on Side 2 is out
of the paper and that on
the opposite side is into
the paper. No net force
tending to rotate the loop
due to either of these forces.
The net force on the loop is
also zero,
pivot
Magnetism
75
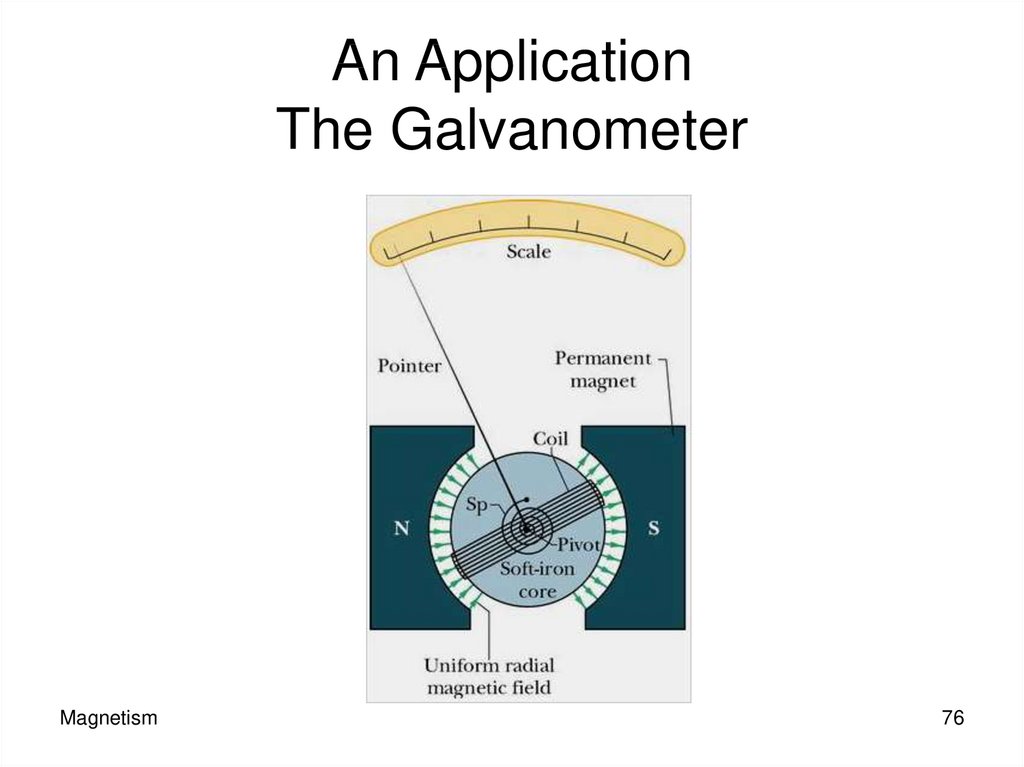
76. An Application The Galvanometer
Magnetism76
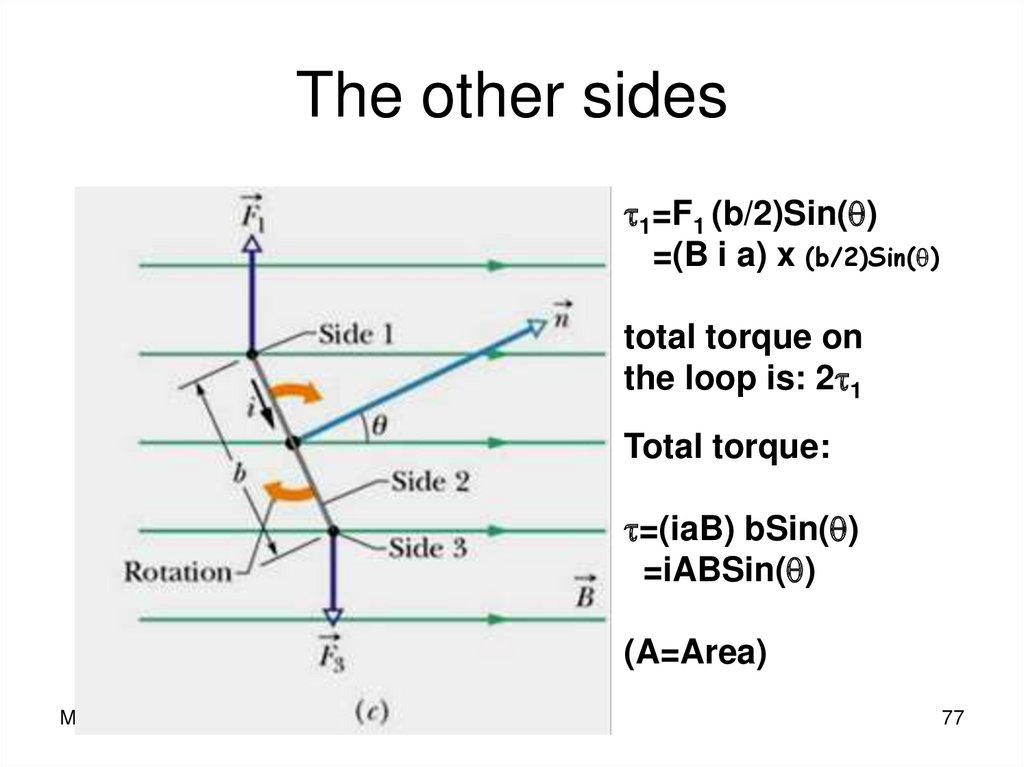
77. The other sides
t1=F1 (b/2)Sin( )=(B i a) x (b/2)Sin( )
total torque on
the loop is: 2t1
Total torque:
t=(iaB) bSin( )
=iABSin( )
(A=Area)
Magnetism
77
78. Watcha Gonna Do
Quiz TodayReturn to Magnetic Material
Exams not yet returned. Sorry.
Magnetism
78
79. Wires
• A wire with a currentcontains moving charges.
• A magnetic field will
apply a force to those
moving charges.
• This results in a force
on the wire itself.
– The electron’s sort of
PUSH on the side of the
wire.
F
Remember: Electrons go the “other way”.
Magnetism
79
80. The Wire in More Detail
Assume all electrons are movingwith the same velocity vd.
q i t i
L
vd
F qvd B i
L
vd B iLB
vd
vector :
F iL B
L in the direction of the motion
of POSITIVE charge (i).
B out of plane of the paper
Magnetism
80
81. Current Loop
What is forceon the ends??
Loop will tend to rotate due to the torque the field applies to the loop.
Magnetism
81
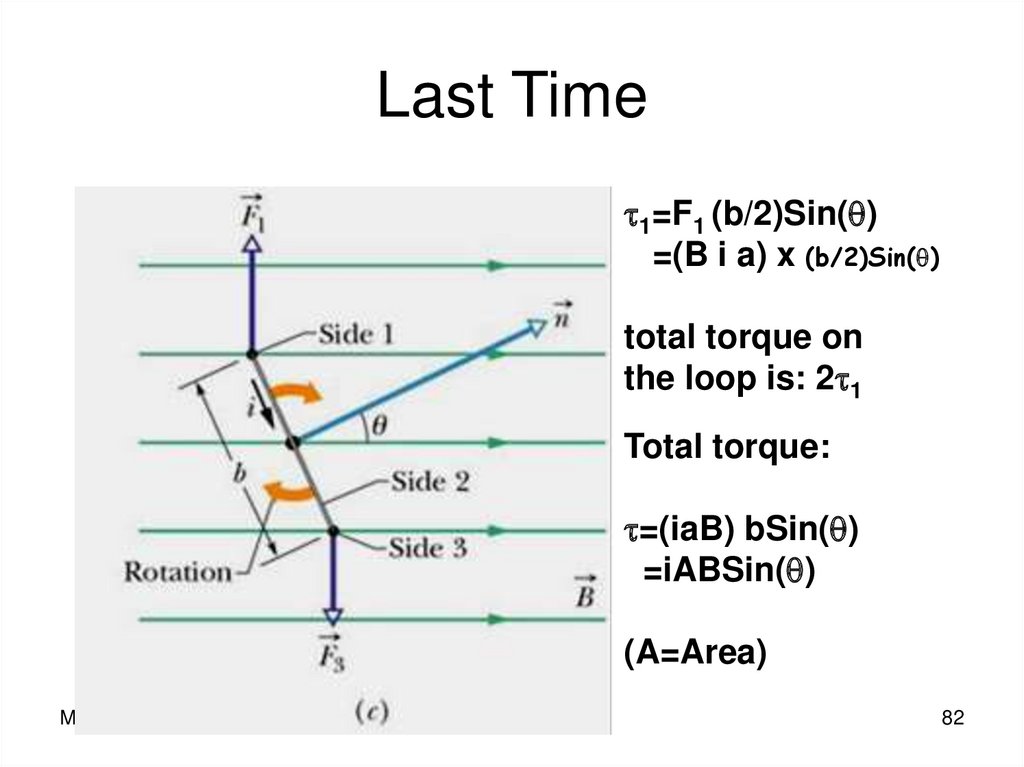
82. Last Time
t1=F1 (b/2)Sin( )=(B i a) x (b/2)Sin( )
total torque on
the loop is: 2t1
Total torque:
t=(iaB) bSin( )
=iABSin( )
(A=Area)
Magnetism
82
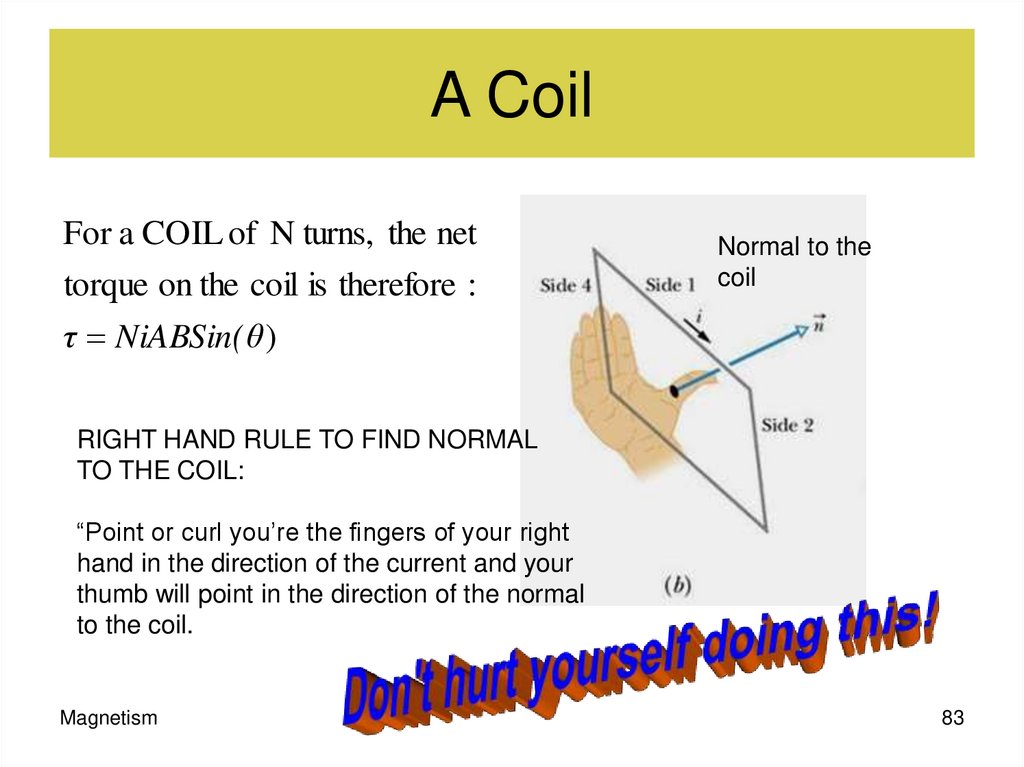
83. A Coil
For a COIL of N turns, the nettorque on the coil is therefore :
τ NiABSin(θ )
Normal to the
coil
RIGHT HAND RULE TO FIND NORMAL
TO THE COIL:
“Point or curl you’re the fingers of your right
hand in the direction of the current and your
thumb will point in the direction of the normal
to the coil.
Magnetism
83
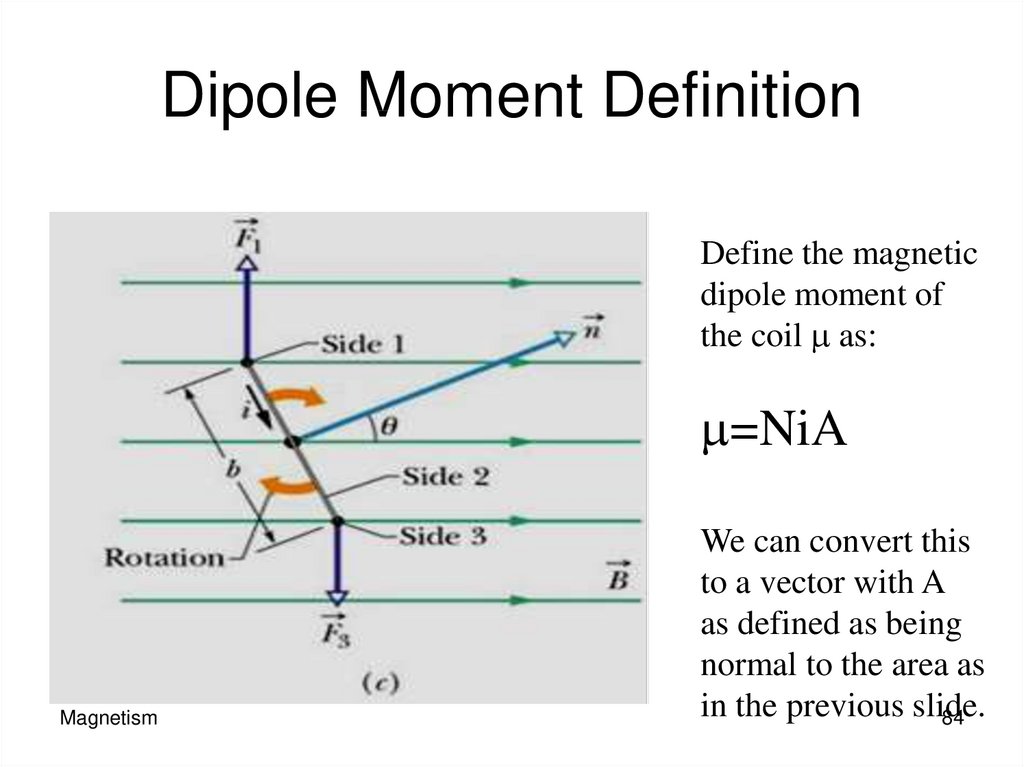
84. Dipole Moment Definition
Define the magneticdipole moment of
the coil m as:
m=NiA
Magnetism
We can convert this
to a vector with A
as defined as being
normal to the area as
in the previous slide.
84
85. Current Loop
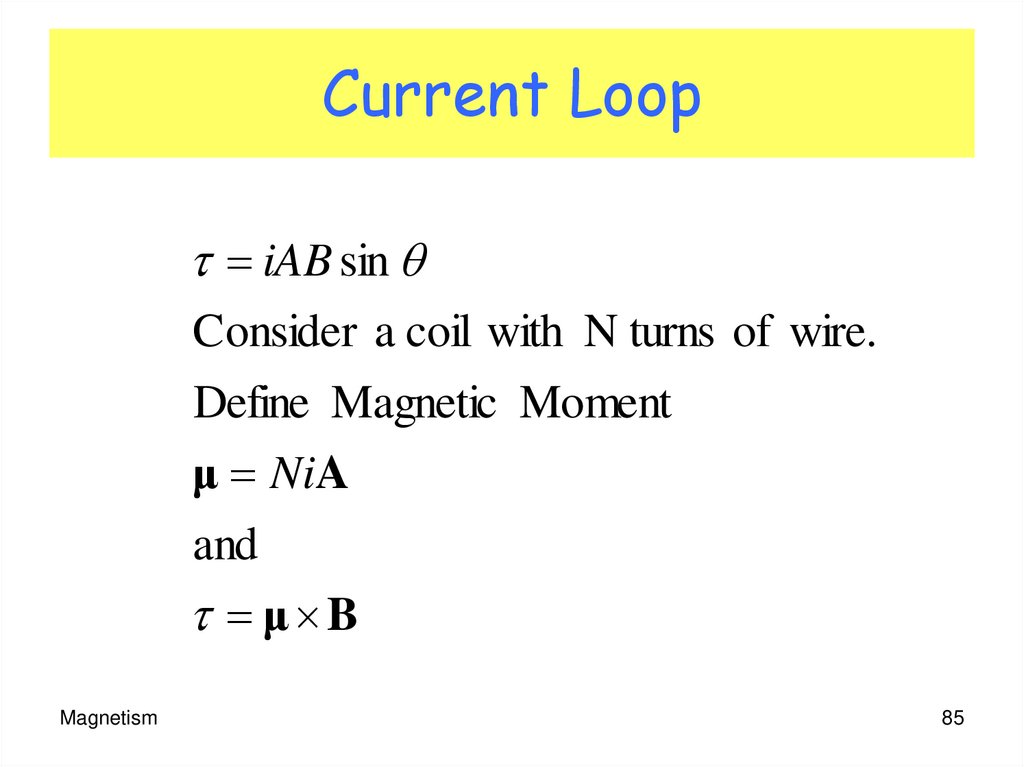
t iAB sinConsider a coil with N turns of wire.
Define Magnetic Moment
μ NiA
and
t μ B
Magnetism
85
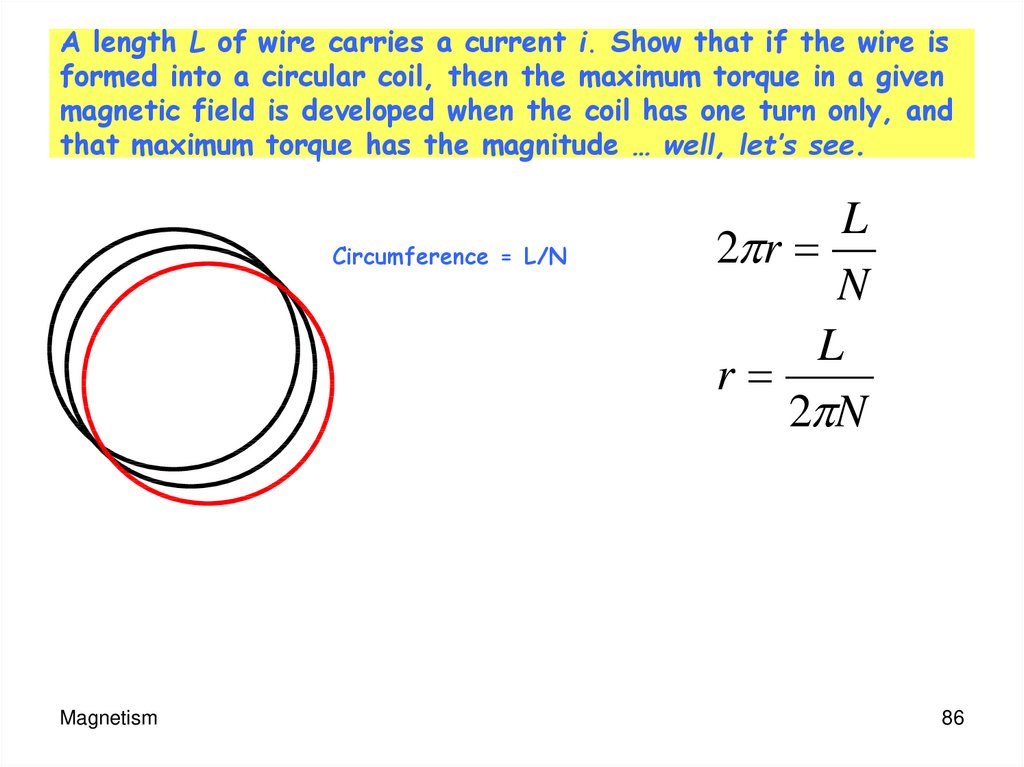
86. A length L of wire carries a current i. Show that if the wire is formed into a circular coil, then the maximum torque in a
givenmagnetic field is developed when the coil has one turn only, and
that maximum torque has the magnitude … well, let’s see.
Circumference = L/N
Magnetism
L
2 r
N
L
r
2 N
86
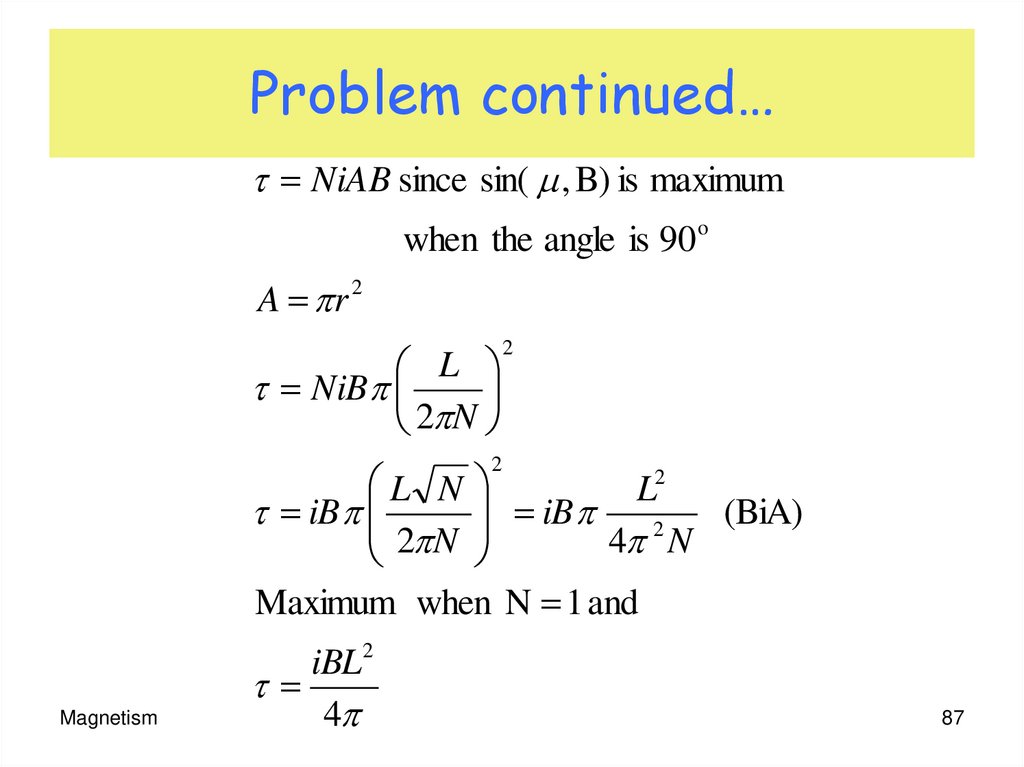
87. Problem continued…
t NiAB since sin( m , B) is maximumwhen the angle is 90 o
A r 2
L
t NiB
2 N
2
2
L N
L2
iB
t iB
(BiA)
2
4 N
2 N
Maximum when N 1 and
Magnetism
iBL2
t
4
87
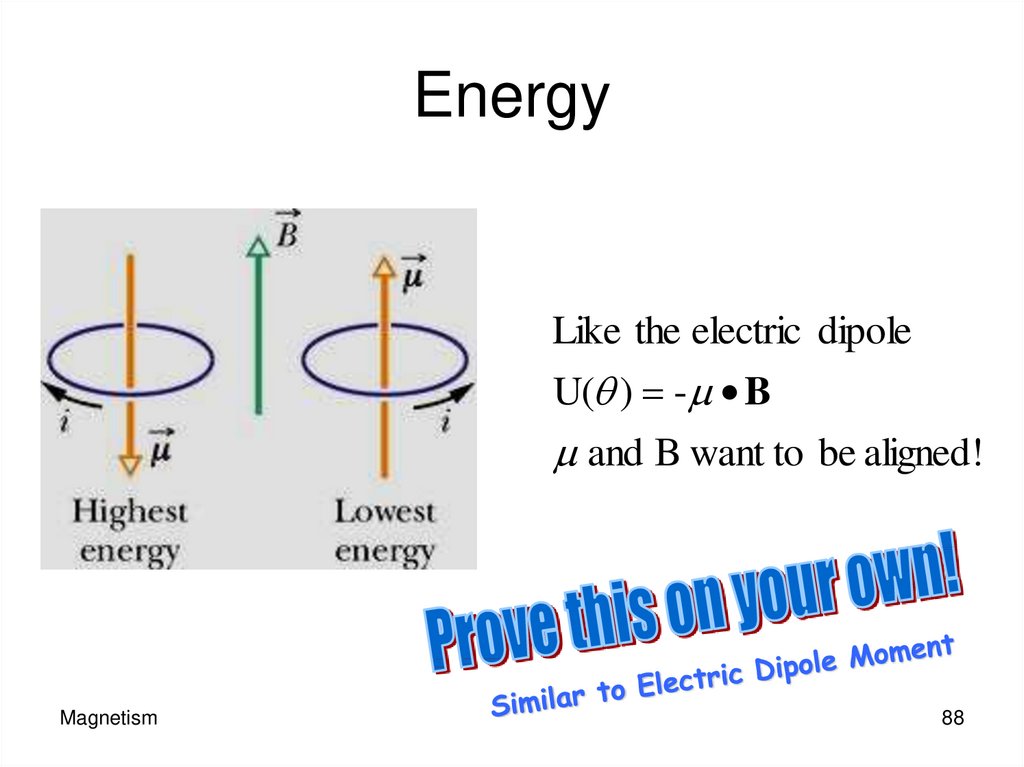
88. Energy
Like the electric dipoleU( ) -m B
m and B want to be aligned!
Magnetism
88
89. The Hall Effect
Magnetism89
90. What Does it Do?
• Allows the measurement ofMagnetic Field if a material is
known.
• Allows the determination of the
“type” of current carrier in
semiconductors if the magnetic
field is known.
• Electrons
Magnetism
• Holes
90
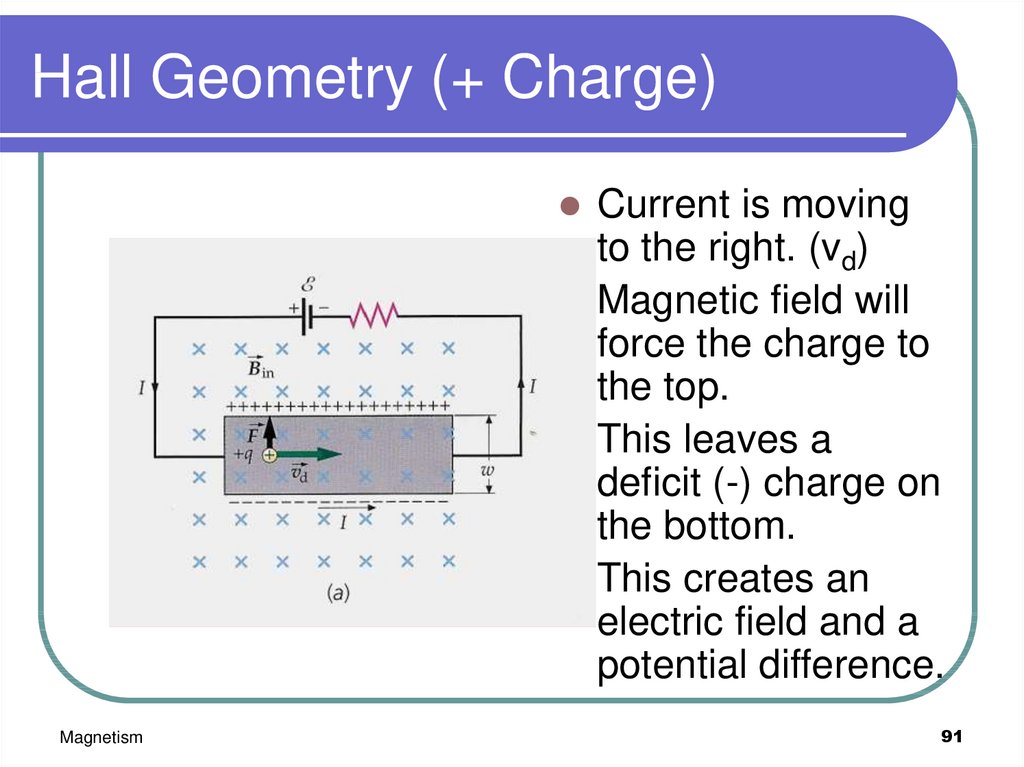
91. Hall Geometry (+ Charge)
Current is movingto the right. (vd)
Magnetic field will
force the charge to
the top.
This leaves a
deficit (-) charge on
the bottom.
This creates an
electric field and a
potential difference.
Magnetism
91
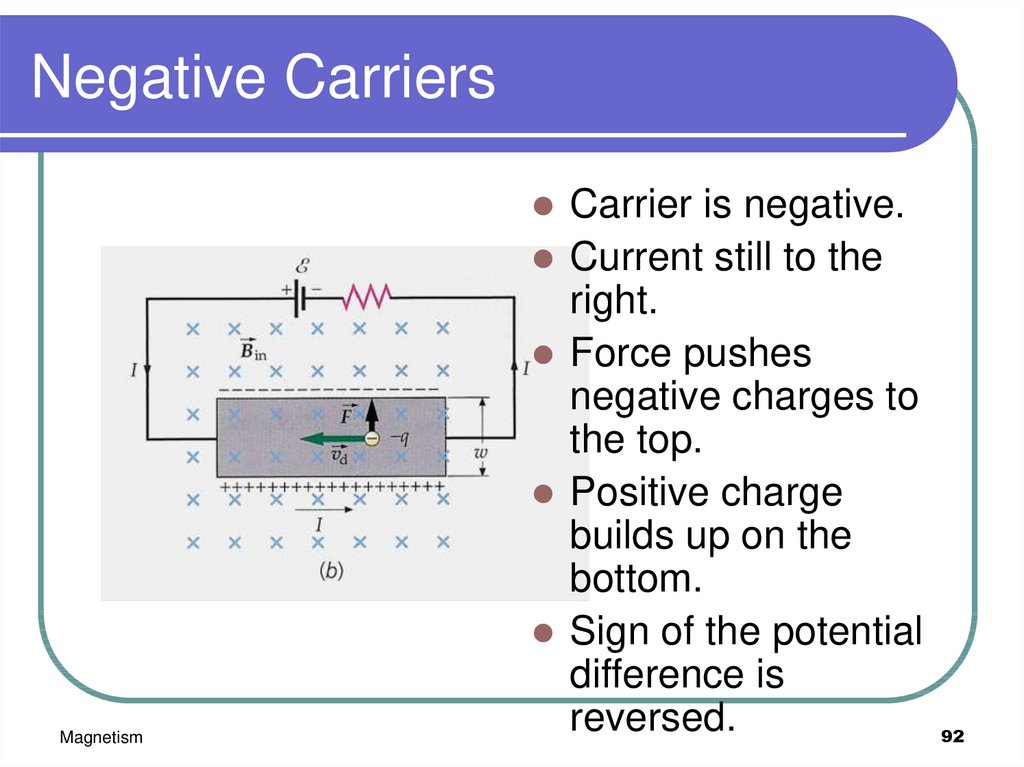
92. Negative Carriers
MagnetismCarrier is negative.
Current still to the
right.
Force pushes
negative charges to
the top.
Positive charge
builds up on the
bottom.
Sign of the potential
difference is
reversed.
92
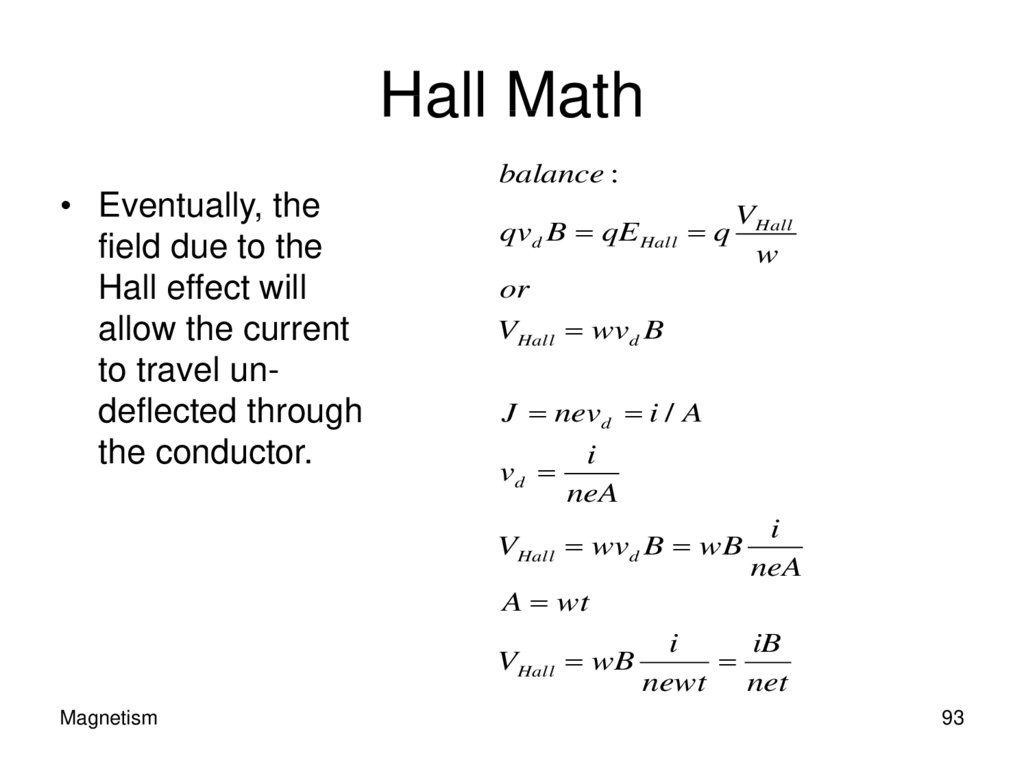
93. Hall Math
• Eventually, thefield due to the
Hall effect will
allow the current
to travel undeflected through
the conductor.
balance :
qvd B qE Hall q
VHall
w
or
VHall wvd B
J nevd i / A
vd
i
neA
VHall wvd B wB
i
neA
A wt
VHall wB
Magnetism
iB
i
newt net
93
94. Magnetic Fields Due to Currents Chapter 30
Magnetism94
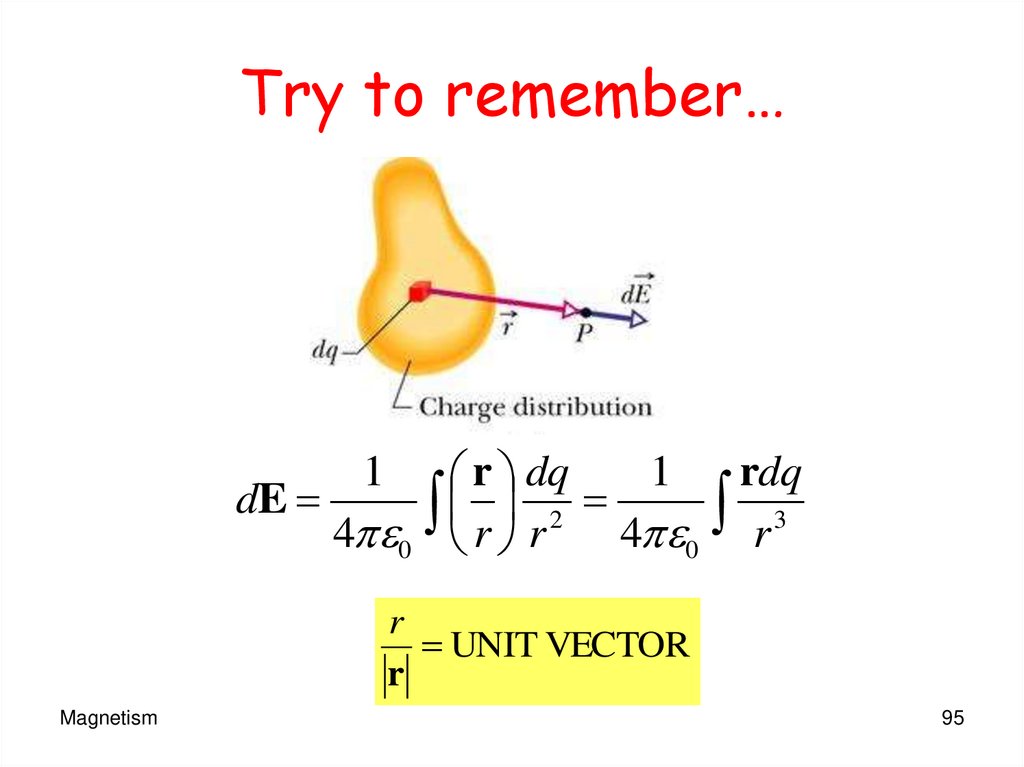
95. Try to remember…
1rdq
r dq
dE
2
3
4 0 r r
4 0 r
1
r
UNIT VECTOR
r
Magnetism
95
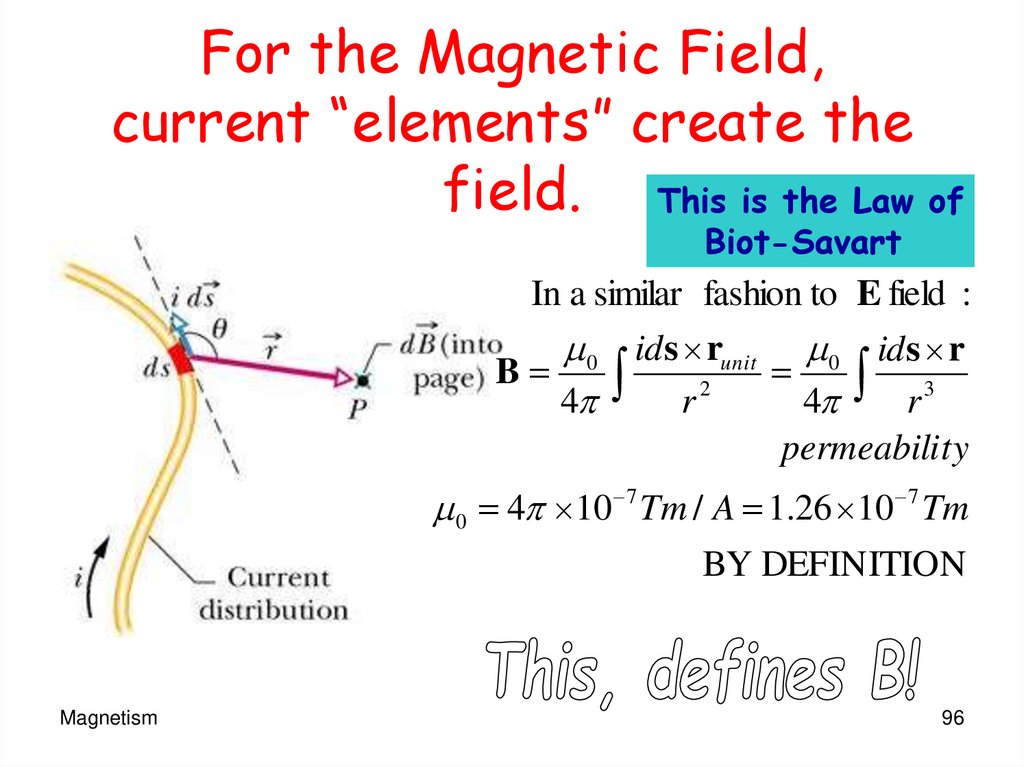
96. For the Magnetic Field, current “elements” create the field.
This is the Law ofBiot-Savart
In a similar fashion to E field :
m 0 id s runit m 0 id s r
B
2
4
r
4 r 3
permeabili ty
m 0 4 10 7 Tm / A 1.26 10 7 Tm
BY DEFINITION
Magnetism
96
97. Magnetic Field of a Straight Wire
• We intimated via magnets that theMagnetic field associated with a
straight wire seemed to vary with 1/d.
• We can now PROVE this!
Magnetism
97
98. From the Past
Using MagnetsMagnetism
98
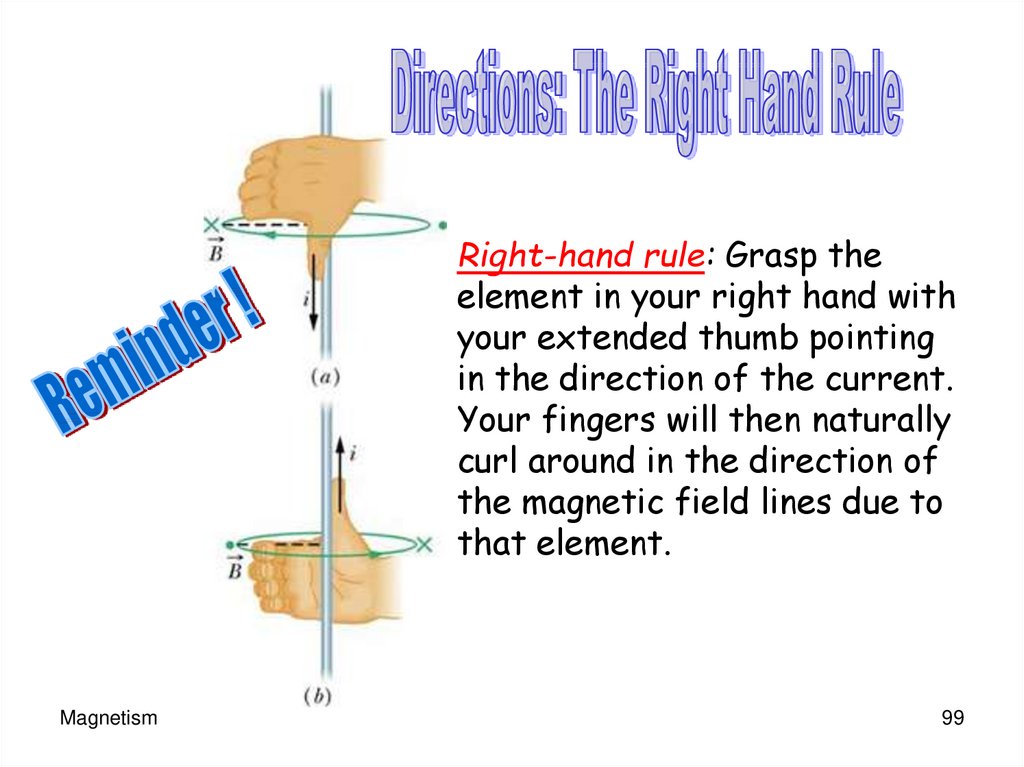
99.
Right-hand rule: Grasp theelement in your right hand with
your extended thumb pointing
in the direction of the current.
Your fingers will then naturally
curl around in the direction of
the magnetic field lines due to
that element.
Magnetism
99
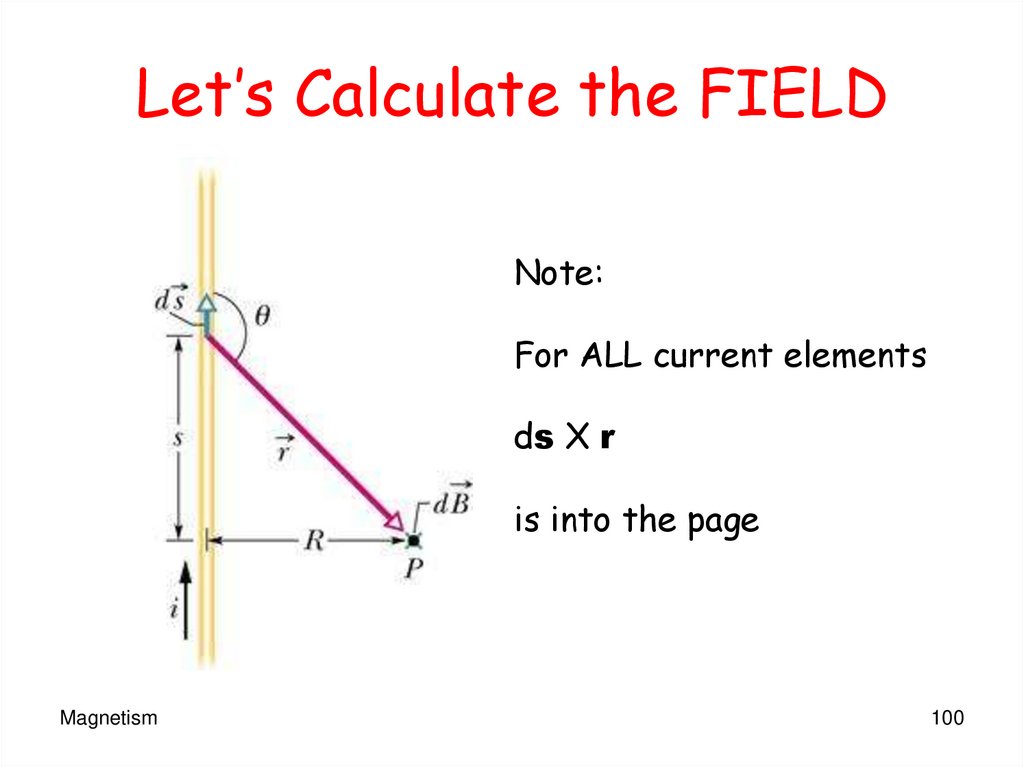
100. Let’s Calculate the FIELD
Note:For ALL current elements
ds X r
is into the page
Magnetism
100
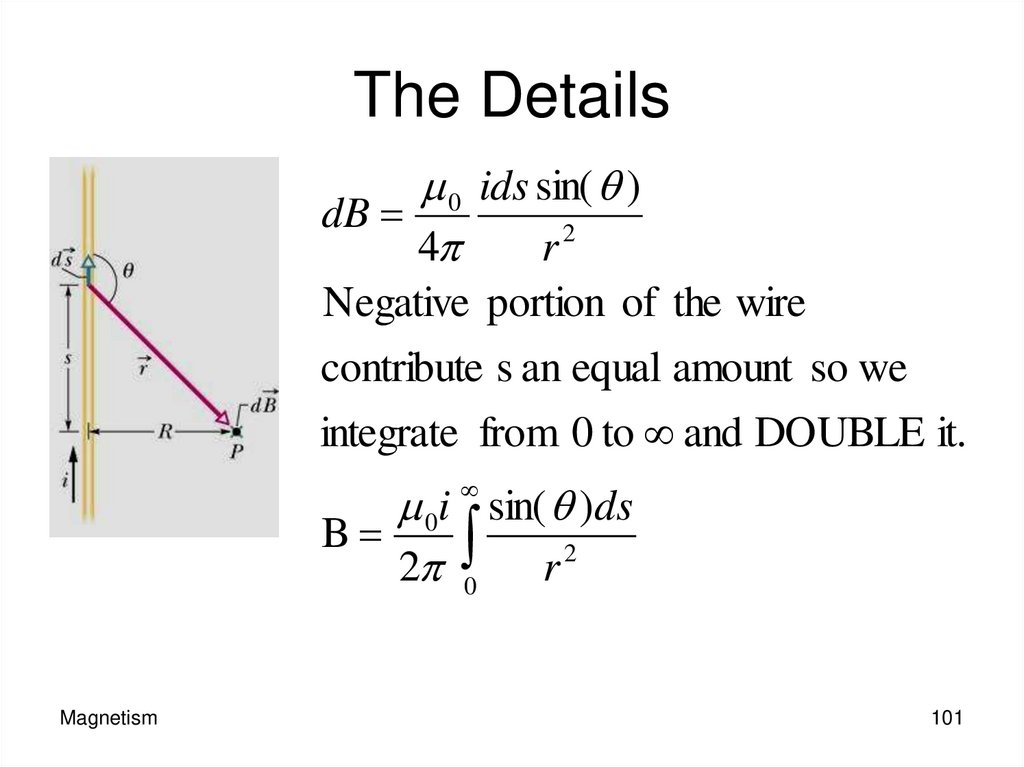
101. The Details
m 0 ids sin( )dB
4
r2
Negative portion of the wire
contribute s an equal amount so we
integrate from 0 to and DOUBLE it.
m 0i sin( )ds
B
2
2 0
r
Magnetism
101
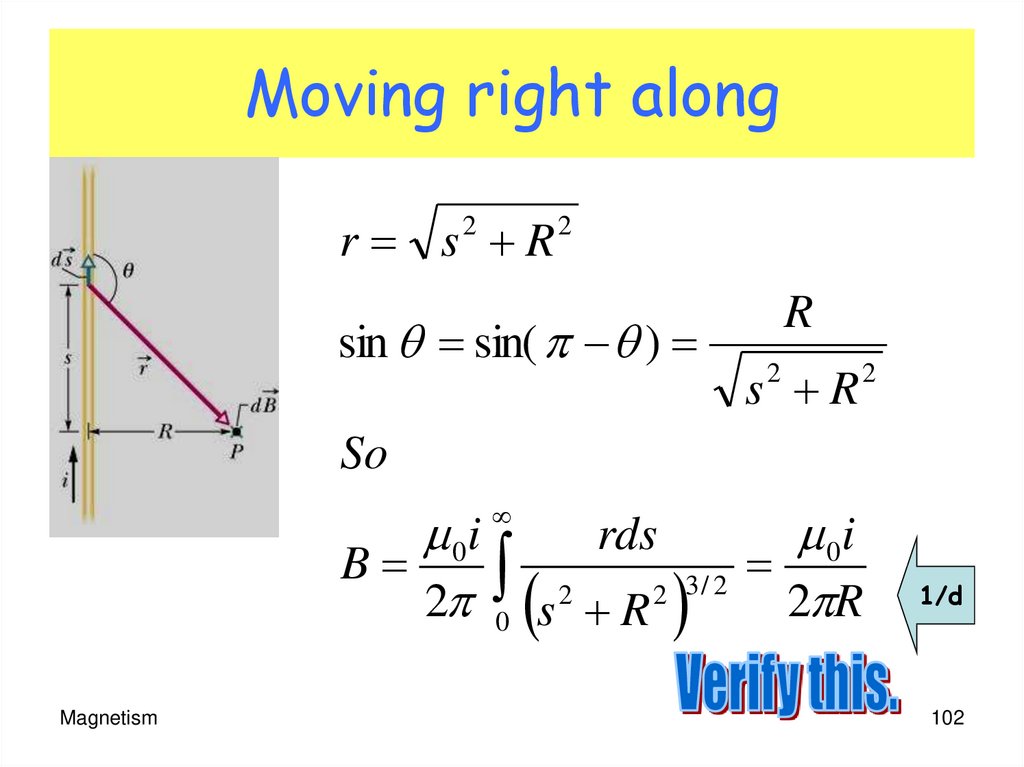
102. Moving right along
r s R2
2
sin sin( )
R
s2 R2
So
m 0i
m 0i
rds
B
3
/
2
2 0 s 2 R 2
2 R
Magnetism
1/d
102
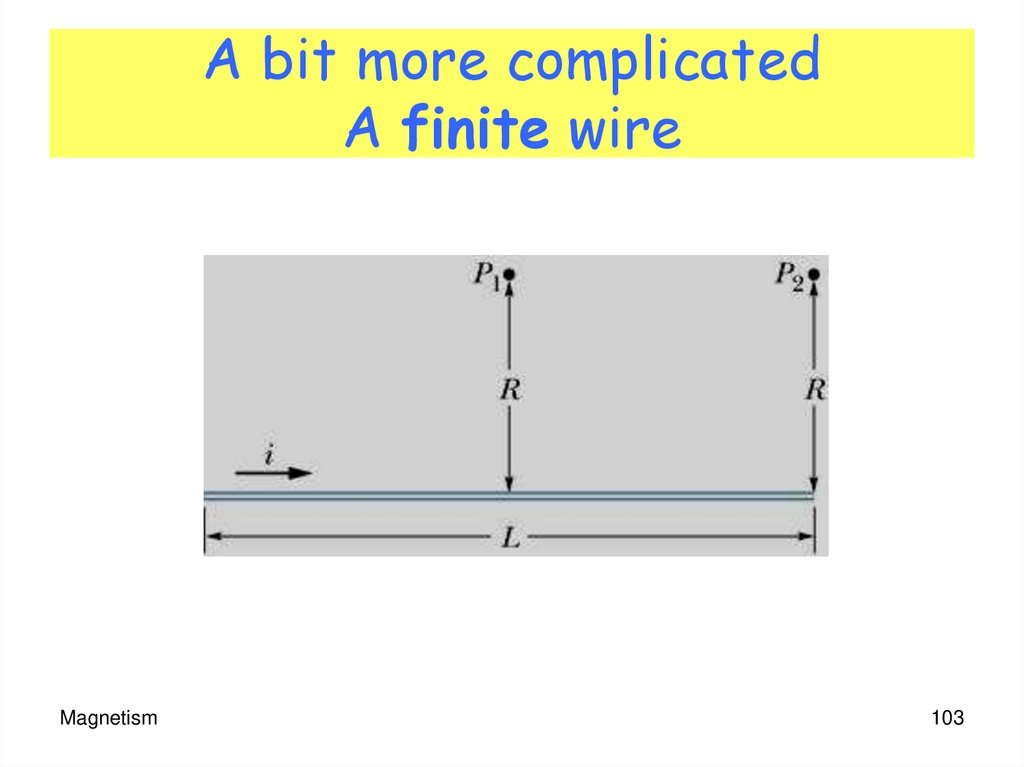
103. A bit more complicated A finite wire
Magnetism103
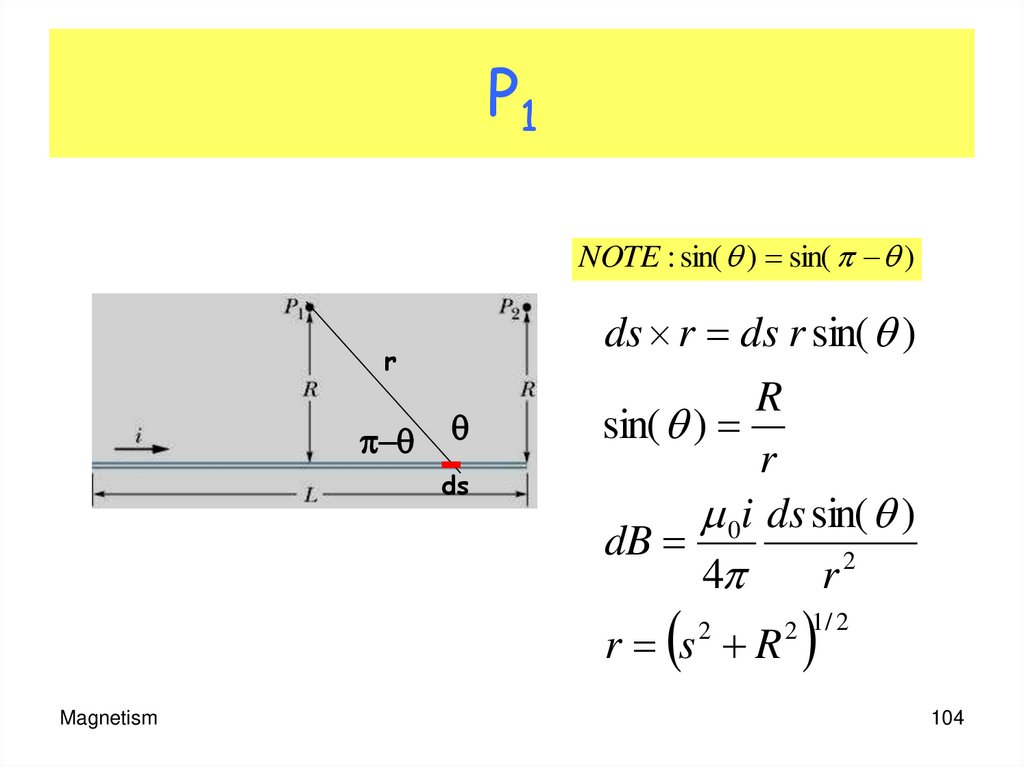
104. P1
NOTE : sin( ) sin( )ds r ds r sin( )
r
ds
R
sin( )
r
m 0i ds sin( )
dB
2
4
r
r s R
Magnetism
2
2 1/ 2
104
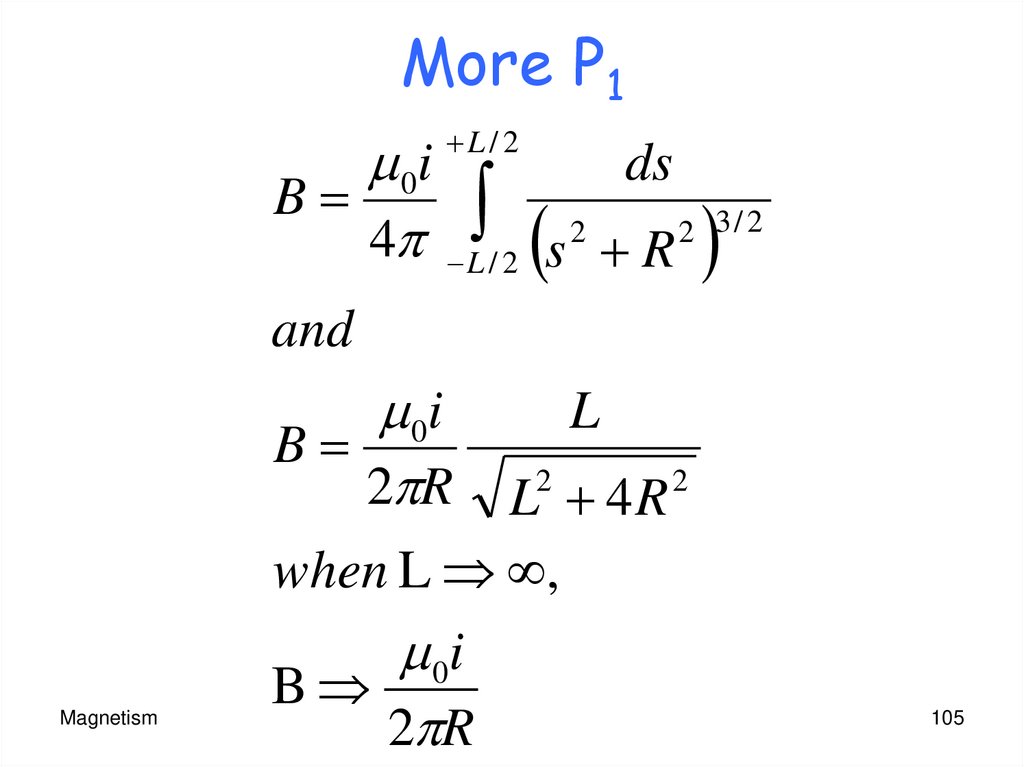
105. More P1
L/2m 0i
ds
B
3/ 2
2
2
4 L / 2 s R
and
m 0i
L
B
2 R L2 4 R 2
Magnetism
when L ,
m 0i
B
2 R
105
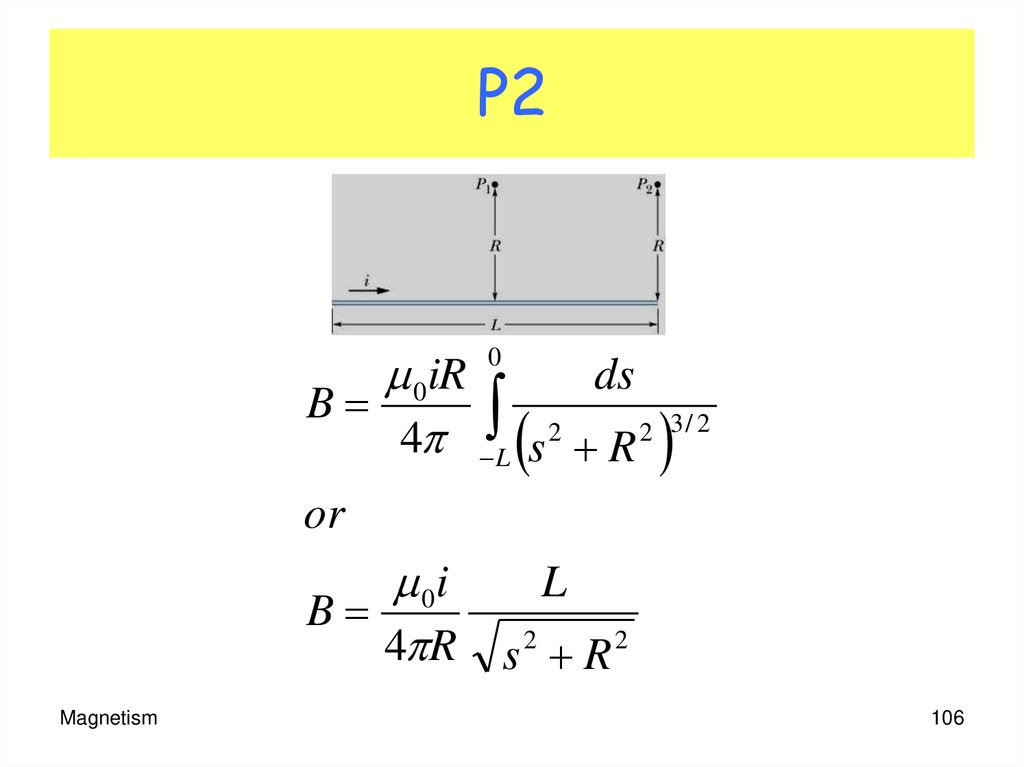
106. P2
m 0iRds
B
3/ 2
2
2
4 L s R
0
or
m 0i
L
B
4 R s 2 R 2
Magnetism
106
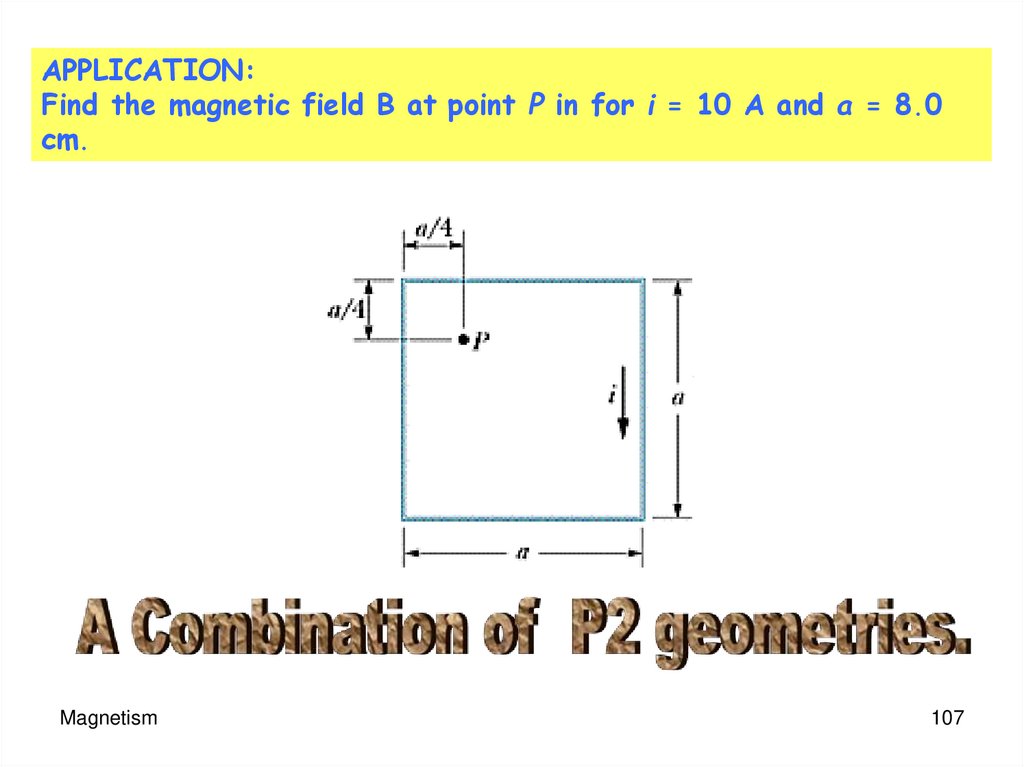
107.
APPLICATION:Find the magnetic field B at point P in for i = 10 A and a = 8.0
cm.
Magnetism
107
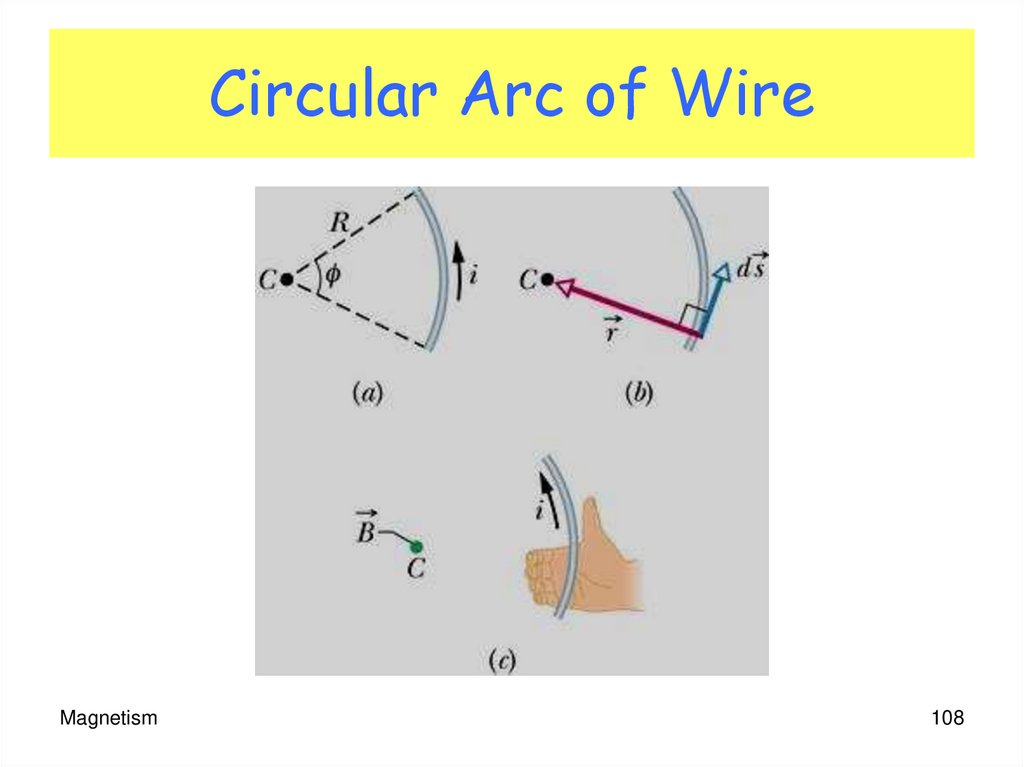
108. Circular Arc of Wire
Magnetism108
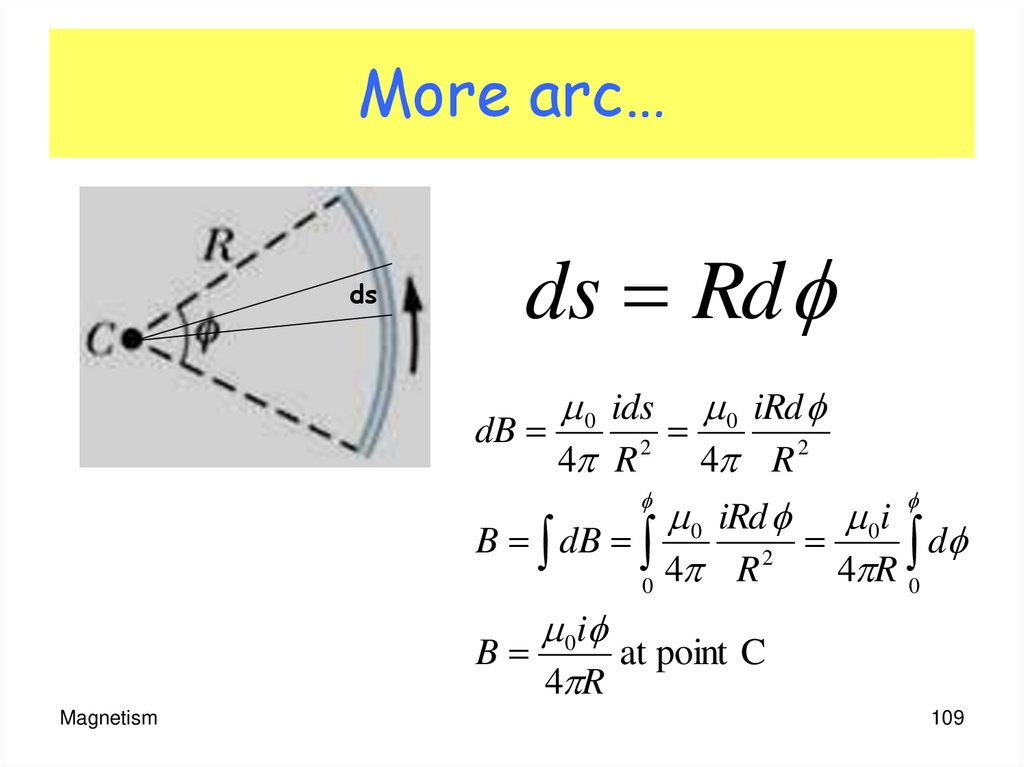
109. More arc…
dsds Rd
m 0 ids m 0 iRd
dB
2
4 R
4 R 2
m 0 iRd m 0i
B dB
d
2
4 R
4 R 0
0
m 0 i
B
at point C
4 R
Magnetism
109
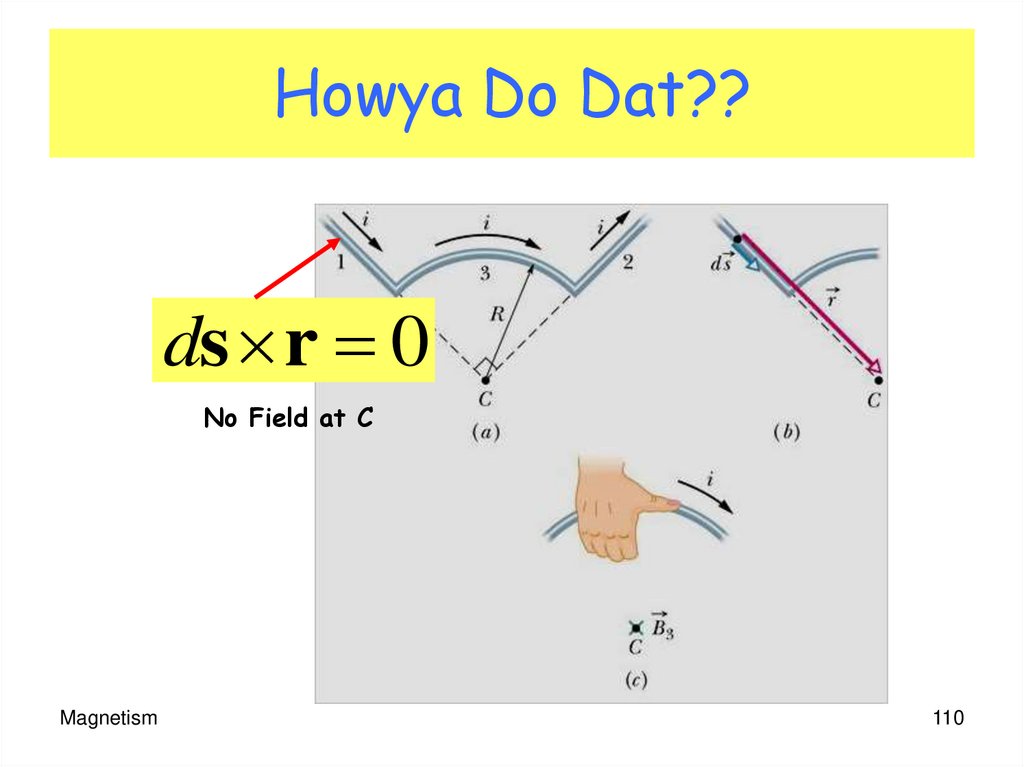
110. Howya Do Dat??
ds r 0No Field at C
Magnetism
110
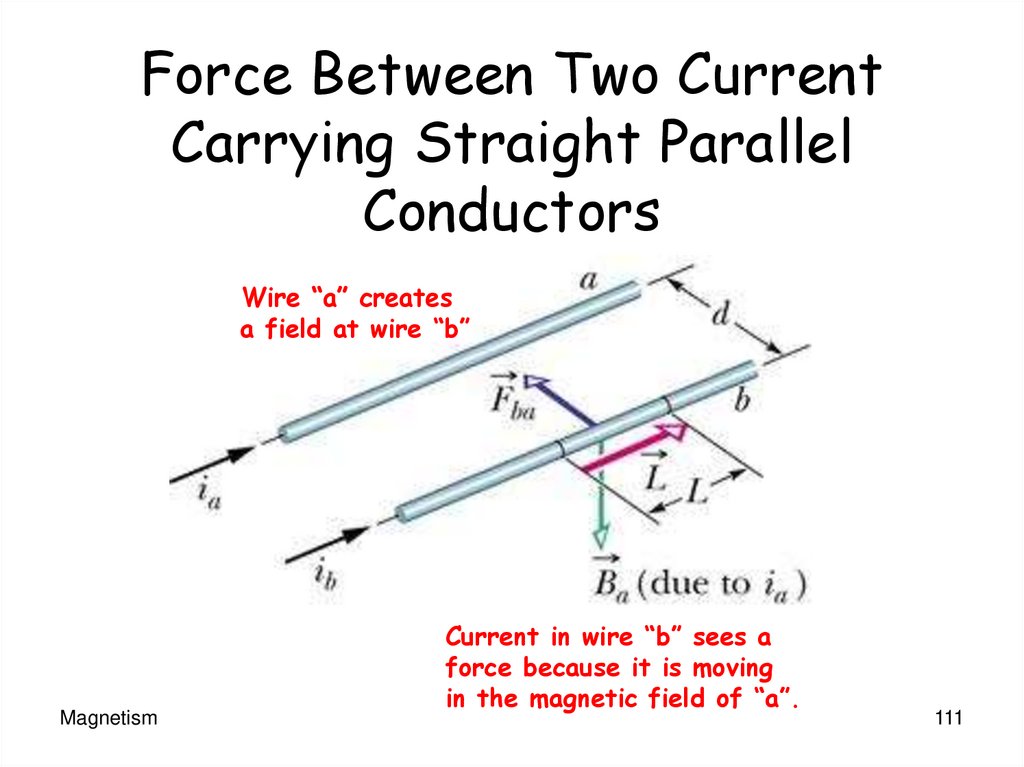
111. Force Between Two Current Carrying Straight Parallel Conductors
Wire “a” createsa field at wire “b”
Magnetism
Current in wire “b” sees a
force because it is moving
in the magnetic field of “a”.
111
112. The Calculation
The FIELD at wire " b" due towire " a" is what we just calculated :
m 0ia
Bat "b"
2 d
Fon "b" ib L B
Since L and B are at right angles...
m 0 Lia ib
F
2 d
Magnetism
112
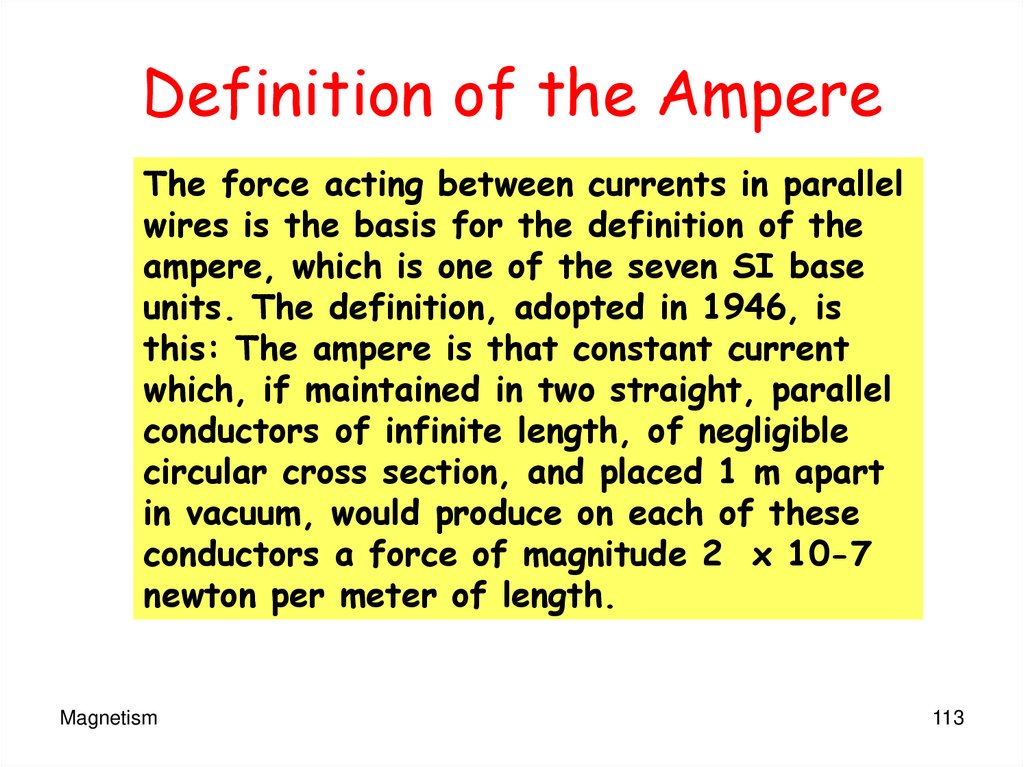
113. Definition of the Ampere
The force acting between currents in parallelwires is the basis for the definition of the
ampere, which is one of the seven SI base
units. The definition, adopted in 1946, is
this: The ampere is that constant current
which, if maintained in two straight, parallel
conductors of infinite length, of negligible
circular cross section, and placed 1 m apart
in vacuum, would produce on each of these
conductors a force of magnitude 2 x 10-7
newton per meter of length.
Magnetism
113
114. TRANSITION
AMPEREMagnetism
114
115. Welcome to Andre’ Marie Ampere’s Law
Normally written as a “circulation” vectorequation.
We will look at another form, but first…
Magnetism
115
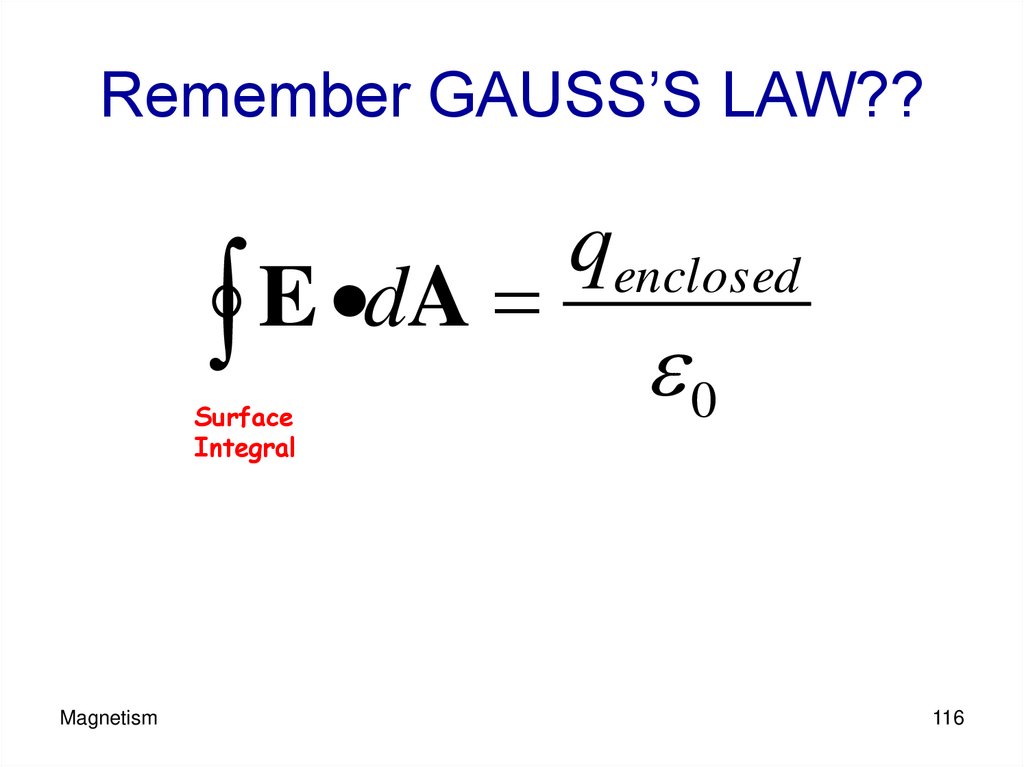
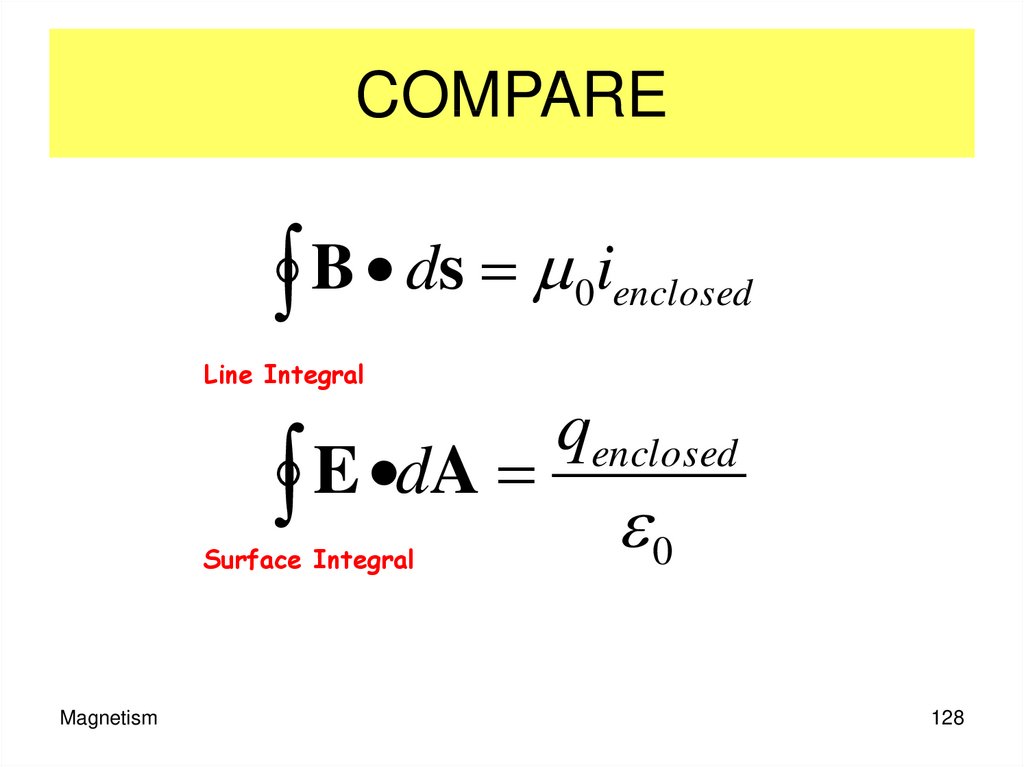
116. Remember GAUSS’S LAW??
Ed
A
Surface
Integral
Magnetism
qenclosed
0
116
117. Gauss’s Law
• Made calculations easier thanintegration over a charge distribution.
• Applied to situations of HIGH
SYMMETRY.
• Gaussian SURFACE had to be defined
which was consistent with the geometry.
• AMPERE’S Law is the Gauss’ Law of
Magnetism! (Sorry)
Magnetism
117
118.
The next few slides have beenlifted from Seb Oliver
on the internet
Whoever he is!
Magnetism
118
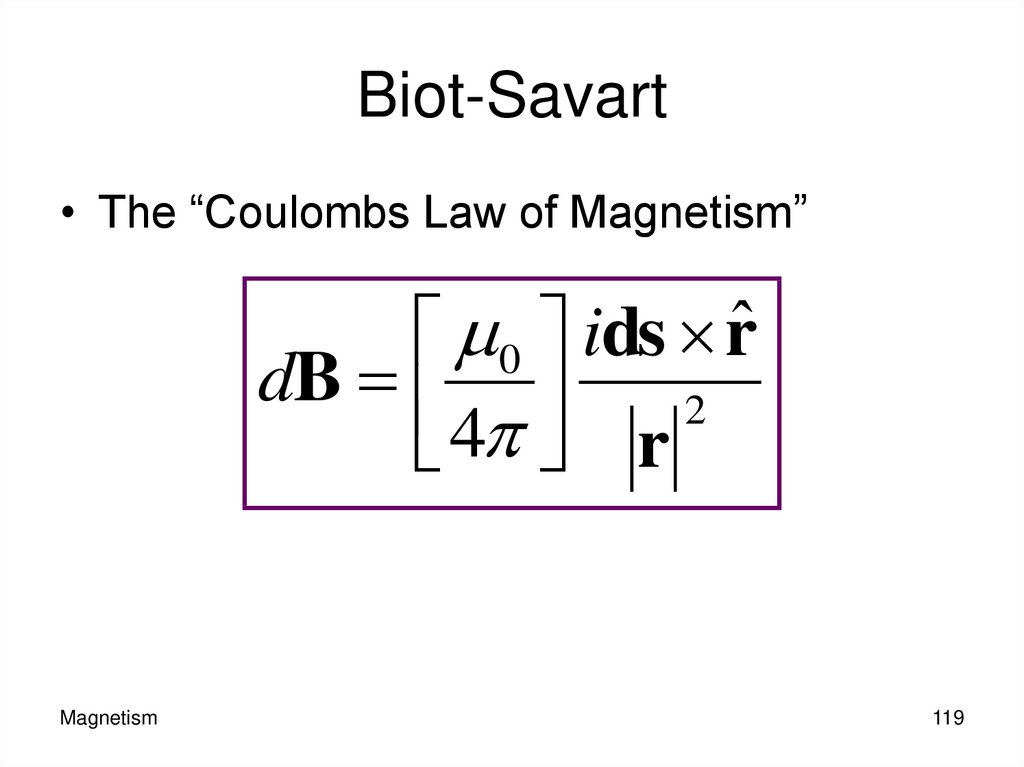
119. Biot-Savart
• The “Coulombs Law of Magnetism”m0 ids rˆ
dB
2
4 r
Magnetism
119
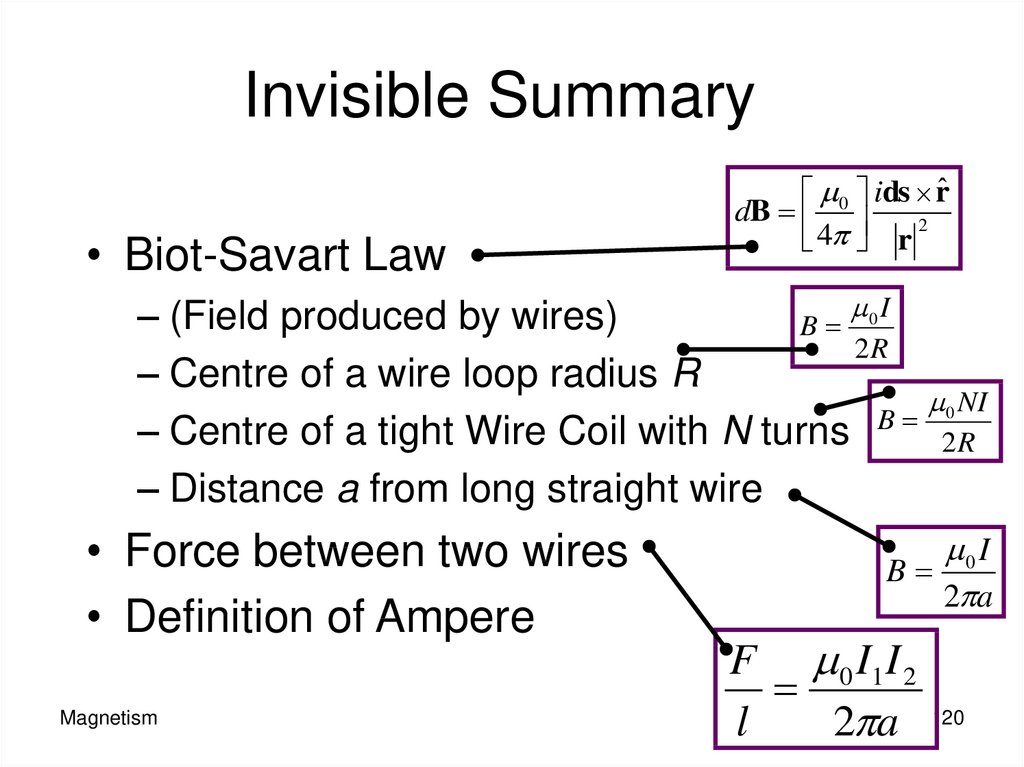
120. Invisible Summary
• Biot-Savart Lawm0 ids rˆ
dB
2
4 r
m0 I
– (Field produced by wires)
B
2R
– Centre of a wire loop radius R
m NI
B 0
– Centre of a tight Wire Coil with N turns
2R
– Distance a from long straight wire
• Force between two wires
• Definition of Ampere
Magnetism
m0 I
B
2 a
F m 0 I1 I 2
l
2 a
120
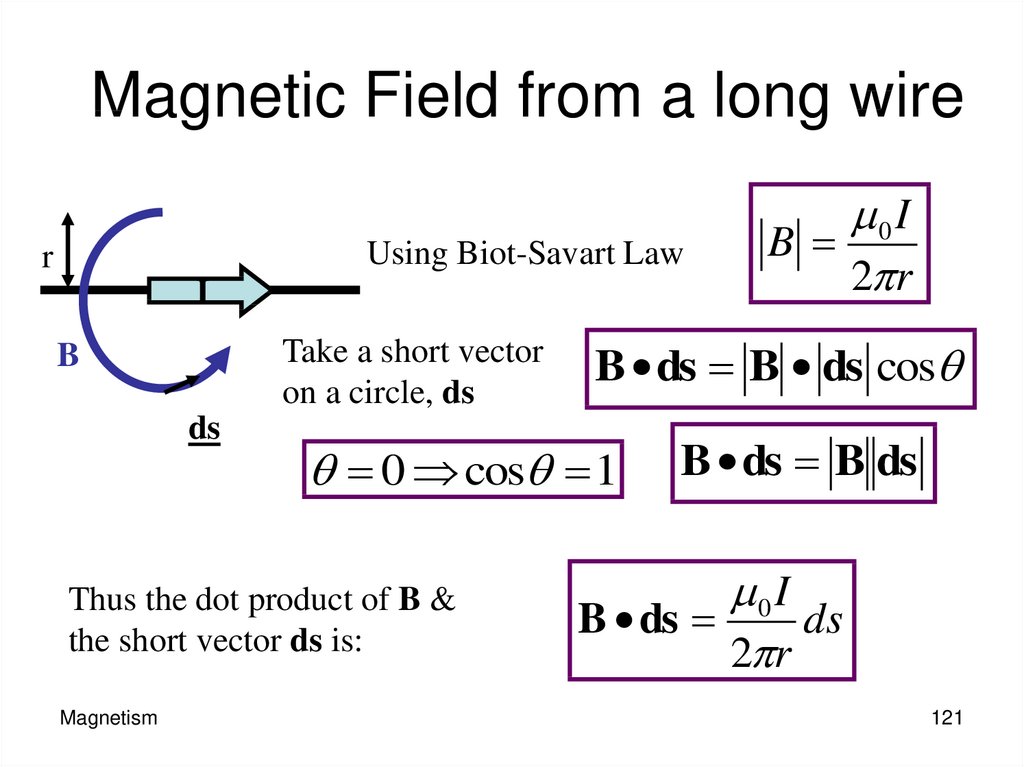
121. Magnetic Field from a long wire
Using Biot-Savart Lawr
I
Take a short vector
on a circle, ds
B
ds
Magnetism
B ds B ds cos
0 cos 1
Thus the dot product of B &
the short vector ds is:
m0 I
B
2 r
B ds B ds
m0 I
B ds
ds
2 r
121
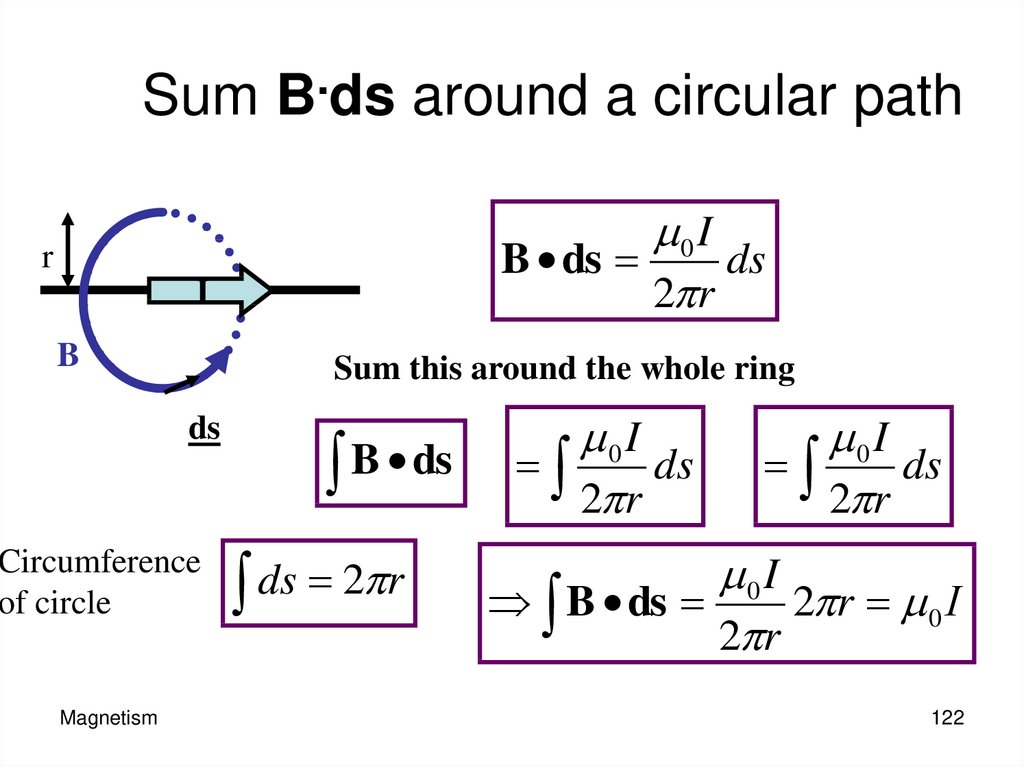
122. Sum B.ds around a circular path
Sum.
B ds
around a circular path
m0 I
B ds
ds
2 r
r
I
B
Sum this around the whole ring
ds
Circumference
of circle
Magnetism
B ds
ds 2 r
m0 I
ds
2 r
m0 I
ds
2 r
m0 I
B ds
2 r m 0 Ι
2 r
122
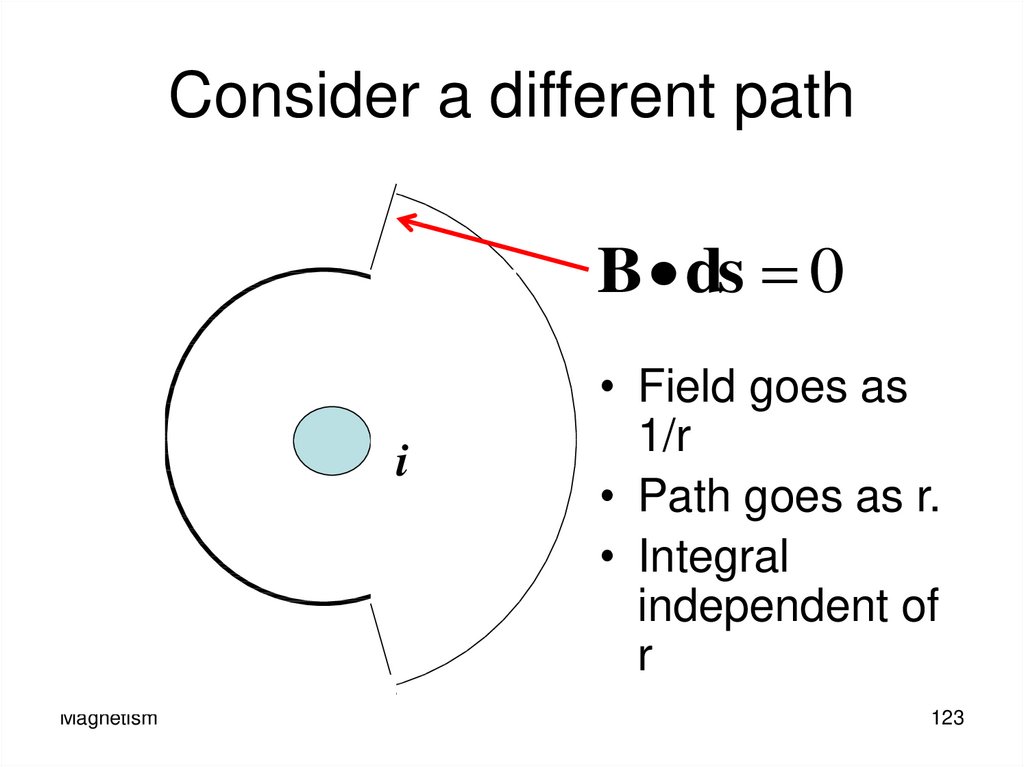
123. Consider a different path
B ds 0i
Magnetism
• Field goes as
1/r
• Path goes as r.
• Integral
independent of
r
123
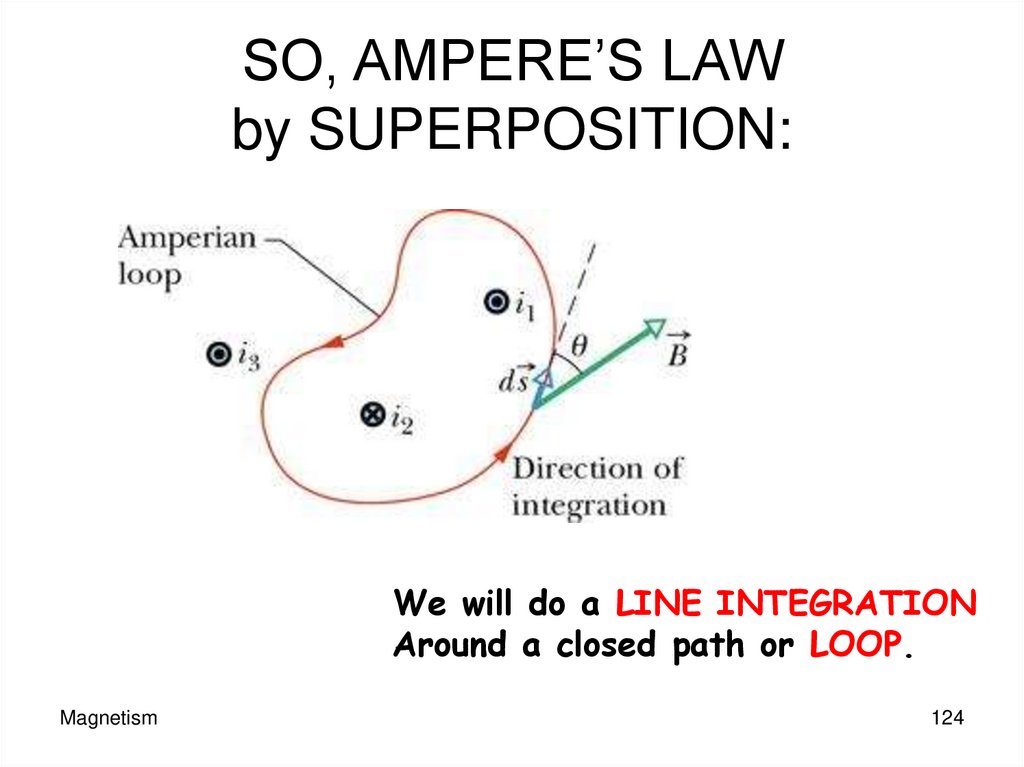
124. SO, AMPERE’S LAW by SUPERPOSITION:
We will do a LINE INTEGRATIONAround a closed path or LOOP.
Magnetism
124
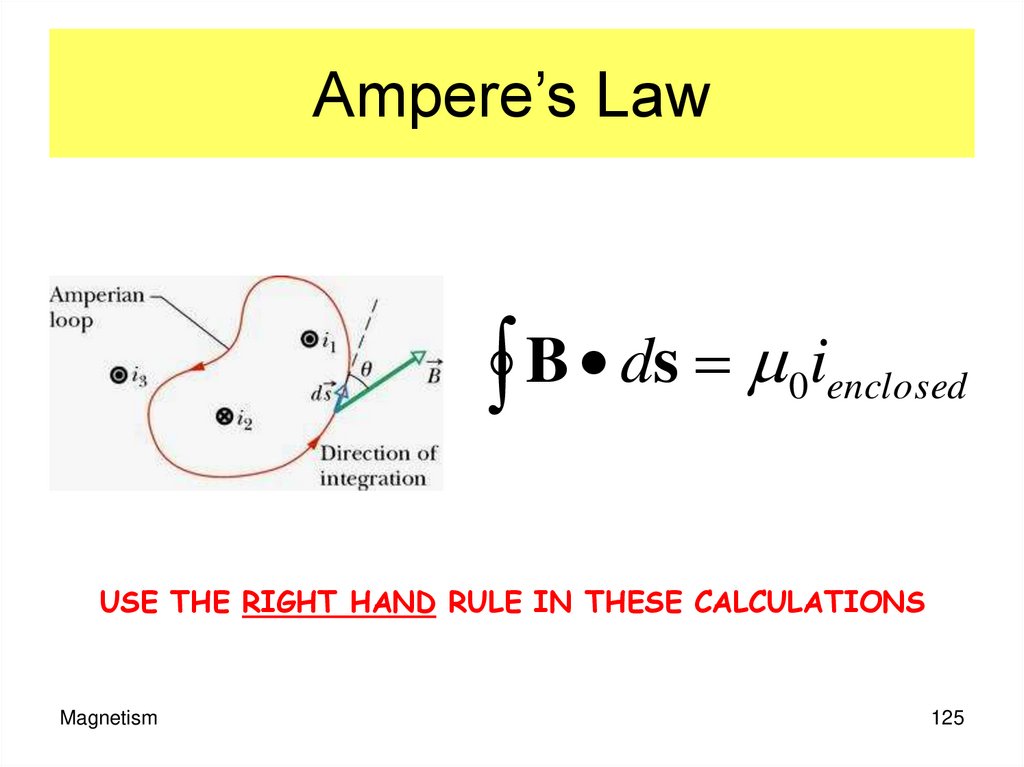
125. Ampere’s Law
Bd
s
m
i
0
enclosed
USE THE RIGHT HAND RULE IN THESE CALCULATIONS
Magnetism
125
126. The Right Hand Rule
Magnetism126
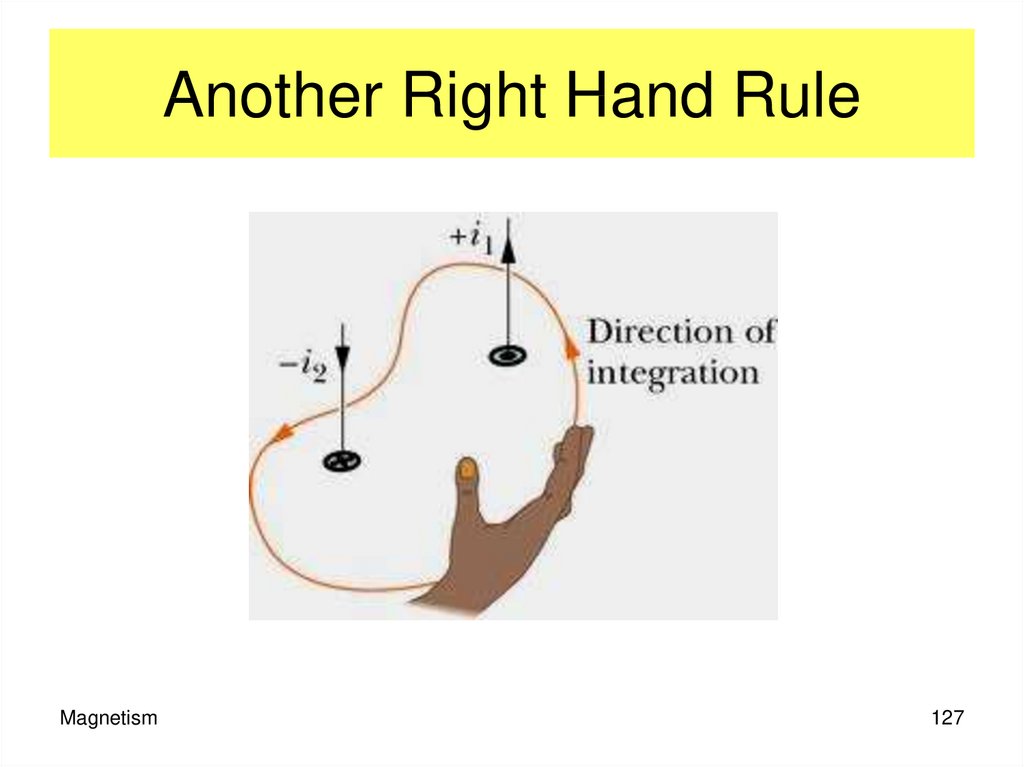
127. Another Right Hand Rule
Magnetism127
128. COMPARE
Bd
s
m
i
0
enclosed
Line Integral
E
d
A
Surface Integral
Magnetism
qenclosed
0
128
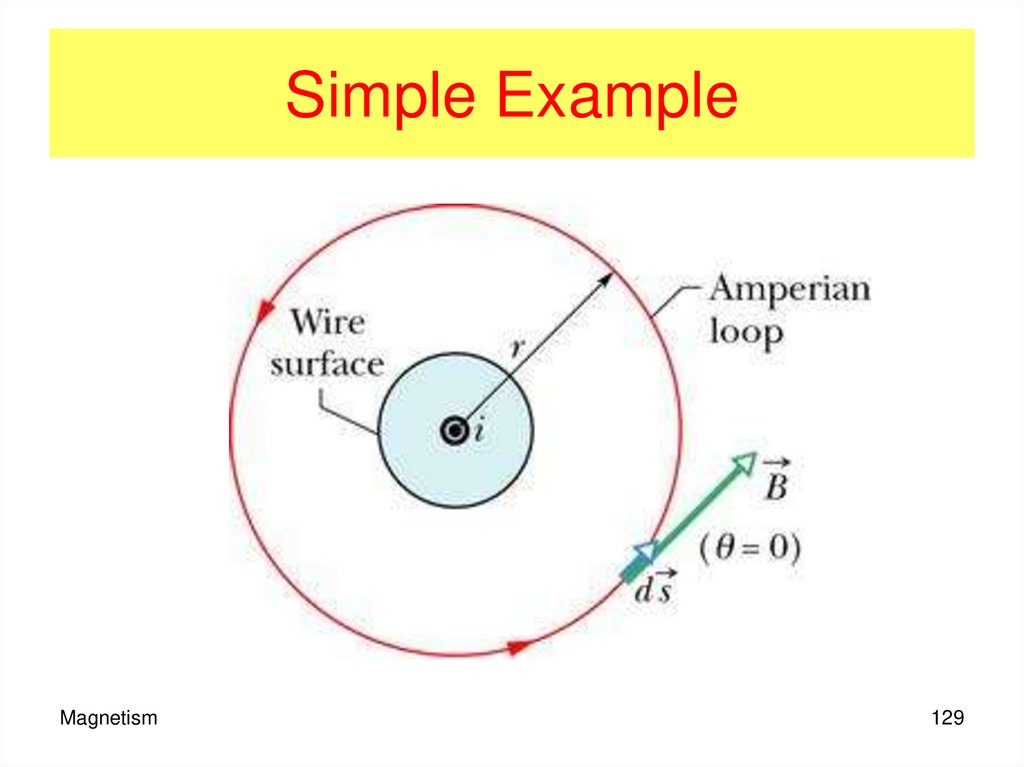
129. Simple Example
Magnetism129
130. Field Around a Long Straight Wire
Bd
s
m
i
0
enclosed
B 2 r m 0i
m 0i
B
2 r
Magnetism
130
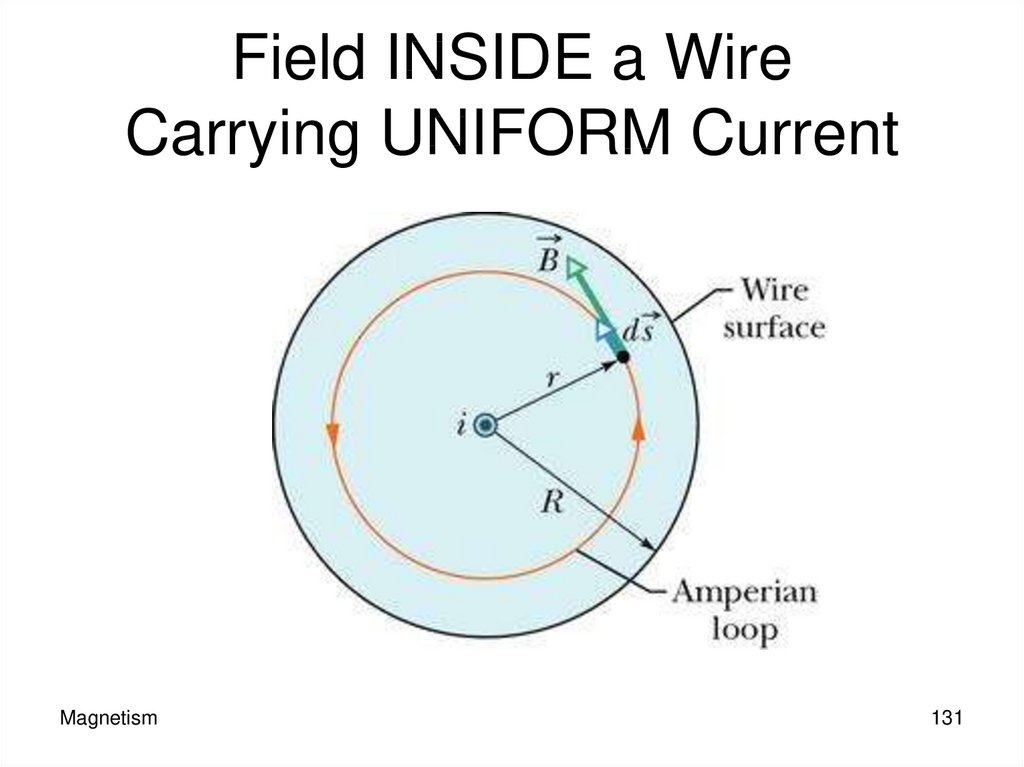
131. Field INSIDE a Wire Carrying UNIFORM Current
Magnetism131
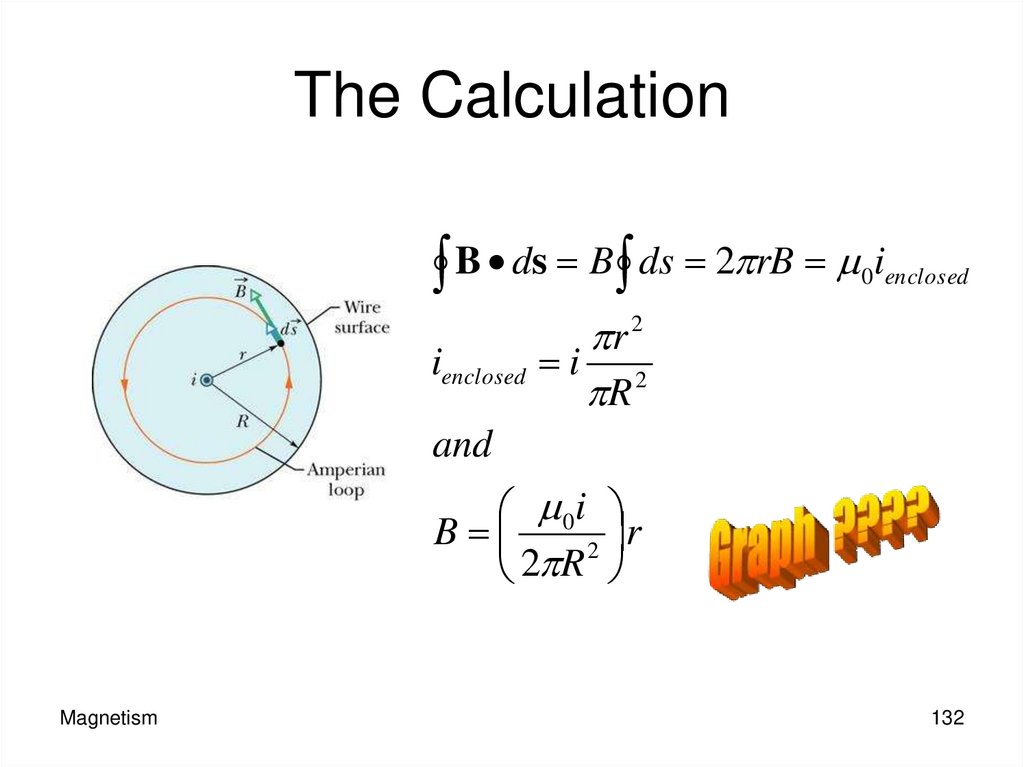
132. The Calculation
B ds B ds 2 rB m i0 enclosed
ienclosed
r 2
i 2
R
and
m 0i
B
r
2
2 R
Magnetism
132
133.
Bm 0i
2 R
R
Magnetism
r
133
134. Procedure
• Apply Ampere’s law only to highly symmetricalsituations.
• Superposition works.
– Two wires can be treated separately and the
results added (VECTORIALLY!)
• The individual parts of the calculation can be
handled (usually) without the use of vector
calculations because of the symmetry.
• THIS IS SORT OF LIKE GAUSS’s LAW
WITH AN ATTITUDE!
Magnetism
134
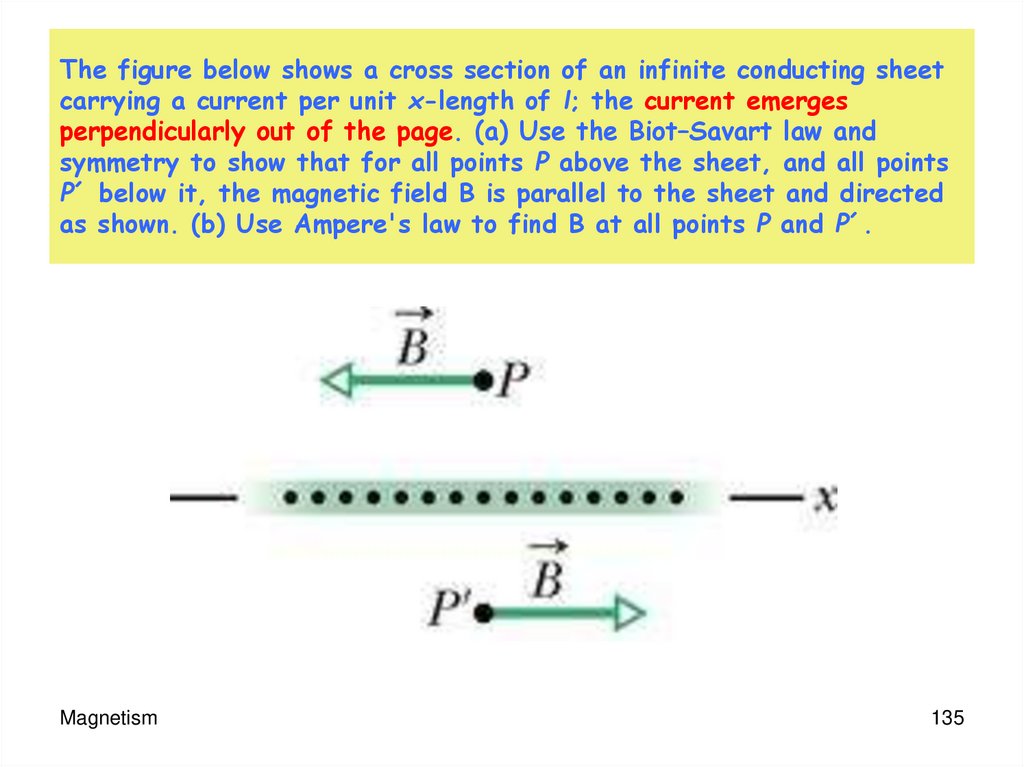
135. The figure below shows a cross section of an infinite conducting sheet carrying a current per unit x-length of l; the current
emergesperpendicularly out of the page. (a) Use the Biot–Savart law and
symmetry to show that for all points P above the sheet, and all points
P´ below it, the magnetic field B is parallel to the sheet and directed
as shown. (b) Use Ampere's law to find B at all points P and P´.
Magnetism
135
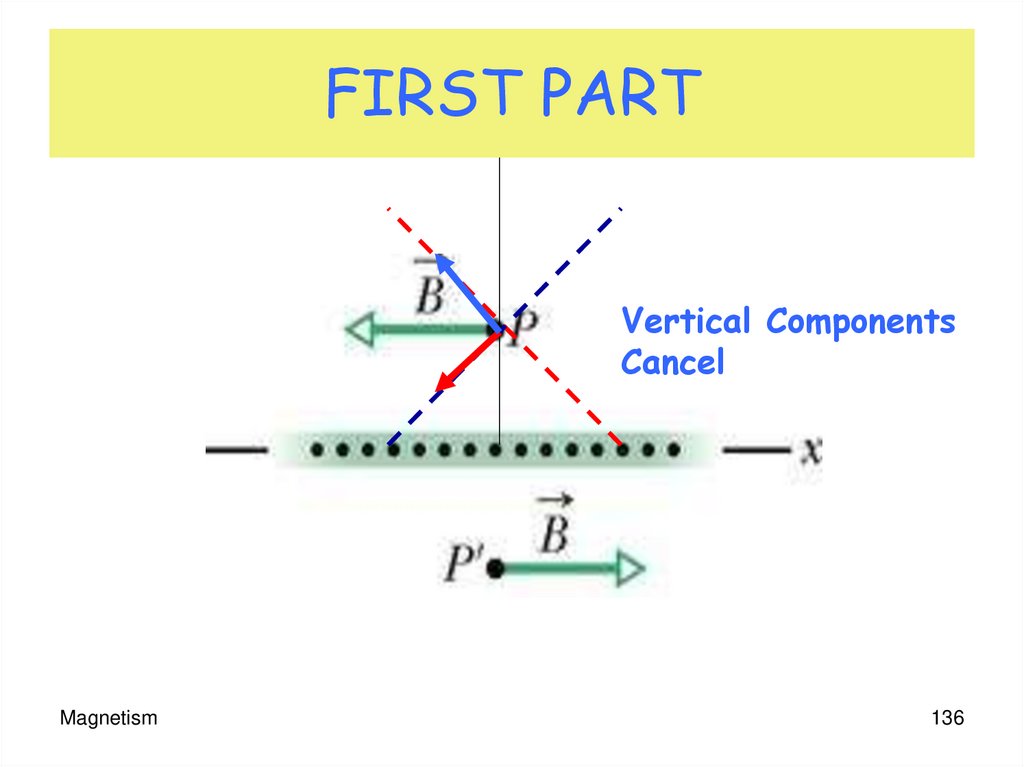
136. FIRST PART
Vertical ComponentsCancel
Magnetism
136
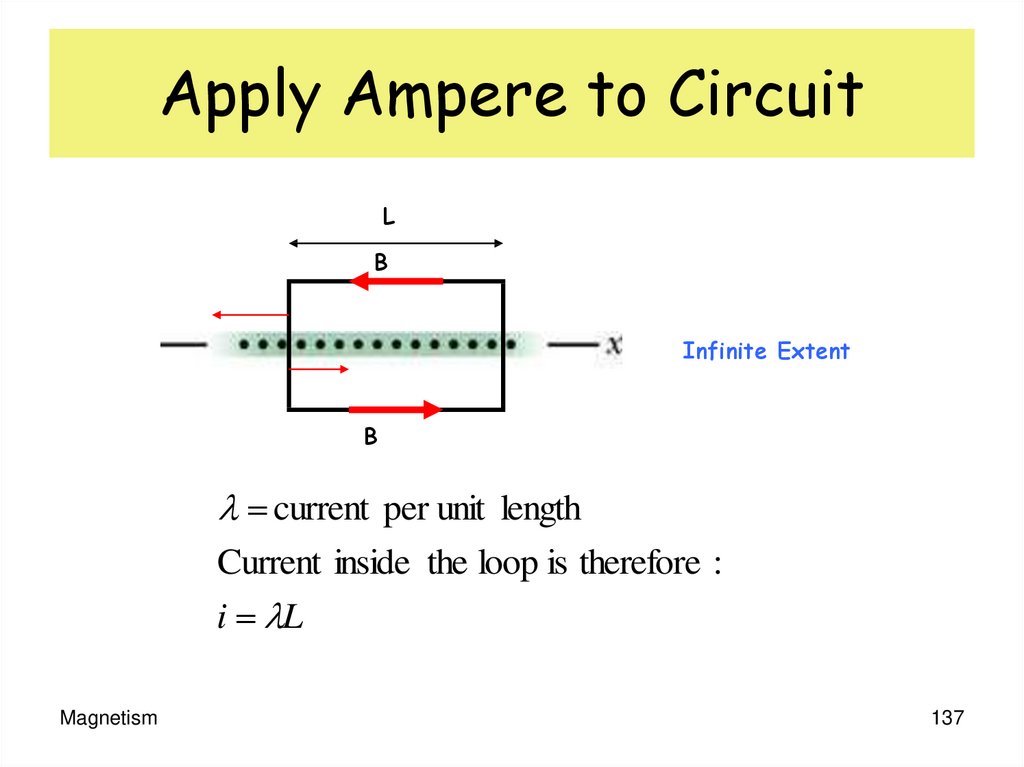
137. Apply Ampere to Circuit
LB
Infinite Extent
B
current per unit length
Current inside the loop is therefore :
i L
Magnetism
137
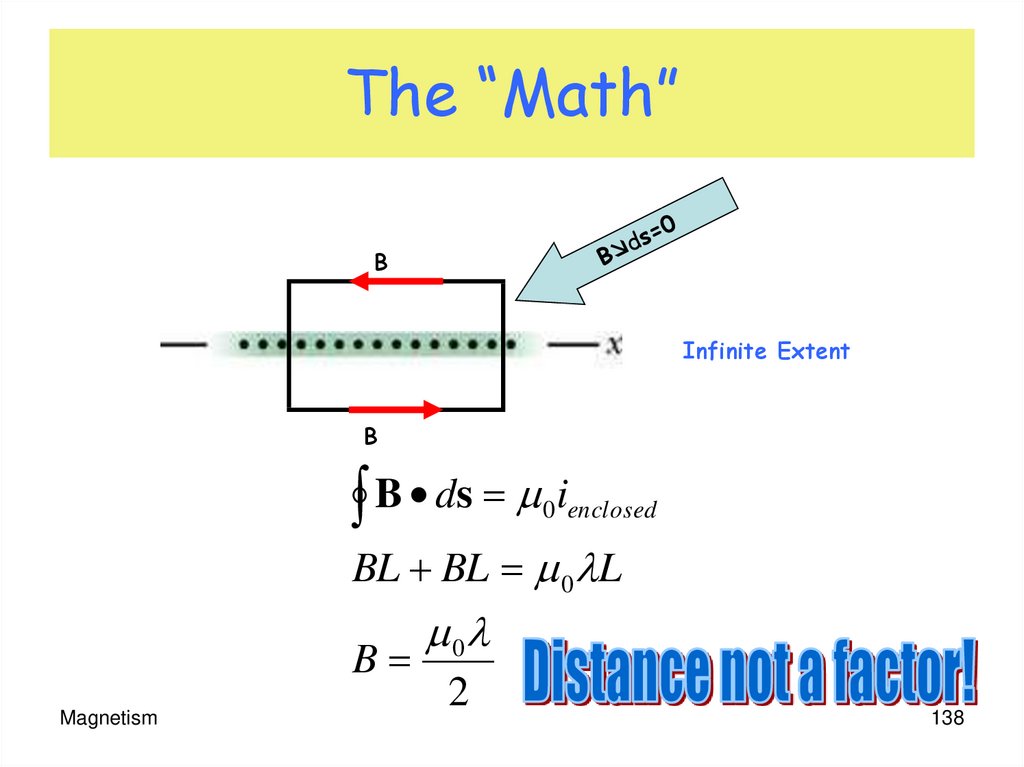
138. The “Math”
BInfinite Extent
B
B ds m i
0 enclosed
BL BL m 0 L
B
Magnetism
m 0
2
138
139. A Physical Solenoid
Magnetism139
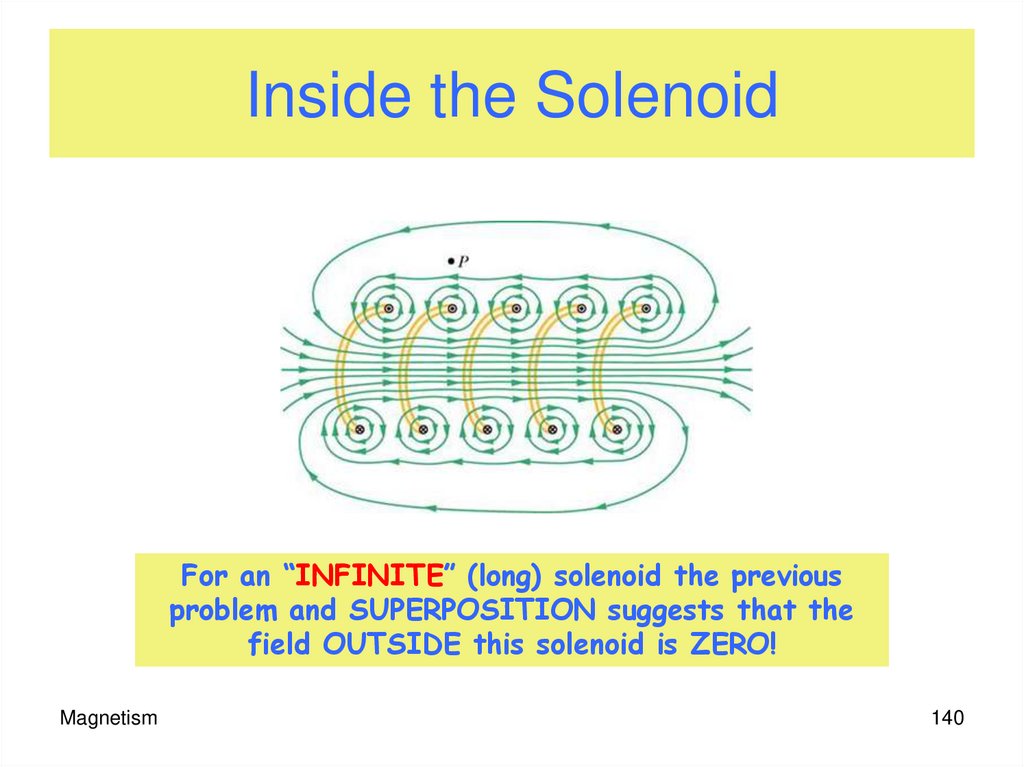
140. Inside the Solenoid
For an “INFINITE” (long) solenoid the previousproblem and SUPERPOSITION suggests that the
field OUTSIDE this solenoid is ZERO!
Magnetism
140
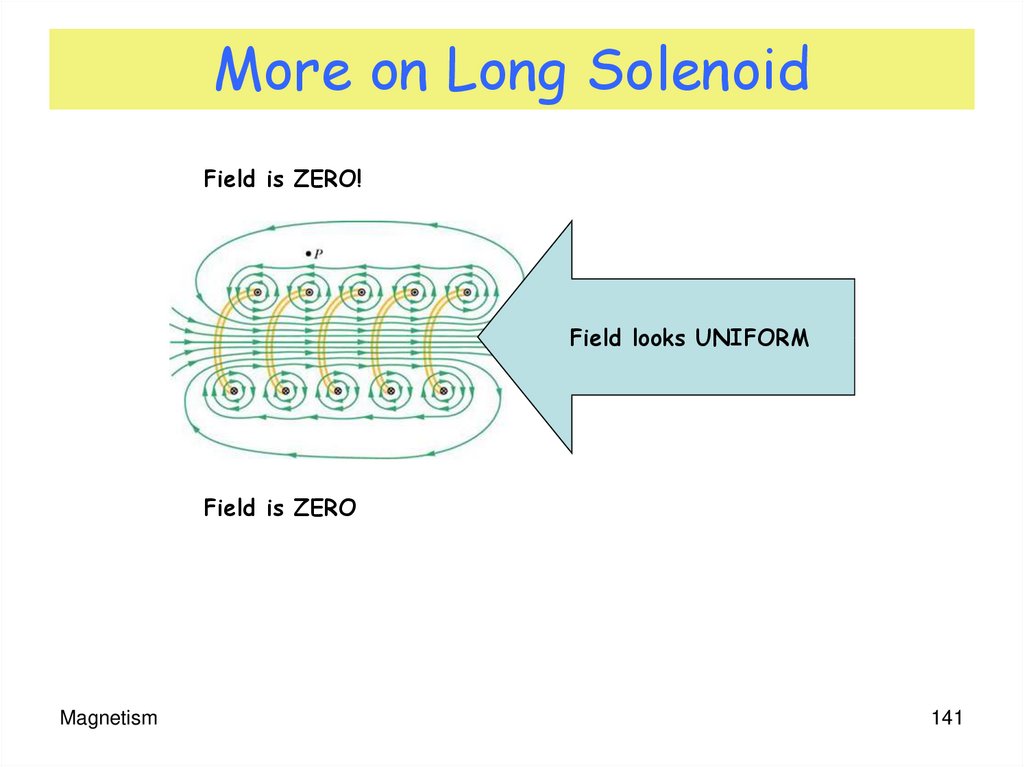
141. More on Long Solenoid
Field is ZERO!Field looks UNIFORM
Field is ZERO
Magnetism
141
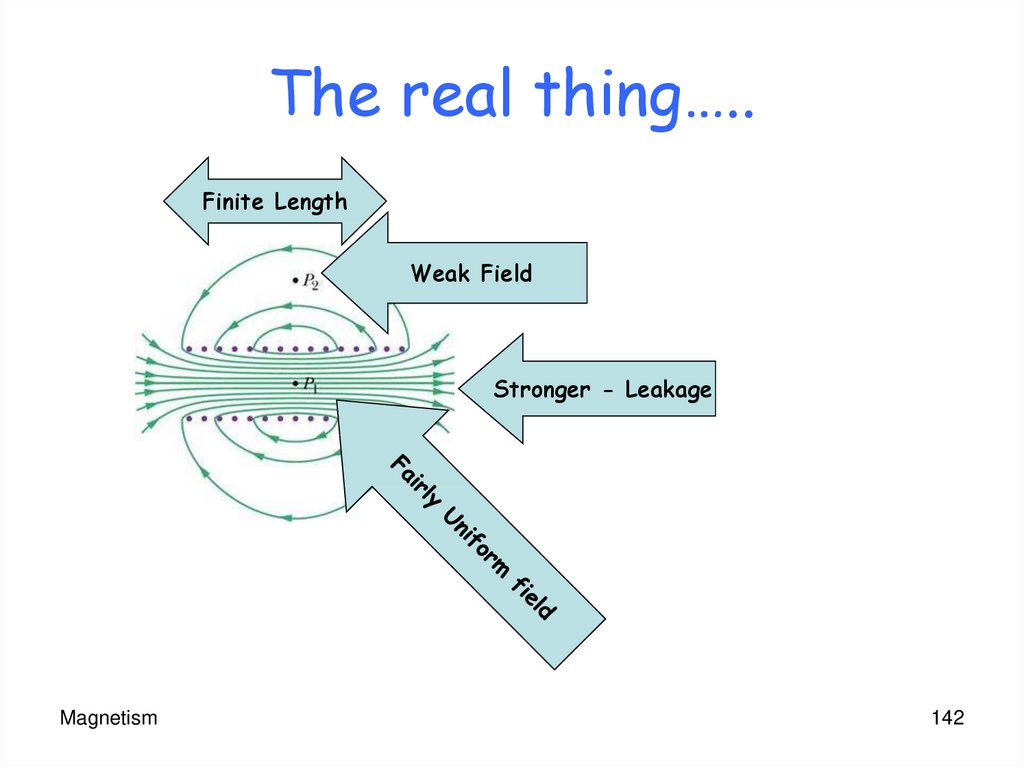
142. The real thing…..
Finite LengthWeak Field
Stronger - Leakage
Magnetism
142
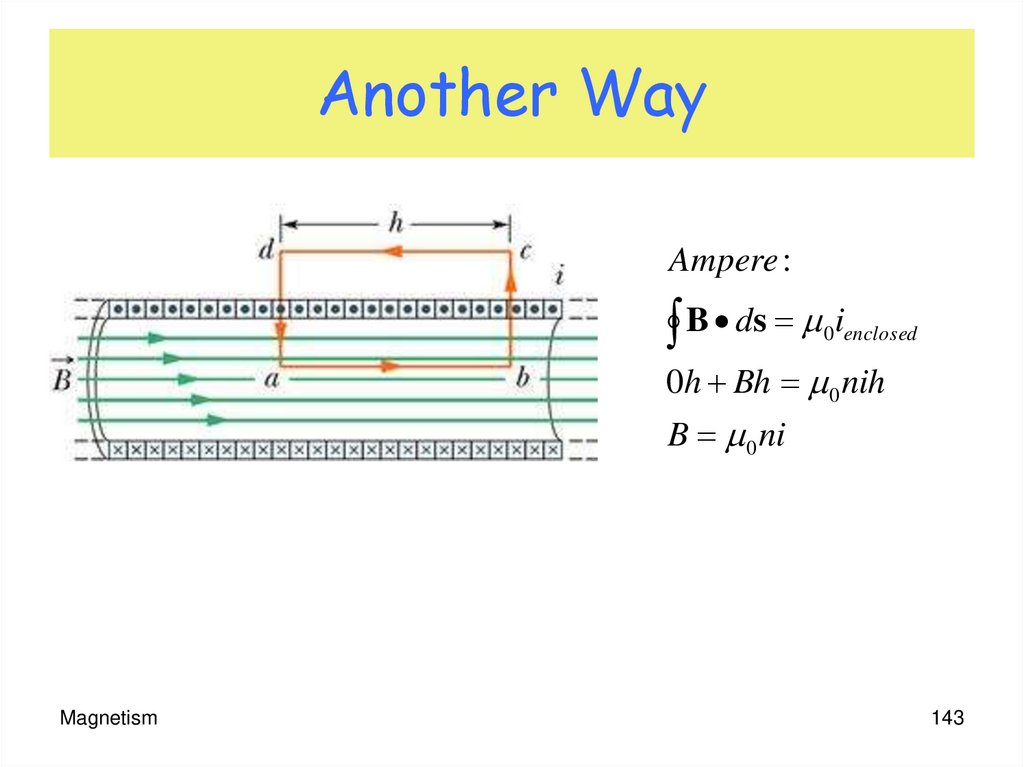
143. Another Way
Ampere :B ds m i
0 enclosed
0h Bh m 0 nih
B m 0 ni
Magnetism
143
144. Application
• Creation of Uniform Magnetic FieldRegion
• Minimal field outside
– except at the ends!
Magnetism
144
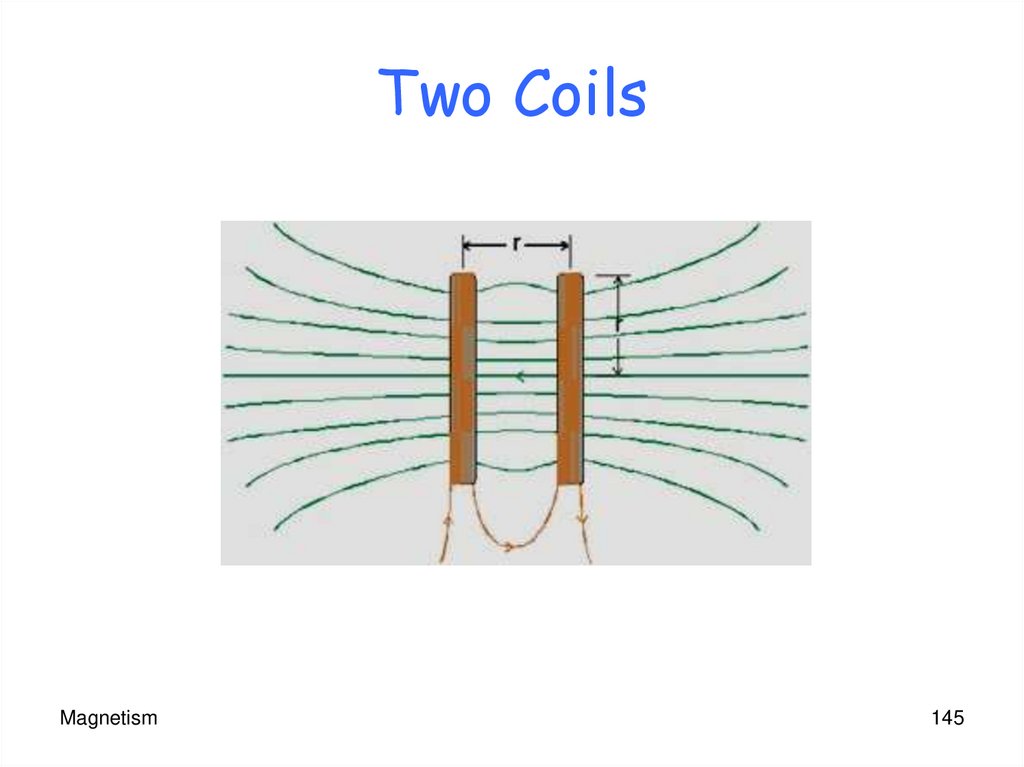
145. Two Coils
Magnetism145
146. “Real” Helmholtz Coils
Used for experiments.Can be aligned to cancel
out the Earth’s magnetic
field for critical measurements.
Magnetism
146
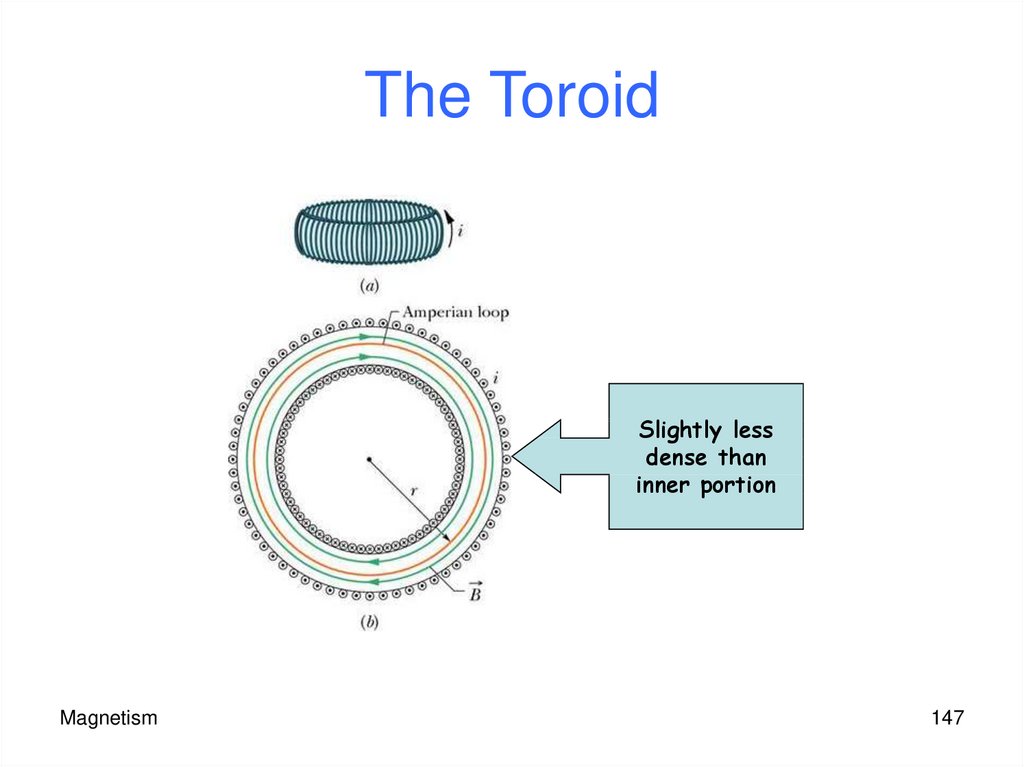
147. The Toroid
Slightly lessdense than
inner portion
Magnetism
147
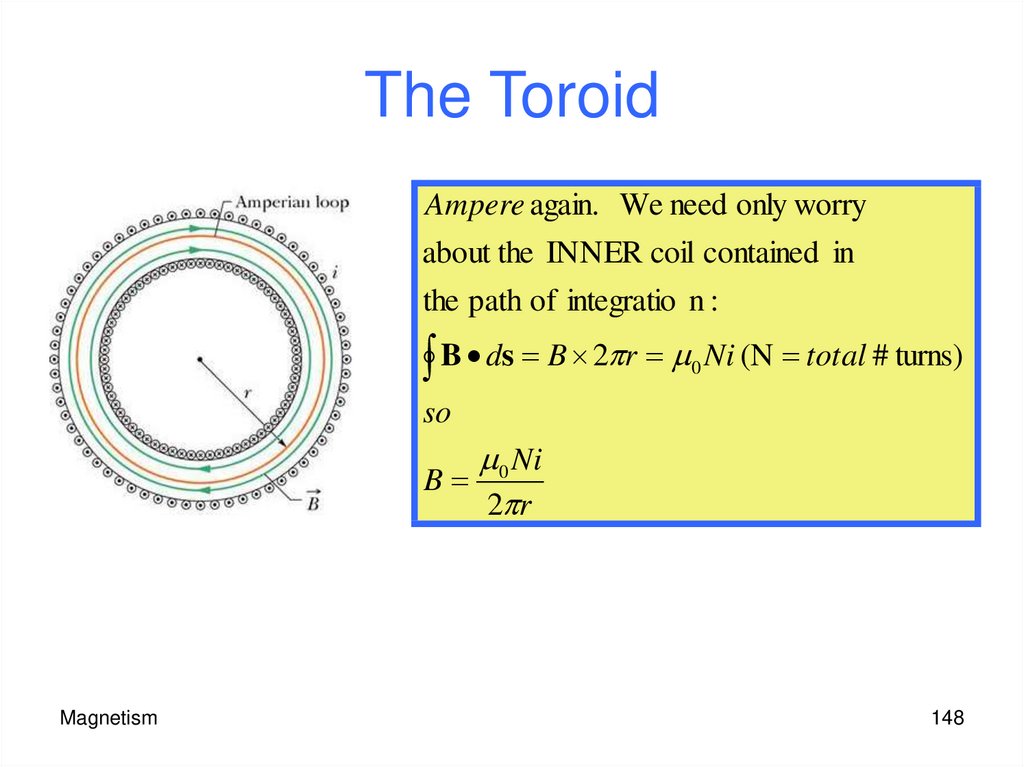
148. The Toroid
Ampere again. We need only worryabout the INNER coil contained in
the path of integratio n :
B ds B 2 r m Ni (N total # turns)
0
so
m 0 Ni
B
2 r
Magnetism
148




































 Физика
Физика








