Похожие презентации:
Matrix form of the displacement method. Stiffness matrix. Lecture 15
1. Lecture 15. Matrix form of the displacement method. Stiffness matrix.
Lr Z R p 0L r - is a matrix consisting of unit coefficients,
Z
Rp
- a vector whose components are unknown
displacements in the introduced links,
is a vector whose components are the free terms of the
canonical equations.
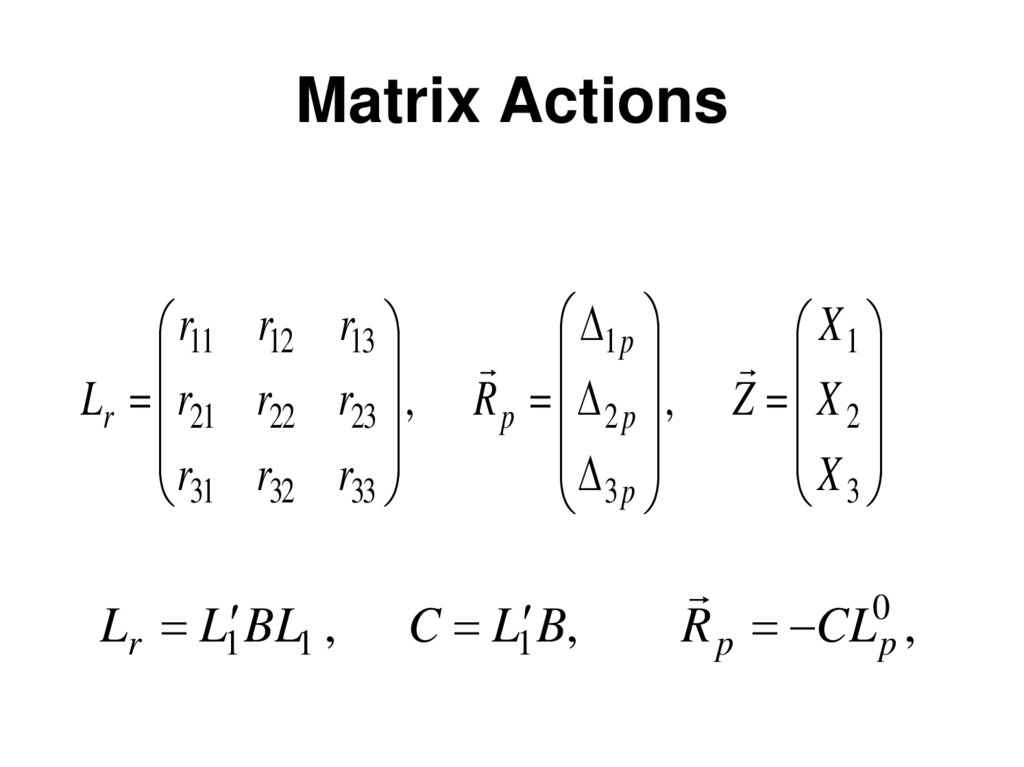
2. Matrix Actions
r11 r12 r13Lr r21 r22 r23 ,
r r r
31 32 33
Lr L1 BL1 ,
1 p
Rp 2p ,
3p
C L1 B,
X1
Z X2
X
3
0
R p CL p ,
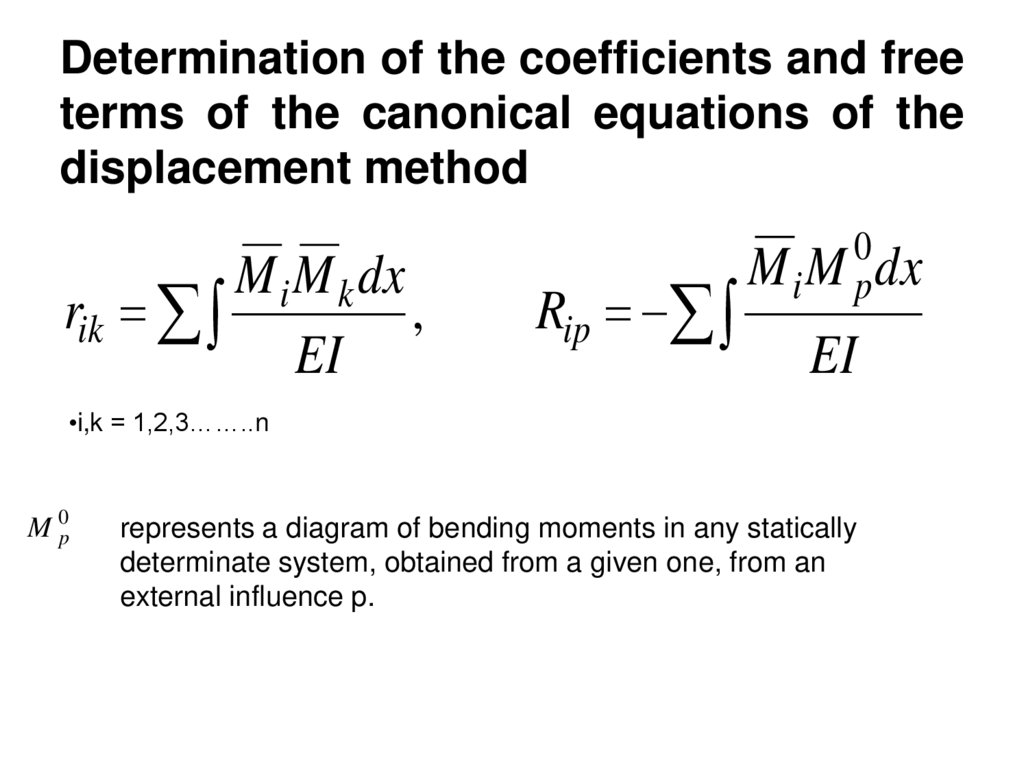
3. Determination of the coefficients and free terms of the canonical equations of the displacement method
M i M k dxrik
,
EI
Rip
0
M i M p dx
EI
•i,k = 1,2,3……..n
M 0p
represents a diagram of bending moments in any statically
determinate system, obtained from a given one, from an
external influence p.
4.
L1 - a matrix whose elements are the ordinatesof unit diagrams of bending moments at fixed
points of the main system of the displacement
method
M i , i 1,2,......n
L1
- transposed matrix, which is obtained from
a direct matrix by replacing rows with columns,
0
Lp
- a matrix, the elements of which are the
ordinates of the load diagram М0р in any
statically determinate system obtained from the
given one at fixed points, i.e. ordinates of the
diagram of bending moments in any basic
system of the method of forces from external
influence R.
5. Compliance matrix B
B10
0
0
B
0
.
.
0
0
B2
0
0
0
.
.
0
0
0
B3
0
0
.
.
0
0
0
0
.
0
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
. 0
. 0
. 0
. 0
.
. 0
. .
. .
. B s
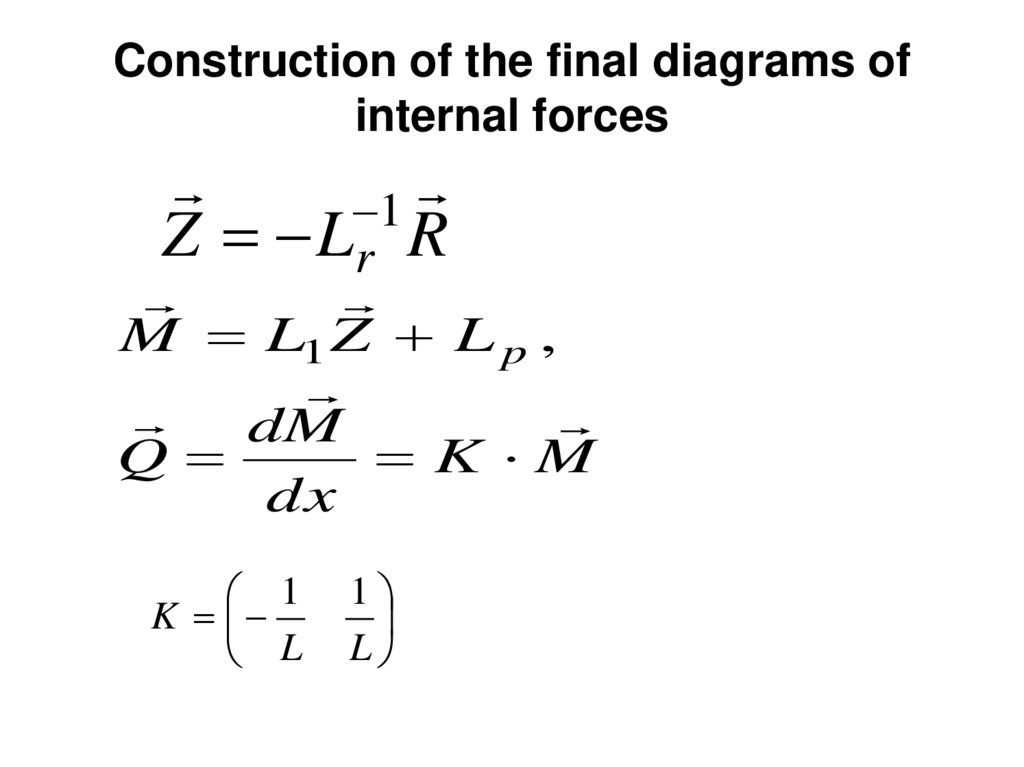
6. Construction of the final diagrams of internal forces
1Z Lr R
M L1 Z L p ,
dM
Q
K M
dx
1
K
L
1
L
7. Matrix K
1L1
0
K
.
.
,
1
L1
0
0
.
.
1 1
L2 L2
.
.
.
.
,
,
0
,
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
,
,
,
,
,
,
.
0
.
.
.
.
1 1
Ls Ls
.
0
8. Final diagrams of internal forces
nM M i Zi M p ,
i 1
n
Q Qi Z i Q p ,
i 1
n
N Ni Zi N p
i 1
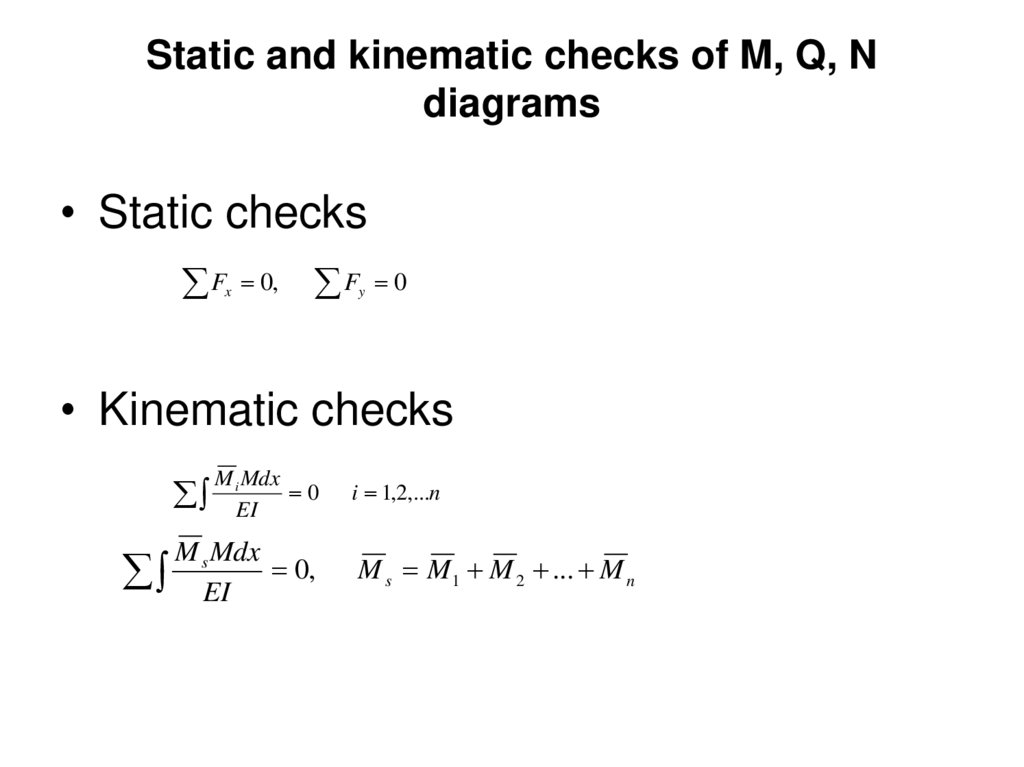
9. Static and kinematic checks of M, Q, N diagrams
• Static checksF 0, F 0
x
y
• Kinematic checks
M i Mdx
0
EI
M s Mdx
EI 0,
i 1,2,...n
M s M 1 M 2 ... M n





 Математика
Математика Физика
Физика








