Похожие презентации:
Resonator modes
1.
TECHNIONIsrael Institute of
Technology
Resonator modes
CLASS EXERCISE 8
הטכניון
מכון טכנולוגי
לישראל
2.
Long. And trans. Resonance frequencies•Resonance frequency of the system:
• Beam full round-trip ↔ phase 2πq (where q is an integer)
•In the FP case this leads to:
c
m
2nL
3.
Long. And trans. Resonance frequencies•In FP the mirrors are flat plane waves
•For curved mirrors the beams have transversal profile
•How does it change the solutions?
4.
Long. And trans. Resonance frequencies•Reminder: beams and mirrors curvatures are matched
•This means that solving for r=0 is enough
5.
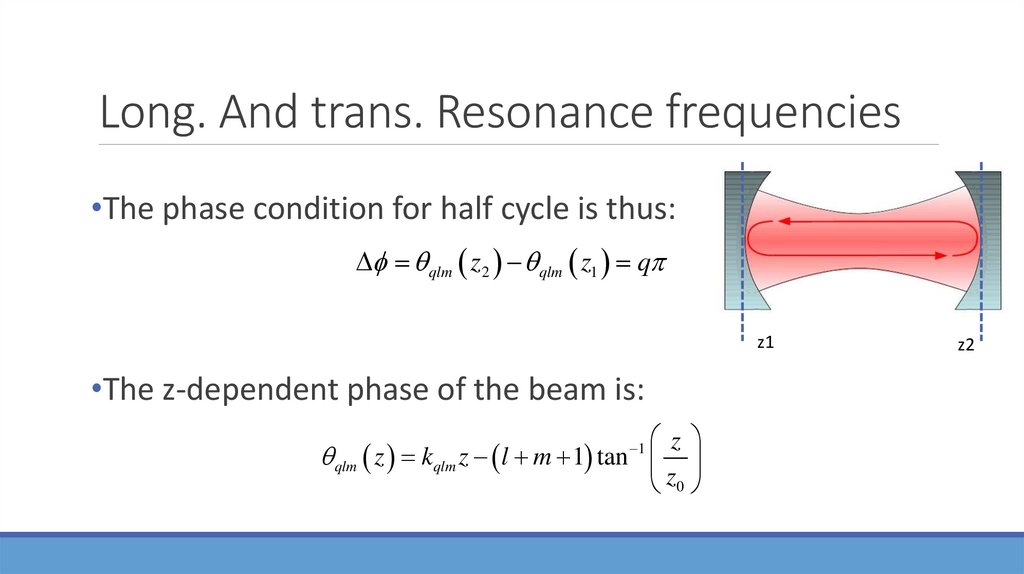
Long. And trans. Resonance frequencies•The phase condition for half cycle is thus:
qlm z2 qlm z1 q
z1
•The z-dependent phase of the beam is:
z
qlm z kqlm z l m 1 tan
z0
1
z2
6.
Long. And trans. Resonance frequencies•Thus we get:
1 z2
1 z1
kqlm z2 z1 l m 1 tan tan q
z0
z0
kqlm L l m 1 q
z1
z2
7.
Long. And trans. Resonance frequencies•From this equation we learn:
kqlm L l m 1 q
• The phase depends on q
• The phase depends on transverse characteristics (l,m)
8.
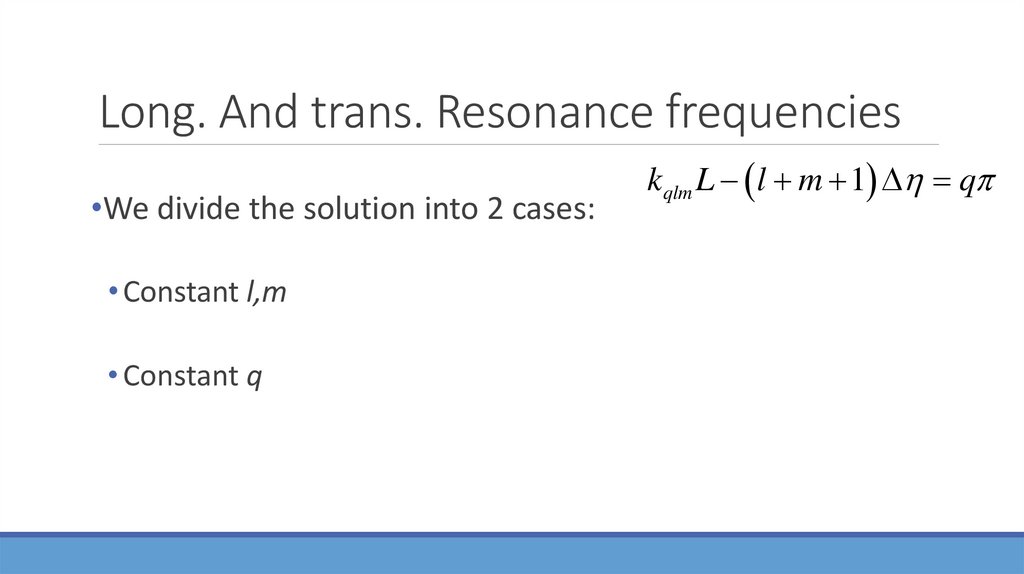
Long. And trans. Resonance frequencies•We divide the solution into 2 cases:
• Constant l,m
• Constant q
kqlm L l m 1 q
9.
Constant l,m – Longitudinal modes•We write the equation for q and q+1:
kq L l m 1 q
kq 1 L l m 1 q 1
kqlm L l m 1 q
kq 1 kq L
2 nL
q 1 q
c
c
q 1 q
2nL
10.
Constant l,m – Longitudinal modes•We got:
c
q 1 q
FSRFP
2nL
•Which is exactly the FSR of a FP resonator
•These modes depend only on the length of the resonator
• they are called, thus, Longitudinal modes
11.
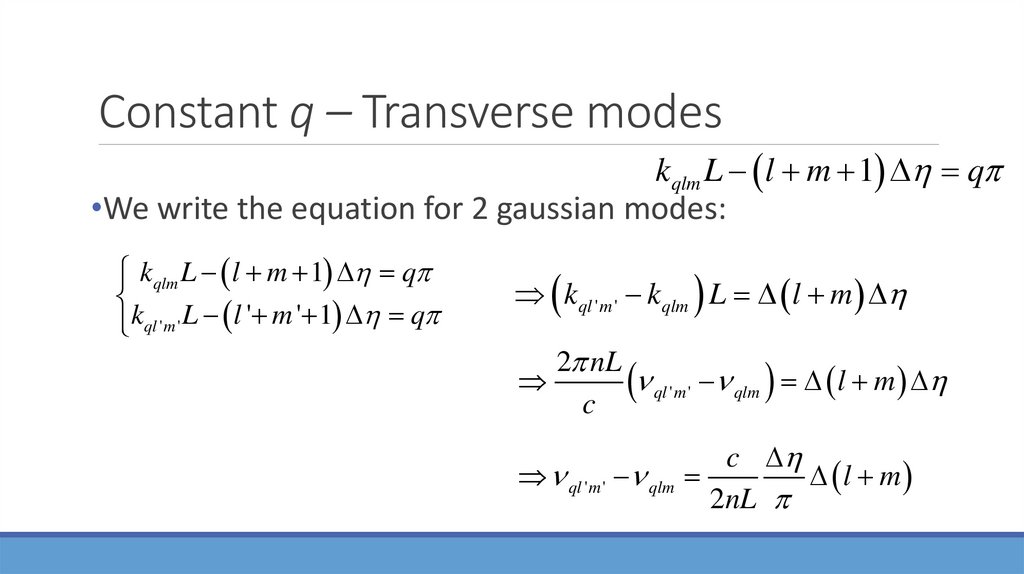
Constant q – Transverse modeskqlm L l m 1 q
•We write the equation for 2 gaussian modes:
kqlm L l m 1 q
kql ' m ' L l ' m ' 1 q
kql ' m ' kqlm L l m
2 nL
ql ' m ' qlm l m
c
ql ' m ' qlm
c
l m
2nL
12.
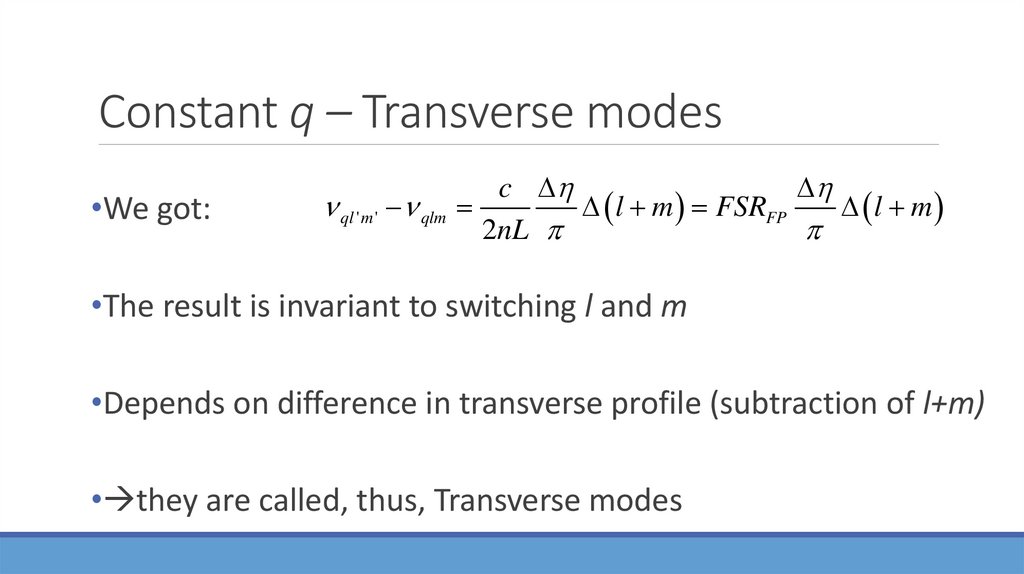
Constant q – Transverse modes•We got:
ql ' m ' qlm
c
l m FSRFP
l m
2nL
•The result is invariant to switching l and m
•Depends on difference in transverse profile (subtraction of l+m)
• they are called, thus, Transverse modes
13.
Examples – symmetric resonator•Symmetric resonator:
z1 z2
• Thus we have:
z1
z2
1 z1
1 z 2
tan tan 2 tan
z0
z0
z0
1
z2
14.
Examples – confocal symmetric resonator•Confocal symmetric resonator:
• If the resonator is also confocal:
z1
z1 z2 z2 L / 2
R L
z2
15.
Examples – confocal symmetric resonator•Solving L as a function of z0:
z0 2
R R z2 z2
L
z2
z0 2
L
2
L
2
L
z0 2 L2 / 4
z0 L / 2
z1 z2 z2 L / 2
R L
16.
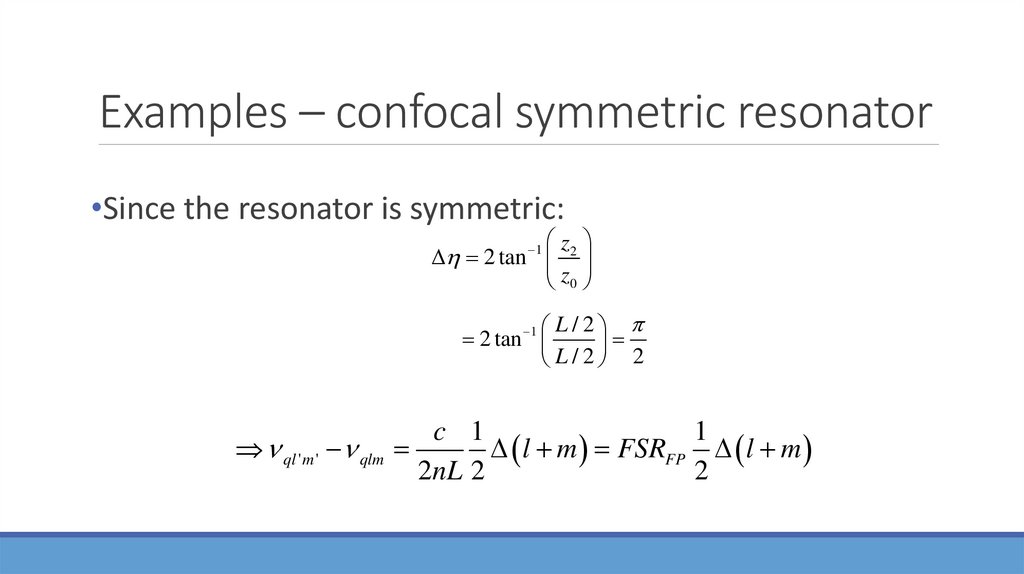
Examples – confocal symmetric resonator•Since the resonator is symmetric:
z2
2 tan
z0
1
L/2
2 tan
L/2 2
1
ql ' m ' qlm
c 1
1
l m FSRFP l m
2nL 2
2
17.
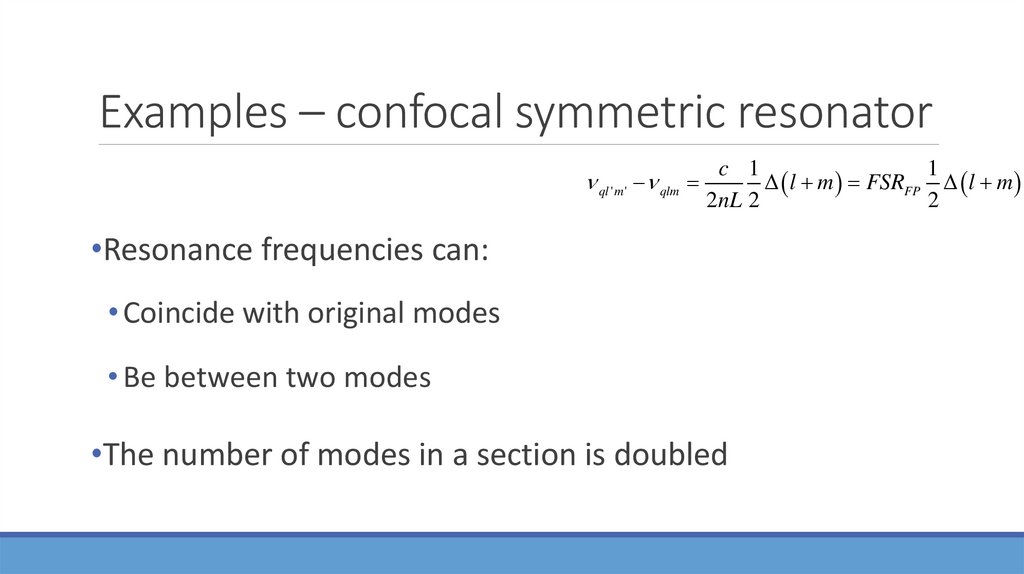
Examples – confocal symmetric resonatorql ' m ' qlm
c 1
1
l m FSRFP l m
2nL 2
2
•Resonance frequencies can:
• Coincide with original modes
• Be between two modes
•The number of modes in a section is doubled
18.
Examples – nearly planar resonator•We assume:
•Thus we have:
R
L
z0 2
R R z2 z2
z2
•This leads to either:
z2
z2
L
z0
L
z1
z2
19.
Examples – nearly planar resonator•The first option is impossible since by definition z2 L
•Thus given z2
z0 we have:
z2
z2 z1 z2 z1
1 z1
tan tan
z0
z0
z0 z0 z0
1
L
z0
20.
Examples – nearly planar resonator•So the resonance frequencies are: ql ' m ' qlm
c
l m
2n z0
•Since z0>>L we have many frequencies between long. freqs.
•This is undesirable since quality and coherence are determined
by the number of operating modes
21.
A circular resonator•Given by 3 mirrors on the vertices of an equilateral triangle
22.
A circular resonator•The upper (entrance) and left (exit) mirrors are
dielectric mirrors with: r=-r’
•The right mirror is fully reflective with R=1
•Notice that reflections add π phase and the
perimeter of the triangle is L
23.
A circular resonator•What are the transmission intensity and the
resonance frequencies?
24.
A circular resonator•We calculate the transmission by adding transmitted waves
as we did for FP:
ikL /3
A1 Ate
t'
i
A2 A1 r ' 1 eikL
2
A3 A2 r ' 1 eikL
2
•And so on
25.
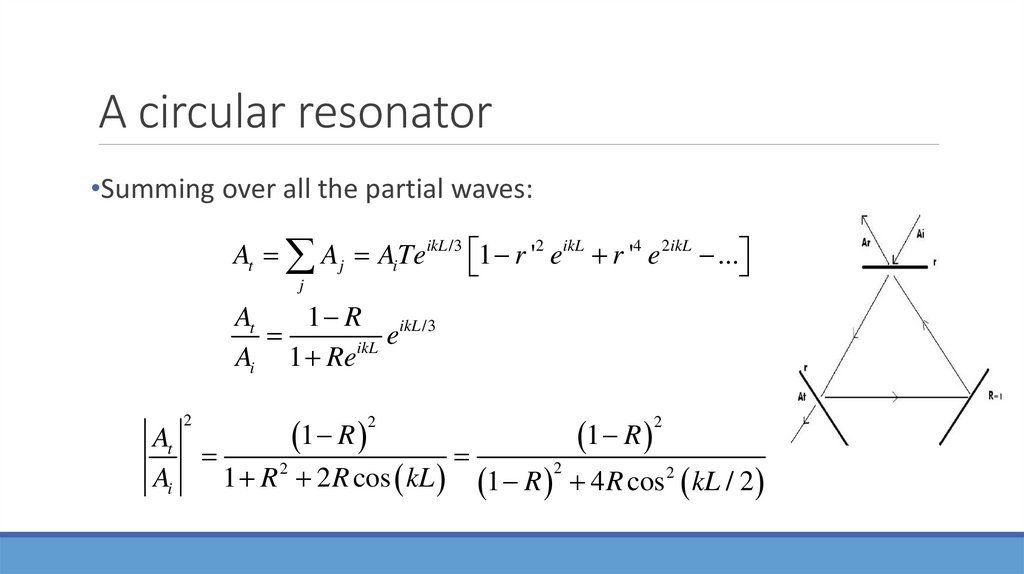
A circular resonator•Summing over all the partial waves:
ikL /3
2 ikL
4 2 ikL
At Aj ATe
1
r
'
e
r
'
e ...
i
j
At
1 R ikL /3
e
ikL
Ai 1 Re
At
Ai
1 R
2
1 R 2 R cos kL
2
1 R
2
1 R
2
2
4 R cos 2 kL / 2
26.
A circular resonator•The resonance frequencies depend on the cosine of the phase, not on
the sine as in FP
It Ii
1 R
1 R
2
2
4 R cos 2 kL / 2
27.
A circular resonator•Thus the resonance frequencies are shifted, but the FSR is not changed:
cos 2 kL / 2 0
km L
2m 1
2
2
2 nL
m 2m 1
2c
2
c
m
2m 1
2nL
c
nL
28.
A circular resonator•We add a mirror between the lower mirrors. Find the waist of the beam
in the resonator
29.
A circular resonator•We use the analogy to curved mirrors resonators:
Waist in the middle
L/3
Waist in the middle
L
30.
A circular resonator•We can calculate the size as in the curved mirrors resonator with R=2f:
z2 L / 2 R 2 f
z0 2 L
z0 2
2 f z2
2
z2 2
L
z0 2
L
L
2
f
2
2
z c
0 0
n
2
L
L
0
2f
n 2
2
2
31.
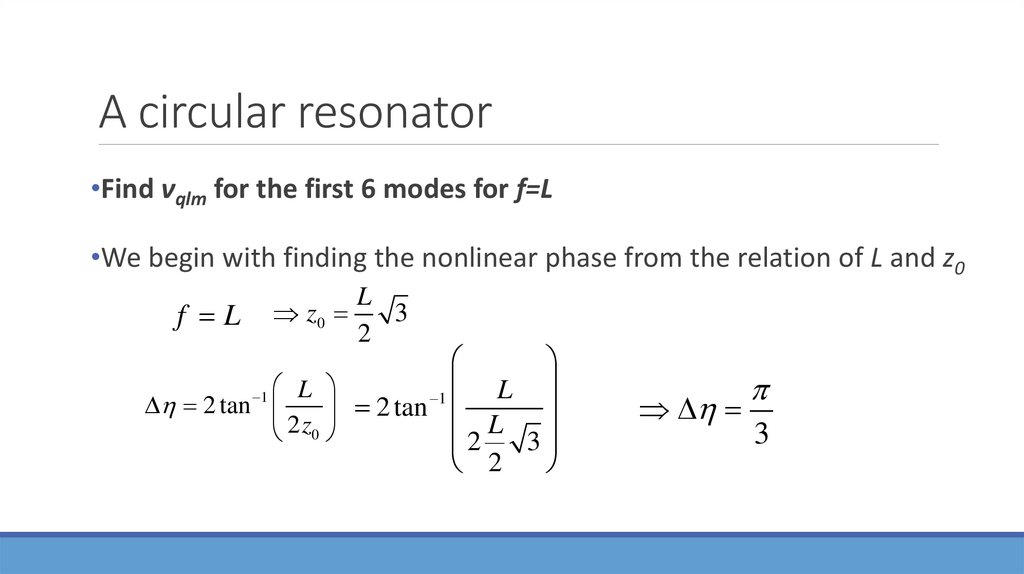
A circular resonator•Find νqlm for the first 6 modes for f=L
•We begin with finding the nonlinear phase from the relation of L and z0
f L
L
z0
3
2
L
1 L
1
2 tan
2 tan
2
z
L
0
2
3
2
3
32.
A circular resonator•We notice that the output should gain a phase of some multiple of 2π
over a distance L (not 2L!)
•We should also add a π phase on each round due to reflection
33.
A circular resonator2 nL
qlm l m 1 2q
c
2 nL
qlm l m 1 2q 1
c
3
qlm
c
l m 1
2q 1
2nL
3
34.
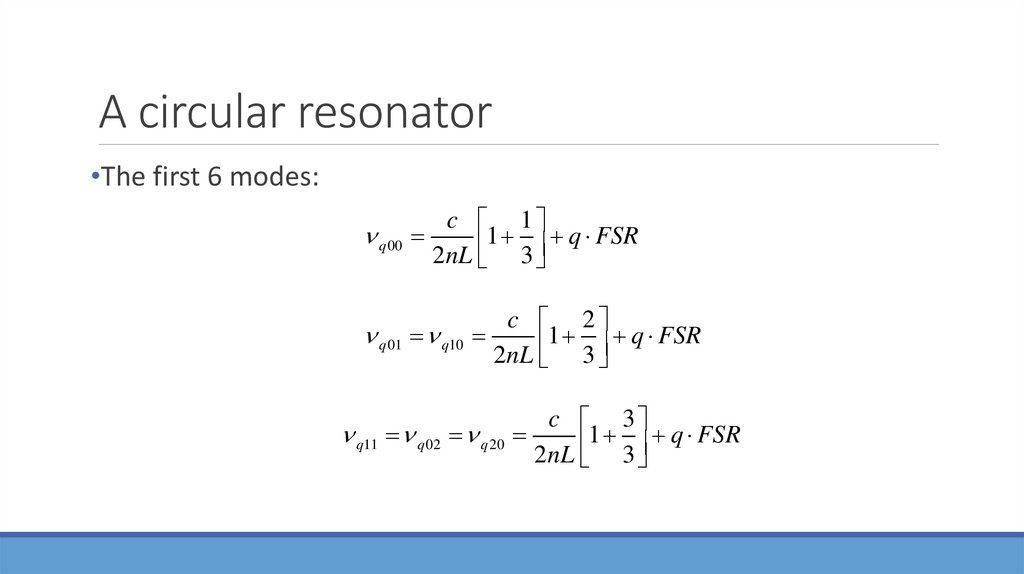
A circular resonator•The first 6 modes:
q 00
c 1
1 q FSR
2nL 3
q 01 q10
c 2
1 q FSR
2nL 3
q11 q 02 q 20
c 3
1 q FSR
2nL 3
35.
A circular resonatorc 1
q 00
1 q FSR
•We see that the new resonances are shifted
2nL 3
by c/2nL from FP (because of the π
c 2
q 01 q10
1 q FSR
2nL 3
reflection phase)
c 3
q11 q 02 q 20
1 q FSR
2nL 3
•There are 5 new frequencies between each
two:
l m
2
3

























 Математика
Математика








