Похожие презентации:
Basic dynamic elements
1. AUTOMATICS and AUTOMATIC CONTROL
LECTURE 4dr inż. Adam Kurnicki
Automation and Metrology Department
Room no 210A
2.
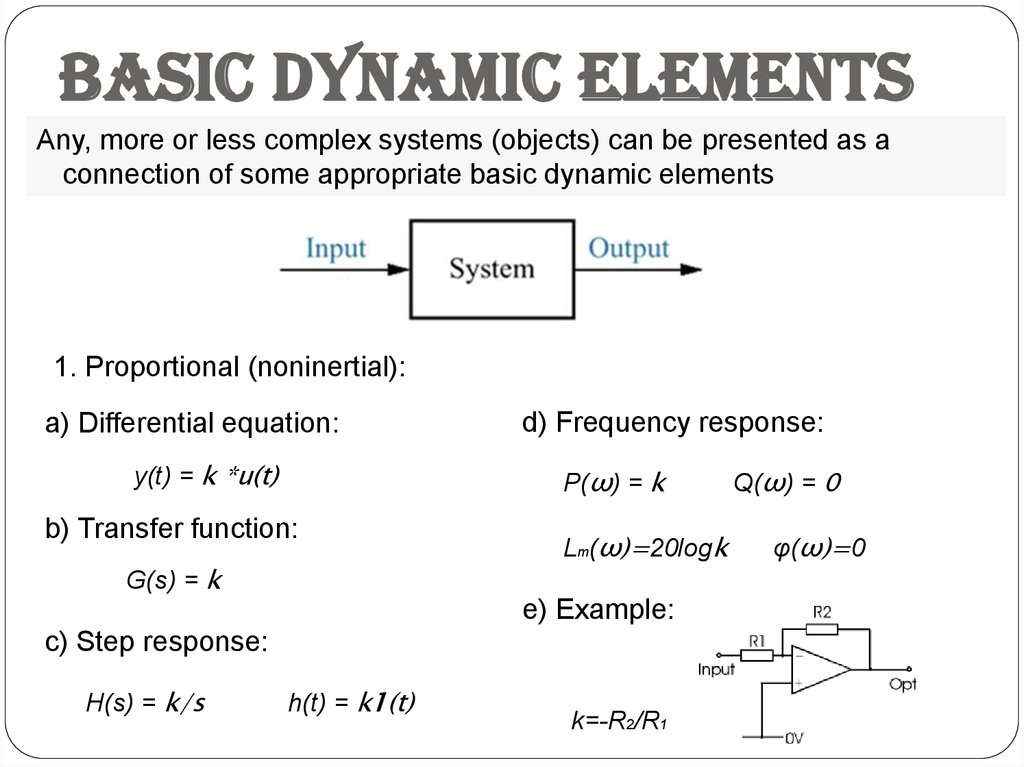
Basic dynamic elementsAny, more or less complex systems (objects) can be presented as a
connection of some appropriate basic dynamic elements
1. Proportional (noninertial):
a) Differential equation:
y(t) = k *u(t)
d) Frequency response:
P(ω) = k
b) Transfer function:
G(s) = k
Lm(ω)=20logk
e) Example:
c) Step response:
H(s) = k/s
h(t) = k1(t)
k=-R2/R1
Q(ω) = 0
φ(ω)=0
3.
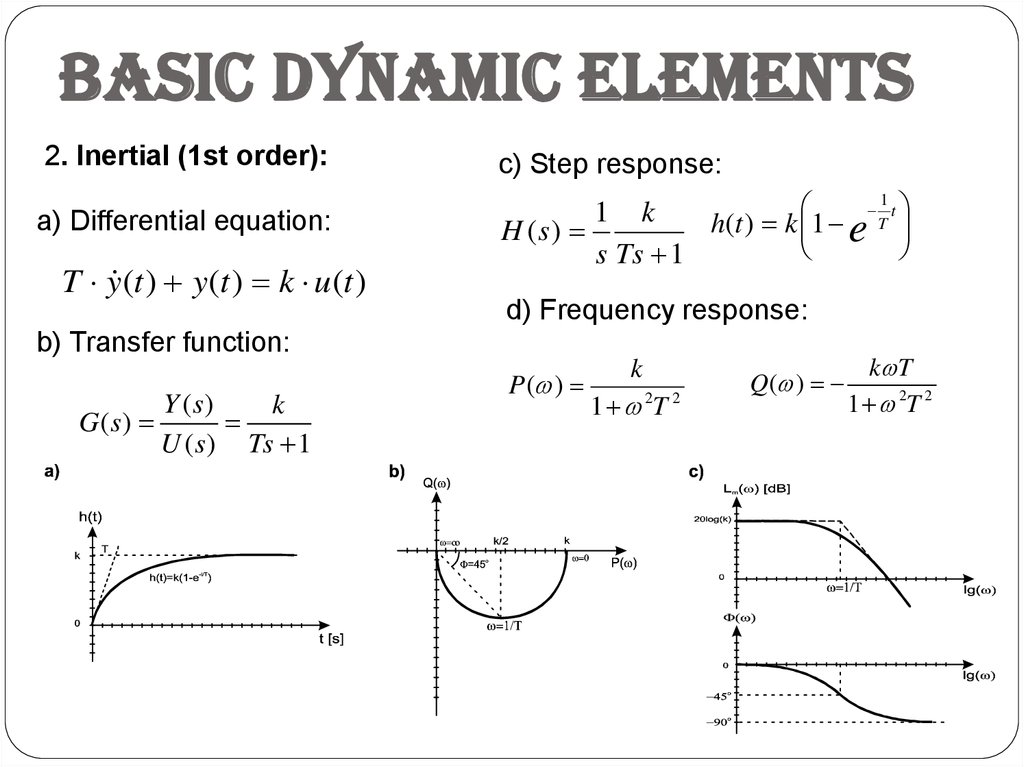
Basic dynamic elements2. Inertial (1st order):
a) Differential equation:
T y (t ) y (t ) k u (t )
c) Step response:
1
t
1 k
h(t ) k 1 e T
H ( s)
s Ts 1
d) Frequency response:
b) Transfer function:
Y ( s)
k
G( s)
U ( s) Ts 1
P ( )
k
1 2T 2
Q( )
k T
1 2T 2
4.
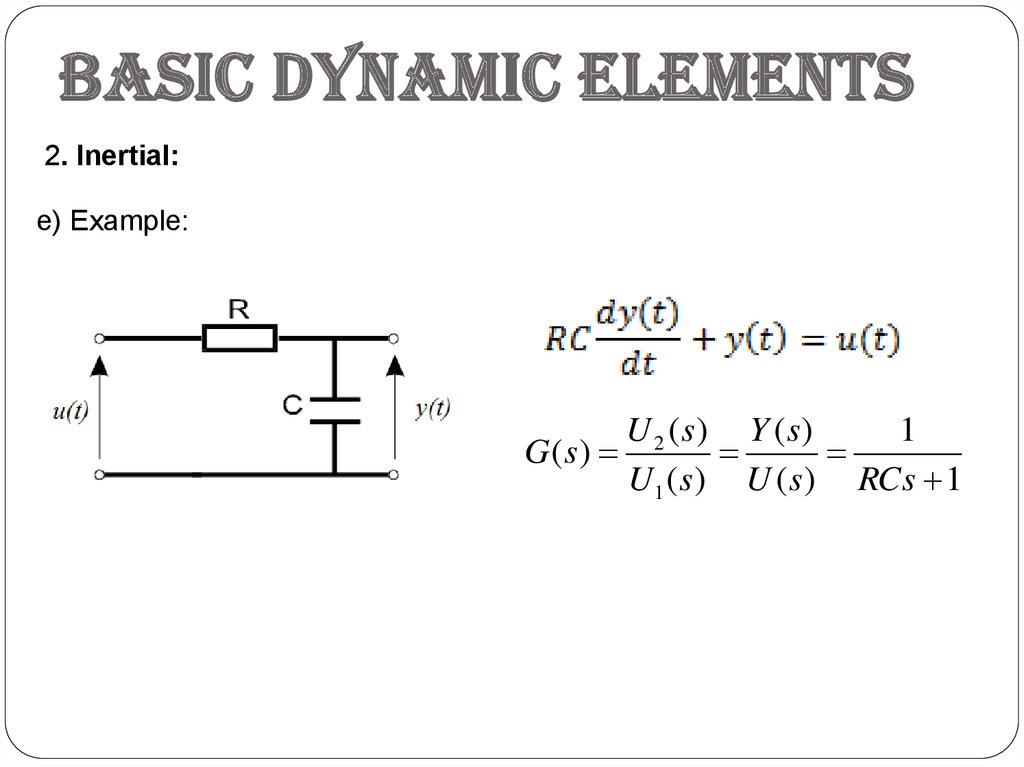
Basic dynamic elements2. Inertial:
e) Example:
U 2 (s) Y (s)
1
G(s)
U1 ( s) U ( s) RCs 1
5.
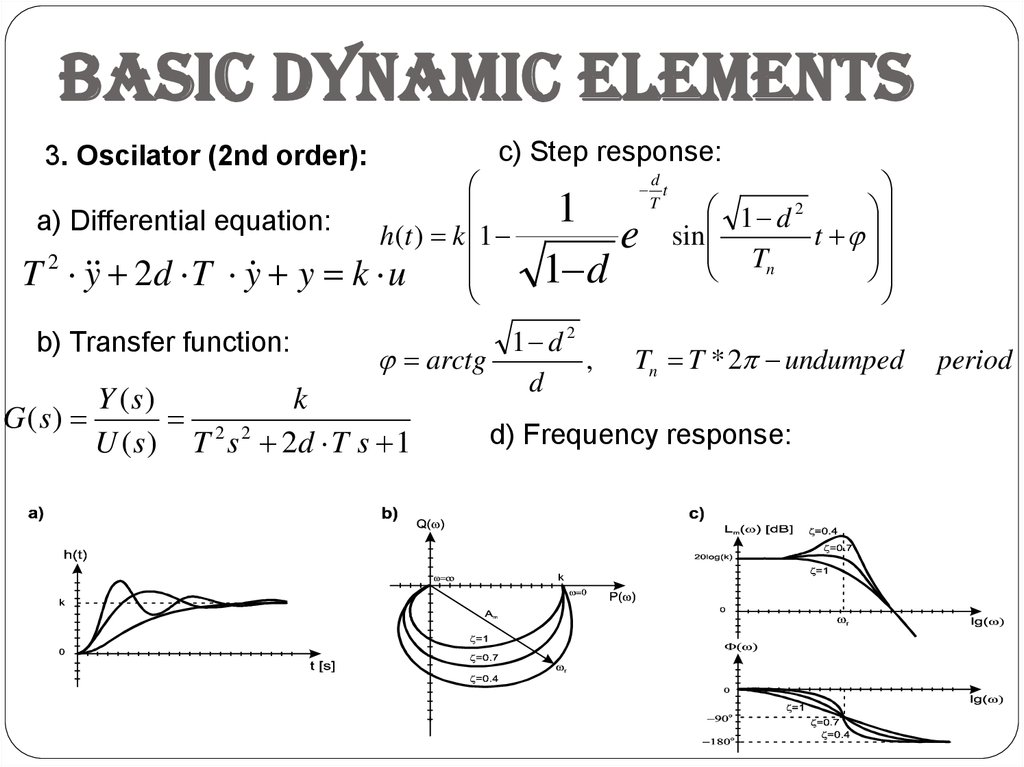
Basic dynamic elementsc) Step response:
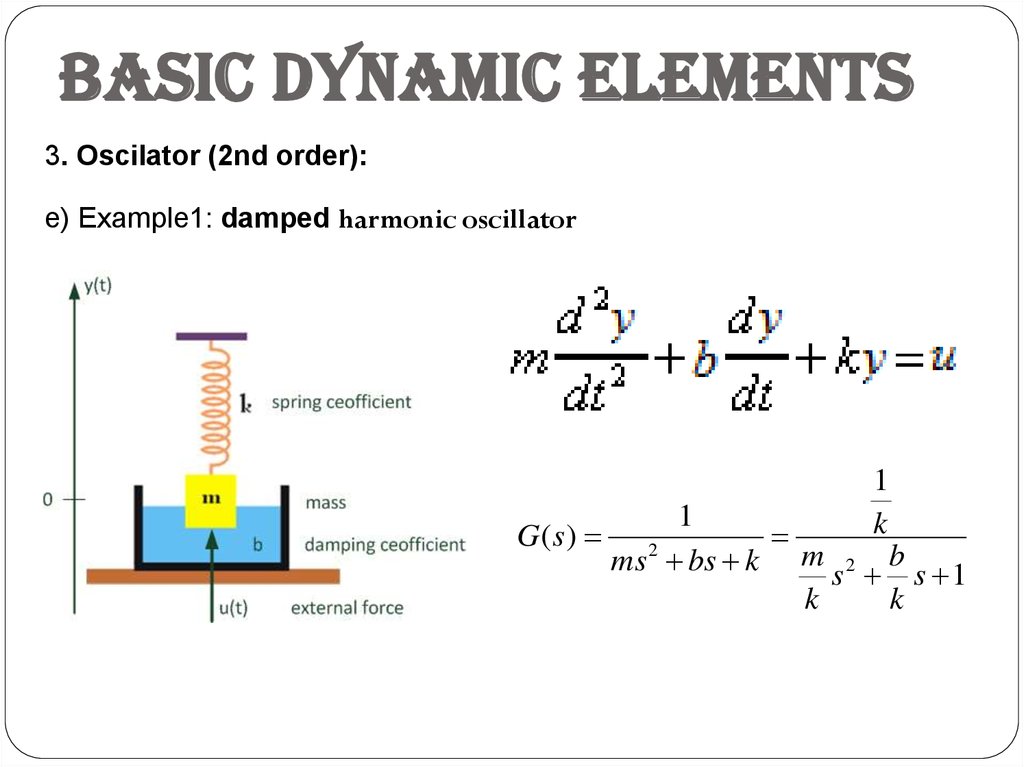
3. Oscilator (2nd order):
a) Differential equation:
h(t ) k 1
T 2 y 2d T y y k u
b) Transfer function:
1
e
1 d
1 d 2
arctg
,
d
Y (s)
k
G (s)
2 2
U ( s ) T s 2d T s 1
d
t
T
sin
1 d
t
Tn
2
Tn T * 2 undumped
d) Frequency response:
period
6.
Basic dynamic elements3. Oscilator (2nd order):
e) Example1: damped harmonic oscillator
G(s)
1
k
1
2
ms bs k m s 2 b s 1
k
k
7.
Basic dynamic elements3. Oscilator (2nd order):
e) Example 2: RLC circuit
di (t )
u (t ) i (t ) R L dt y (t )
dy (t )
i (t ) C
dt
dy 2 (t )
dy (t )
LC
RC
y (t ) u (t )
2
dt
dt
G( s)
1
LCs 2 RCs 1
8.
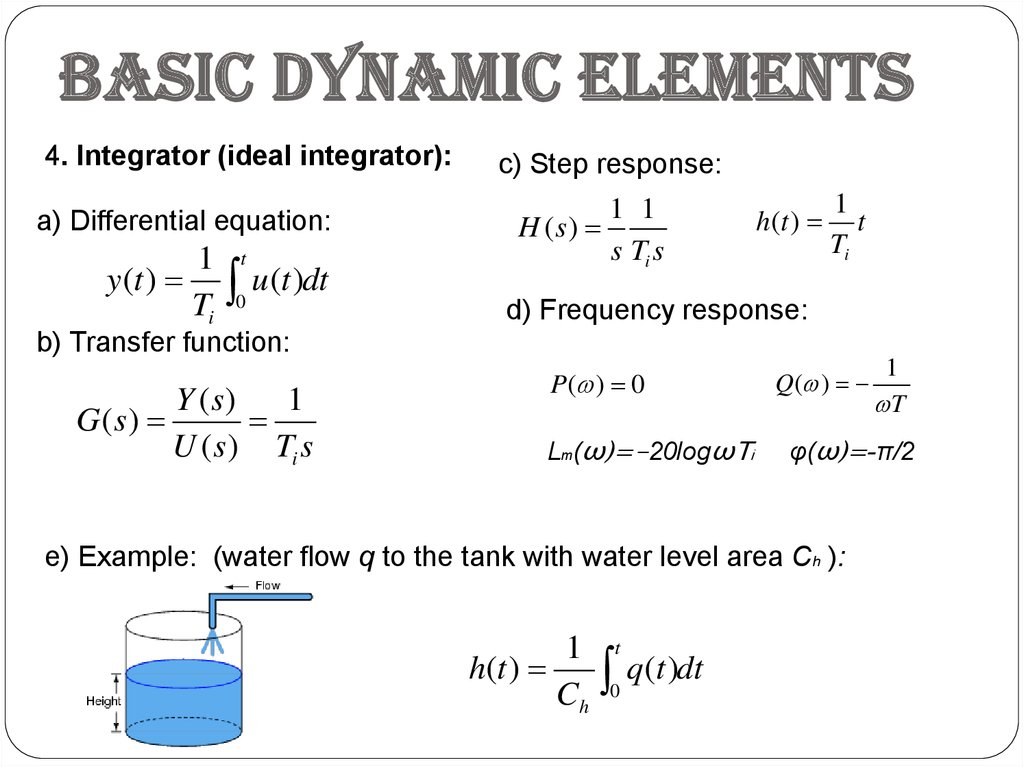
Basic dynamic elements4. Integrator (ideal integrator):
a) Differential equation:
1
y (t )
Ti
t
u (t )dt
0
c) Step response:
1 1
H ( s)
s Ti s
h (t )
1
t
Ti
d) Frequency response:
b) Transfer function:
G (s)
Y (s)
1
U ( s ) Ti s
P( ) 0
Lm(ω)=-20logωTi
Q( )
φ(ω)=-π/2
e) Example: (water flow q to the tank with water level area Ch ):
1
h(t )
Ch
t
q(t )dt
0
1
T
9.
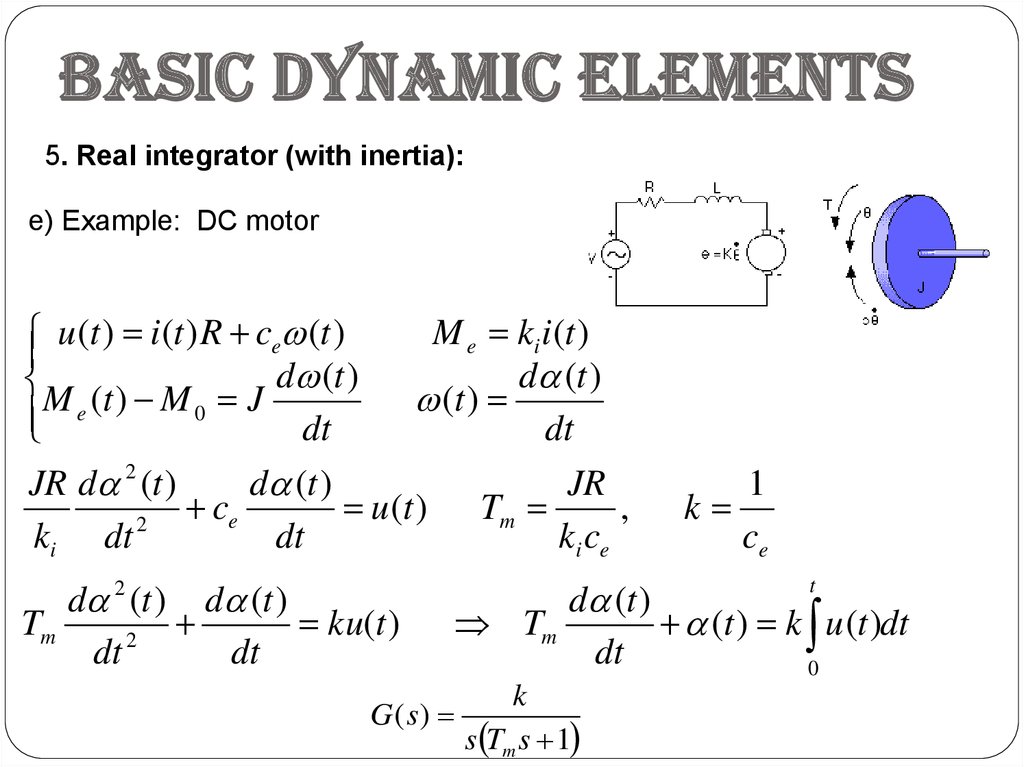
Basic dynamic elements5. Real integrator (with inertia):
a) Differential equation:
dy (t )
1
T
y (t )
dt
Ti
t
u(t )dt
0
c) Step response:
1
1
1
1
1
t
H (s)
h(t ) t T 1 e T
s Ti s (Ts 1)
Ti
Ti
d) Frequency response:
b) Transfer function:
G(s)
Y (s)
1
U ( s ) Ti s (Ts 1)
P( )
T
Ti (1 2T 2 )
Q( )
1
Ti (1 2T 2 )
10.
Basic dynamic elements5. Real integrator (with inertia):
e) Example: DC motor
M e ki i (t )
u (t ) i (t ) R ce (t )
d (t )
M (t ) M J d (t )
(t )
0
e
dt
dt
JR
d (t )
JR d 2 (t )
,
u (t ) Tm
ce
2
ki ce
dt
ki dt
d 2 (t ) d (t )
ku(t )
Tm
2
dt
dt
1
k
ce
d (t )
(t ) k u (t )dt
Tm
dt
0
k
G ( s)
s Tm s 1
t
11.
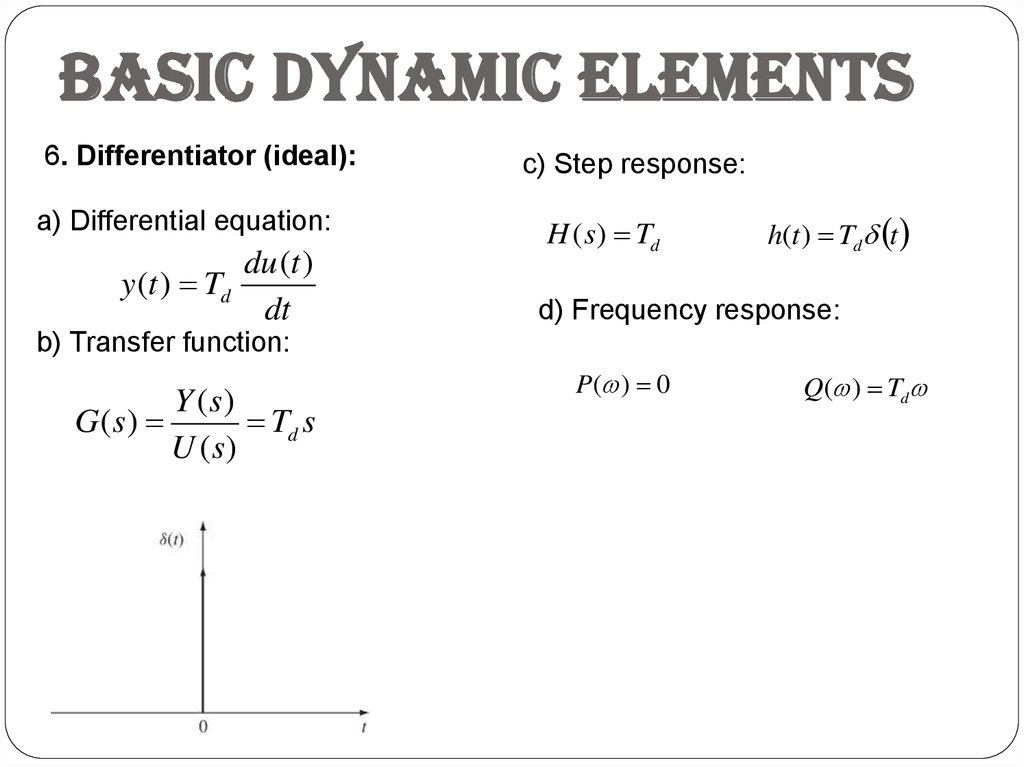
Basic dynamic elements6. Differentiator (ideal):
a) Differential equation:
y (t ) Td
du (t )
dt
c) Step response:
H ( s) Td
h(t ) Td t
d) Frequency response:
b) Transfer function:
Y ( s)
G( s)
Td s
U ( s)
P( ) 0
Q( ) Td
12.
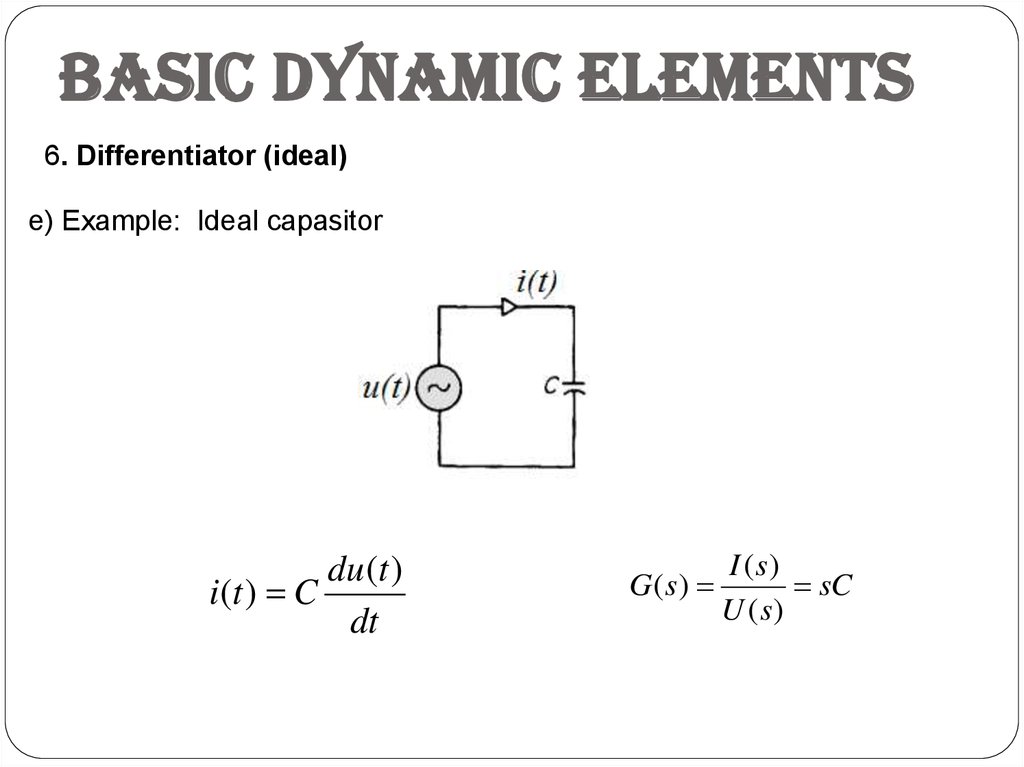
Basic dynamic elements6. Differentiator (ideal)
e) Example: Ideal capasitor
du (t )
i (t ) C
dt
G( s)
I (s)
sC
U ( s)
13.
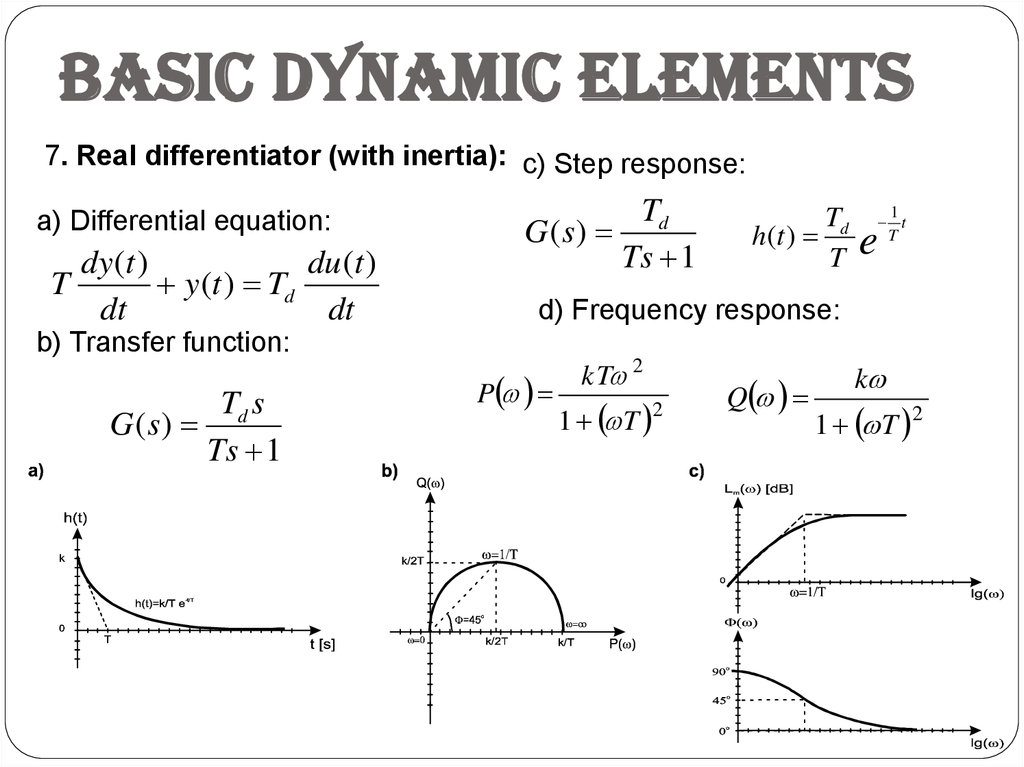
Basic dynamic elements7. Real differentiator (with inertia): c) Step response:
a) Differential equation:
T
dy (t )
du (t )
y (t ) Td
dt
dt
Td
G ( s)
Ts 1
1
t
T
e
d) Frequency response:
b) Transfer function:
Td s
G ( s)
Ts 1
T
h(t ) d
T
P
kT 2
1 T 2
Q
k
1 T 2
14.
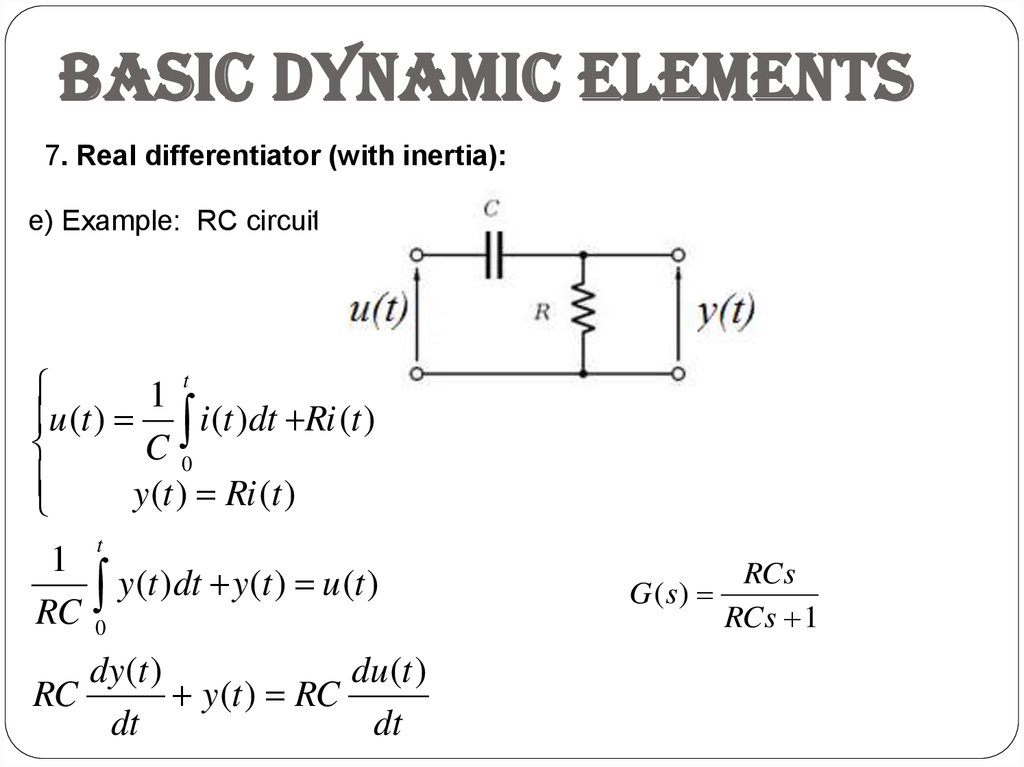
Basic dynamic elements7. Real differentiator (with inertia):
e) Example: RC circuit
t
1
u (t ) i (t )dt Ri (t )
C0
y (t ) Ri (t )
t
1
y (t )dt y (t ) u (t )
RC 0
dy (t )
du (t )
RC
y (t ) RC
dt
dt
G ( s)
RCs
RCs 1
15.
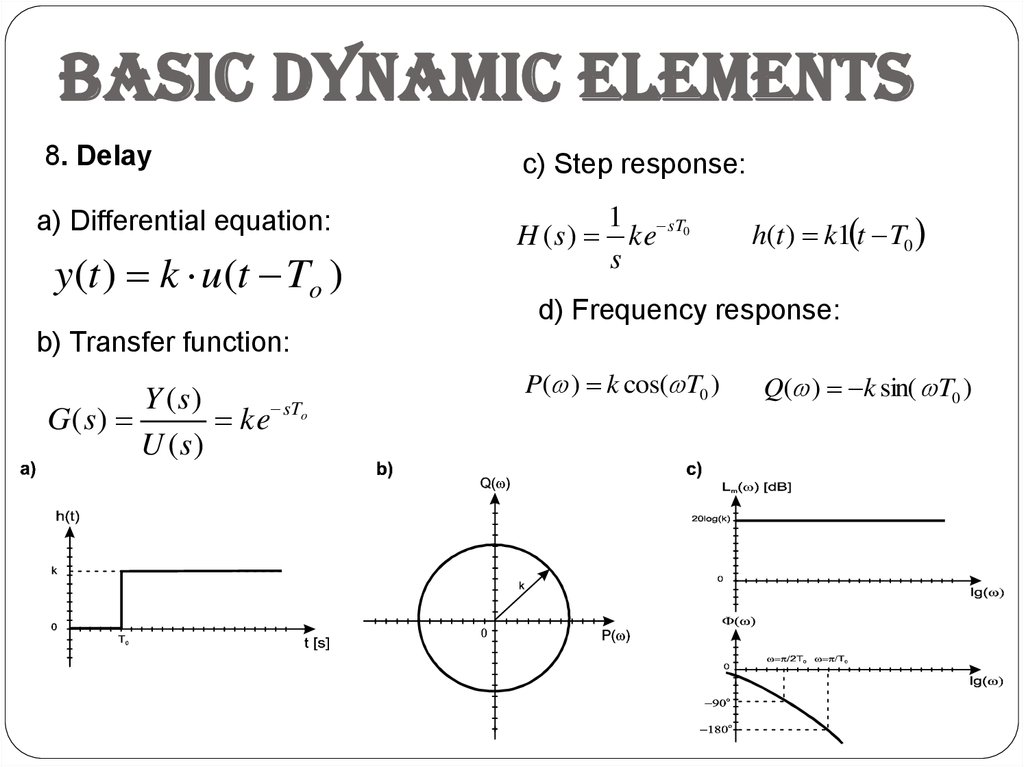
Basic dynamic elements8. Delay
c) Step response:
a) Differential equation:
1 sT0
H ( s ) ke
s
y(t ) k u(t To )
h(t ) k1 t T0
d) Frequency response:
b) Transfer function:
Y ( s)
G( s)
ke sTo
U ( s)
P( ) k cos( T0 )
Q( ) k sin( T0 )
16.
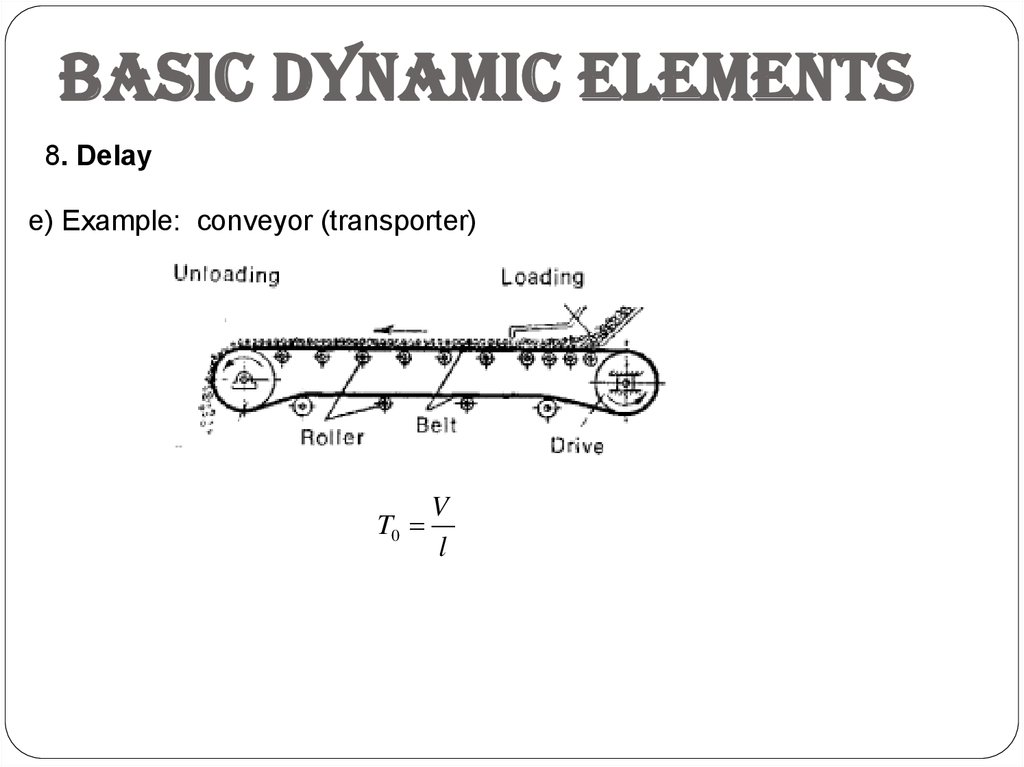
Basic dynamic elements8. Delay
e) Example: conveyor (transporter)
T0
V
l





 Математика
Математика








