Похожие презентации:
The photon and thefor vacuum cleaner
1.
The photonand thefor
vacuum
cleaner
Continuous
variables
discrete
photons
Alfred U’Ren
Daryl Achilles
Peter Mosley
Lijian Zhang
Christine Silberhorn
Konrad Banaszek
Michael G. Raymer
Ian A. Walmsley
The Center for
Quantum
Information
2.
Outline• Continuous variables for single photons
• Reduced noise: Fock states
• Increased correlations: Engineered space-time entanglement
• Application: single-photon CV QKD
Ultrafast ?
• Peak intensity vs average power: brighter nonclassical light
• Precise timing: concatenating nonclassical sources
• Broad bandwidth: engineering space-time correlations
3.
Continuous variables for singlephotons
• Localized modes
• Role in QIP
• Reduced noise: Fock states
• Increased correlations: Engineered space-time entanglement
• Application: single-photon CV QKD
4.
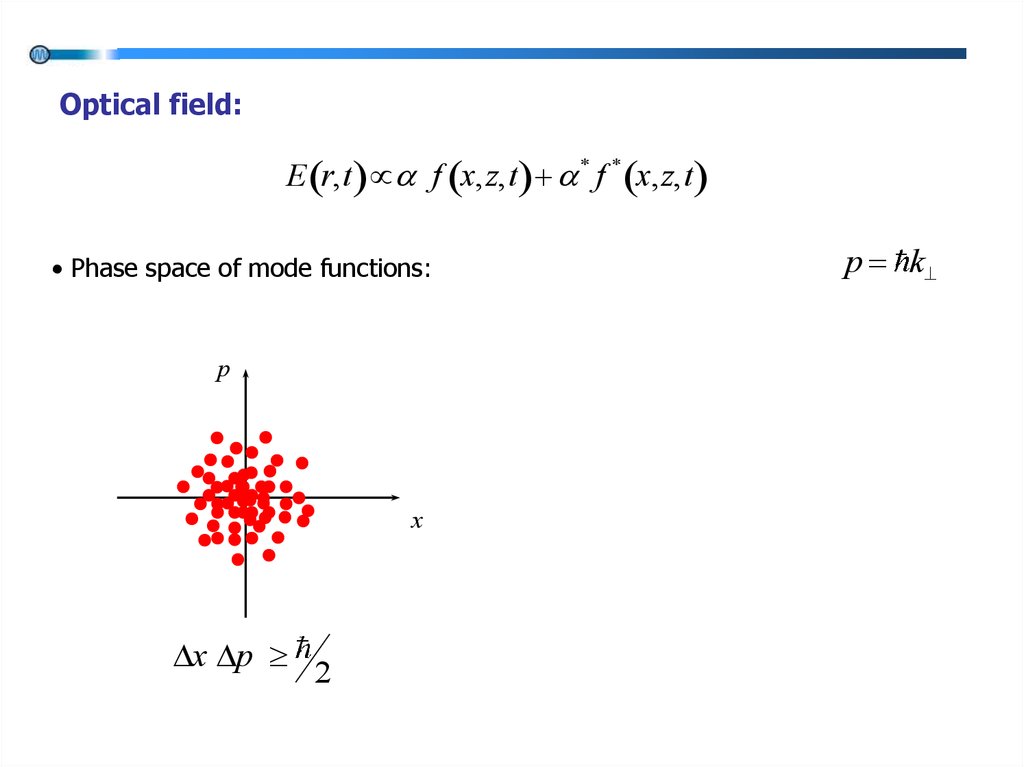
Optical field:E r,t f x,z,t * f * x,z,t
p k
• Phase space of mode functions:
p
x
x p 2
5.
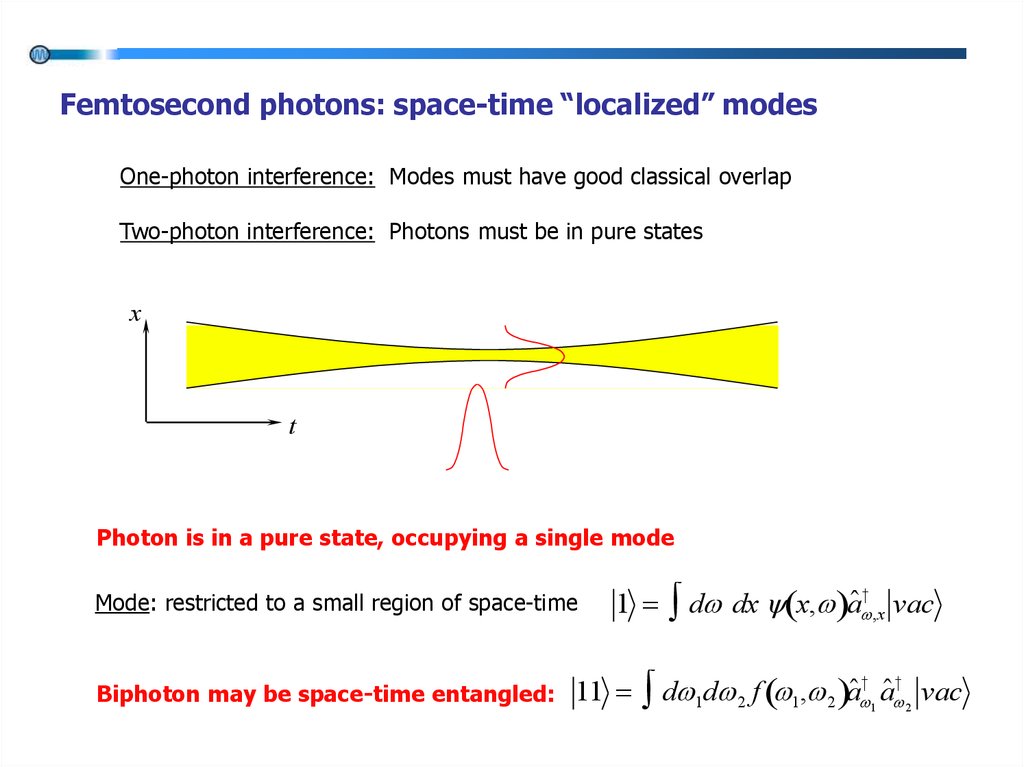
Femtosecond photons: space-time “localized” modesOne-photon interference: Modes must have good classical overlap
Two-photon interference: Photons must be in pure states
x
t
Photon is in a pure state, occupying a single mode
Mode: restricted to a small region of space-time
Biphoton may be space-time entangled:
1
11
d dx x, aˆ
†
,x
vac
d d f , aˆ aˆ
†
1
2
1
2
†
1
2
vac
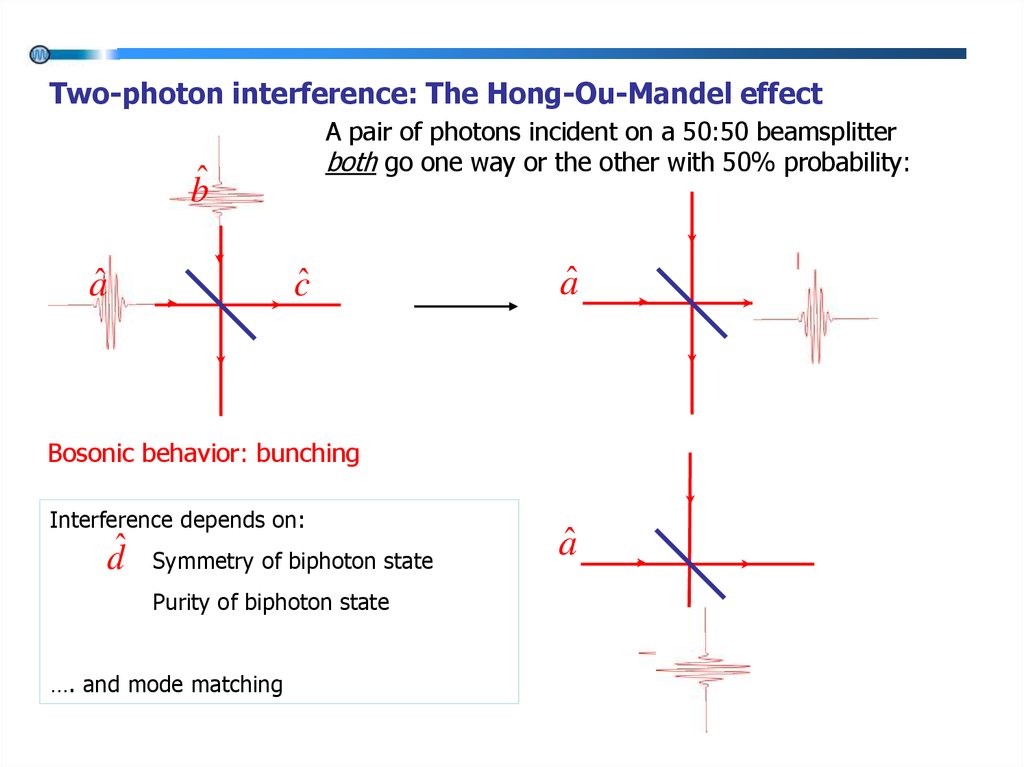
6. Two-photon interference: The Hong-Ou-Mandel effect
A pair of photons incident on a 50:50 beamsplitterboth go one way or the other with 50% probability:
bˆ
cˆ
aˆ
aˆ
Bosonic behavior: bunching
Interference depends on:
dˆ
Symmetry of biphoton state
Purity of biphoton state
…. and mode matching
aˆ
7.
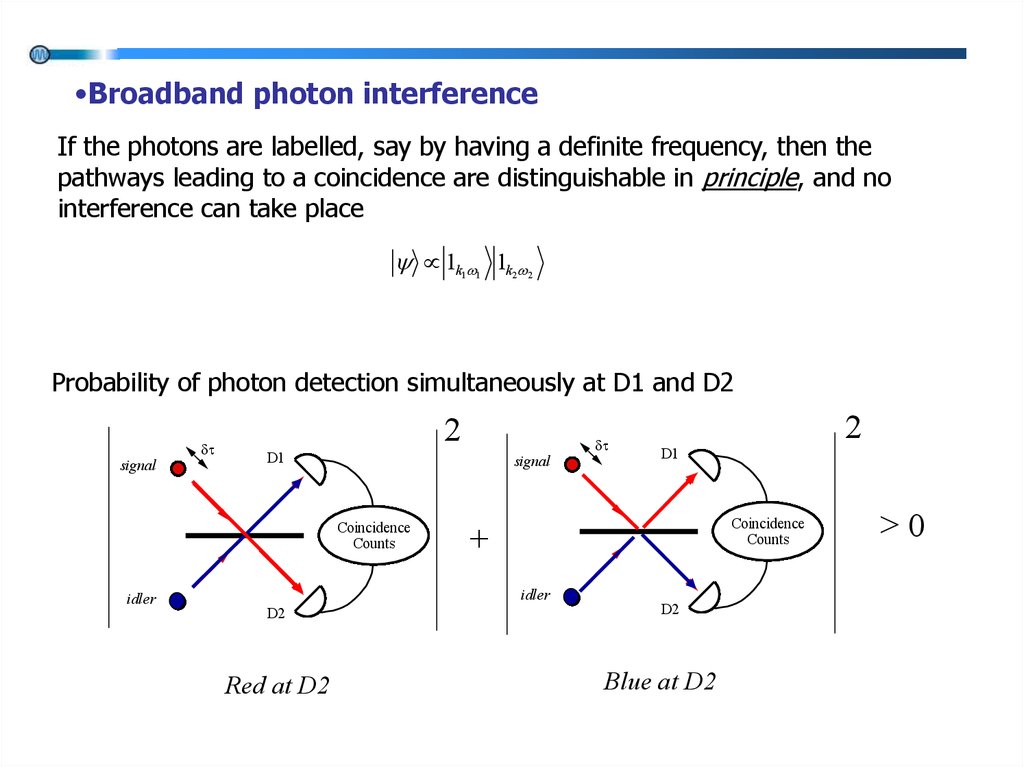
•Broadband photon interferenceIf the photons are labelled, say by having a definite frequency, then the
pathways leading to a coincidence are distinguishable in principle, and no
interference can take place
1k 1k
1 1
2
2
Probability of photon detection simultaneously at D1 and D2
signal
2
D1
signal
Coincidence
Counts
idler
Red at D2
2
D1
Coincidence
Counts
+
idler
D2
D2
Blue at D2
>0
8.
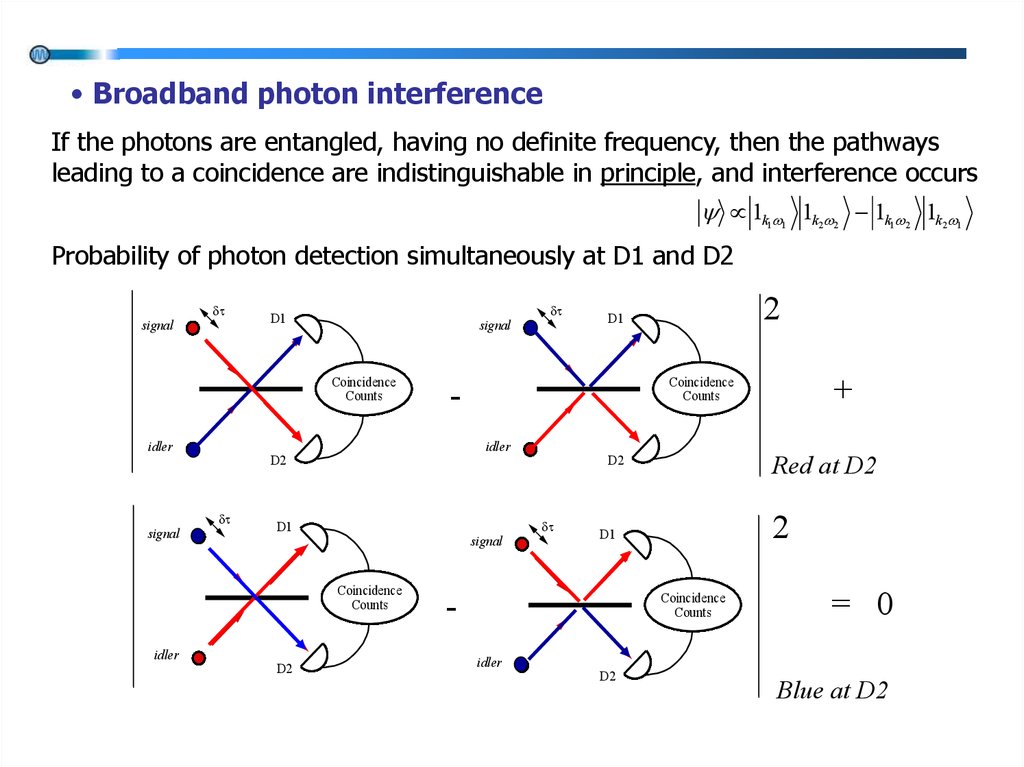
• Broadband photon interferenceIf the photons are entangled, having no definite frequency, then the pathways
leading to a coincidence are indistinguishable in principle, and interference occurs
1k 1k 1k 1k
1 1
2
2
1
Probability of photon detection simultaneously at D1 and D2
signal
D1
signal
Coincidence
Counts
idler
signal
signal
Coincidence
Counts
idler
D2
+
Coincidence
Counts
idler
D1
2
D1
-
D2
Red at D2
D2
2
D1
-
Coincidence
Counts
idler
D2
= 0
Blue at D2
2
2 1
9.
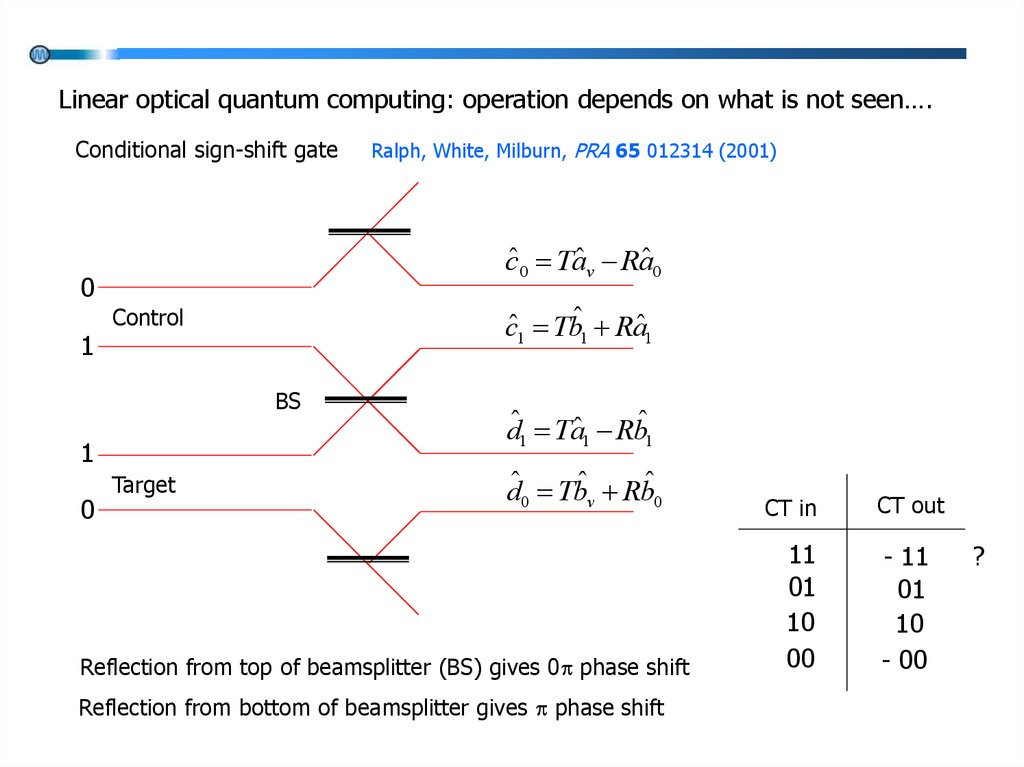
Linear optical quantum computing: operation depends on what is not seen….Conditional sign-shift gate
Ralph, White, Milburn, PRA 65 012314 (2001)
cˆ0 Taˆ v Raˆ 0
0
1
cˆ1 Tbˆ1 Raˆ1
Control
BS
1
0
dˆ1 Taˆ1 Rbˆ1
dˆ0 Tbˆv Rbˆ0
Target
Reflection from top of beamsplitter (BS) gives 0p phase shift
Reflection from bottom of beamsplitter gives p phase shift
CT in
11
01
10
00
CT out
- 11
01
10
- 00
?
10.
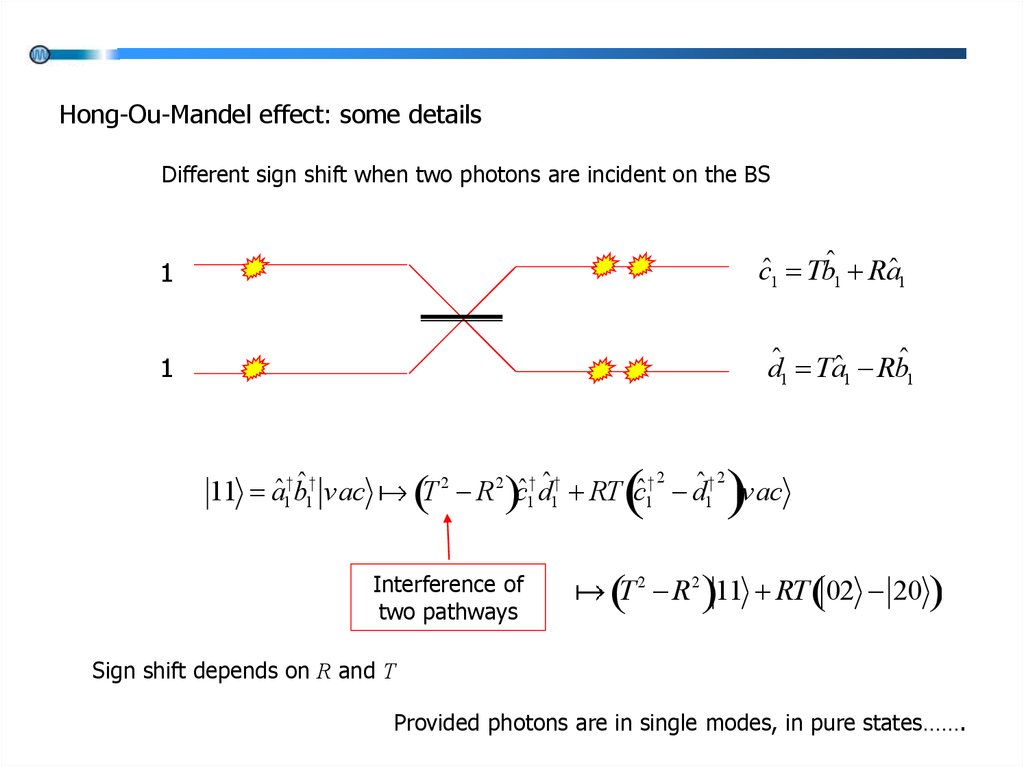
Hong-Ou-Mandel effect: some detailsDifferent sign shift when two photons are incident on the BS
cˆ1 Tbˆ1 Raˆ1
1
1
2
2
† ˆ†
†2
†2
ˆ
ˆ
ˆ
T
R
c
d
RT
c
d
vac
1 1
1
1
11 aˆ1†bˆ1† vac
dˆ1 Taˆ1 Rbˆ1
Interference of
two pathways
T
2
R2 11 RT 02 20
Sign shift depends on R and T
photons are in single modes, in pure states…….
Provided
11.
• Continuous variables for single photonsReduced noise
• Efficient generation of Fock states
• Testing sub-Poissonian photon number fluctuations
• Increased correlations: Engineered space-time entanglement
• Application: single-photon CV QKD
12.
Spontaneous emission from single “atoms” generates single photonssingle photon
detector, A
50/50 beam-splitter
device
emission
mesa
aperture
single
photon
detector, B
ncontact
time interval
analyser
Correlation
(ii) conventional LED
p-contact
single photon emission
electron
injector
Start
Stop
quantum dot layer
0
(i) single photon LED
2-photon probability
n-contact
p-contact
0
insulator
-40
hole injector
substrate/buffer
-20
0
20
40
Delay (ns)
A. Shields et al., Science 295, 102 (2002)
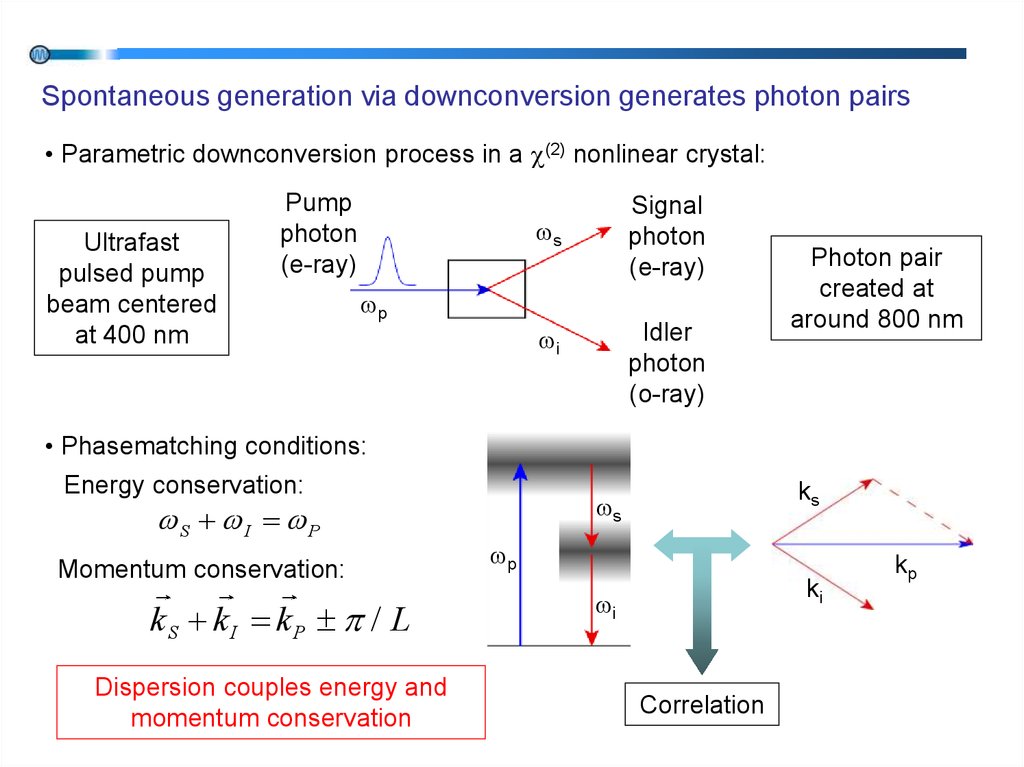
13. Spontaneous generation via downconversion generates photon pairs
• Parametric downconversion process in a c(2) nonlinear crystal:Ultrafast
pulsed pump
beam centered
at 400 nm
Pump
photon
(e-ray)
Signal
photon
(e-ray)
s
p
i
Idler
photon
(o-ray)
Photon pair
created at
around 800 nm
• Phasematching conditions:
Energy conservation:
S I P
Momentum conservation:
kS kI kP p / L
Dispersion couples energy and
momentum conservation
ks
s
p
ki
i
Correlation
kp
14.
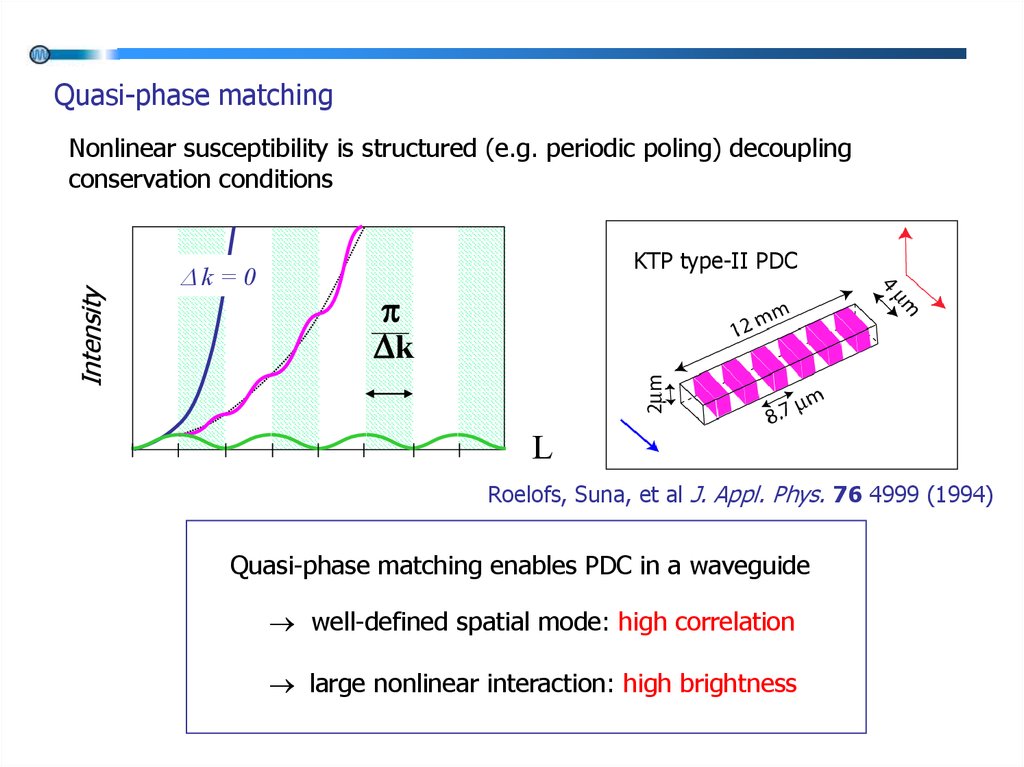
Quasi-phase matchingIntensity
Nonlinear susceptibility is structured (e.g. periodic poling) decoupling
conservation conditions
k = 0
KTP type-II PDC
p
k
L
Roelofs, Suna, et al J. Appl. Phys. 76 4999 (1994)
Quasi-phase matching enables PDC in a waveguide
well-defined spatial mode: high correlation
large nonlinear interaction: high brightness
15.
Experimental apparatus: fs PDC in KTP T-II waveguideBlue pump
Power: 2mW
PDC
30kHz coinc. rate
KTP waveguide
16.
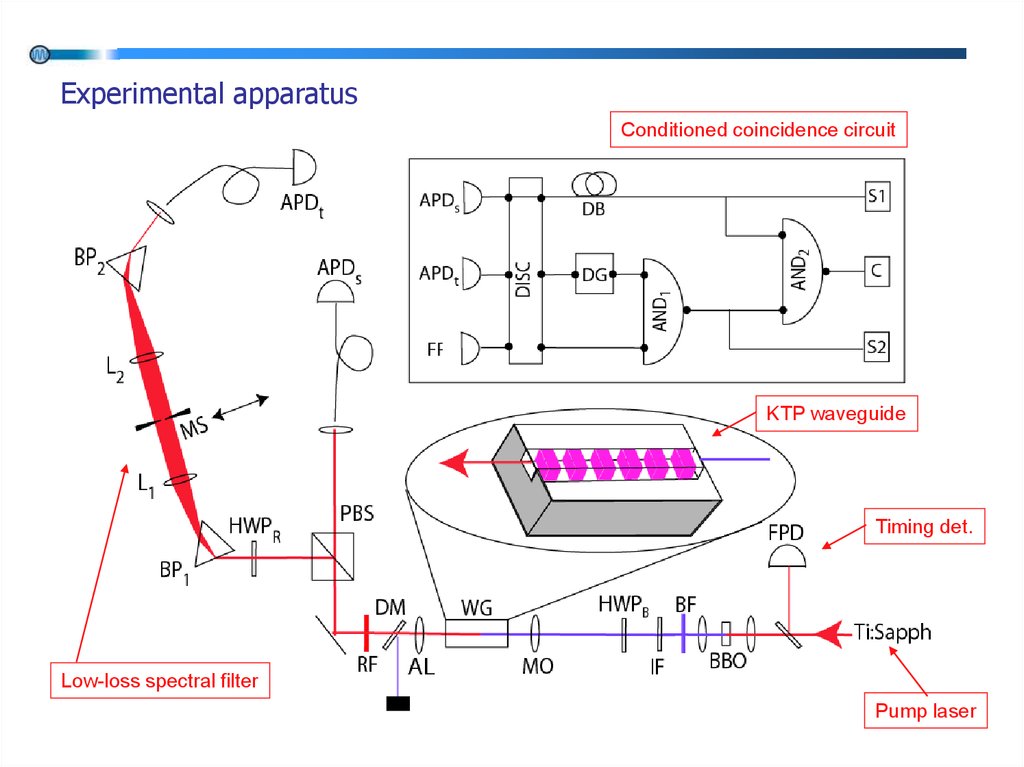
Experimental apparatusConditioned coincidence circuit
KTP waveguide
Timing det.
Low-loss spectral filter
Pump laser
17.
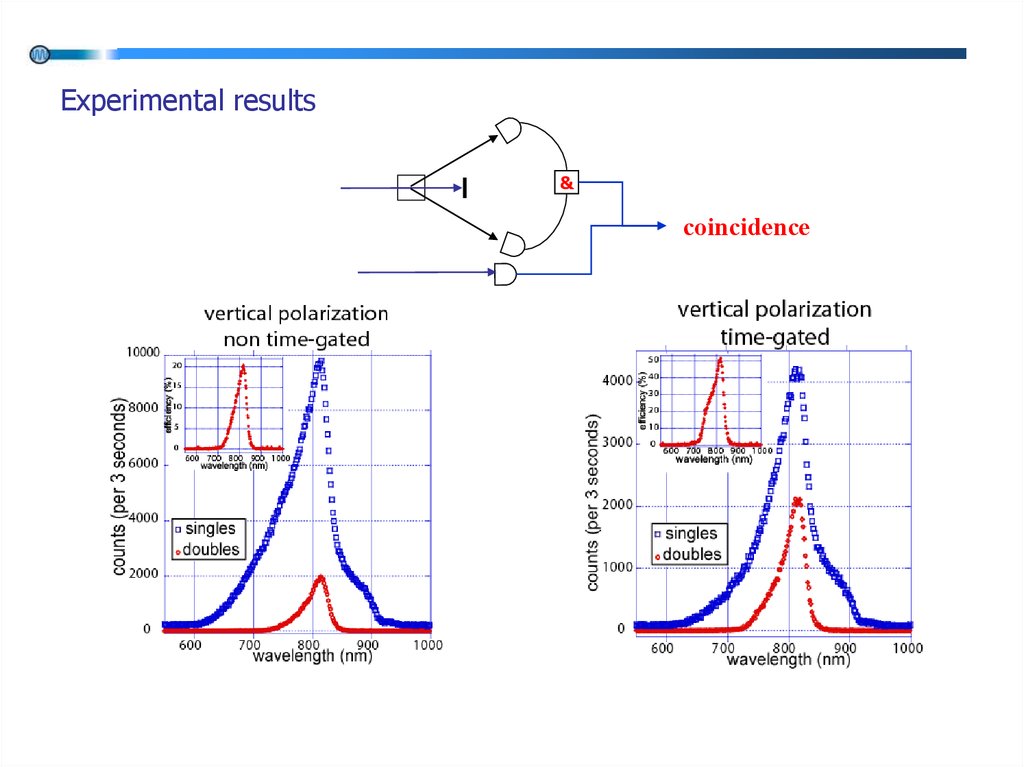
Experimental results&
coincidence
18.
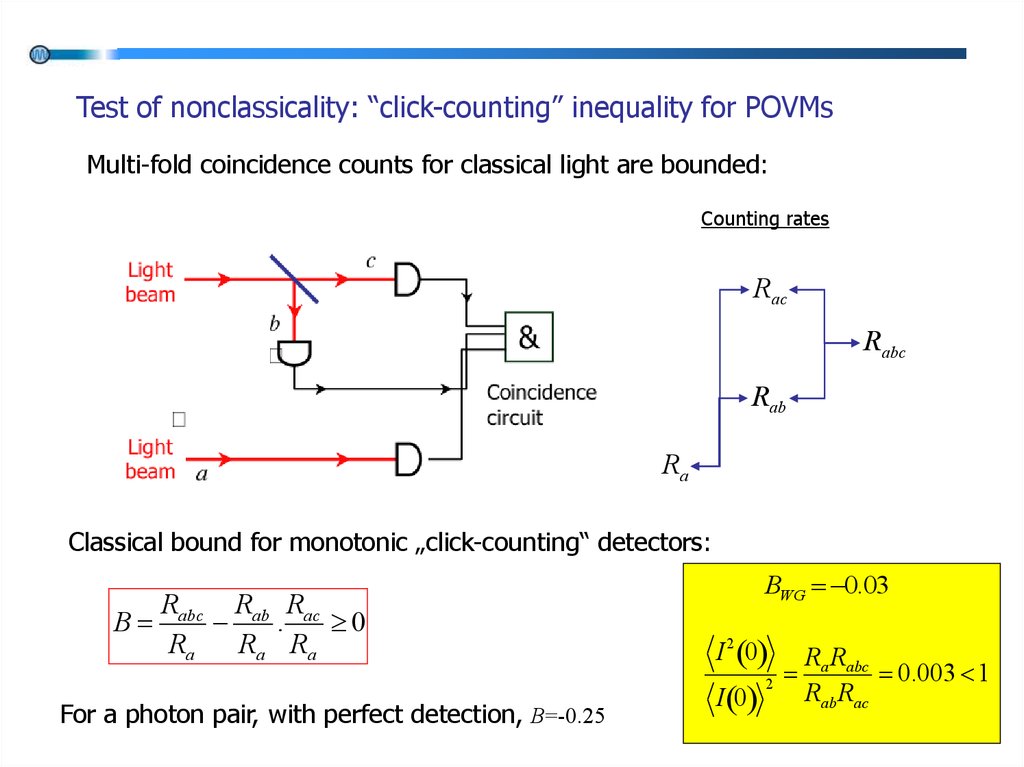
Test of nonclassicality: “click-counting” inequality for POVMsMulti-fold coincidence counts for classical light are bounded:
Counting rates
Rac
Rabc
Rab
Ra
Classical bound for monotonic „click-counting“ detectors:
R
R R
B abc ab . ac 0
Ra
Ra Ra
BWG 0.03
For a photon pair, with perfect detection, B=-0.25
I 2 0
I 0
2
Ra Rabc
0.003 1
Rab Rac
19.
N-photon generationGenerate photons in correlated beams, and use the detection of n in one
beam to herald the presence of n in the other.
Concatentation of sources requires pulsed pump
Pulsed blue light
trigger if n
1
filter
1
C.K. Hong and L. Mandel, Phys. Rev. Lett. 56, 58 (1986)
More recently, twin beams developed by Kumar, Raymer..
20.
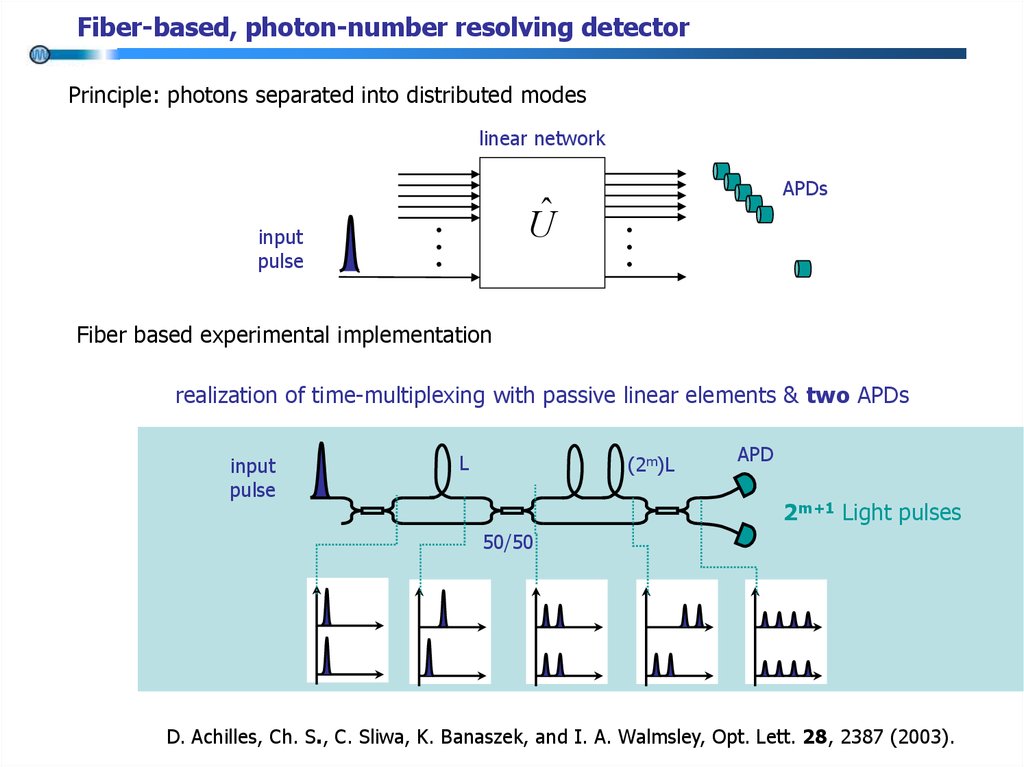
Fiber-based, photon-number resolving detectorPrinciple: photons separated into distributed modes
linear network
input
pulse
Uˆ
APDs
Fiber based experimental implementation
realization of time-multiplexing with passive linear elements & two APDs
input
pulse
L
(2m)L
APD
2m+1 Light pulses
50/50
D. Achilles, Ch. S., C. Sliwa, K. Banaszek, and I. A. Walmsley, Opt. Lett. 28, 2387 (2003).
21.
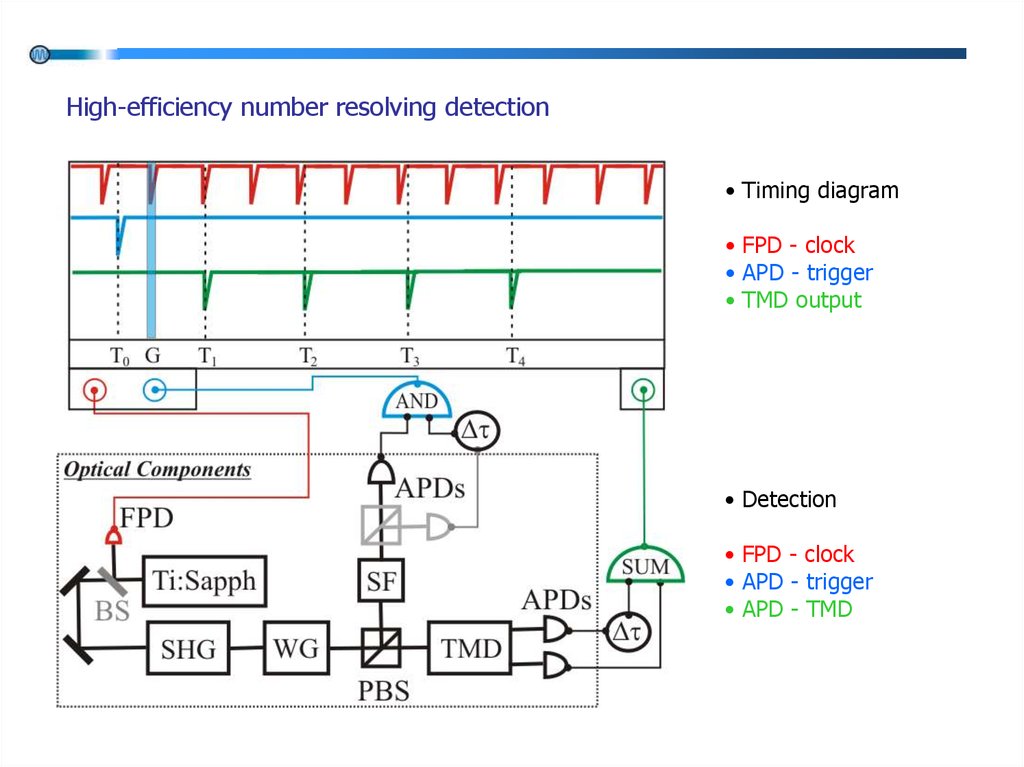
High-efficiency number resolving detection• Timing diagram
• FPD - clock
• APD - trigger
• TMD output
• Detection
• FPD - clock
• APD - trigger
• APD - TMD
22.
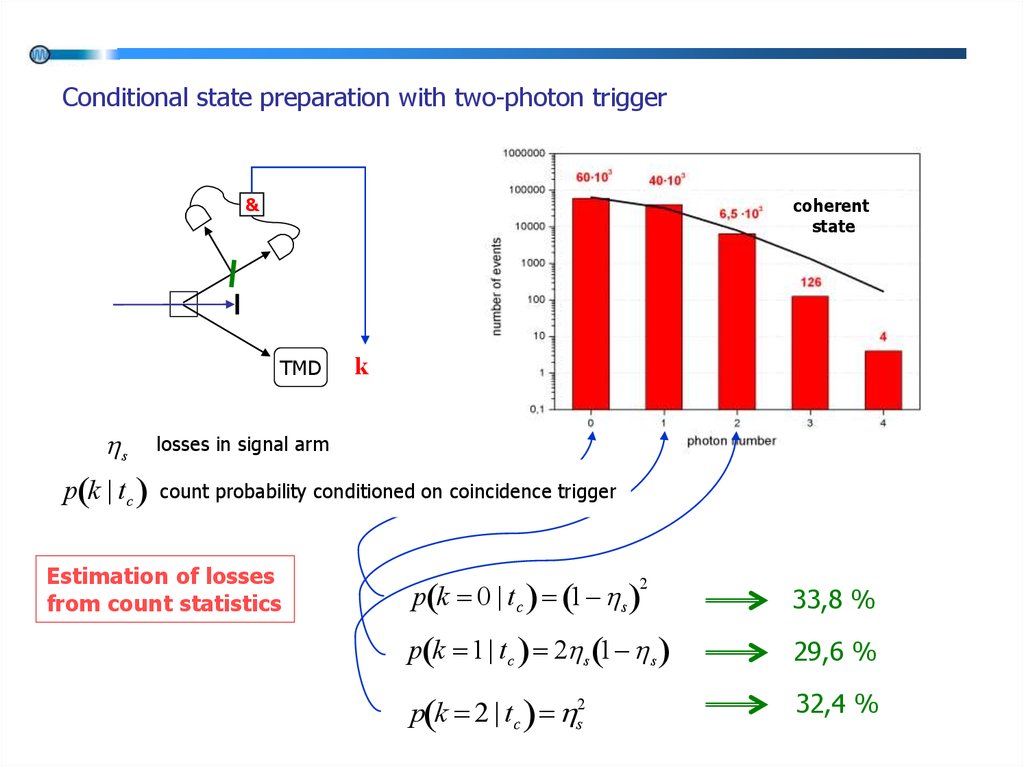
Conditional state preparation with two-photon trigger&
coherent
state
TMD
s
p k | t c
k
losses in signal arm
count probability conditioned on coincidence trigger
Estimation of losses
from count statistics
p k 0 | t c 1 s
33,8 %
p k 1 | tc 2 s 1 s
29,6 %
p k 2 | tc s2
32,4 %
2
23.
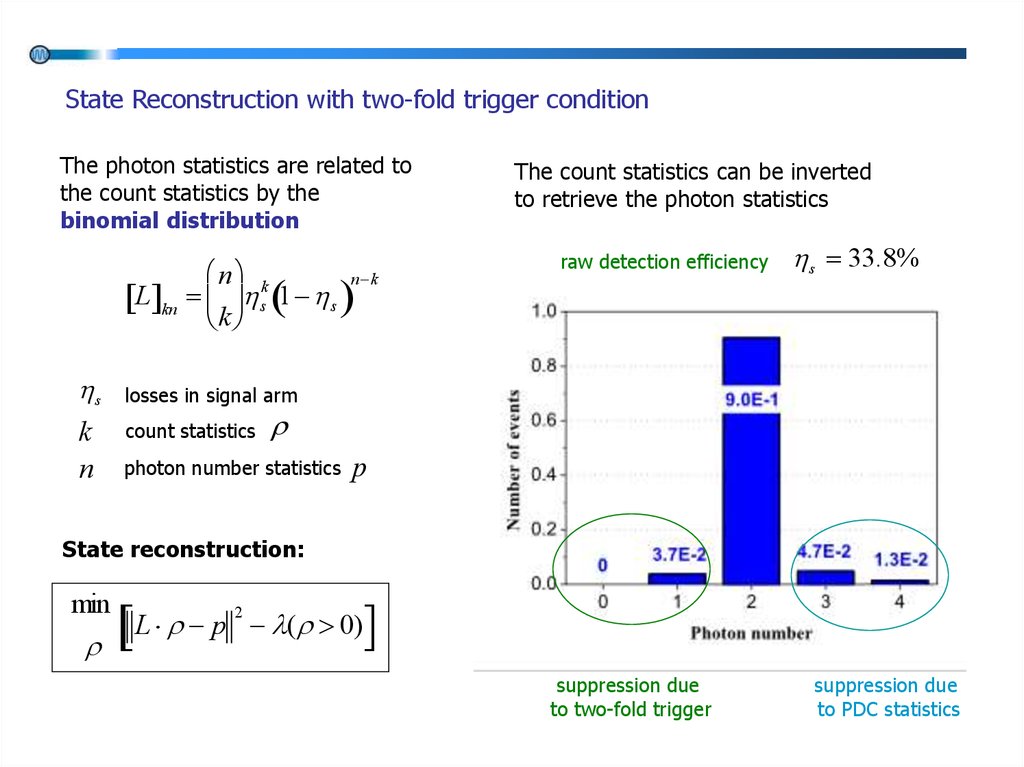
State Reconstruction with two-fold trigger conditionThe photon statistics are related to
the count statistics by the
binomial distribution
L kn
s
k
n
n k
n k
s 1 s
k
raw detection efficiency
s 33.8%
losses in signal arm
count statistics
The count statistics can be inverted
to retrieve the photon statistics
photon number statistics
p
State reconstruction:
min
L p ( 0)
2
suppression due
to two-fold trigger
suppression due
to PDC statistics
24.
• Continuous variables for single photons• Reduced noise: Fock states
Increased correlations:
Engineering space-time
entanglement
• Entanglement and pure state generation
• Engineering entanglement in PDC
• Application: single-photon CV QKD
25.
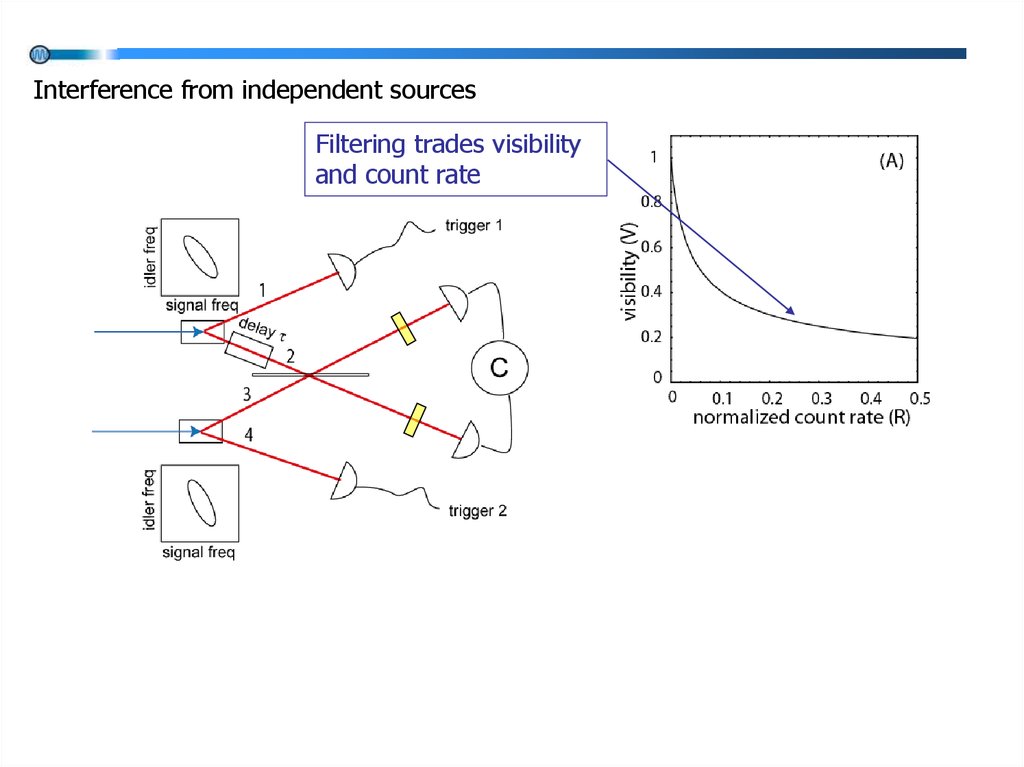
Interference from independent sourcesFiltering trades visibility
and count rate
26.
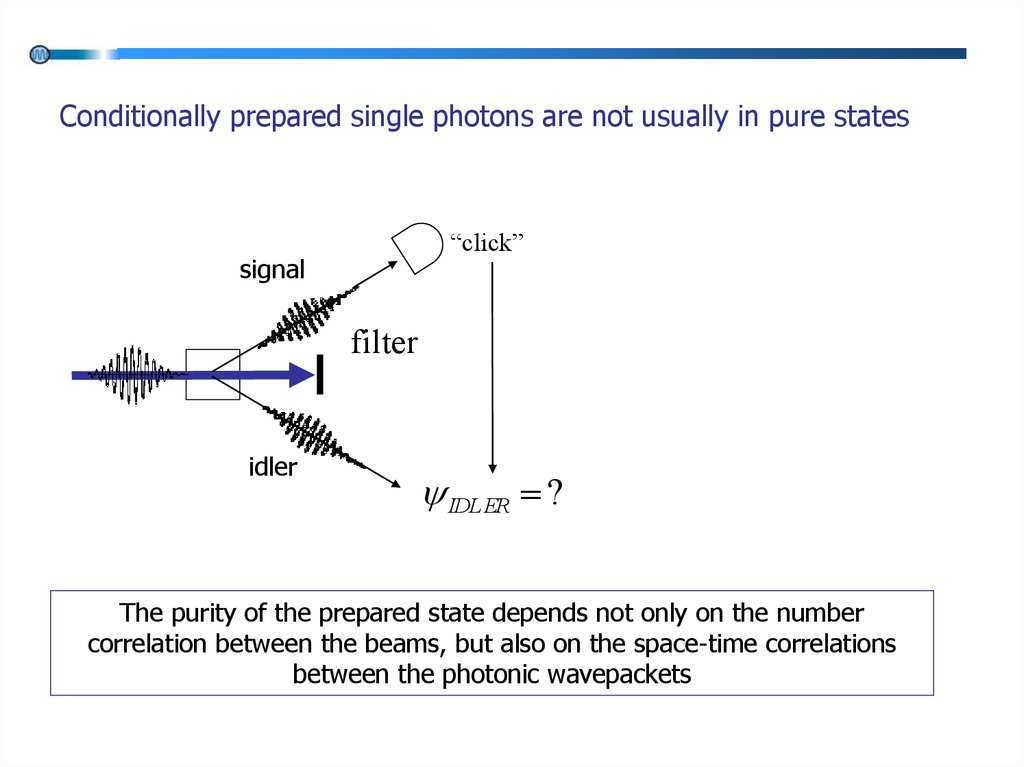
Conditionally prepared single photons are not usually in pure states“click”
signal
filter
idler
IDL ER ?
The purity of the prepared state depends not only on the number
correlation between the beams, but also on the space-time correlations
between the photonic wavepackets
27.
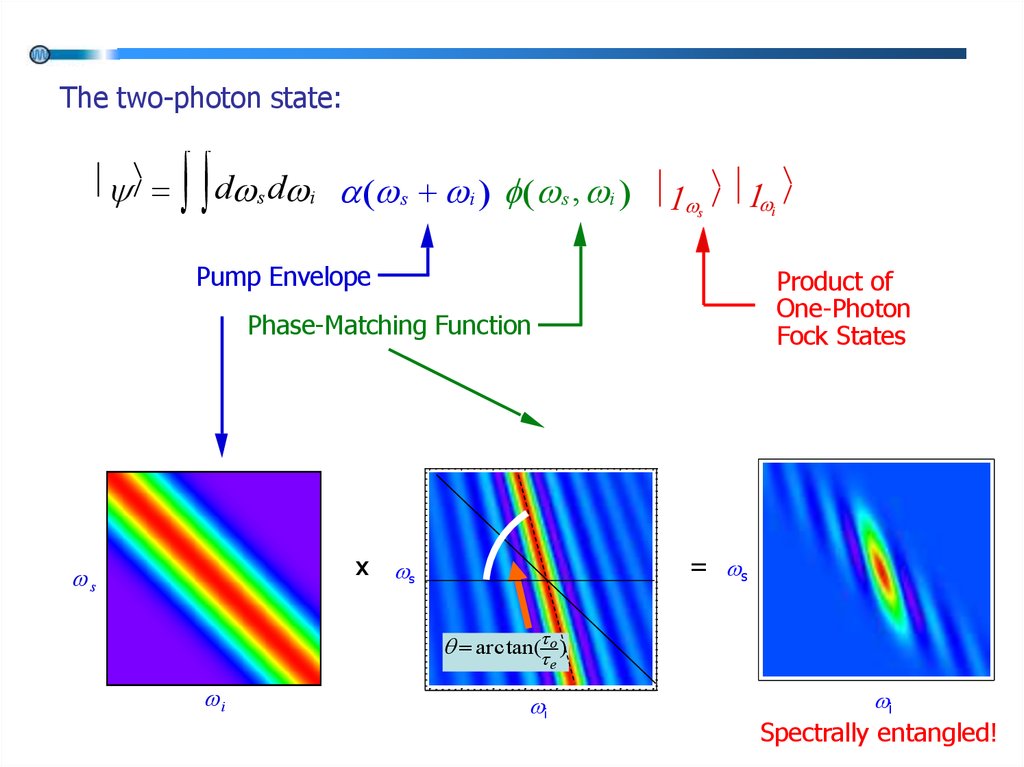
The two-photon state:d d
s
i
s i f s , i 1
s
1
Pump Envelope
i
Product of
One-Photon
Fock States
Phase-Matching Function
2.5
2.45
2.4
x
s
= s
s
2.35
2.3
2.25
2.2
i
2.15
2.15
arctan( o )
e
2.2
2.25
i
2.3
2.35
2.4
2.45
2.5
i
Spectrally entangled!
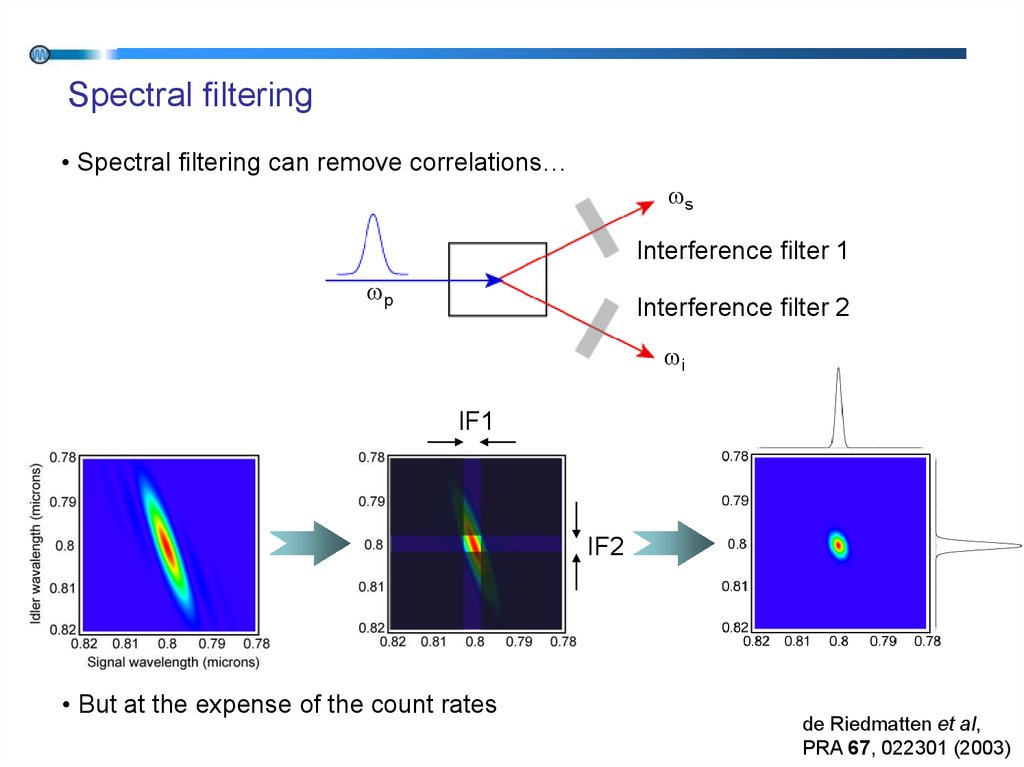
28. Spectral filtering
• Spectral filtering can remove correlations…s
Interference filter 1
p
Interference filter 2
i
IF1
IF2
• But at the expense of the count rates
de Riedmatten et al,
PRA 67, 022301 (2003)
29.
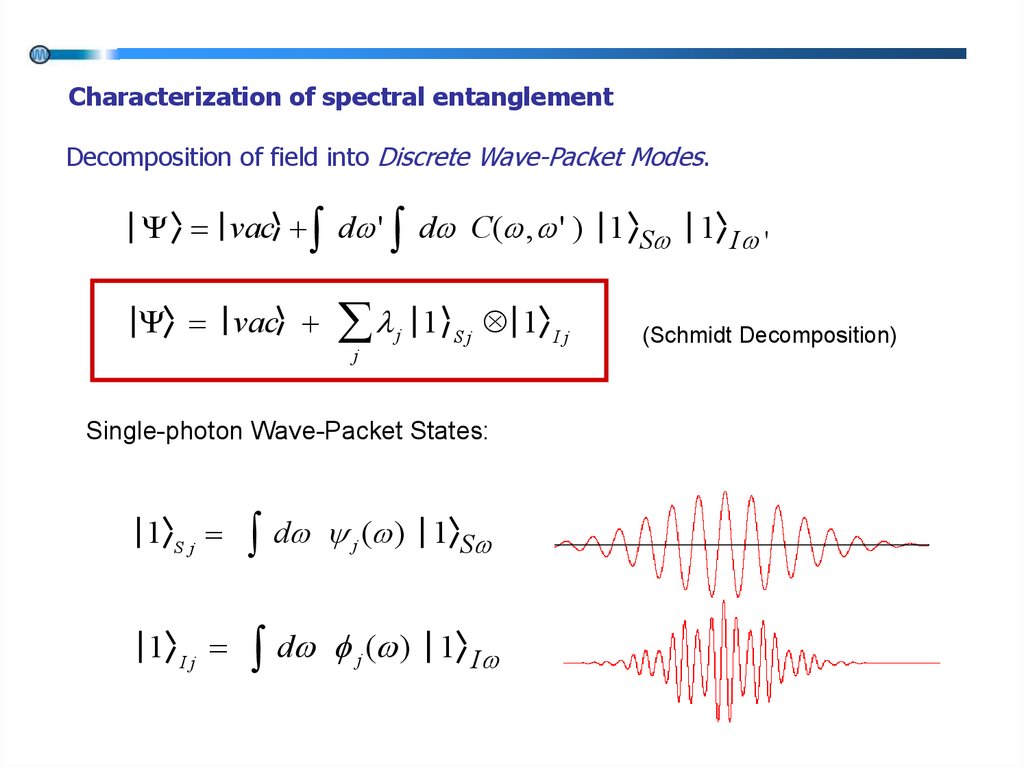
Characterization of spectral entanglementDecomposition of field into Discrete Wave-Packet Modes.
vac d ' d C( , ' ) 1 S 1 I '
vac
j
j
1
Sj
1
Single-photon Wave-Packet States:
1
Sj
d ( )
1
Ij
d
j
1 S
f j ( ) 1 I
Ij
(Schmidt Decomposition)
30.
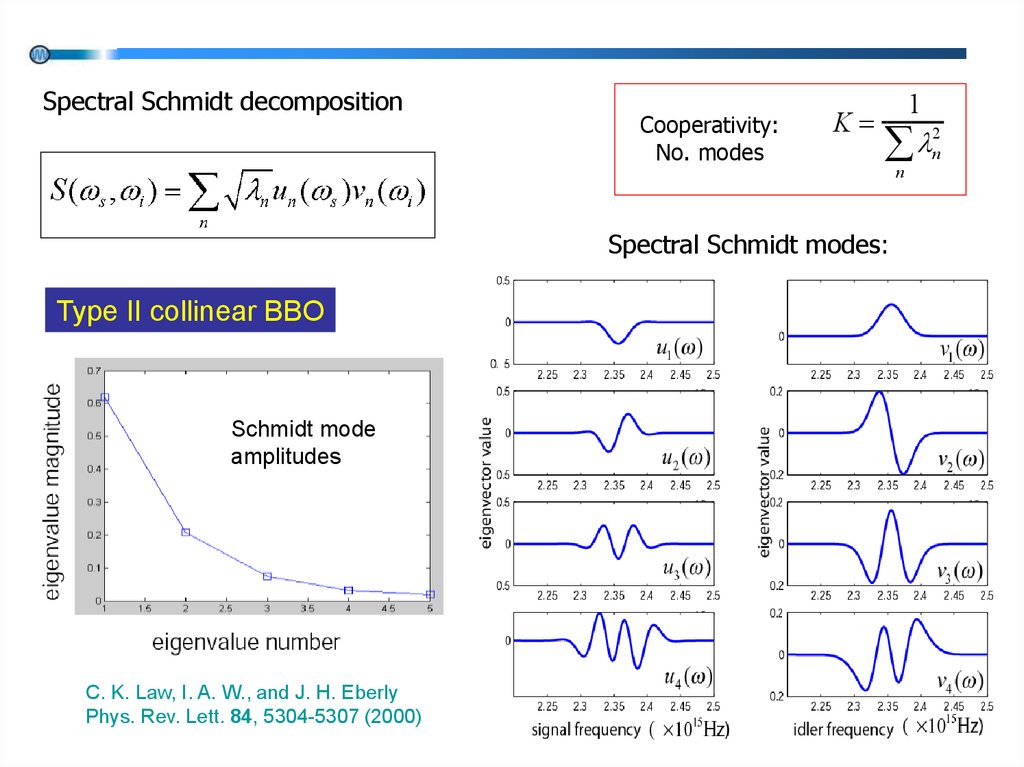
Spectral Schmidt decompositionCooperativity:
No. modes
K
Spectral Schmidt modes:
Type II collinear BBO
Schmidt mode
amplitudes
C. K. Law, I. A. W., and J. H. Eberly
Phys. Rev. Lett. 84, 5304-5307 (2000)
1
n
2
n
31.
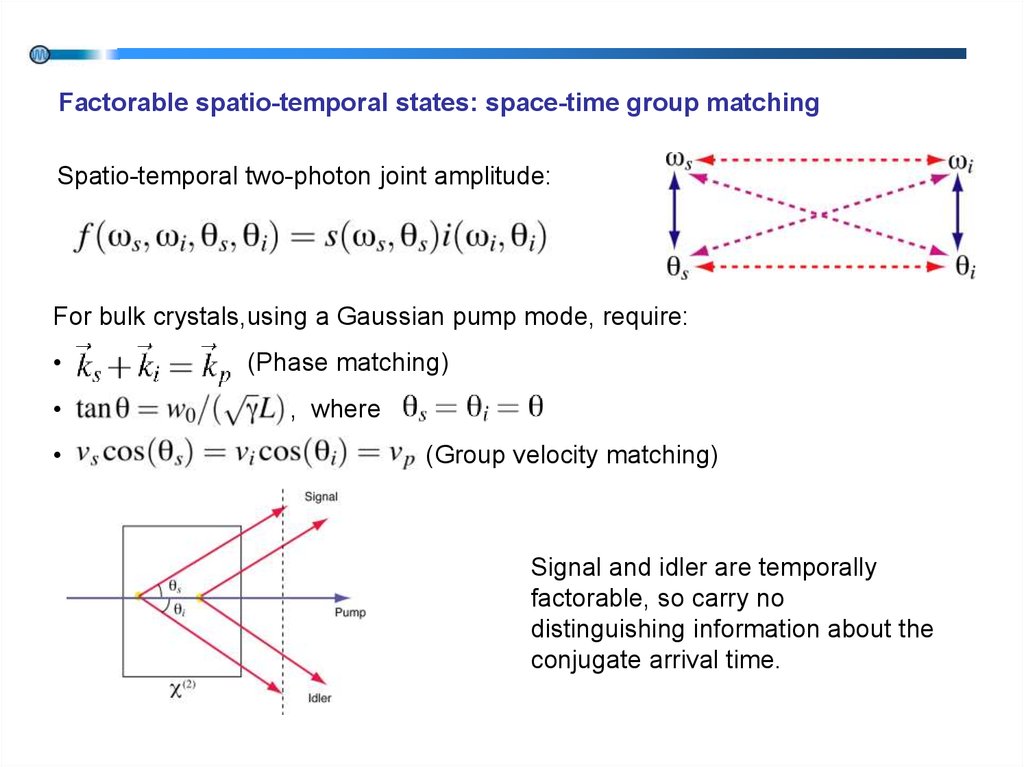
Factorable spatio-temporal states: space-time group matchingSpatio-temporal two-photon joint amplitude:
For bulk crystals,using a Gaussian pump mode, require:
(Phase matching)
, where
(Group velocity matching)
Signal and idler are temporally
factorable, so carry no
distinguishing information about the
conjugate arrival time.
32.
Example: Binary entanglementL
Controlling the number of Schmidt modes.
By:
•Suppressing the degenerate mode
and
•Balancing the crystal length and
the beam waist diameter
w0
….can isolate one
non-degenerate pair.
Transverse
momentum
contribution
i
Longitudinal
momentum
contribution
i
i
=
s
s
s
33.
Pure state generation using heralding: source engineering requiredSignal in a pure state if f s1 , i1 s1 m i1
This can be achieved by group delay matching.
The pump wavelength,
bandwidth and spectra phase, the parameters
of the crystal material, and in the case of quasi-phasematching the poling
period can be chosen, such that the joint spectral amplitude factors.
Asymmetric (Grice,U’Ren & IAW,PRA (2001))
Symmetric (Keller & Rubin, PRA,1997)
v p vs
Ultrafast pump pulse:
v v
vp s i
2
o-photon matched to pump
Very broad band (20 fs)
s
s
Very precise timing
e - photon
Narrow band (10 ps)
Very precise timing
• BBO @ 800 nm
i
K=1.001, pure photons, no timing jitter
• KD*P @ 405 nm
i
34.
Interference from independent engineered sourcesFiltering trades visibility
and count rate
f12 ( 1 , 2 )
f34 ( 3 , 4 )
Engineering sources to have K=1
leads to unit visibility without
compromising count rate
35.
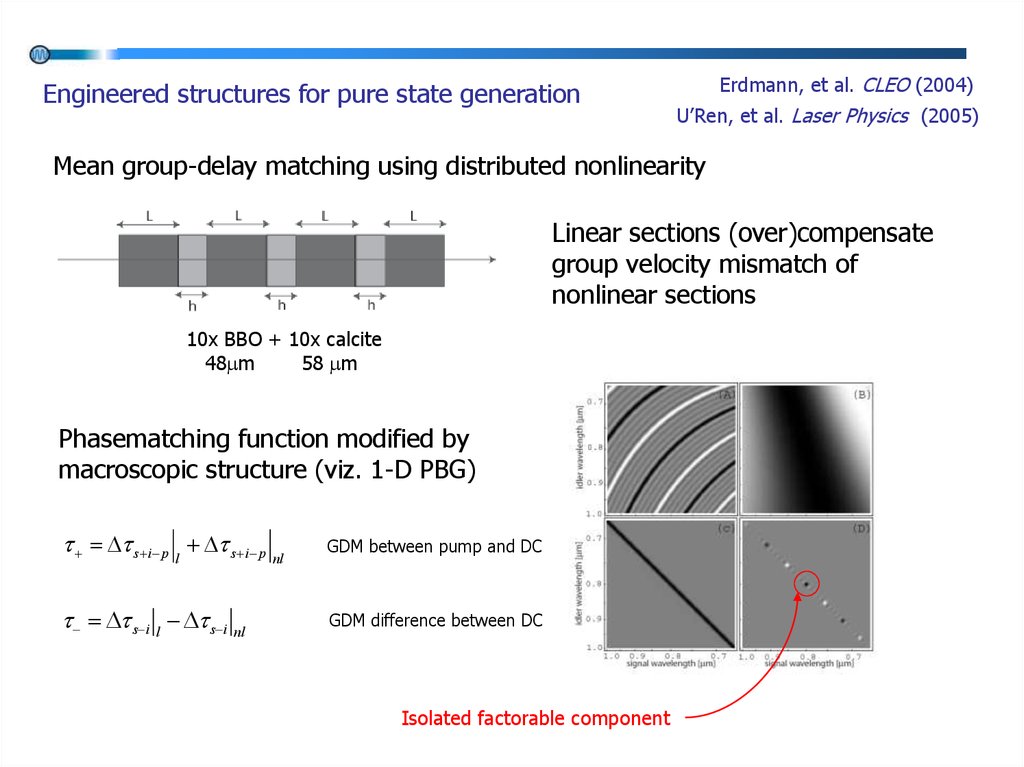
Engineered structures for pure state generationErdmann, et al. CLEO (2004)
U’Ren, et al. Laser Physics (2005)
Mean group-delay matching using distributed nonlinearity
Linear sections (over)compensate
group velocity mismatch of
nonlinear sections
10x BBO + 10x calcite
48mm
58 mm
Phasematching function modified by
macroscopic structure (viz. 1-D PBG)
s i p l s i p nl
GDM between pump and DC
s i l s i nl
GDM difference between DC
Isolated factorable component
36.
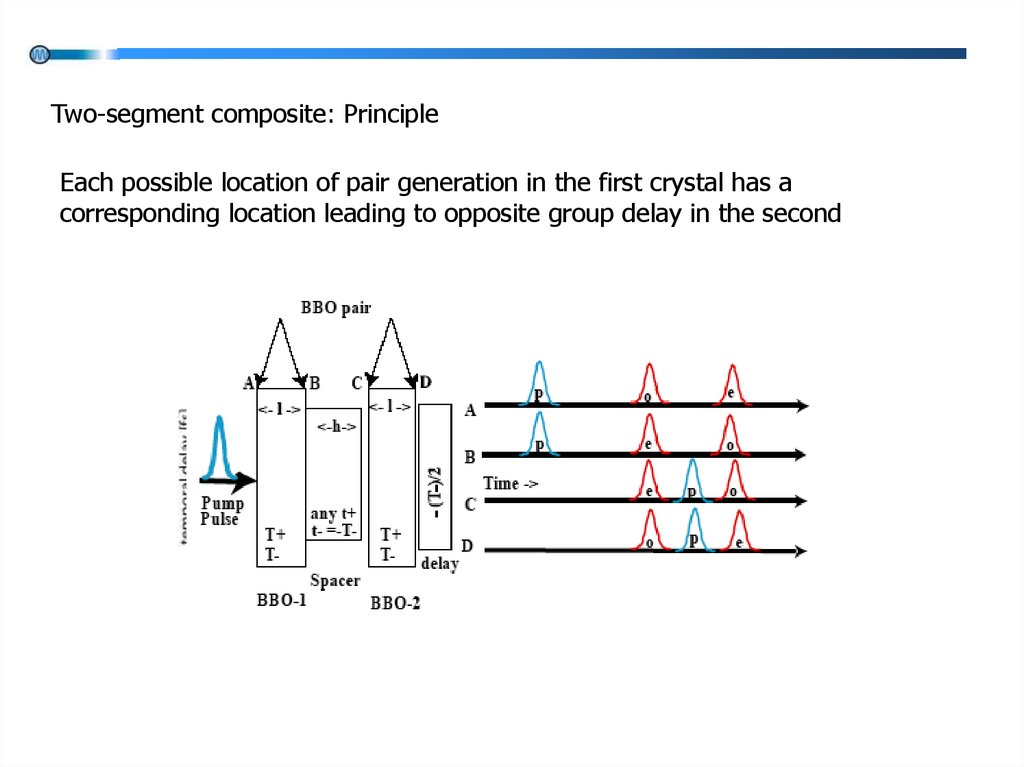
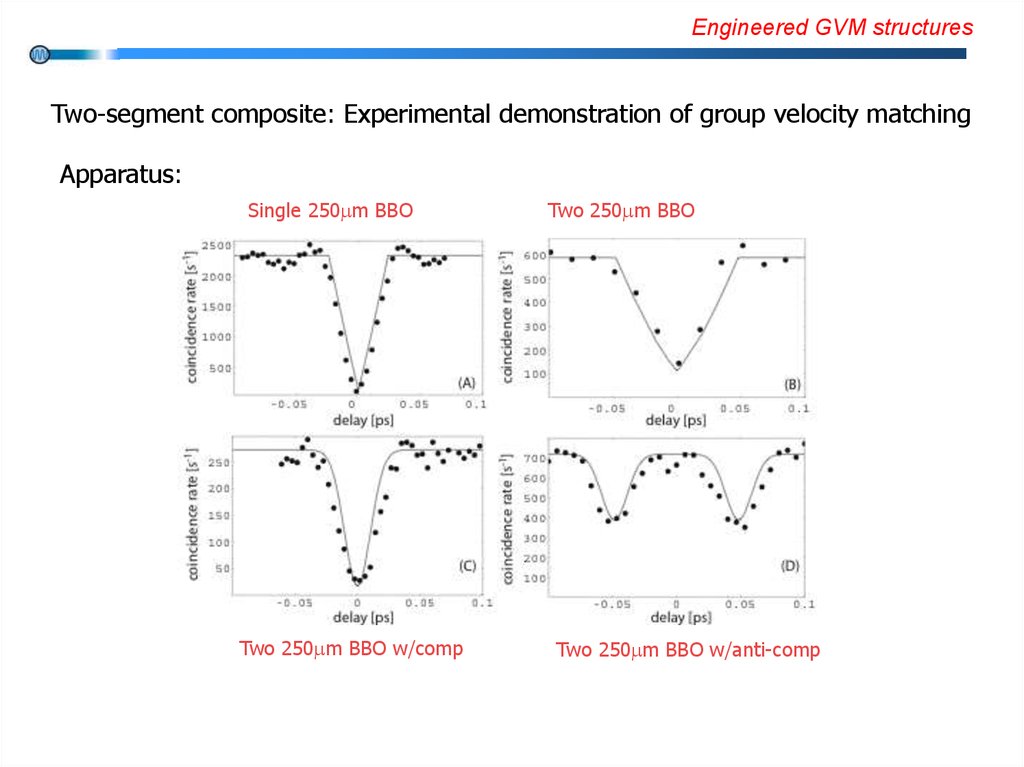
Two-segment composite: PrincipleEach possible location of pair generation in the first crystal has a
corresponding location leading to opposite group delay in the second
37.
Engineered GVM structuresTwo-segment composite: Experimental demonstration of group velocity matching
Apparatus:
Single 250mm BBO
Two 250mm BBO w/comp
Two 250mm BBO
Two 250mm BBO w/anti-comp
38.
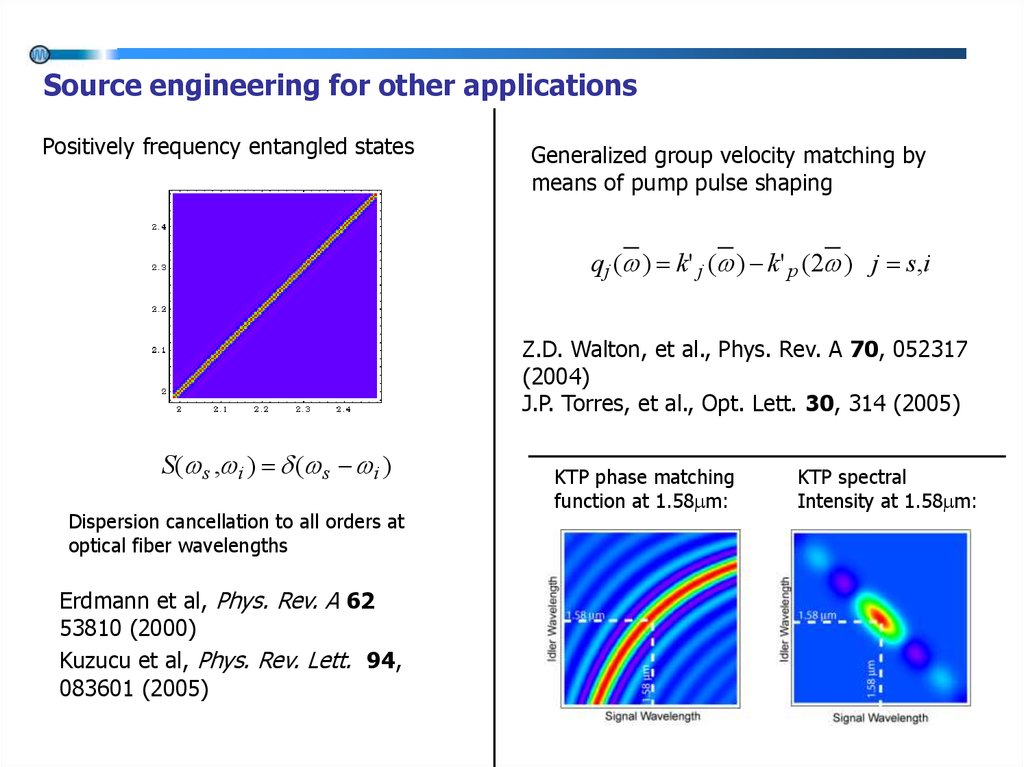
Source engineering for other applicationsPositively frequency entangled states
Generalized group velocity matching by
means of pump pulse shaping
q j ( ) k' j ( ) k' p (2 ) j s,i
Z.D. Walton, et al., Phys. Rev. A 70, 052317
(2004)
J.P. Torres, et al., Opt. Lett. 30, 314 (2005)
S( s , i ) ( s i )
Dispersion cancellation to all orders at
optical fiber wavelengths
Erdmann et al, Phys. Rev. A 62
53810 (2000)
Kuzucu et al, Phys. Rev. Lett. 94,
083601 (2005)
KTP phase matching
function at 1.58mm:
KTP spectral
Intensity at 1.58mm:
39.
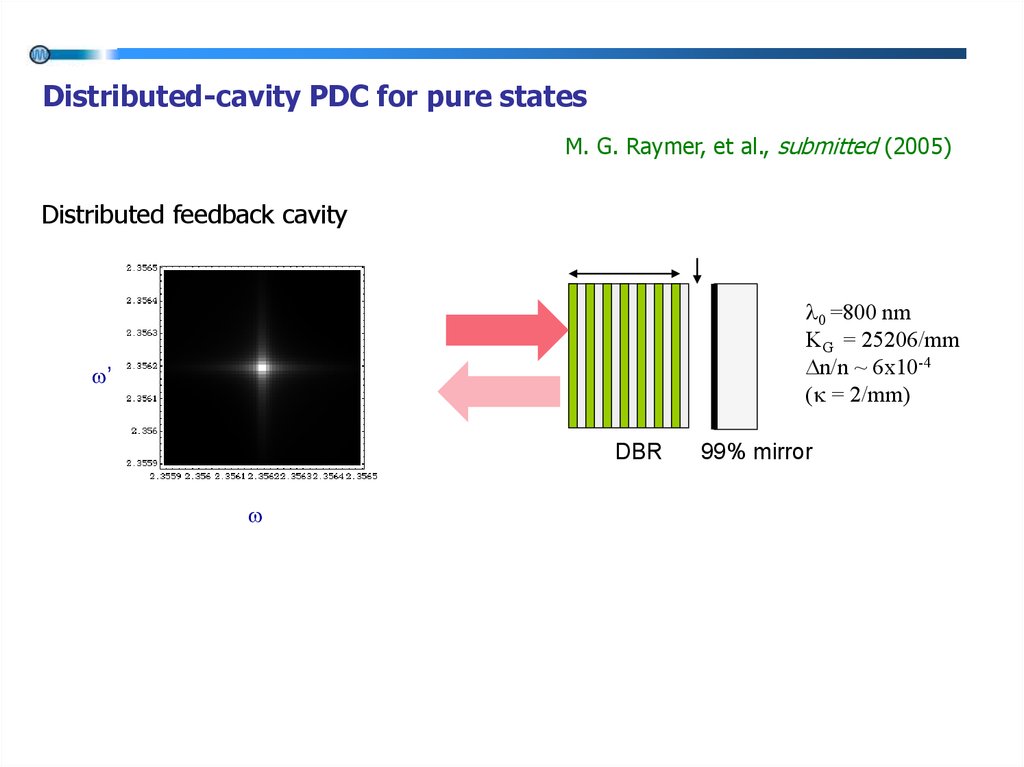
Distributed-cavity PDC for pure statesM. G. Raymer, et al., submitted (2005)
Distributed feedback cavity
0 =800 nm
KG = 25206/mm
n/n ~ 6x10-4
(k = 2/mm)
’
DBR
99% mirror
40.
• Continuous variables for single photons• Reduced noise: Fock states
• Increased correlations: Engineered space-time entanglement
Application: QKD using single photon
continuous variables
• Spatial entanglement and CV QKD
• Mutual information and eavesdropping
41.
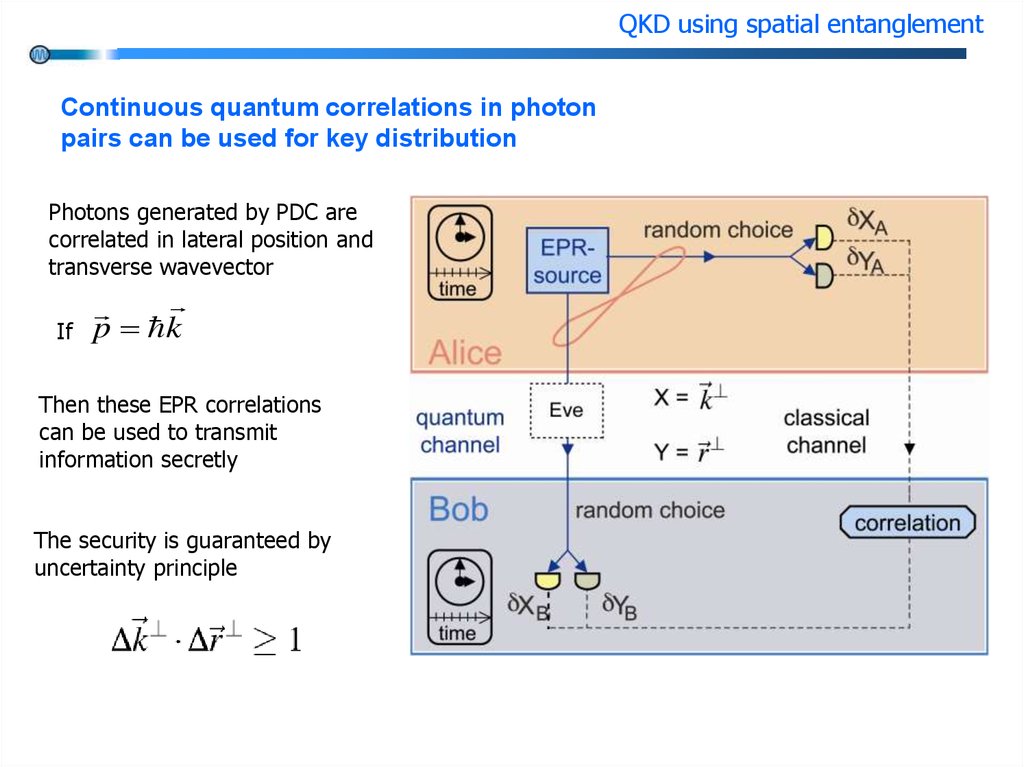
QKD using spatial entanglementContinuous quantum correlations in photon
pairs can be used for key distribution
Photons generated by PDC are
correlated in lateral position and
transverse wavevector
If
p k
Then these EPR correlations
can be used to transmit
information secretly
The security is guaranteed by
uncertainty principle
42.
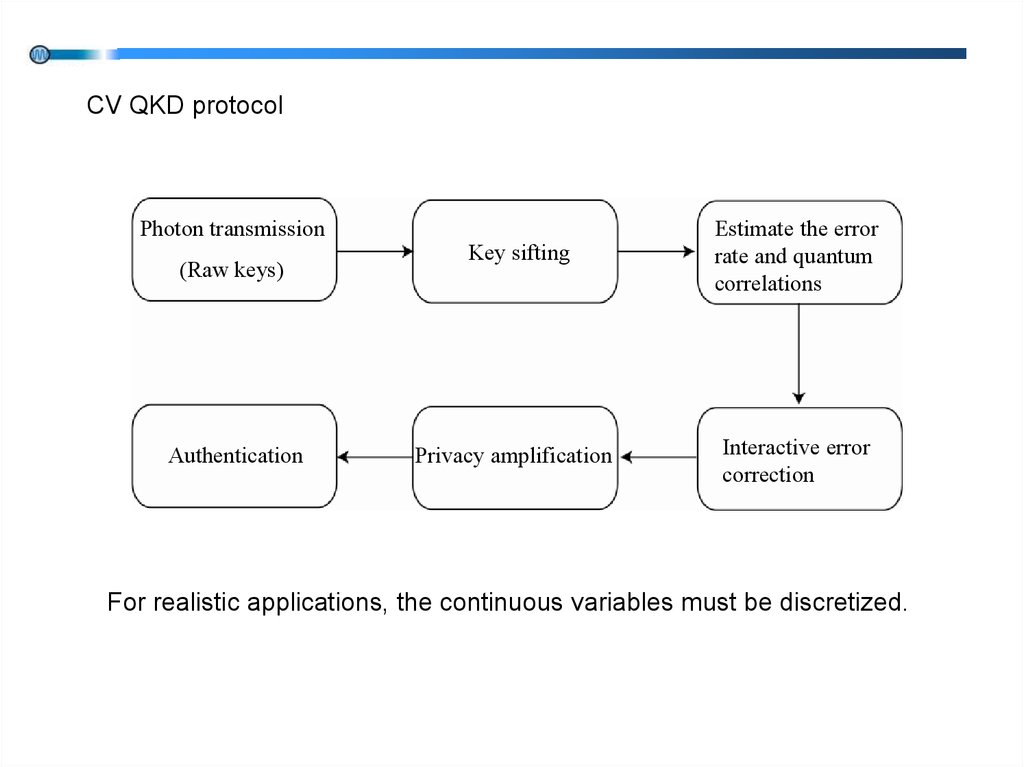
CV QKD protocolPhoton transmission
(Raw keys)
Authentication
Key sifting
Estimate the error
rate and quantum
correlations
Privacy amplification
Interactive error
correction
For realistic applications, the continuous variables must be discretized.
43.
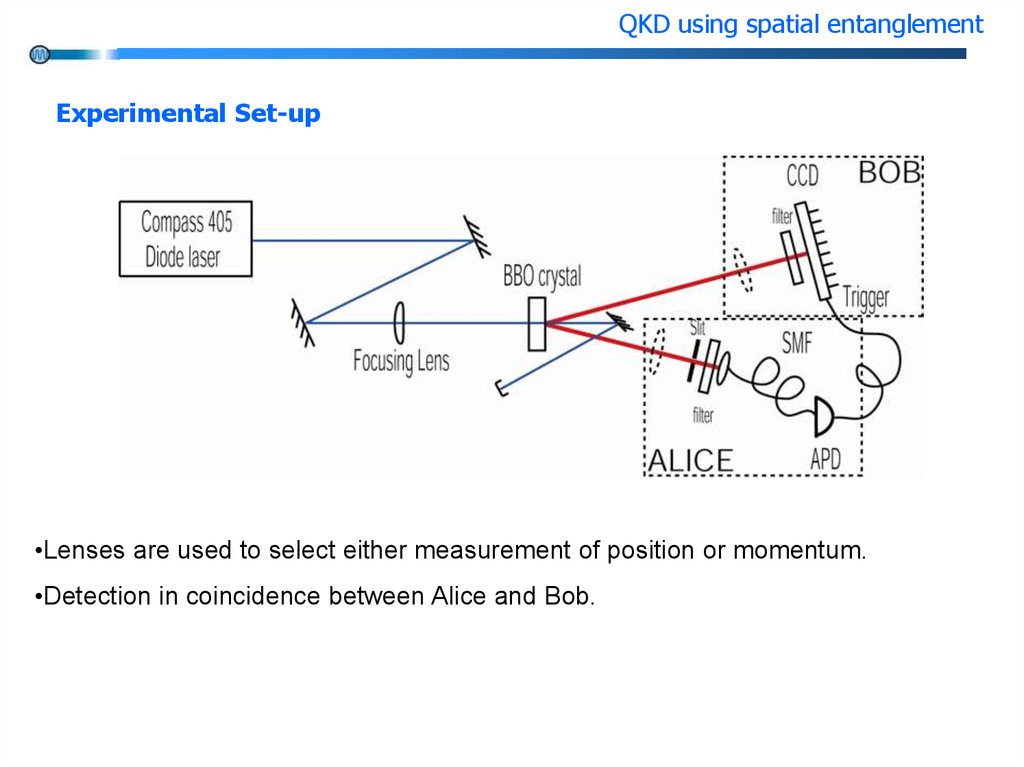
QKD using spatial entanglementExperimental Set-up
•Lenses are used to select either measurement of position or momentum.
•Detection in coincidence between Alice and Bob.
44.
QKD using spatial entanglementMutual information analysis
• Since the Hilbert space of the photonic degree of freedom is large, we can expect to
transmit more than one bit per photon
• For actual PDC sources, the mutual information per photon pair is determined by the
length of the crystal Lz and the spot size of the pump w0
45.
QKD using spatial entanglementEavesdropping: Intercept and resend strategy
Eve intercepts the photon sent to Bob, measures the position or the momentum, prepares
another photon and resends it to Bob. The state of the photons Eve resends (eigenstate,
squeezing state, etc) will affect the security of the system.
Fraction of photons sent by Alice to Bob that
are intercepted by Eve
(a) Mutual information between Alice and Bob
when Eve resends position eigenstate I AB
(b)
I AB when Eve resends the ‘optimal’ state
(c) Mutual information between Alice and Eve
I AE
To extract a secure key, it is sufficient that
I AB I AE
46.
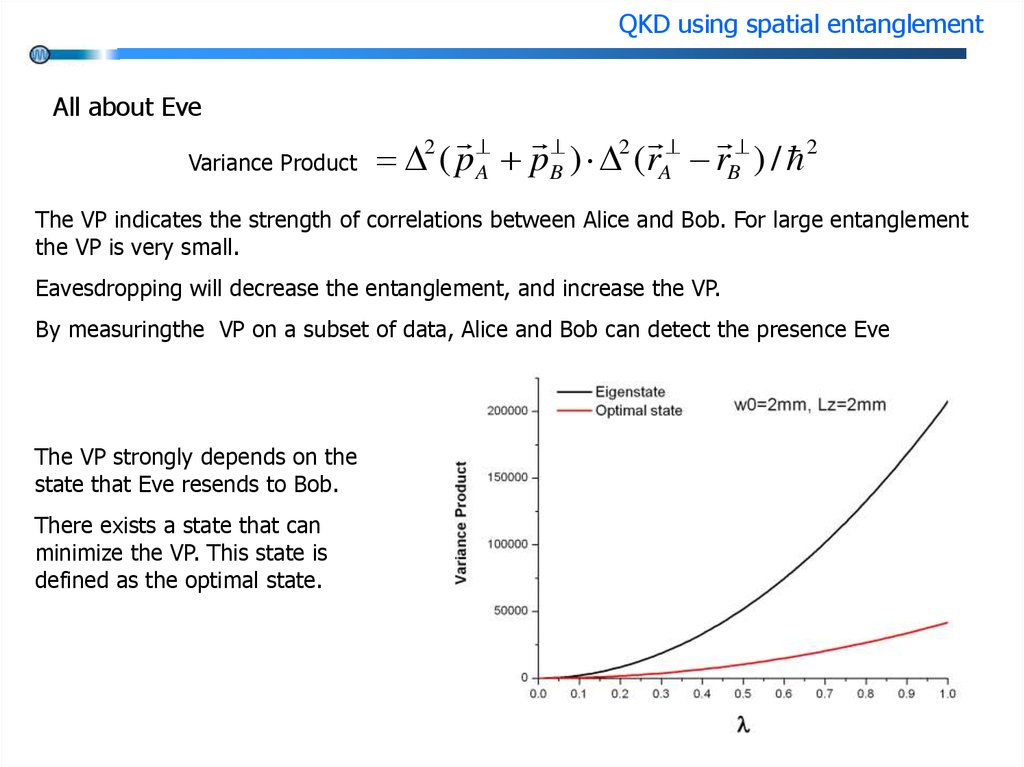
QKD using spatial entanglementAll about Eve
Variance Product
2
( p A pB ) (rA rB ) / 2
2
The VP indicates the strength of correlations between Alice and Bob. For large entanglement
the VP is very small.
Eavesdropping will decrease the entanglement, and increase the VP.
By measuringthe VP on a subset of data, Alice and Bob can detect the presence Eve
The VP strongly depends on the
state that Eve resends to Bob.
There exists a state that can
minimize the VP. This state is
defined as the optimal state.
47.
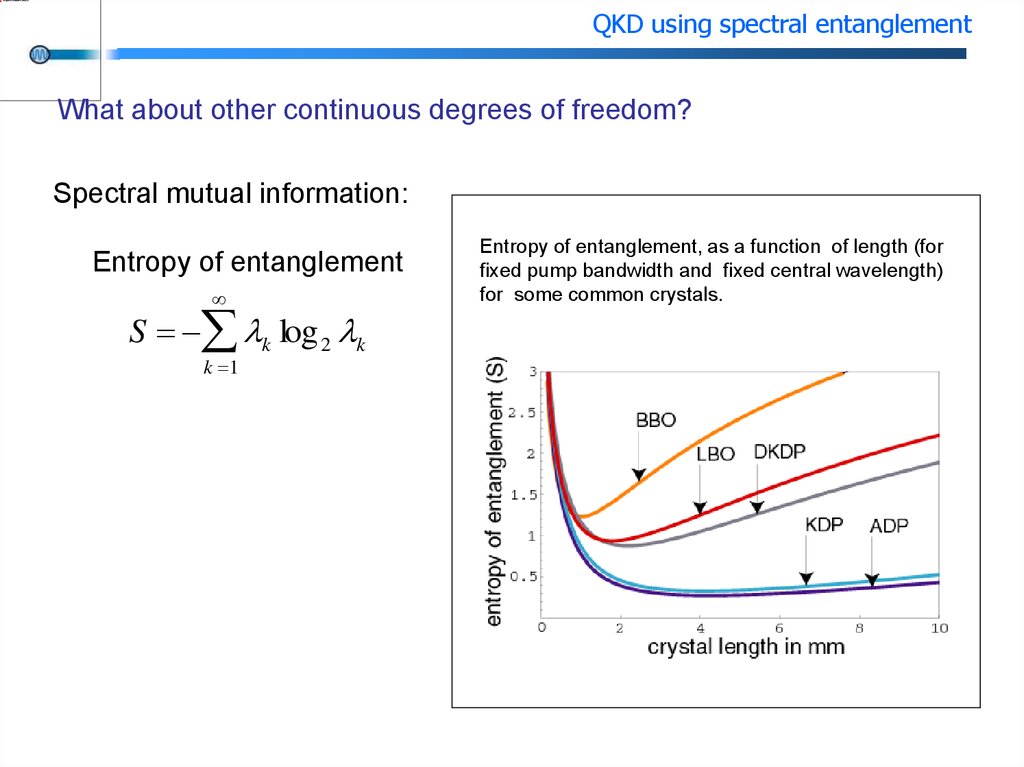
QKD using spectral entanglementWhat about other continuous degrees of freedom?
Spectral mutual information:
Entropy of entanglement
S k log 2 k
k 1
Entropy of entanglement, as a function of length (for
fixed pump bandwidth and fixed central wavelength)
for some common crystals.
48.
Summary• Continuous variables are useful things even at the level of individual photons
Pulsed sources
- can be concatenated
- allow flexible space-time engineering
- enable new kinds of detectors
• Reduced noise:
Efficient conditional nonclassical state preparation
• Engineered correlations:
Conditional pure-state preparation
• Application:
CV QKD using entangled photon pairs
49.
50.
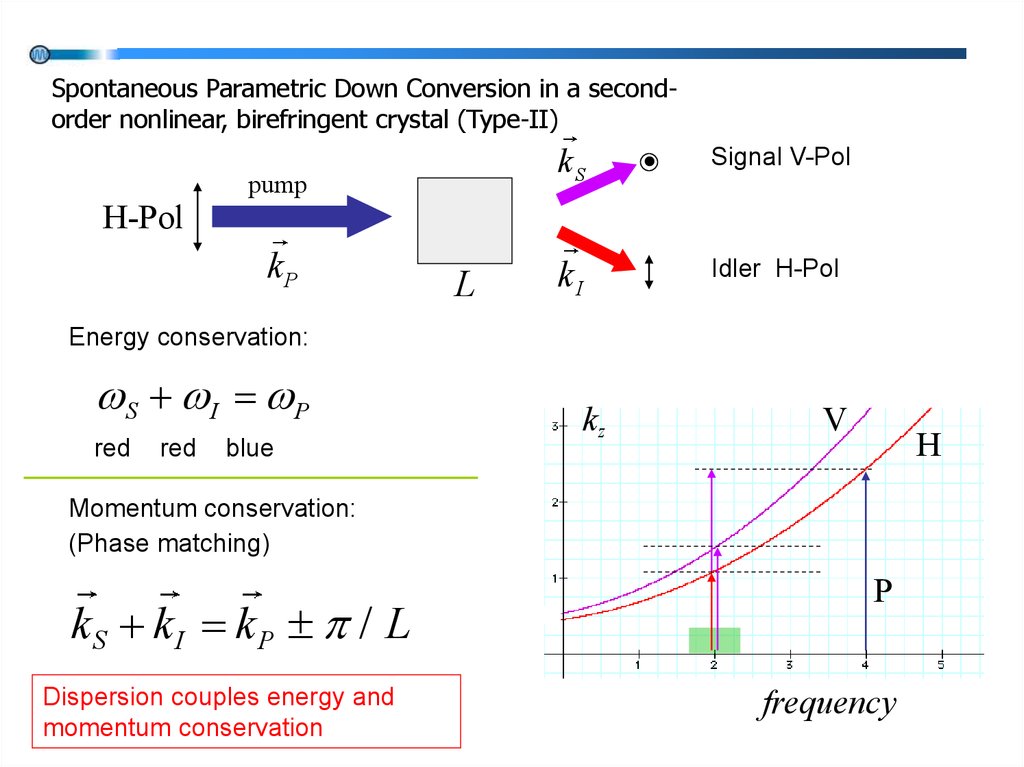
Spontaneous Parametric Down Conversion in a secondorder nonlinear, birefringent crystal (Type-II)H-Pol
pump
kS
Signal V-Pol
kP
kI
Idler H-Pol
L
Energy conservation:
S I P
red
red
blue
kz
V
H
Momentum conservation:
(Phase matching)
kS kI kP p / L
Dispersion couples energy and
momentum conservation
P
frequency
51.
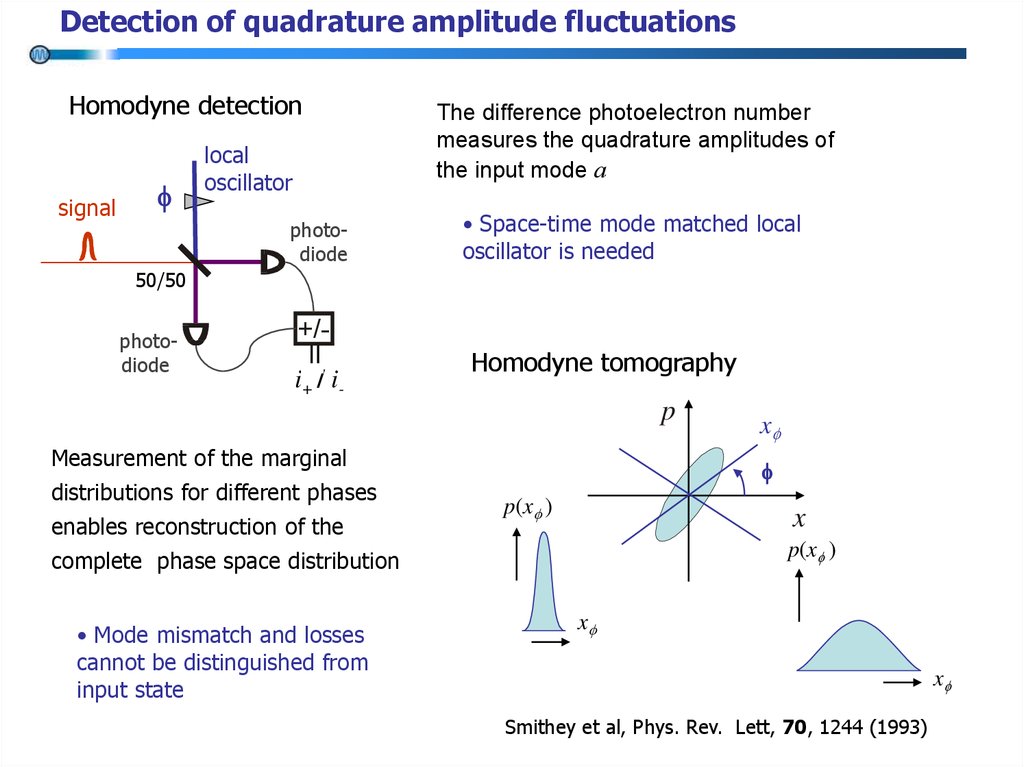
Detection of quadrature amplitude fluctuationsHomodyne detection
signal
f
local
oscillator
photodiode
The difference photoelectron number
measures the quadrature amplitudes of
the input mode a
• Space-time mode matched local
oscillator is needed
50/50
photodiode
+/i+ / i-
Homodyne tomography
p
Measurement of the marginal
distributions for different phases
enables reconstruction of the
f
p(xf )
x
p(xf )
complete phase space distribution
• Mode mismatch and losses
cannot be distinguished from
input state
xf
xf
xf
Smithey et al, Phys. Rev. Lett, 70, 1244 (1993)
52.
Detection of intensity fluctuations• Intensity fluctuations
I
2
• Photon number fluctuations
• Prob. Of generating n
photoelectrons in detector
of efficiency from a pulse
of fixed energy
n
F. T. Arecchi, Phys. Rev. Lett. 15, 912 (1965)
(Poissonian)
G – Bose-Einstein statistics (thermal light)
L – Poissonian statistics (coherent light)
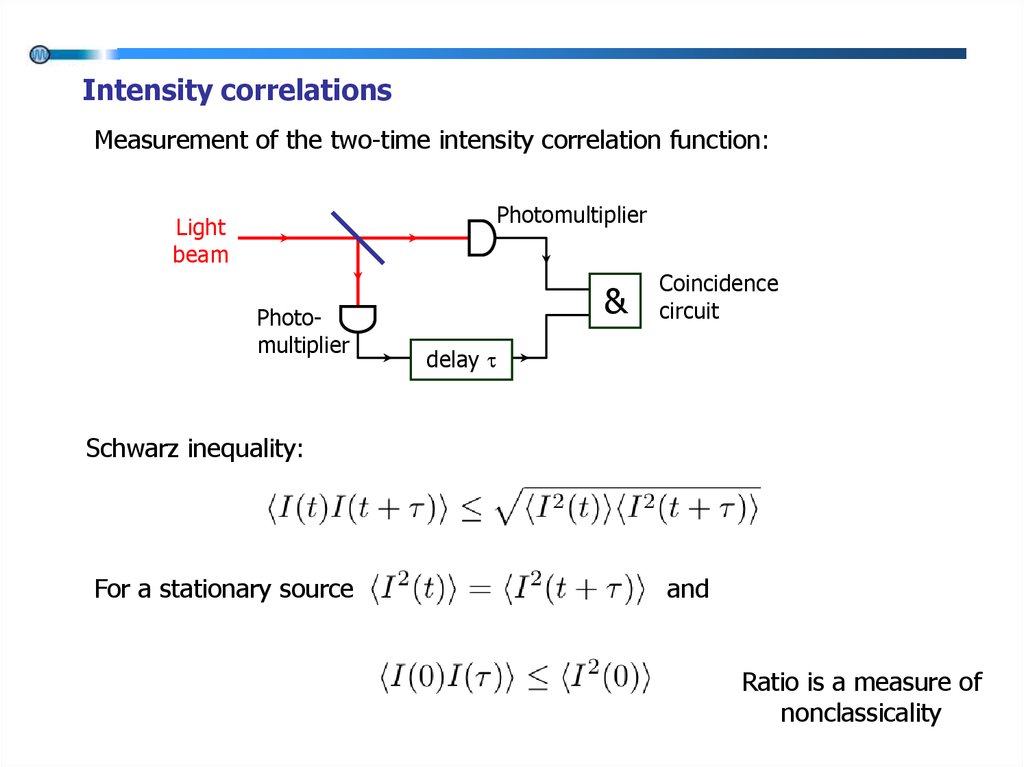
53. Intensity correlations
Measurement of the two-time intensity correlation function:Photomultiplier
Light
beam
Photomultiplier
&
Coincidence
circuit
delay
Schwarz inequality:
For a stationary source
and
Ratio is a measure of
nonclassicality
54.
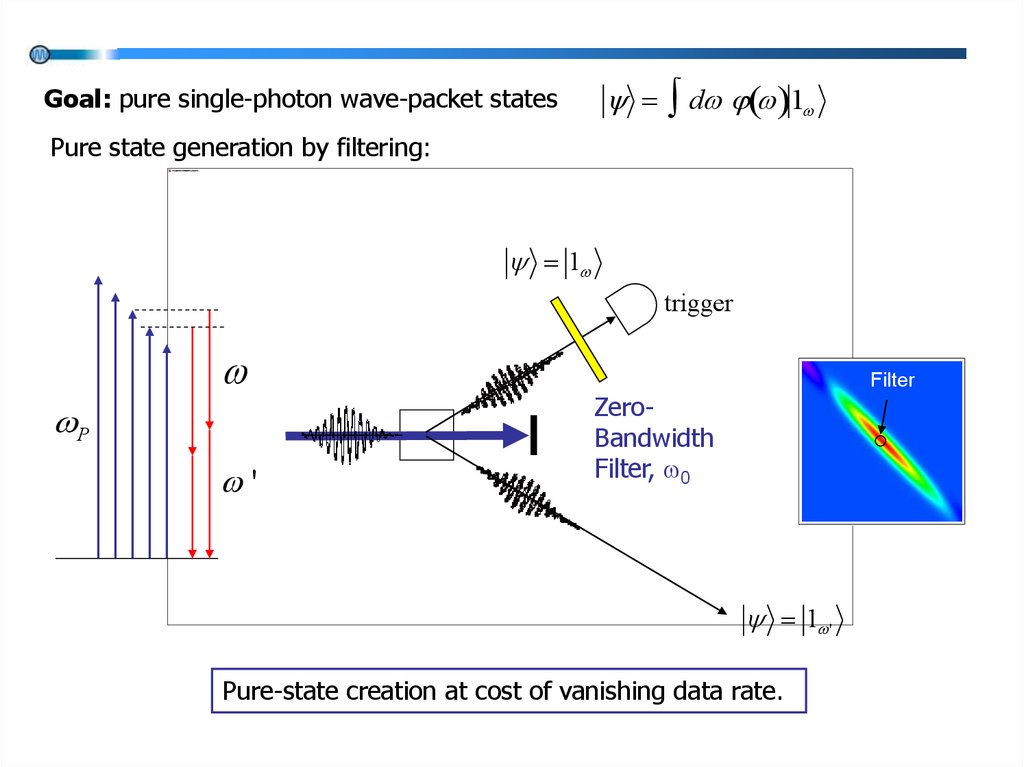
Goal: pure single-photon wave-packet statesd 1
Pure state generation by filtering:
1
trigger
P
'
Filter
ZeroBandwidth
Filter, 0
1 '
Pure-state creation at cost of vanishing data rate.












 Физика
Физика








