Похожие презентации:
The Aerodynamics Of A Single-Blade Rotor
1. The Aerodynamics Of A Single-Blade Rotor
The Aerodynamics Of A SingleBlade RotorThe Mathematical Model Of SingleBlade Rotor Flight
Performed by Halyna
Kyiko & Kate Yefimova
2. Why did we start our investigation?
• Because of curiosity;• The interest to the super light aircraft;
• To evaluate the correspondence of
obtained results to the real winged seed
flight parameters.
3. The Aim of the work
• To investigate the aerodynamics of singleblade rotor (SBR);
• To construct its (SBR) mathematical model;
• To get acquainted with results of scientific
researches in this area;
• To solve the equation of motion of SBR: to find
the angular velocity of autorotation.
• To sophisticate the idea of SBR motion.
4.
We assumed the model of a winged seedaerodynamics and, based on it, we
decided to create the single blade rotor.
5.
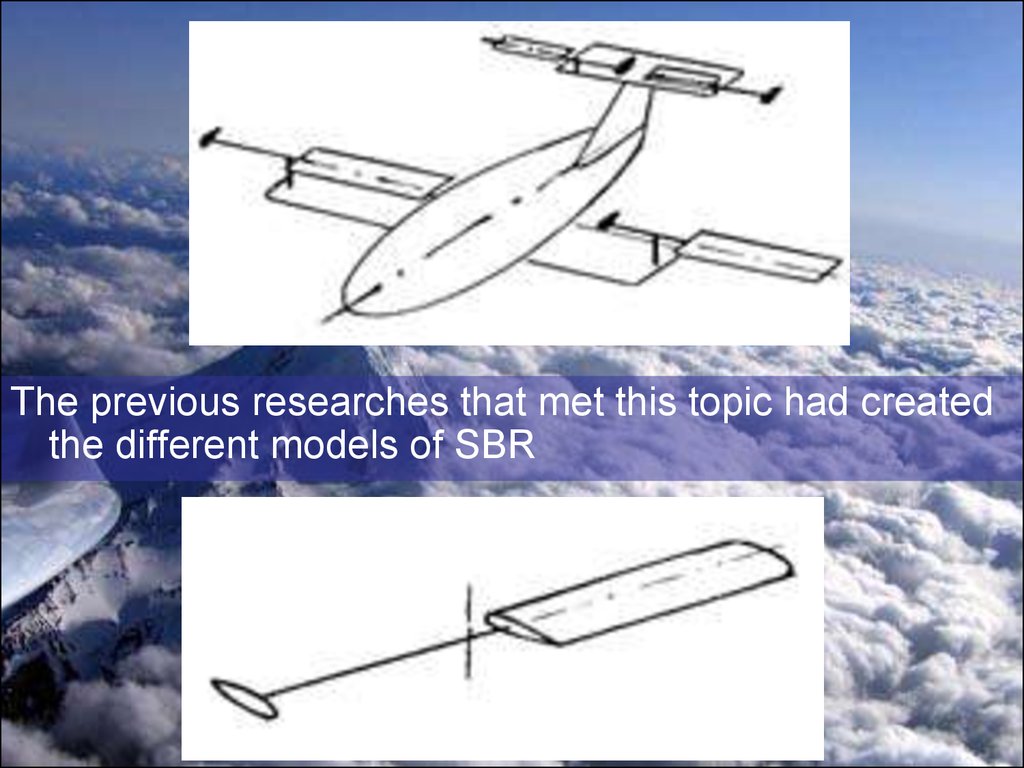
The previous researches that met this topic had createdthe different models of SBR
6. Gluhareff MEG-1x
7. MEG-1X
MEG-1X was created byAmerican engineer
Eugene Gluhareff in
1955. The creator of
MEG-1X, following the
ancient wisdom “the
less, the better”, has
coherently thrown out all
the excessive details, he
did not spare even the
blades: only one of them
stayed with a jet engine,
attached to its end.
8.
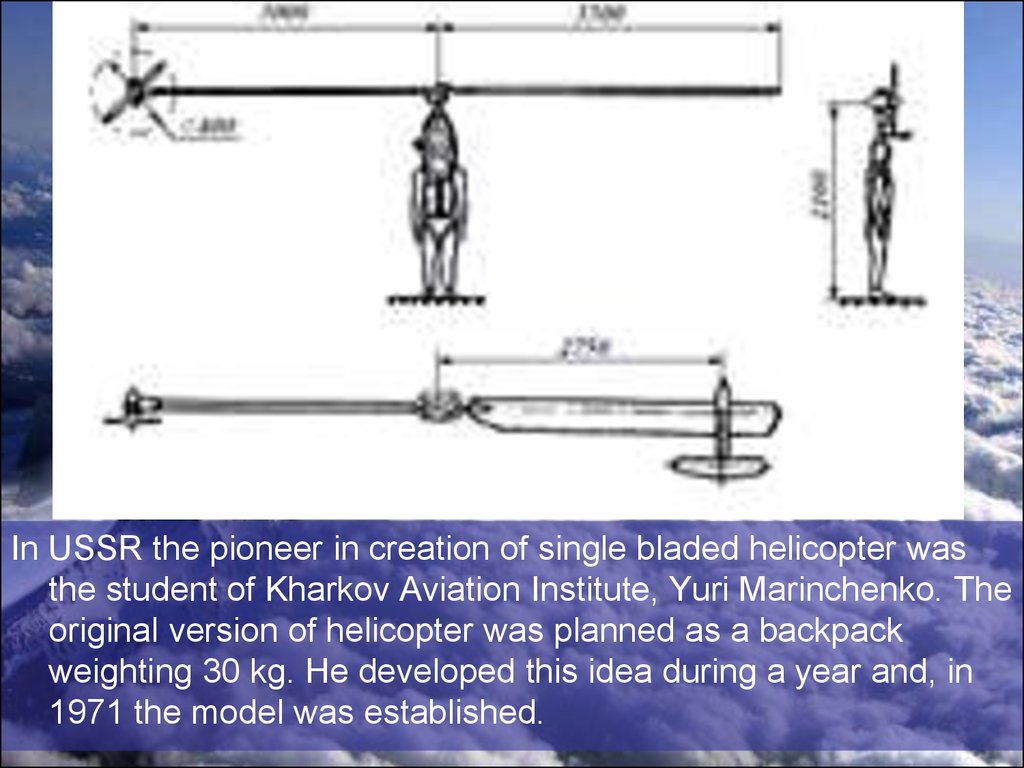
In USSR the pioneer in creation of single bladed helicopter wasthe student of Kharkov Aviation Institute, Yuri Marinchenko. The
original version of helicopter was planned as a backpack
weighting 30 kg. He developed this idea during a year and, in
1971 the model was established.
9. AliSport
Nowadays the only firm, Alisport,creates the full-size aircraft with
single blade rotor.
They created the famous onebladed gliders.
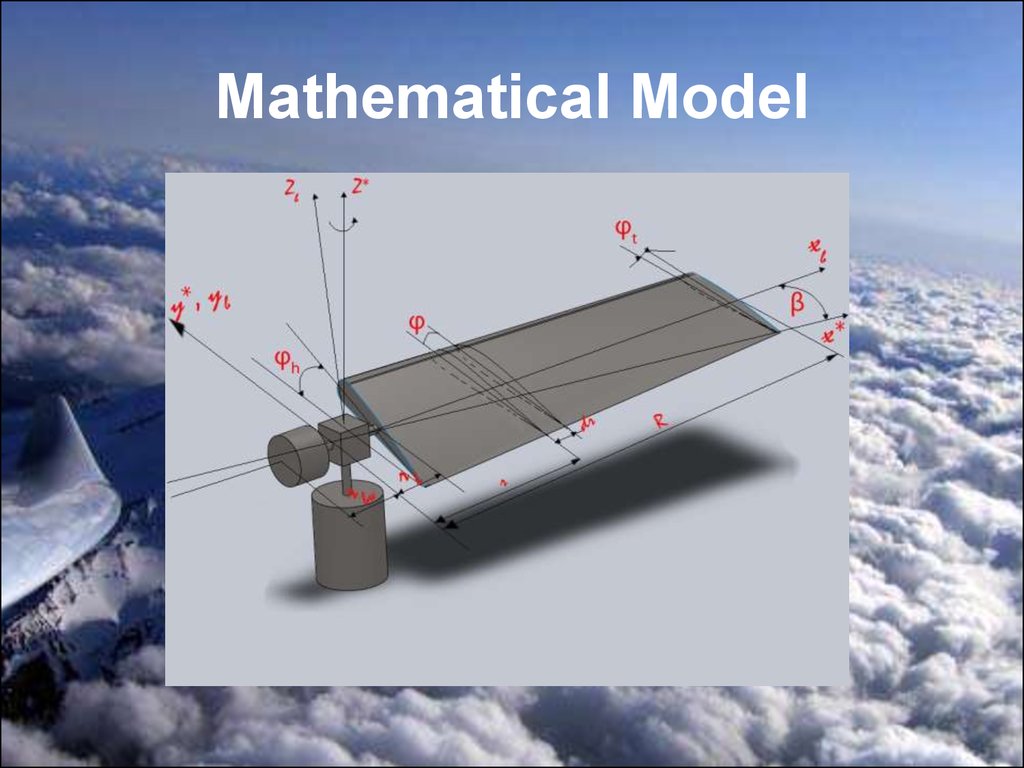
10. Mathematical Model
11.
dVz *1
T
dt
M
dz *
Vz *
dt
d z *
1
M z*
dt
I z*
d
t *
dt
12.
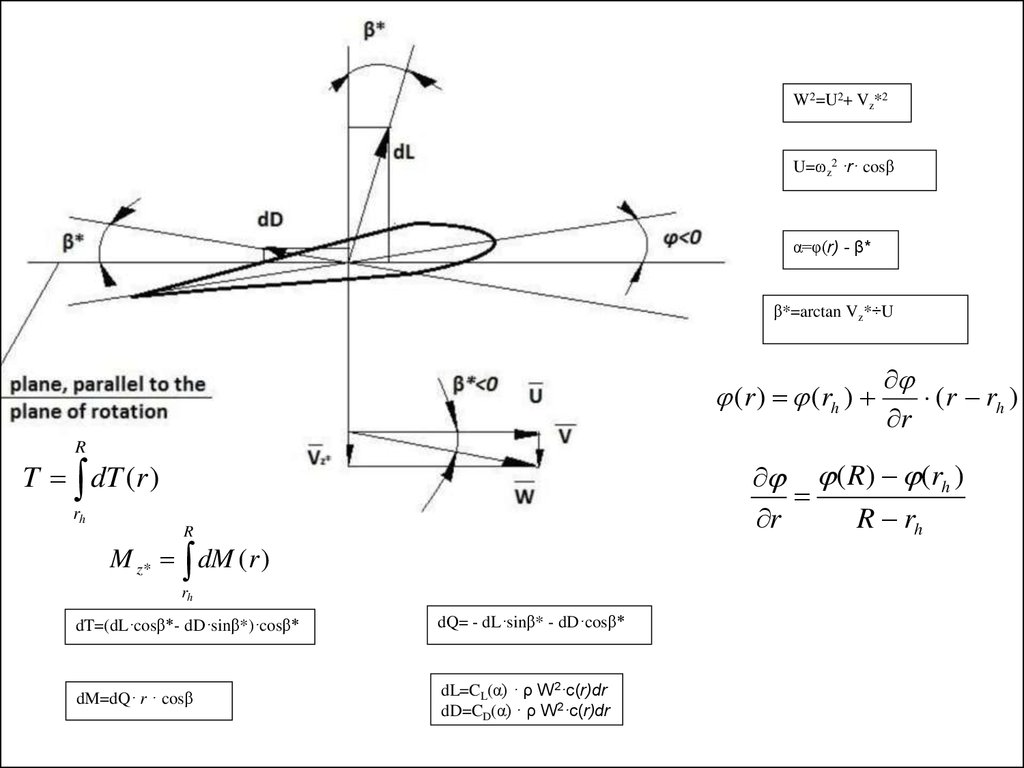
W2=U2+ Vz*2U=ωz2 ·r· cosβ
α=φ(r) - β*
β*=arctan Vz*÷U
(r ) (rh )
R
T dT (r )
rh
(r rh )
r
( R) (rh )
r
R rh
R
M z * dM (r )
rh
dT=(dL·cosβ*- dD·sinβ*)·cosβ*
dQ= - dL·sinβ* - dD·cosβ*
dM=dQ· r · cosβ
dL=CL(α) · ρ W 2·c(r)dr
dD=CD(α) · ρ W 2·c(r)dr
13.
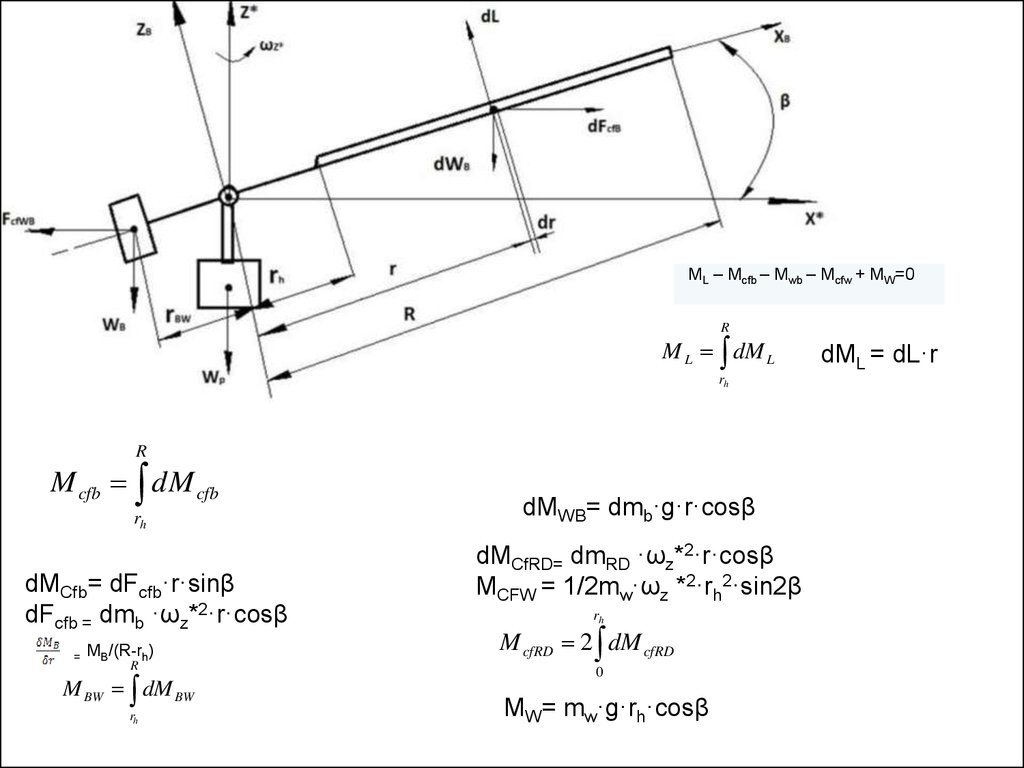
ML – Mcfb – Mwb – Mcfw + MW=0R
M L dM L
rh
R
M cfb d M cfb
rh
dMCfb= dFcfb·r·sinβ
dFcfb = dmb ·ωz*2·r·cosβ
=
dMWB= dmb·g·r·cosβ
dMCfRD= dmRD ·ωz*2·r·cosβ
MCFW = 1/2mw·ωz *2·rh2·sin2β
rh
R
M cfRD 2 dM cfRD
rh
MW= mw·g·rh·cosβ
MB/(R-rh)
M BW dM BW
0
dML = dL·r
14.
0.5N/w
0
-0.5
efficiency,
-1
-1.5
-2
-2.5
120
0.3
100
0.25
80
0.2
0.15
60
0.1
omega,1/s
40
0.05
bal. weight radius, m







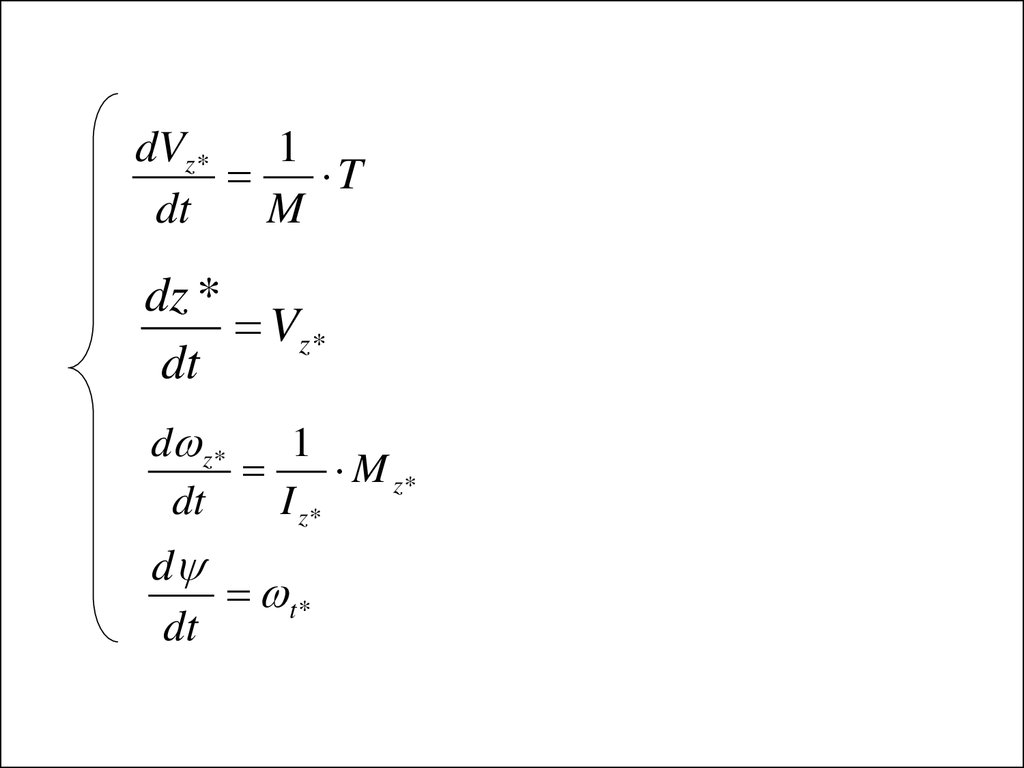


 Математика
Математика Физика
Физика








