Похожие презентации:
Reduced Hierarchy Equations of Motion approach to a quantum dissipative system: Theory
1.
Reduced Hierarchy Equations ofMotion approach to a quantum
dissipative system: Theory
11/28/2022
Yoshitaka Tanimura
Department of Chemistry
Kyoto University
2.
References and ProgramsReview article:
Y. Tanimura, J. Phys. Soc. Jpn, 75, 082001 (2006).
Comprehensive articles:
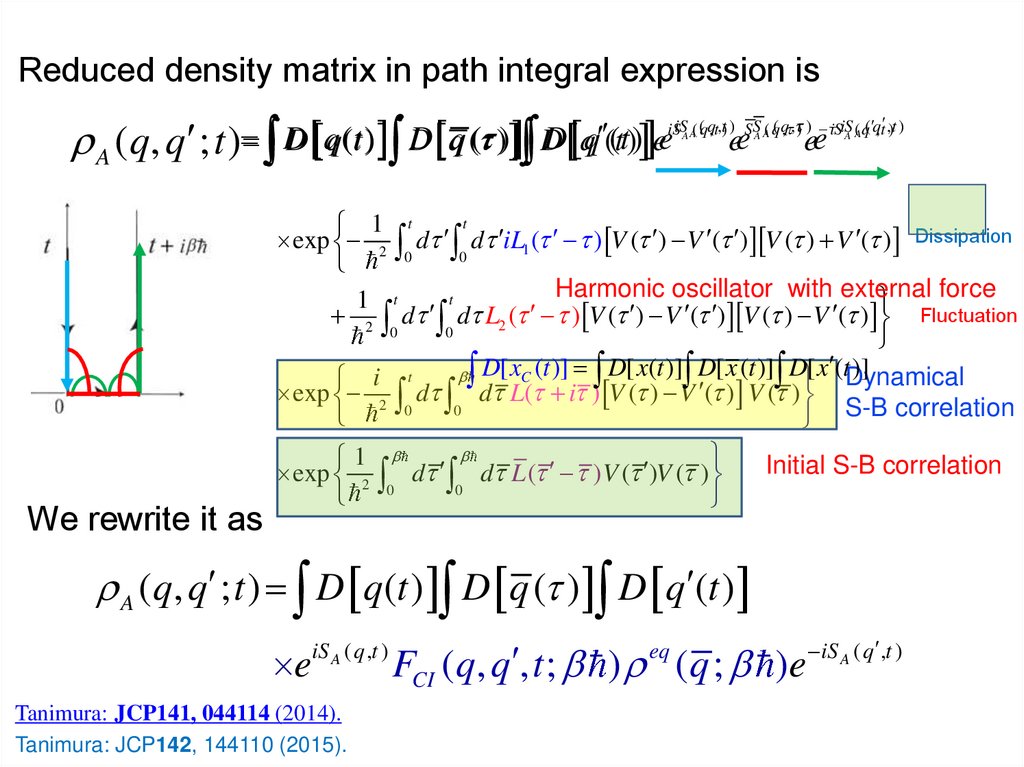
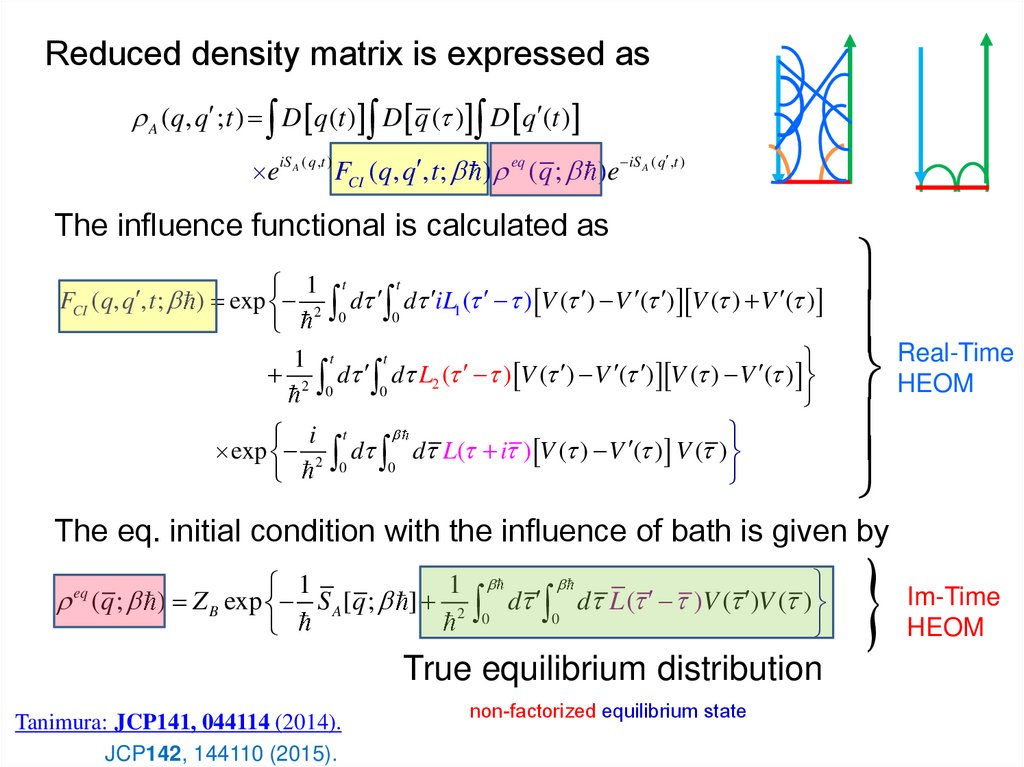
Y. Tanimura: J. Chem. Phys. 141, 044114 (2014).
Y. Tanimura: J. Chem. Phys. 142, 144110 (2015).
Publication Lists & PDF archive
http://theochem.kuchem.kyoto-u.ac.jp/public/
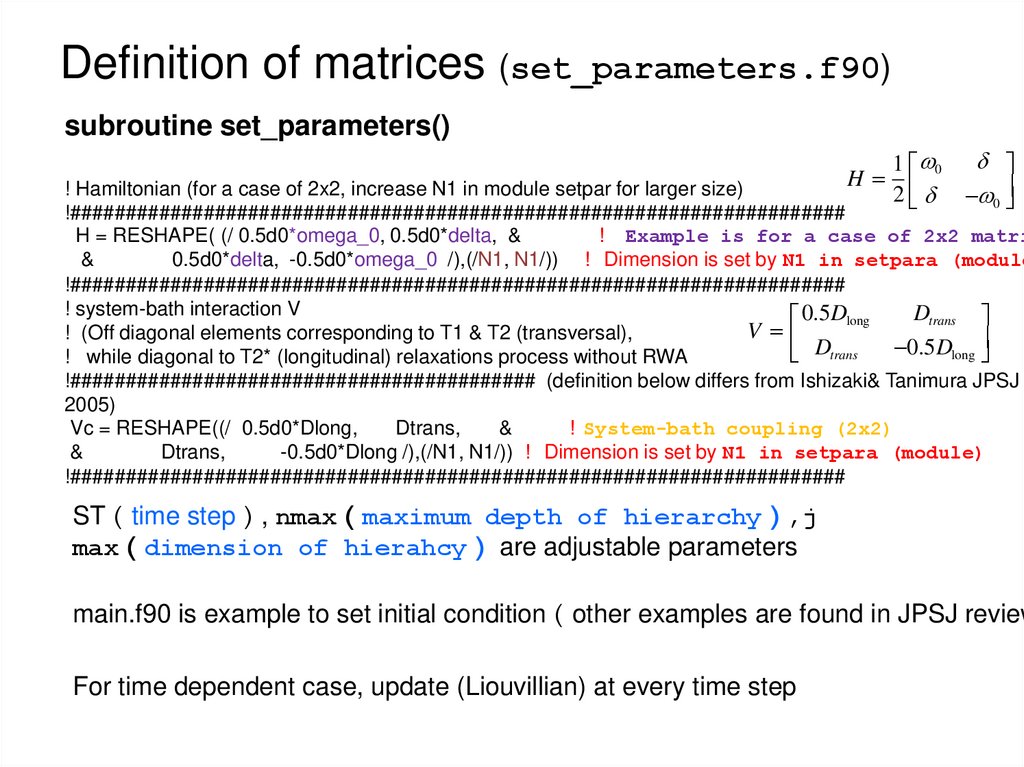
Programs
Without low temperature corrections: (markovian05)
http://theochem.kuchem.kyoto-u.ac.jp/public/GM2DNMR.zip
With low temperature corrections: (nonMarkovian09)
http://theochem.kuchem.kyoto-u.ac.jp/public/nonMarkovian09.zip
With low temperature corrections: (nonMarkovian09)
http://theochem.kuchem.kyoto-u.ac.jp/public/nonMarkovian09.zip
with low temperature correction terms+ subroutines for linear and 2D spectra (nonMarkovian2009+2D )
http://theochem.kuchem.kyoto-u.ac.jp/tanimura/Lectures/nonMarkovian092D.zip
Drude+Brownian mode (nonMarkovian2012+2D )
http://theochem.kuchem.kyoto-u.ac.jp/tanimura/Lectures/nonMarkovian092D.zip
Real-time and imaginary-time quantum hierarchal Fokker-Planck equations (Tanimuran15)
http://theochem.kuchem.kyoto-u.ac.jp/tanimura/Lectures/TanimuranFP.zip
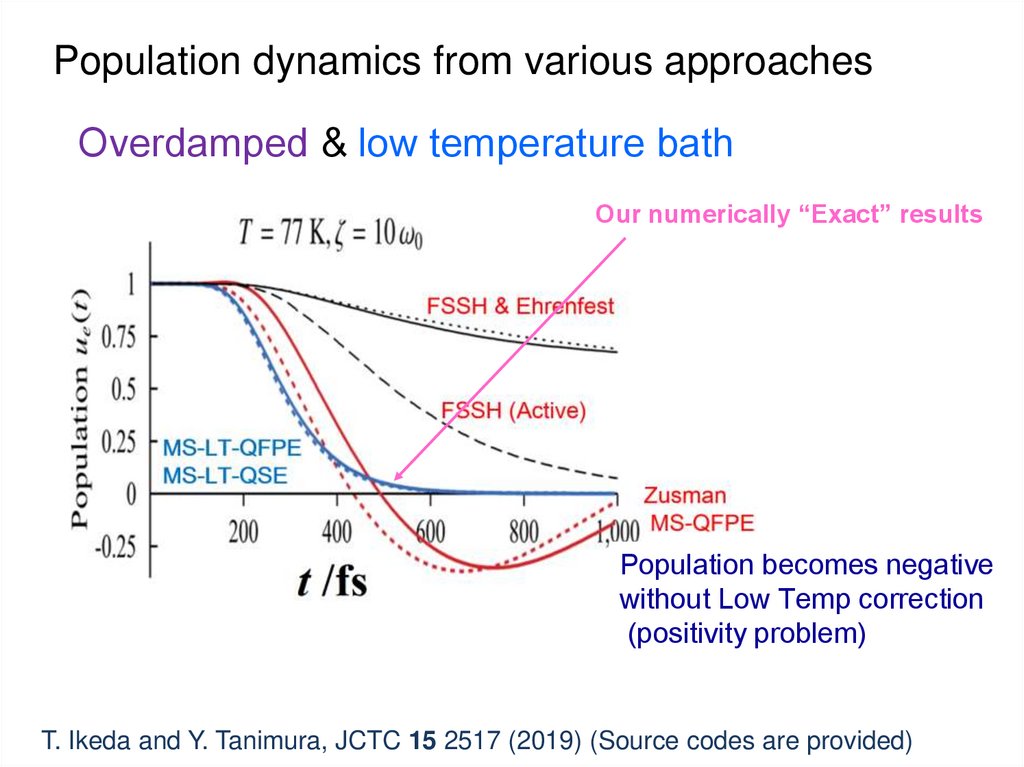
Low-Temp. Quantum Fokker-Planck & Smoluchowski Equations and their extension to multi-state systems
J. Chem. Theory Comput. 15 2517-2534 (2019).
Copy right: Y. Tanimura ([email protected])
3.
Contents1.
2.
3.
4.
5.
6.
7.
8.
9.
10.
11.
12.
13.
14.
15.
16.
Introduction
Standard Approach
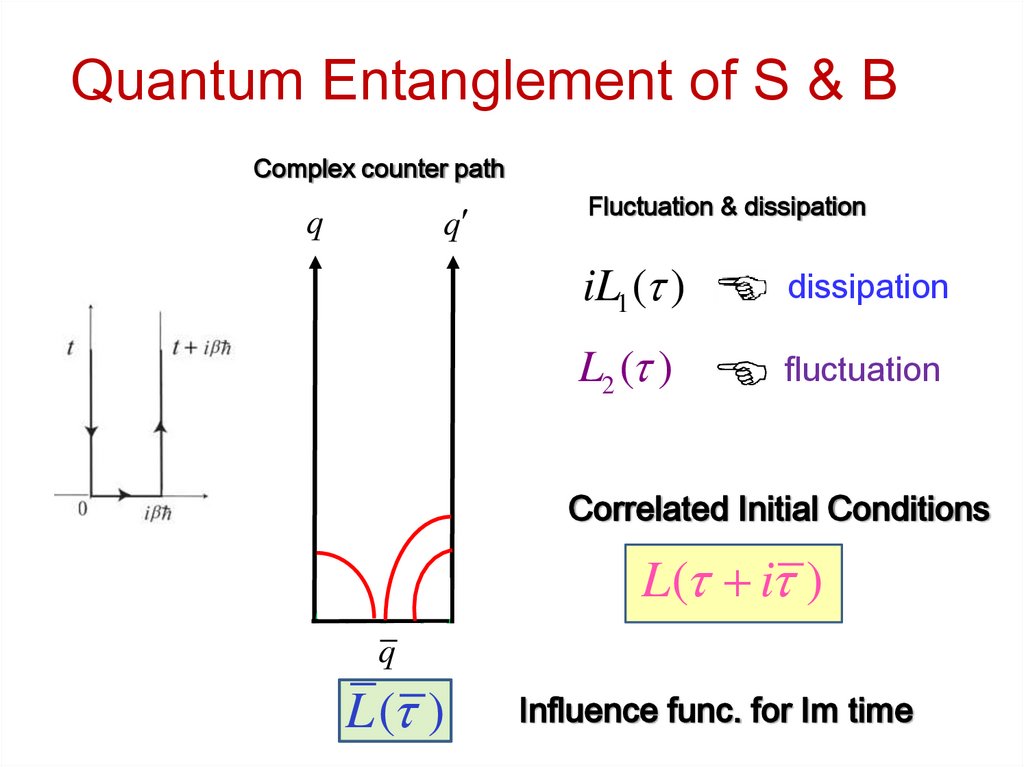
Influence Functional
Quantum Fokker-Planck Equation in Hierarchy Form
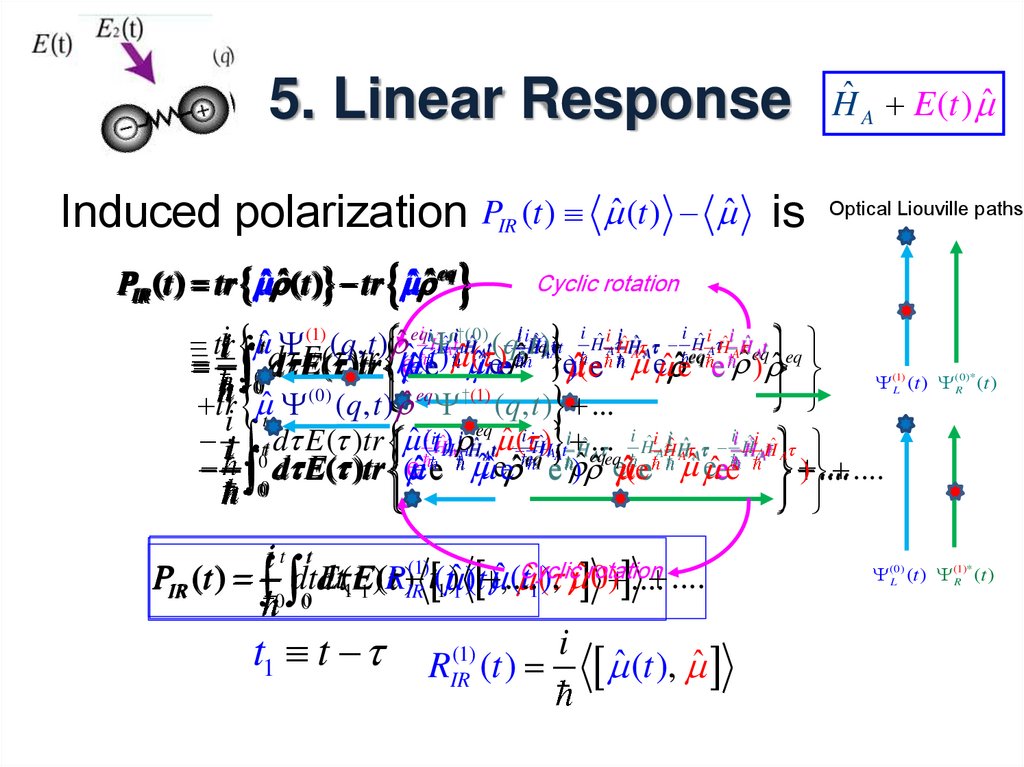
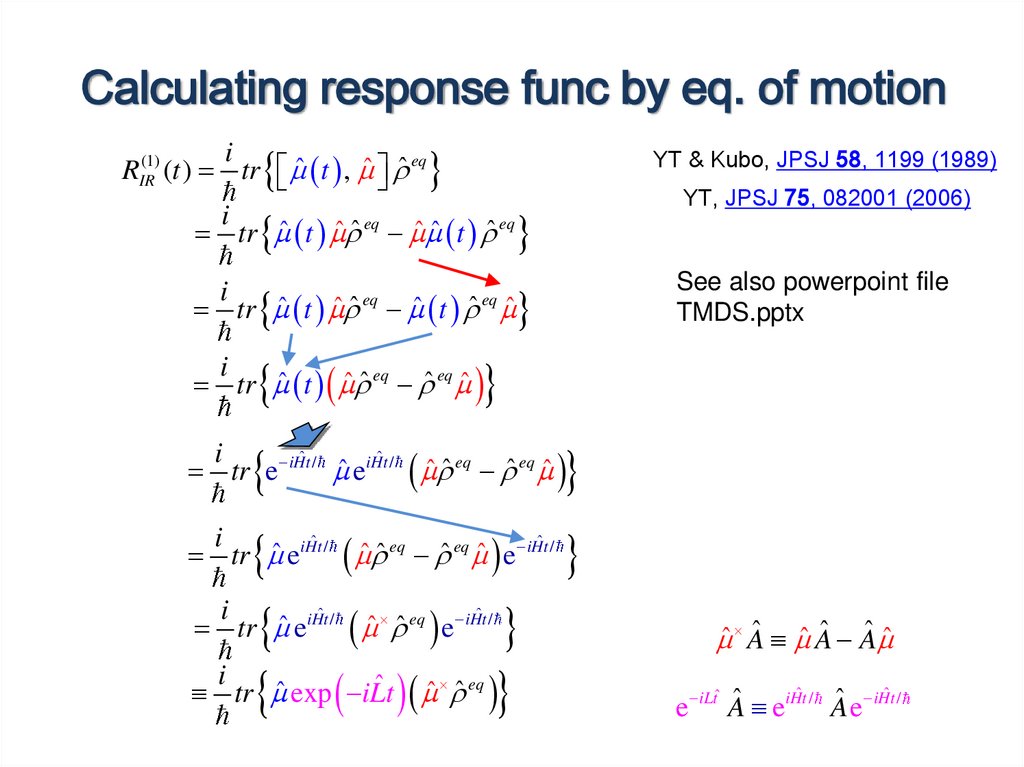
Linear Response
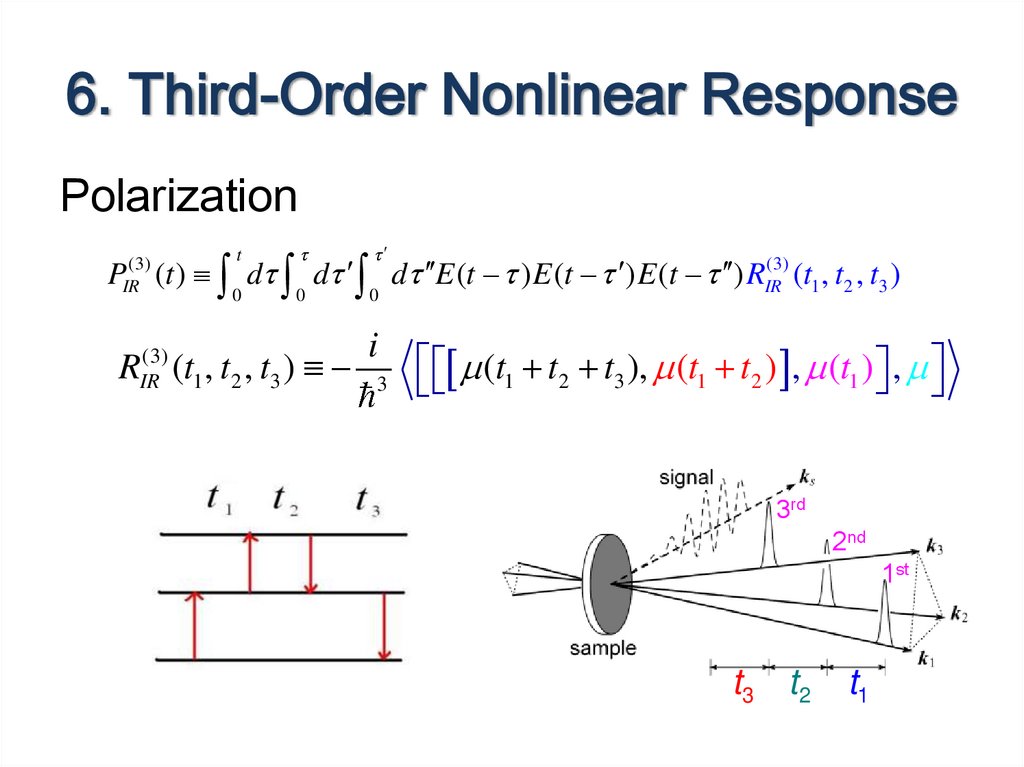
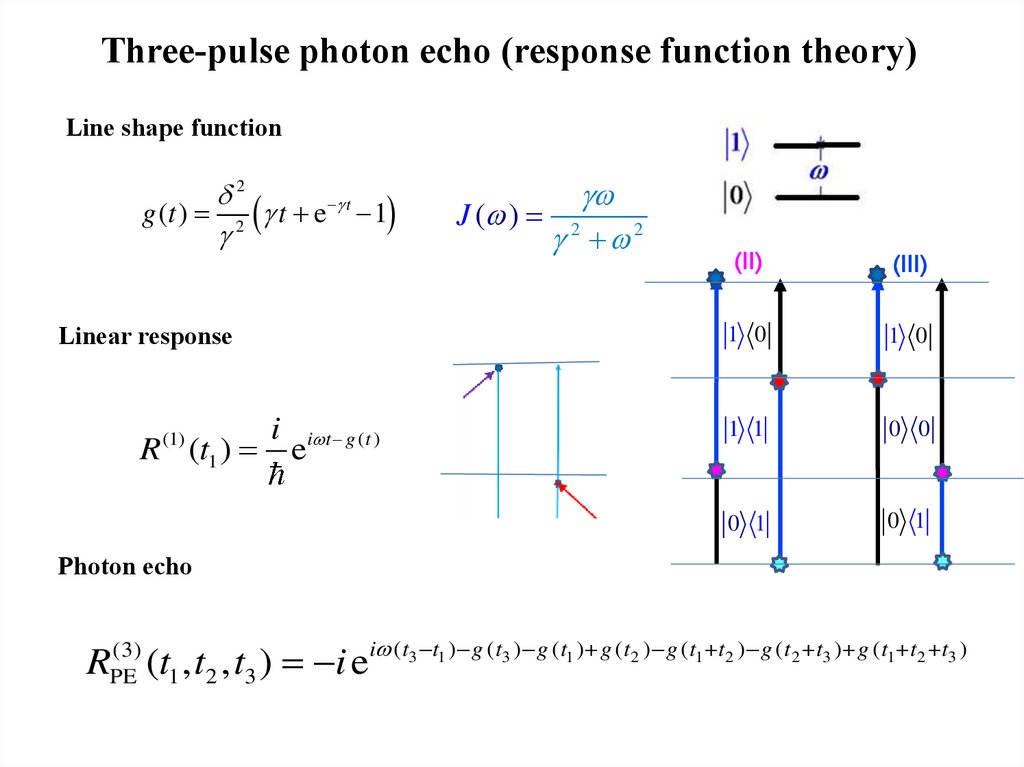
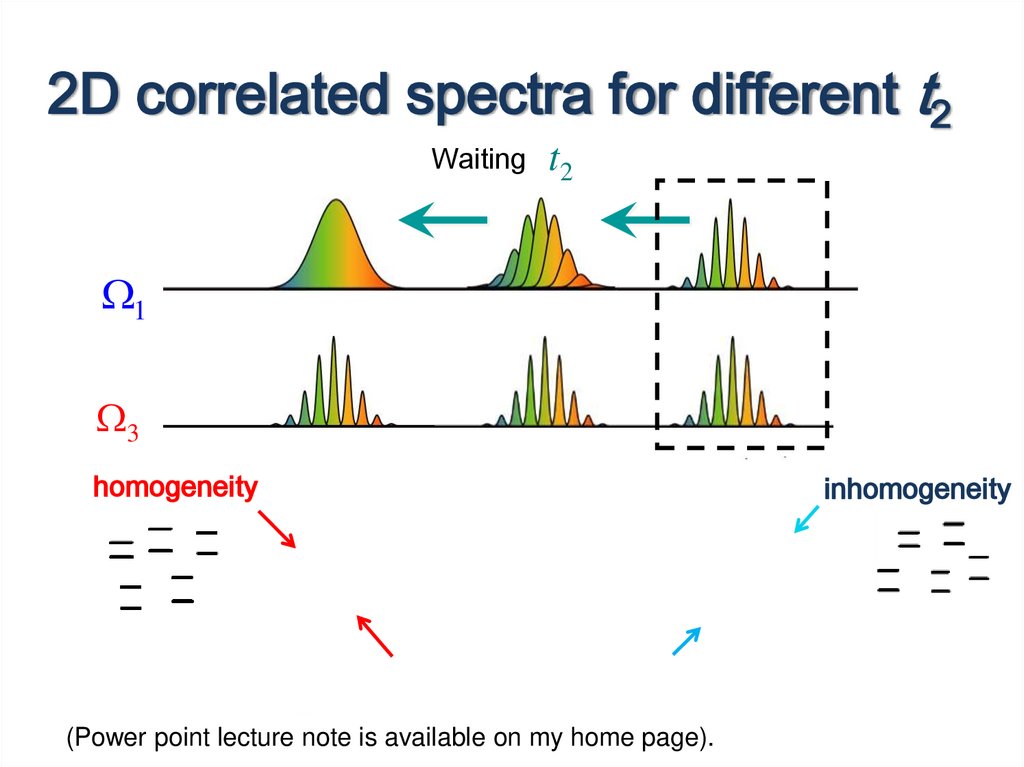
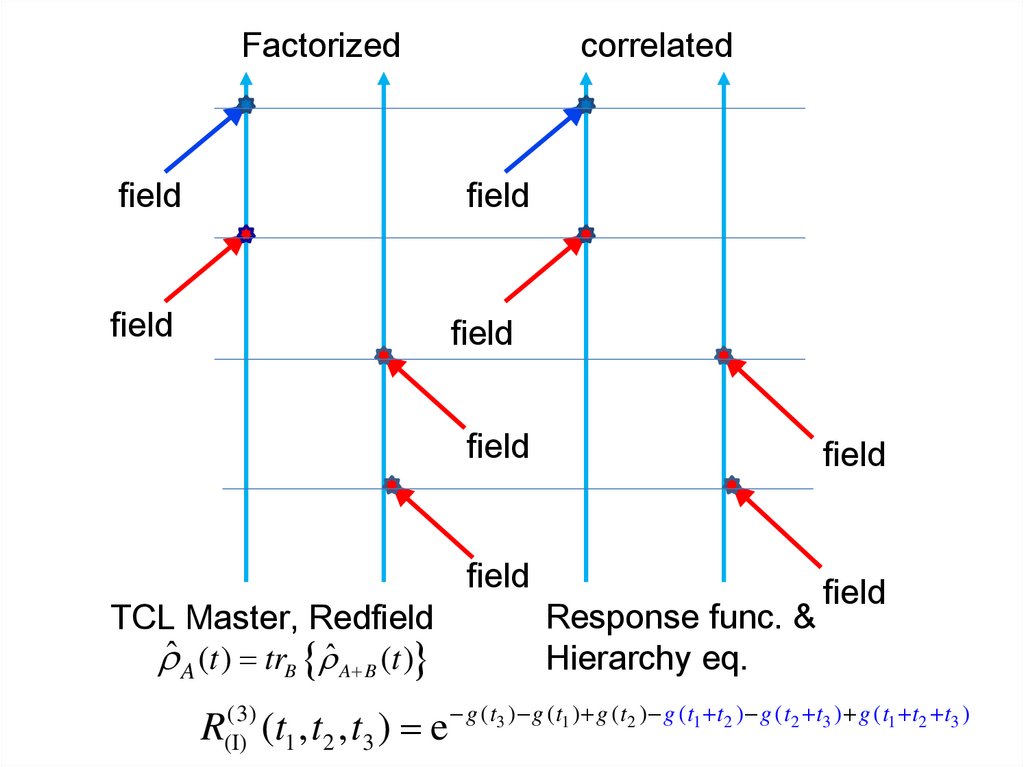
Third-order Nonlinear Response
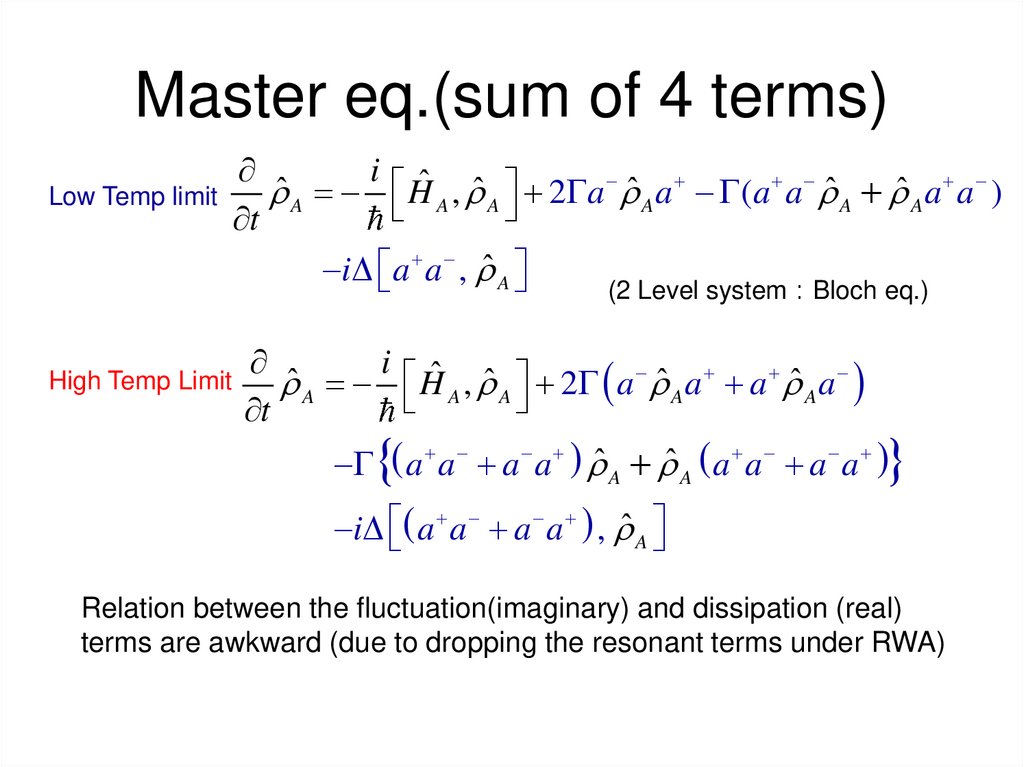
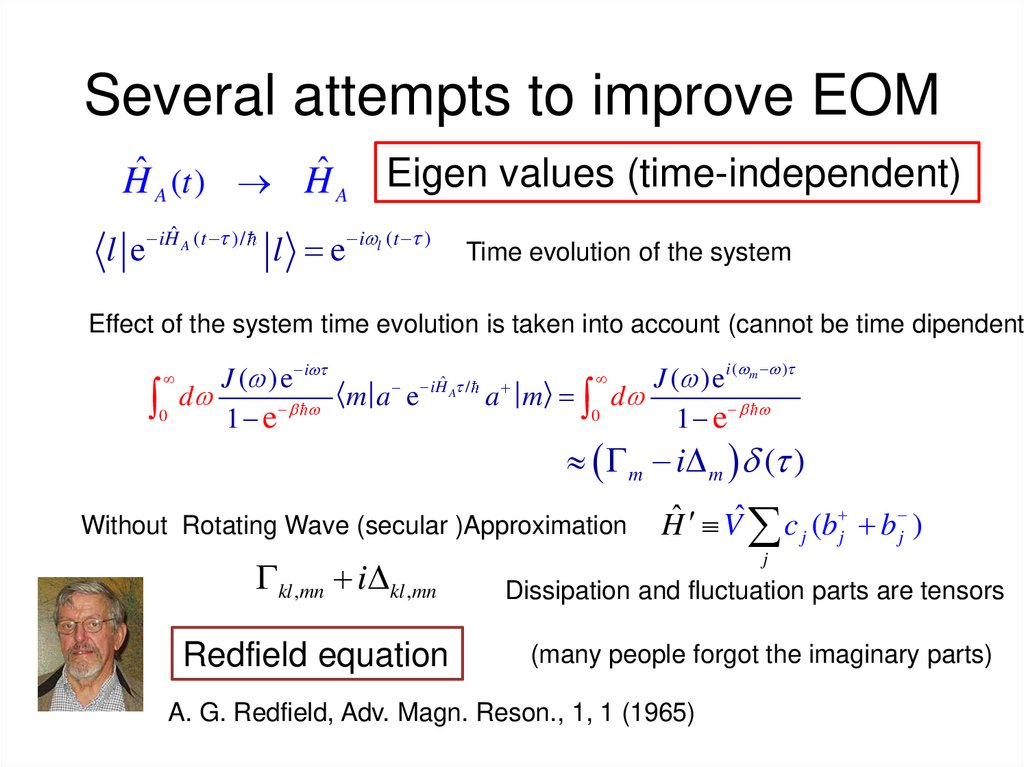
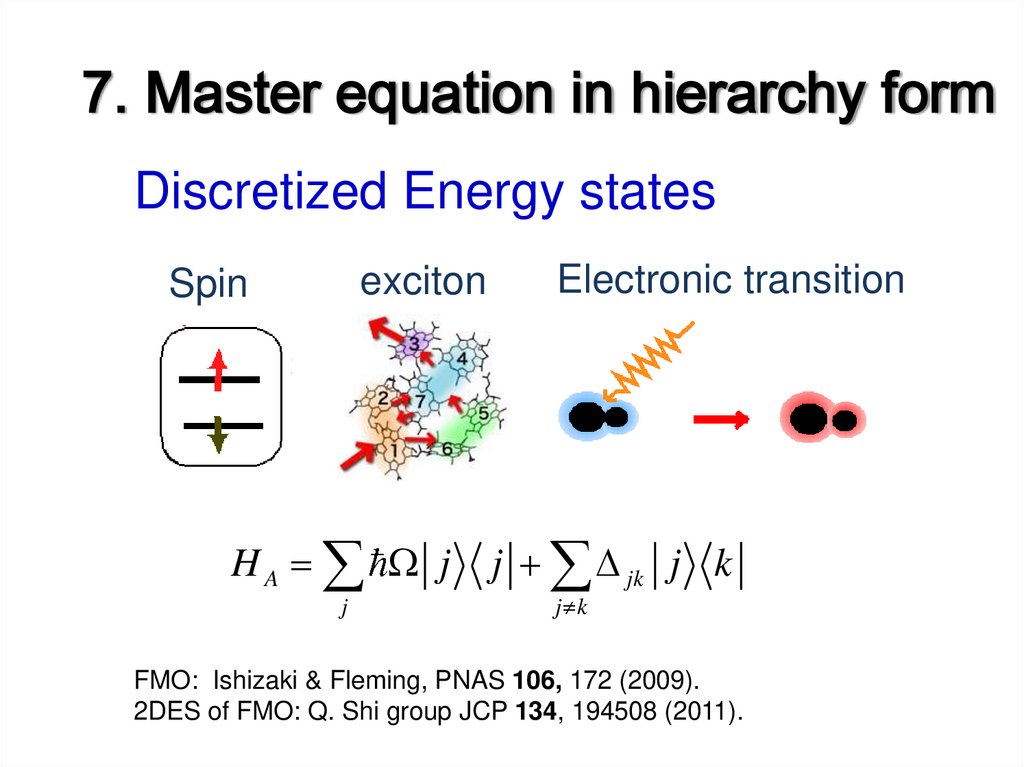
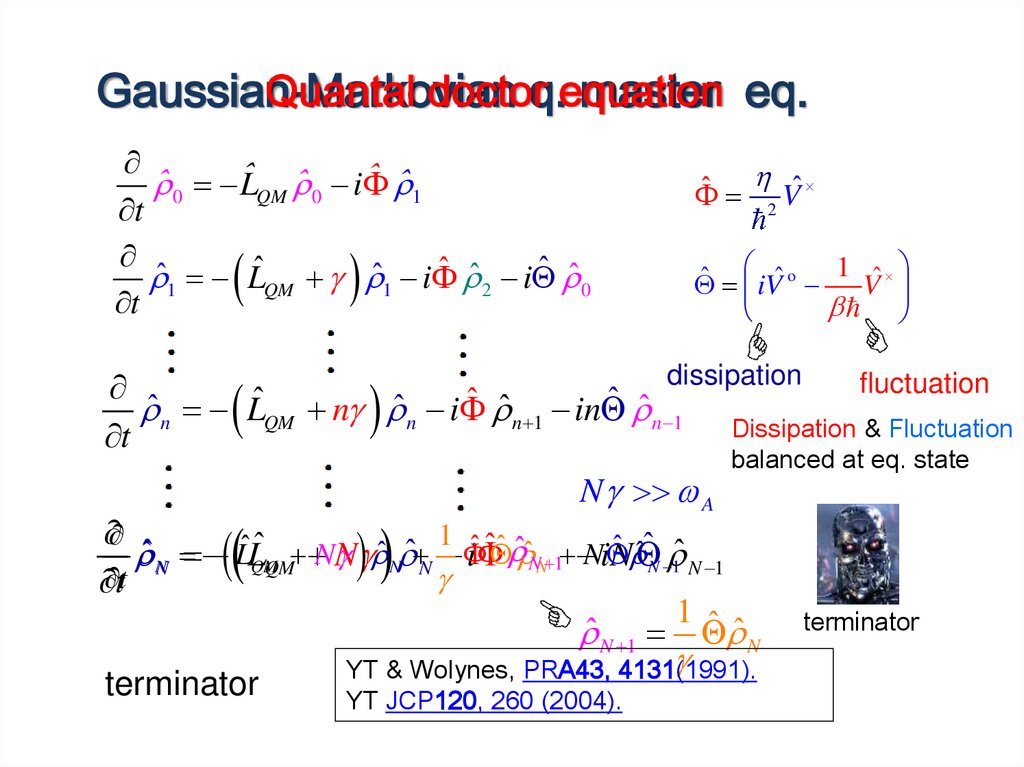
Master Equation in Hierarchy Form
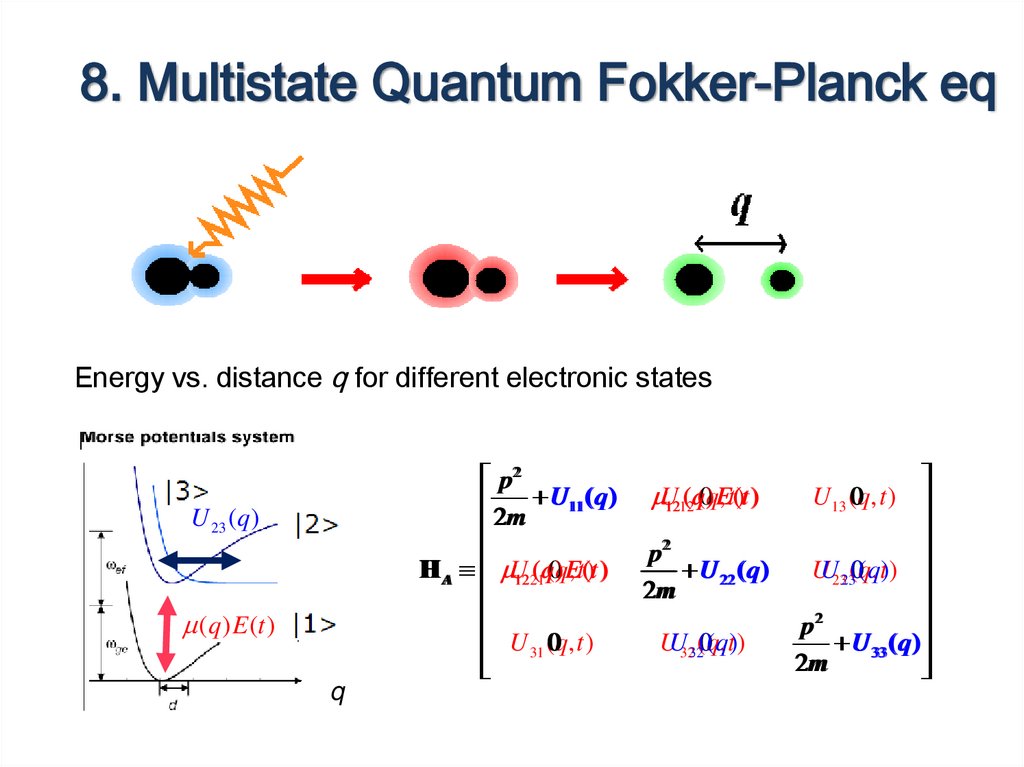
Multi-state Quantum Fokker-Planck Equations
Low Temperature Correction Terms
Imaginary time HEOM
Non-Markovian tests
Low-Temp. Q. Fokker-Planck & Smoluchowski Equations
Hierarchy Equations of Motion for nonOhmic Bath
Improving a computational performance
Stochastic HEOM
NonMarkovian09
4.
1. IntroductionReactant
Product
Thermal
Activation
Relaxation
Tunneling
System-bath model
2
2
2
pj
m j j
cjq
p
H
U (q)
x j
2
2m
2
m j j
j 2m j
2
5.
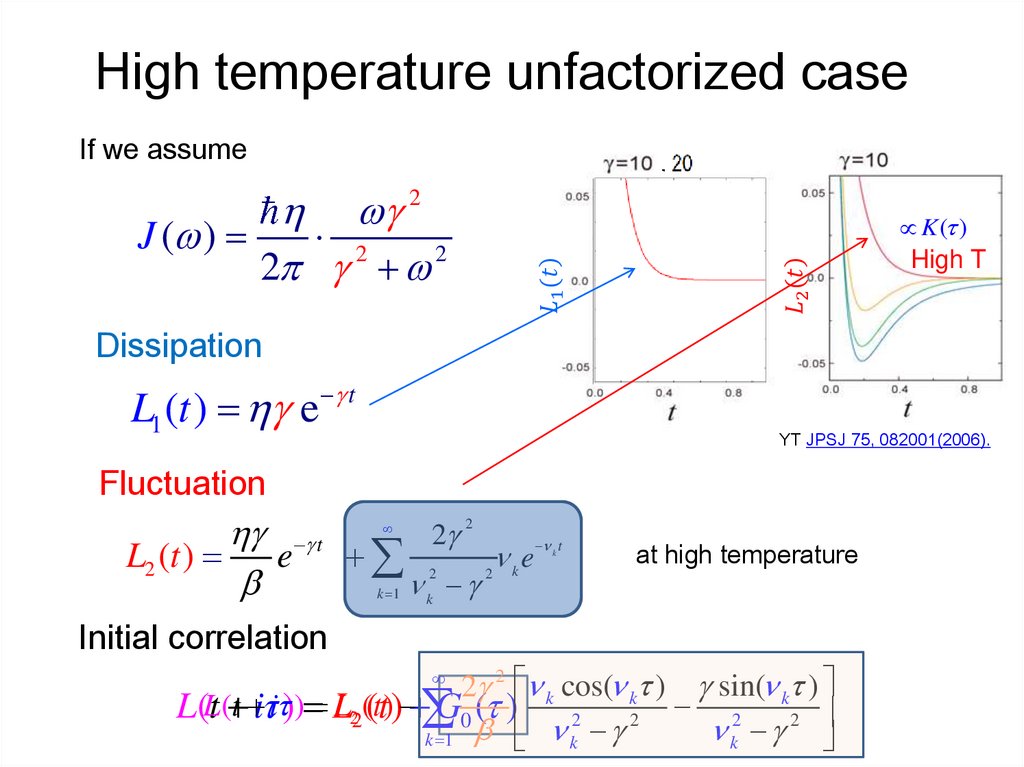
The character of the heat bath is determined byc 2j
J ( )
j
j 4m j j
By computer simulations or experimental means
Moritsugu, Kitao & Kidera,
PRL 85, 3970 (2000)
Hasegawa & Tanimura,
JPC B 115, 5545(2011)
6.
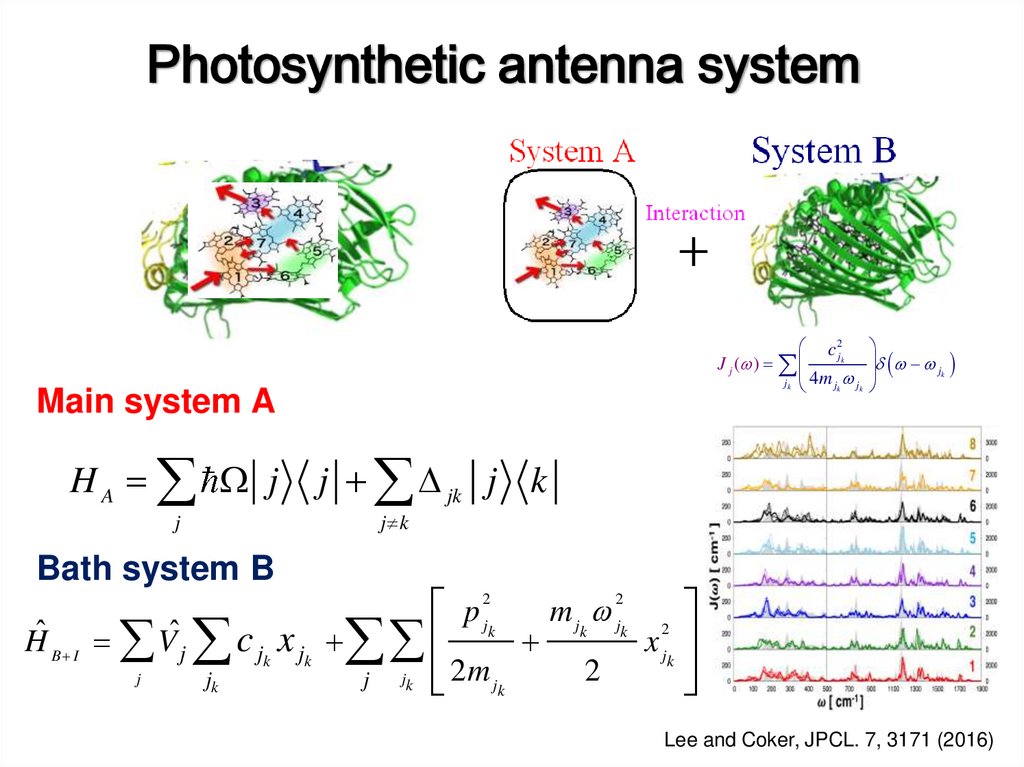
Photosynthetic antenna system+
c 2jk
J j ( )
jk
jk 4m jk jk
Main system A
HA j
j
j jk j k
j k
Bath system B
p 2jk m jk 2jk 2
Hˆ B I Vˆj c jk x jk
x jk
2
j
jk
j jk 2 m jk
Lee and Coker, JPCL. 7, 3171 (2016)
7.
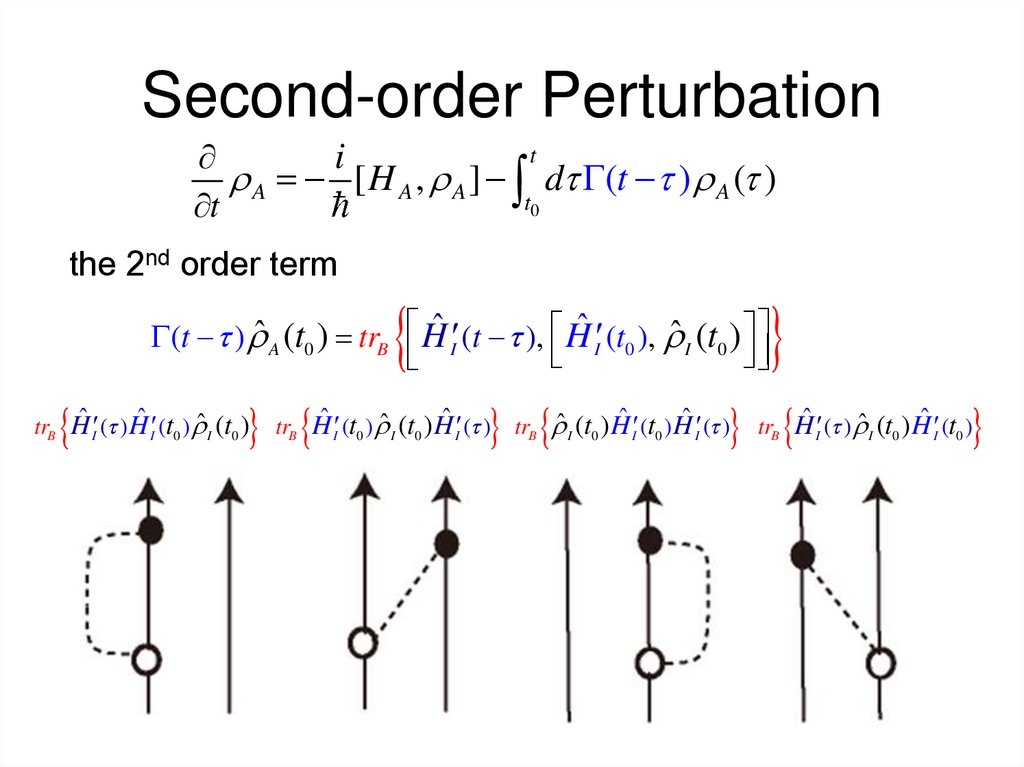
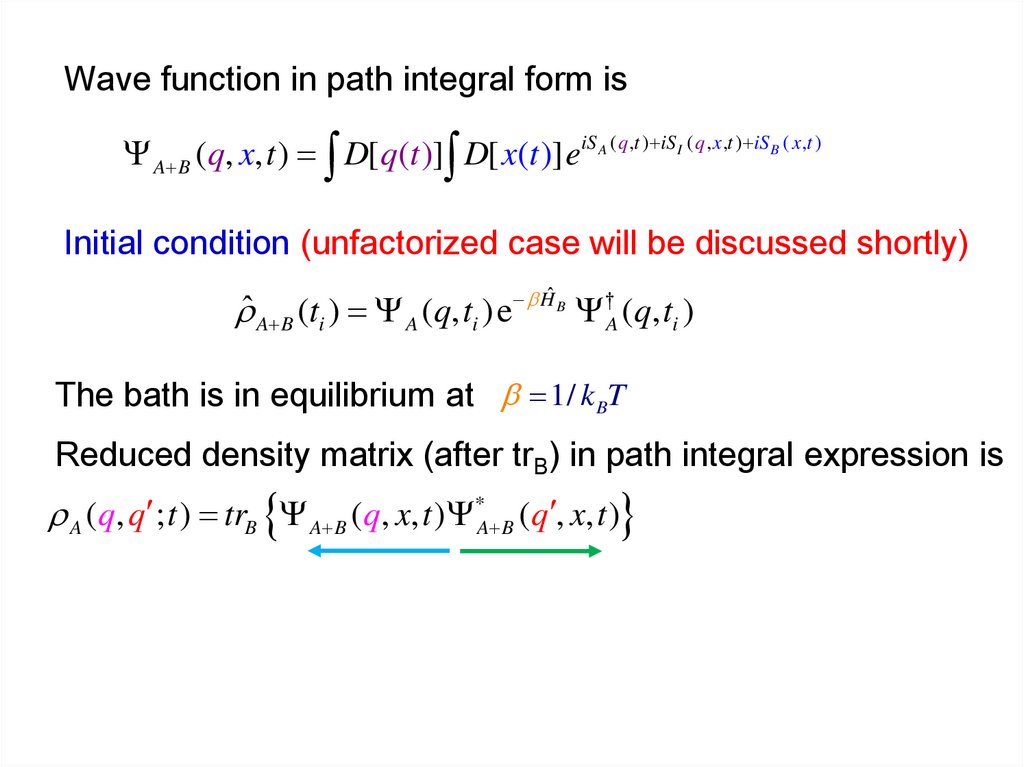
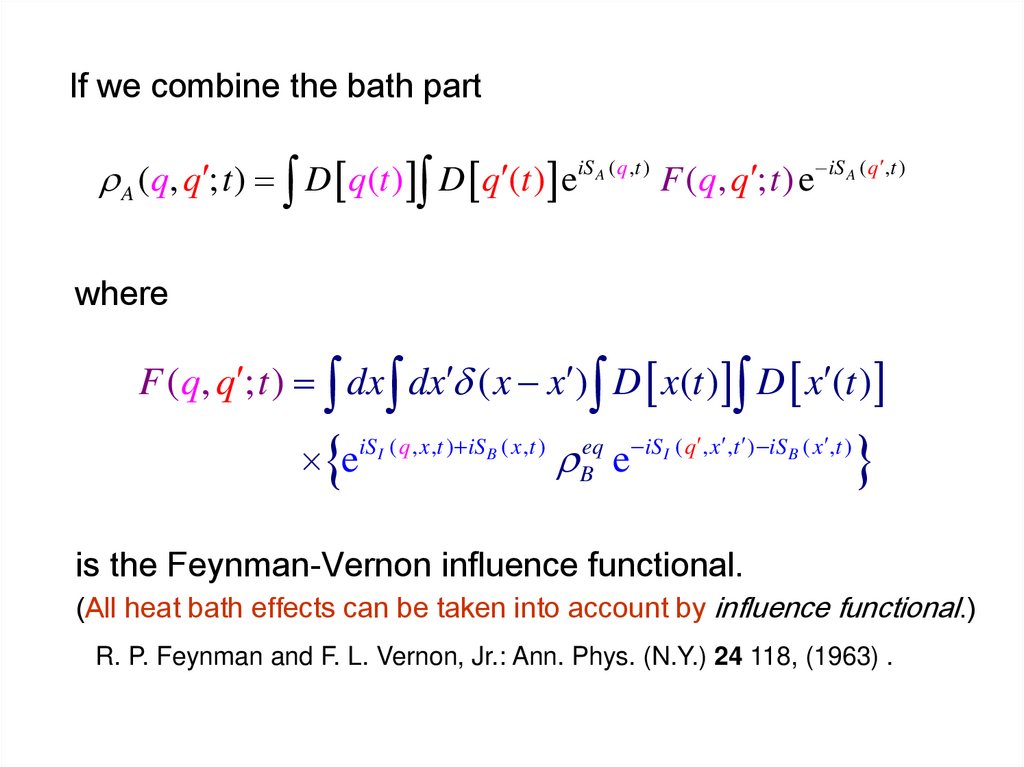
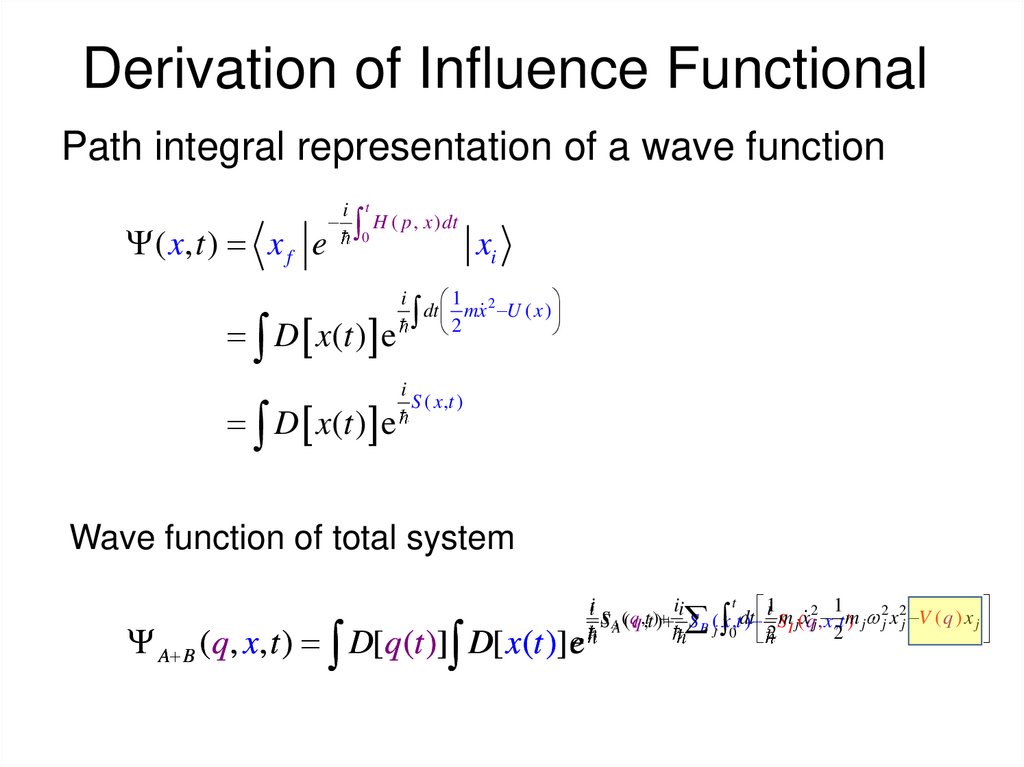
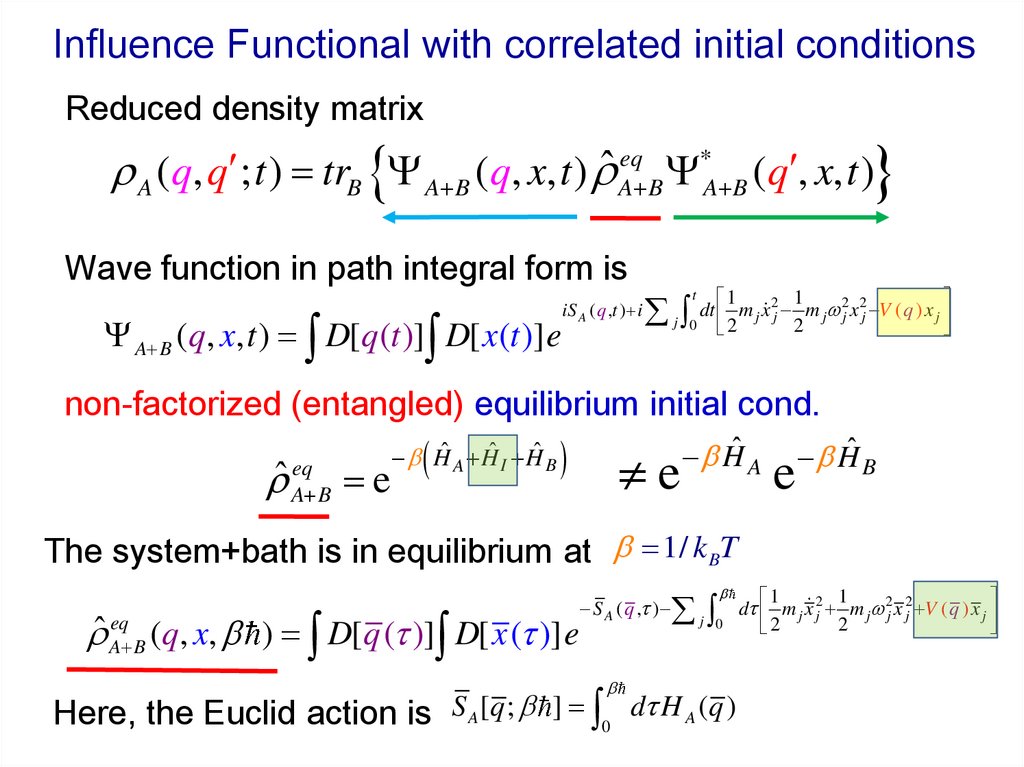
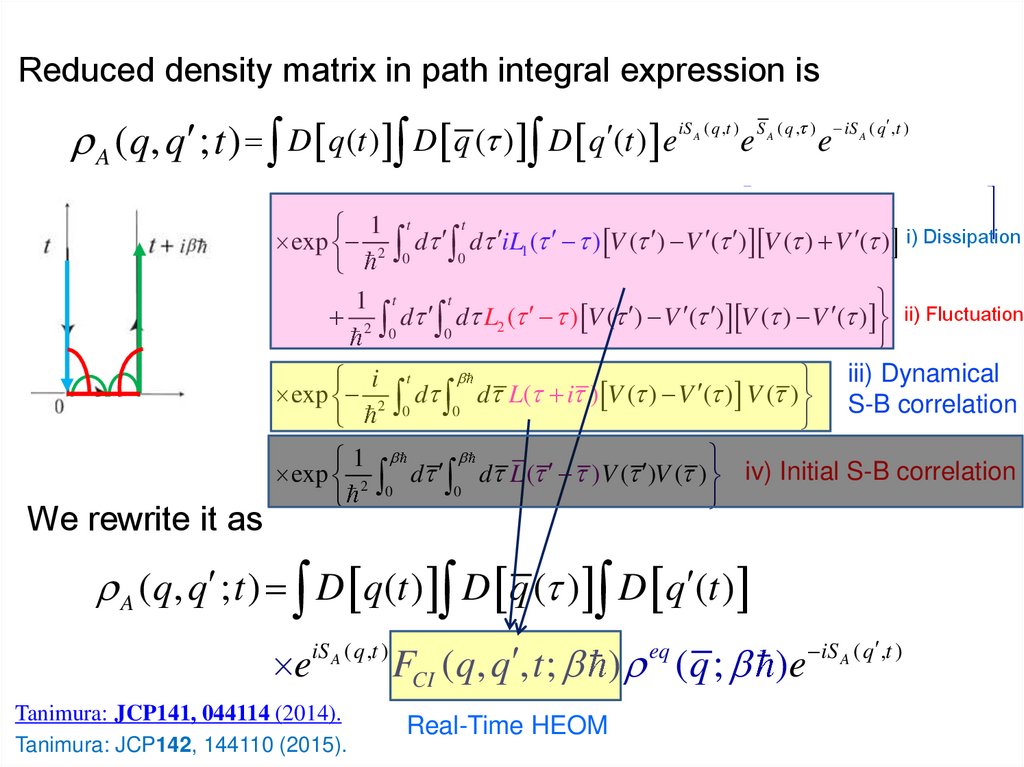
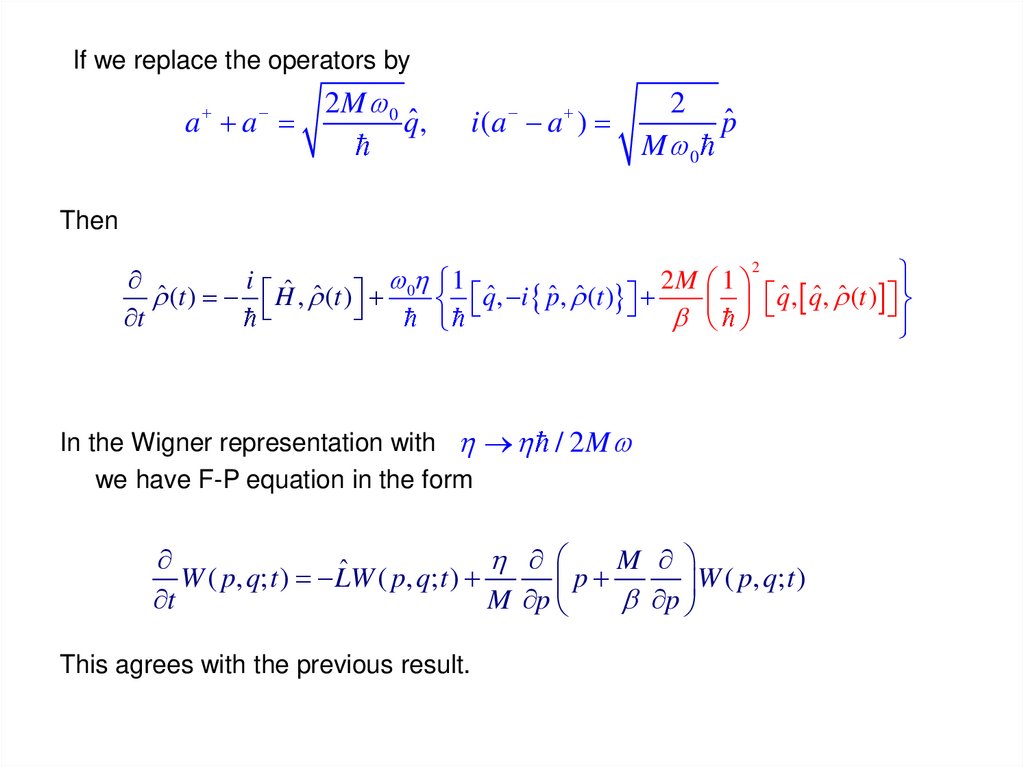
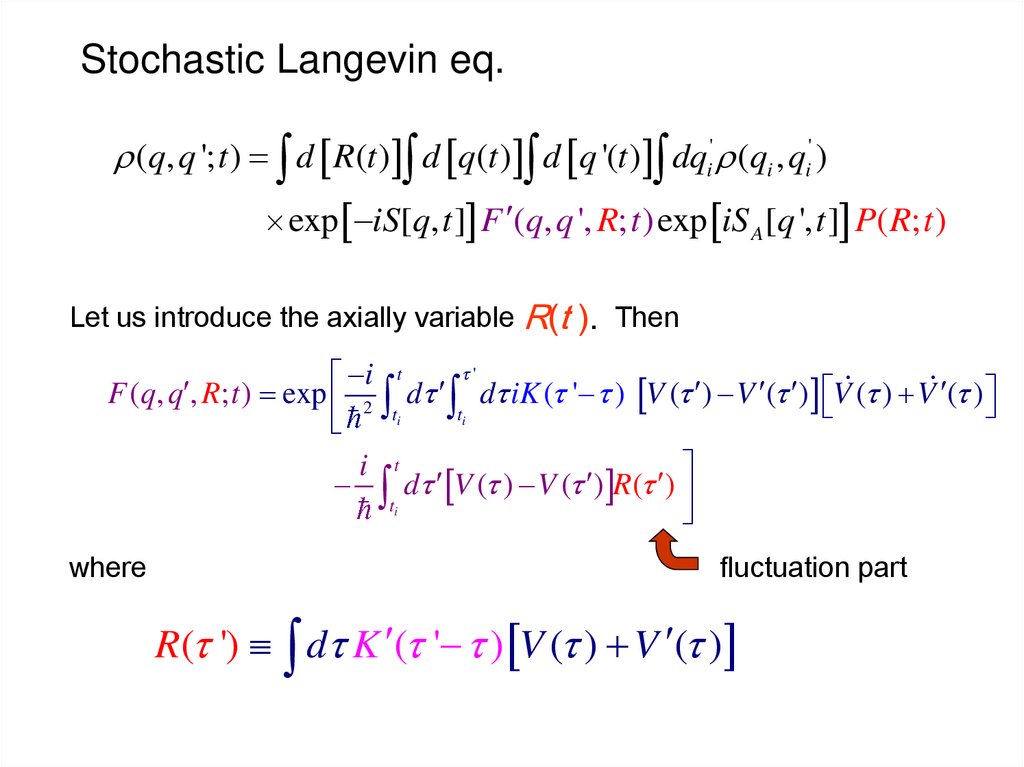
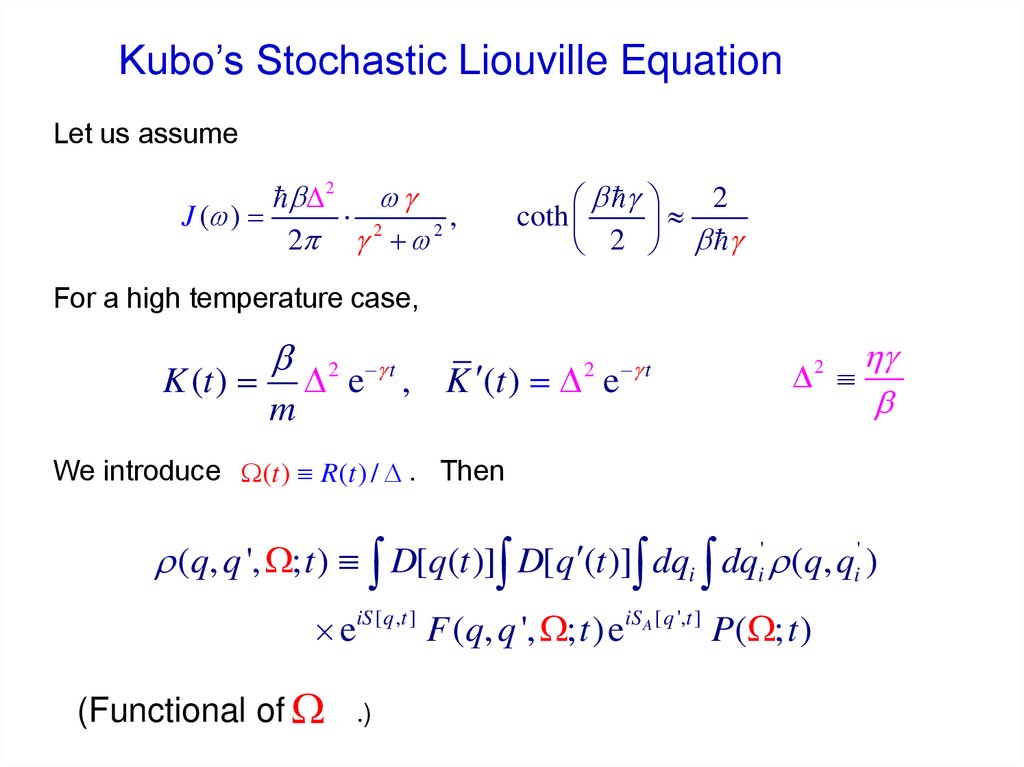
Nonequilibrium statistical physicsFor wave func. A B (q, x, t ), density matrix elements are
ˆ
ˆ † (t )
ˆ A B (t )
(
t
)
A B
A B































































































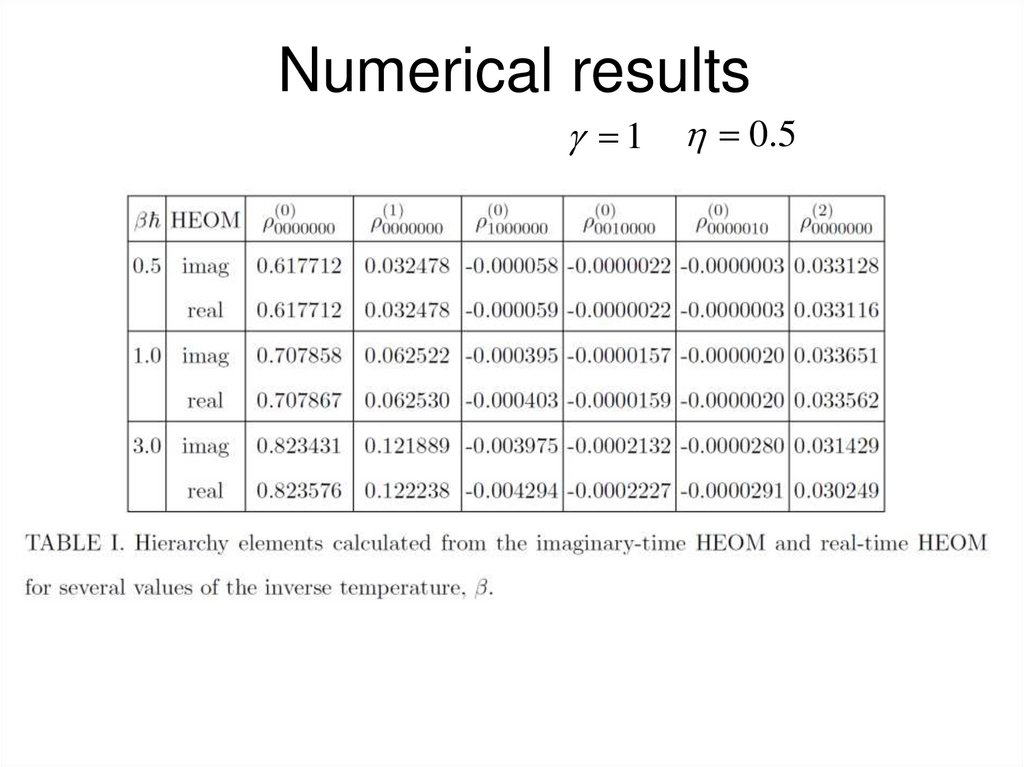
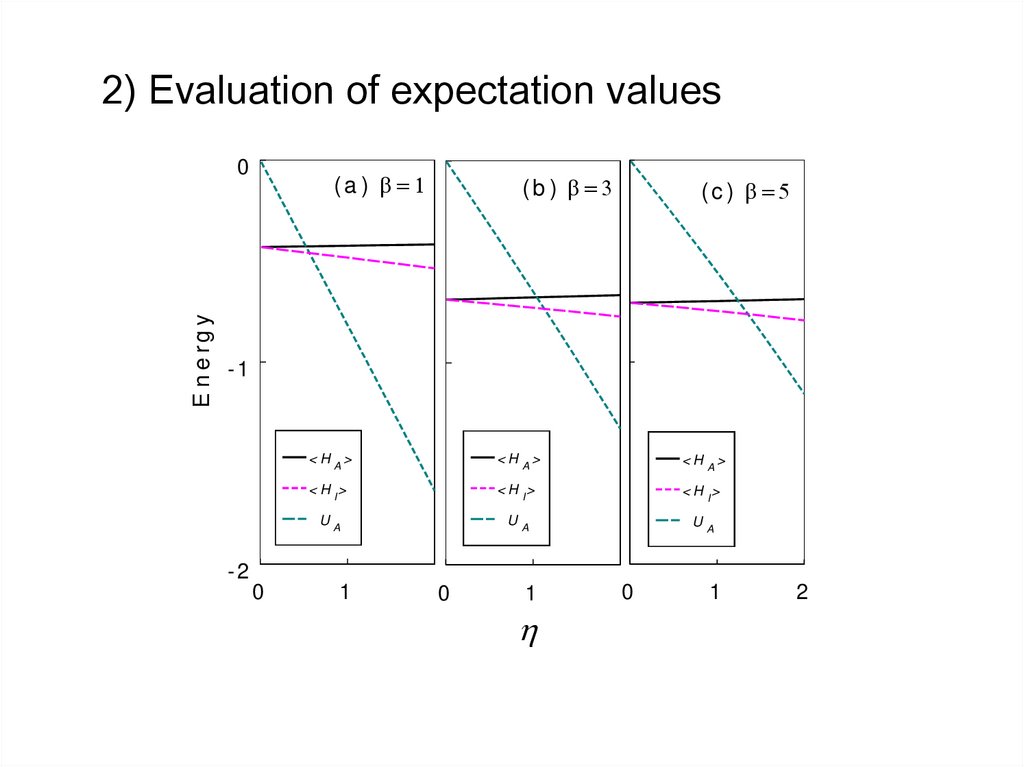
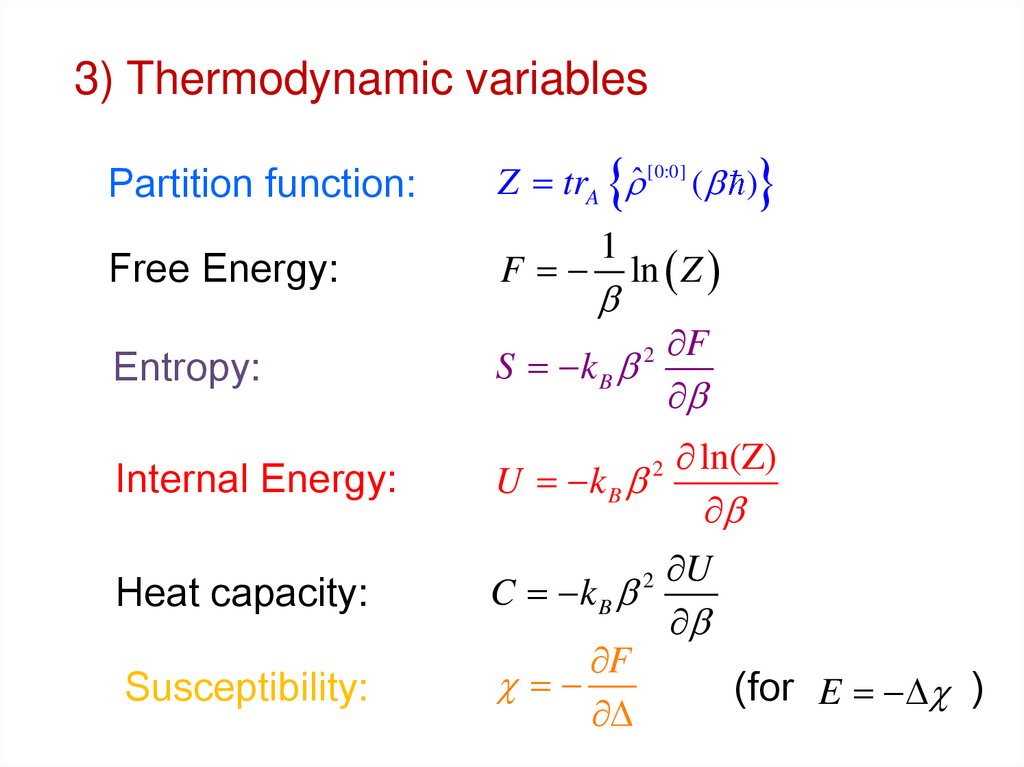




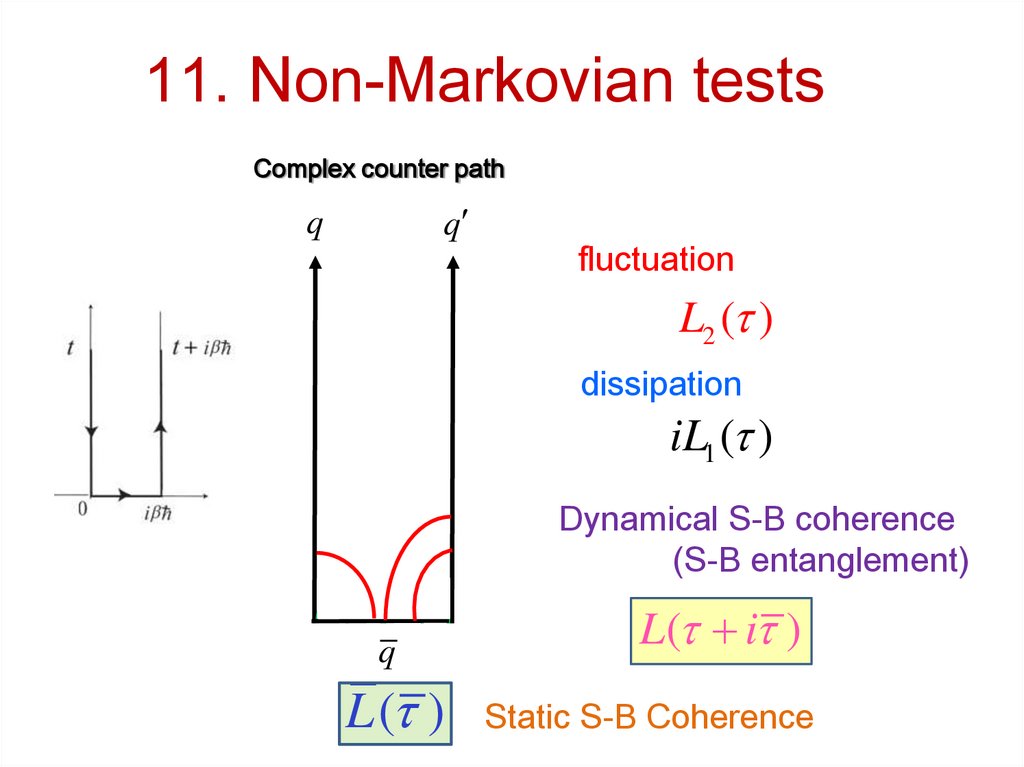











































































 Электроника
Электроника








